“复杂系统生物学”的版本间的差异
| 第5行: | 第5行: | ||
{{multiple issues| | {{multiple issues| | ||
| − | {多个问题 | | + | {多个问题 | |
{{cleanup|reason=Article devolves into lists-of-lists and needs experienced editors to give it focus|date=June 2014}} | {{cleanup|reason=Article devolves into lists-of-lists and needs experienced editors to give it focus|date=June 2014}} | ||
| 第22行: | 第22行: | ||
<font color="#ff8000"> 复杂系统生物学(Complex system biology, CSB)</font>是数学和理论生物学的一个分支或子领域,研究生物有机体结构和功能的复杂性,以及生物和物种的出现与进化,重点研究生物网络及其内部的复杂相互作用,以及对生命至关重要的基本关系和关系模式。因此,CBS是一个理论科学领域,旨在发现和建模生命所必需的关系模式,它只与复杂系统理论有部分重叠,也与生物学的系统方法称为系统生物学;这是因为后者主要局限于生物组织和有机体的简化模型,以及对与生物学复杂性相关的哲学或语义问题的一般性考虑。此外,广泛的抽象理论复杂系统被作为应用数学的一个领域进行研究,无论其是否与生物学、化学或物理相关。 | <font color="#ff8000"> 复杂系统生物学(Complex system biology, CSB)</font>是数学和理论生物学的一个分支或子领域,研究生物有机体结构和功能的复杂性,以及生物和物种的出现与进化,重点研究生物网络及其内部的复杂相互作用,以及对生命至关重要的基本关系和关系模式。因此,CBS是一个理论科学领域,旨在发现和建模生命所必需的关系模式,它只与复杂系统理论有部分重叠,也与生物学的系统方法称为系统生物学;这是因为后者主要局限于生物组织和有机体的简化模型,以及对与生物学复杂性相关的哲学或语义问题的一般性考虑。此外,广泛的抽象理论复杂系统被作为应用数学的一个领域进行研究,无论其是否与生物学、化学或物理相关。 | ||
| + | |||
--[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“以及对生命至关重要的基本关系和关系模式”改为“以及生命所必须的基本关系与关系模式” | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“以及对生命至关重要的基本关系和关系模式”改为“以及生命所必须的基本关系与关系模式” | ||
| + | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“它只与复杂系统理论有部分重叠,也与生物学的系统方法称为系统生物学”改为“它与复杂系统理论以及被称为系统生物学的系统生物学方法只有部分重叠” | ||
| + | |||
[[File:Complex-adaptive-system.jpg|right|thumb|276px|Network Representation of a Complex Adaptive System]] | [[File:Complex-adaptive-system.jpg|right|thumb|276px|Network Representation of a Complex Adaptive System]] | ||
| 第48行: | 第51行: | ||
大多数复杂系统模型通常是根据统计物理学、信息论和非线性动力学的概念来制定的;然而,这些方法并不关注或不包括与组织、拓扑属性或代数拓扑有关的复杂性的概念部分,如非常重要的基因组、交互体和生物有机体的网络连通性。近年来,以信息论、网络拓扑/抽象图论概念为基础的两种互补方法在神经科学和人类认知领域得到了结合。人们普遍认为,组织的复杂程度存在一种层次结构,应与本体论的现实层次相区别。生物圈的复杂层次结构在现代分类等级分类中也得到承认,例如:生物领域和生物圈、生物界、门、生物纲、目、科、属和种。由于生物体具有动态性和组成的可变性、内在的“模糊性”、自生属性、自我繁殖的能力等等,生物体不符合一般系统的“标准”定义,因此它们在功能和结构上都是“超级复杂”的;因此,在CSB中,生物体只能被定义为简单动态系统的“元系统”。这样一个有机体、物种、“生态系统”等等的元系统定义,并不等同于Autopoietic系统理论中对系统中的系统的定义。它也不同于k·d·帕尔默在元系统工程中提出的定义,即生物体不同于具有固定输入输出转换函数的机器和自动机,或不同于具有固定相空间的连续动力系统,这与笛卡尔哲学思想相反;因此,尽管“非确定性自动机”和“模糊自动机”也被定义了,但生物体不能仅仅用五组a(状态、启动状态、输入和输出集/字母、转换函数)来定义。然而,棋盘自动机(tessellation automata)或元胞自动机(cellular automata)提供了一种直观的、可视化的/计算的视角来洞察较低层次的复杂性,因此已经成为一种越来越流行的离散模型,研究领域包括可计算理论、应用数学、物理、计算机科学、理论生物学/系统生物学、癌症模拟和微观结构建模。利用遗传算法实现元胞自动机也是一个新兴的领域,试图在CSB中填补棋盘自动机与更高层次复杂性方法之间的空白。 | 大多数复杂系统模型通常是根据统计物理学、信息论和非线性动力学的概念来制定的;然而,这些方法并不关注或不包括与组织、拓扑属性或代数拓扑有关的复杂性的概念部分,如非常重要的基因组、交互体和生物有机体的网络连通性。近年来,以信息论、网络拓扑/抽象图论概念为基础的两种互补方法在神经科学和人类认知领域得到了结合。人们普遍认为,组织的复杂程度存在一种层次结构,应与本体论的现实层次相区别。生物圈的复杂层次结构在现代分类等级分类中也得到承认,例如:生物领域和生物圈、生物界、门、生物纲、目、科、属和种。由于生物体具有动态性和组成的可变性、内在的“模糊性”、自生属性、自我繁殖的能力等等,生物体不符合一般系统的“标准”定义,因此它们在功能和结构上都是“超级复杂”的;因此,在CSB中,生物体只能被定义为简单动态系统的“元系统”。这样一个有机体、物种、“生态系统”等等的元系统定义,并不等同于Autopoietic系统理论中对系统中的系统的定义。它也不同于k·d·帕尔默在元系统工程中提出的定义,即生物体不同于具有固定输入输出转换函数的机器和自动机,或不同于具有固定相空间的连续动力系统,这与笛卡尔哲学思想相反;因此,尽管“非确定性自动机”和“模糊自动机”也被定义了,但生物体不能仅仅用五组a(状态、启动状态、输入和输出集/字母、转换函数)来定义。然而,棋盘自动机(tessellation automata)或元胞自动机(cellular automata)提供了一种直观的、可视化的/计算的视角来洞察较低层次的复杂性,因此已经成为一种越来越流行的离散模型,研究领域包括可计算理论、应用数学、物理、计算机科学、理论生物学/系统生物学、癌症模拟和微观结构建模。利用遗传算法实现元胞自动机也是一个新兴的领域,试图在CSB中填补棋盘自动机与更高层次复杂性方法之间的空白。 | ||
| + | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“k·d·帕尔默”改为“K·D·帕尔默” | ||
| + | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“生物体只能被定义为简单动态系统的“元系统””改为“生物体只能被定义为一种简单动态系统,“元系统”” | ||
| + | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“并不等同于Autopoietic系统理论“改为”并不等同于自生系统理论“ | ||
| + | --[[用户:Ebteddy|Ebteddy] ([[用户讨论:Ebteddy:|讨论]]) 【审校】“利用遗传算法实现元胞自动机也是一个新兴的领域,试图在CSB中填补棋盘自动机与更高层次复杂性方法之间的空白。”修改为“利用遗传算法实现元胞自动机是一个桥接棋盘自动机和CSB中的高层次复杂性方法之间差距的新兴领域。” | ||
of taxonomic ranks, such as: [[domain (biology)|biological domain]] and biosphere, [[Kingdom (biology)|biological kingdom]], [[Phylum]], [[Class (biology)|biological class]], [[Order (biology)|order]], [[Family (biology)|family]], [[genus]] and [[species]]. Because of their dynamic and composition variability, intrinsic "fuzziness", autopoietic attributes, ability to self-reproduce, and so on, organisms do not fit into the 'standard' definition of general systems, and they are therefore 'super-complex' in both their function and structure; organisms can be thus be defined in CSB only as '[[meta-system]]s' of simpler dynamic systems<ref name="springerlink" /><ref>[http://pespmc1.vub.ac.be/MST.html Metasystem Transition Theory], [[Valentin Turchin]], [[Cliff Joslyn]], 1993-1997</ref> Such a meta-system definition of organisms, species, 'ecosystems', and so on, is not equivalent to the definition of a ''system of systems'' as in [[Autopoiesis|Autopoietic System]]s Theory,;<ref>[http://archonic.net Reflexive Autopoietic Systems Theory]</ref> it also differs from the definition proposed for example by K.D. Palmer in meta-system engineering,<ref>[http://archonic.net/incosewg/ppframe.htm Meta-system Engineering], Kent D. Palmer, 1996</ref> organisms being quite different from machines and [[Automata theory|automata]] with fixed input-output transition functions, or a continuous [[dynamical system]] with fixed [[phase space]],<ref>Hoff, M.A., Roggia, K.G., Menezes, P.B.:(2004). Composition of Transformations: A | of taxonomic ranks, such as: [[domain (biology)|biological domain]] and biosphere, [[Kingdom (biology)|biological kingdom]], [[Phylum]], [[Class (biology)|biological class]], [[Order (biology)|order]], [[Family (biology)|family]], [[genus]] and [[species]]. Because of their dynamic and composition variability, intrinsic "fuzziness", autopoietic attributes, ability to self-reproduce, and so on, organisms do not fit into the 'standard' definition of general systems, and they are therefore 'super-complex' in both their function and structure; organisms can be thus be defined in CSB only as '[[meta-system]]s' of simpler dynamic systems<ref name="springerlink" /><ref>[http://pespmc1.vub.ac.be/MST.html Metasystem Transition Theory], [[Valentin Turchin]], [[Cliff Joslyn]], 1993-1997</ref> Such a meta-system definition of organisms, species, 'ecosystems', and so on, is not equivalent to the definition of a ''system of systems'' as in [[Autopoiesis|Autopoietic System]]s Theory,;<ref>[http://archonic.net Reflexive Autopoietic Systems Theory]</ref> it also differs from the definition proposed for example by K.D. Palmer in meta-system engineering,<ref>[http://archonic.net/incosewg/ppframe.htm Meta-system Engineering], Kent D. Palmer, 1996</ref> organisms being quite different from machines and [[Automata theory|automata]] with fixed input-output transition functions, or a continuous [[dynamical system]] with fixed [[phase space]],<ref>Hoff, M.A., Roggia, K.G., Menezes, P.B.:(2004). Composition of Transformations: A | ||
| 第93行: | 第100行: | ||
* [[Organism]]s and [[species]] relations and evolution | * [[Organism]]s and [[species]] relations and evolution | ||
| − | + | 生物与物种的关系与进化 | |
* [[Intertidal ecology|Interactions among Species]] | * [[Intertidal ecology|Interactions among Species]] | ||
| − | + | 物种间的相互作用 | |
* [[Evolution]] theories and [[population genetics]] | * [[Evolution]] theories and [[population genetics]] | ||
进化论和种群遗传学 | 进化论和种群遗传学 | ||
| 第136行: | 第143行: | ||
{引用期刊 | {引用期刊 | ||
| − | + | | author = Faith, JJ | |
| − | + | | author = Faith, JJ | |
作者 = Faith,JJ | 作者 = Faith,JJ | ||
| − | + | | year = 2007 | |
| − | + | | year = 2007 | |
2007年 | 2007年 | ||
| − | + | | title = Large-Scale Mapping and Validation of Escherichia coli Transcriptional Regulation from a Compendium of Expression Profiles | |
| − | + | | title = Large-Scale Mapping and Validation of Escherichia coli Transcriptional Regulation from a Compendium of Expression Profiles | |
| title = 大比例绘制和验证表达式概要中的大肠桿菌转录调控 | | title = 大比例绘制和验证表达式概要中的大肠桿菌转录调控 | ||
| − | + | | journal = [[PLOS Biology]] | |
| − | + | | journal = PLOS Biology | |
| journal = PLOS Biology | | journal = PLOS Biology | ||
| − | + | | volume = 5 | issue = 1 | pages = 54–66 | |
| − | + | | volume = 5 | issue = 1 | pages = 54–66 | |
5 | issue = 1 | pages = 54-66 | 5 | issue = 1 | pages = 54-66 | ||
| − | + | | doi = 10.1371/journal.pbio.0050008 | |
| − | + | | doi = 10.1371/journal.pbio.0050008 | |
10.1371/journal.pbio. 0050008 | 10.1371/journal.pbio. 0050008 | ||
| − | + | | pmid = 17214507 | |
| − | + | | pmid = 17214507 | |
17214507 | 17214507 | ||
| − | + | | pmc = 1764438 | |
| − | + | | pmc = 1764438 | |
1764438 | 1764438 | ||
| − | + | | display-authors = 1 | |
| − | + | | display-authors = 1 | |
| display-authors = 1 | | display-authors = 1 | ||
| − | + | | last2 = Hayete | |
| − | + | | last2 = Hayete | |
2 = Hayete | 2 = Hayete | ||
| − | + | | first2 = Boris | |
| − | + | | first2 = Boris | |
2 = Boris | 2 = Boris | ||
| − | + | | last3 = Thaden | |
| − | + | | last3 = Thaden | |
3 = Thaden | 3 = Thaden | ||
| − | + | | first3 = Joshua T. | |
| − | + | | first3 = Joshua T. | |
3 = Joshua t. | 3 = Joshua t. | ||
| − | + | | last4 = Mogno | |
| − | + | | last4 = Mogno | |
4 = Mogno | 4 = Mogno | ||
| − | + | | first4 = Ilaria | |
| − | + | | first4 = Ilaria | |
4 = Ilaria | 4 = Ilaria | ||
| − | + | | last5 = Wierzbowski | |
| − | + | | last5 = Wierzbowski | |
5 = Wierzbowski | 5 = Wierzbowski | ||
| − | + | | first5 = Jamey | |
| − | + | | first5 = Jamey | |
5 = Jamey | 5 = Jamey | ||
| − | + | | last6 = Cottarel | |
| − | + | | last6 = Cottarel | |
6 = Cottarel | 6 = Cottarel | ||
| − | + | | first6 = Guillaume | |
| − | + | | first6 = Guillaume | |
6 = Guillaume | 6 = Guillaume | ||
| − | + | | last7 = Kasif | |
| − | + | | last7 = Kasif | |
7 = Kasif | 7 = Kasif | ||
| − | + | | first7 = Simon | |
| − | + | | first7 = Simon | |
7 = Simon | 7 = Simon | ||
| − | + | | last8 = Collins | |
| − | + | | last8 = Collins | |
8 = Collins | 8 = Collins | ||
| − | + | | first8 = James J. | |
| − | + | | first8 = James J. | |
8 = James j. | 8 = James j. | ||
| − | + | | last9 = Gardner | |
| − | + | | last9 = Gardner | |
9 = Gardner | 9 = Gardner | ||
| − | + | | first9 = Timothy S. | |
| − | + | | first9 = Timothy S. | |
9 = Timothy s. | 9 = Timothy s. | ||
| 第290行: | 第297行: | ||
}}</ref><ref name="Hayete2007"> | }}</ref><ref name="Hayete2007"> | ||
| − | } </ref > < ref name ="hayete2007"> | + | } </ref > < ref name ="hayete2007"> |
{{cite journal | {{cite journal | ||
| 第298行: | 第305行: | ||
{引用期刊 | {引用期刊 | ||
| − | + | |author1=Hayete, B |author2=Gardner, TS |author3=Collins, JJ | year = 2007 | |
| − | + | |author1=Hayete, B |author2=Gardner, TS |author3=Collins, JJ | year = 2007 | |
1 = Hayete,b | author2 = Gardner,TS | author3 = Collins,JJ | year = 2007 | 1 = Hayete,b | author2 = Gardner,TS | author3 = Collins,JJ | year = 2007 | ||
| − | + | | title = Size matters: network inference tackles the genome scale | |
| − | + | | title = Size matters: network inference tackles the genome scale | |
| title = 规模问题: 网络推理解决了基因组规模 | | title = 规模问题: 网络推理解决了基因组规模 | ||
| − | + | | journal = [[Molecular Systems Biology]] | |
| − | + | | journal = Molecular Systems Biology | |
分子系统生物学 | 分子系统生物学 | ||
| − | + | | volume = 3 |issue = 1| pages = 77 | |
| − | + | | volume = 3 |issue = 1| pages = 77 | |
3 | issue = 1 | pages = 77 | 3 | issue = 1 | pages = 77 | ||
| − | + | | doi = 10.1038/msb4100118 | |
| − | + | | doi = 10.1038/msb4100118 | |
| doi = 10.1038/msb4100118 | | doi = 10.1038/msb4100118 | ||
| − | + | | pmid = 17299414 | |
| − | + | | pmid = 17299414 | |
17299414 | 17299414 | ||
| − | + | | pmc = 1828748 | |
| − | + | | pmc = 1828748 | |
1828748 | 1828748 | ||
| 第344行: | 第351行: | ||
}}</ref> | }}</ref> | ||
| − | } </ref > | + | } </ref > |
* [[Cell signaling]] | * [[Cell signaling]] | ||
2020年9月14日 (一) 00:40的版本
已由吕倩倩初步翻译
}}
Complex systems biology (CSB) is a branch or subfield of mathematical and theoretical biology concerned with complexity of both structure and function in biological organisms, as well as the emergence and evolution of organisms and species, with emphasis being placed on the complex interactions of, and within, bionetworks,[1] and on the fundamental relations and relational patterns that are essential to life.[2][3][4][5][6] CSB is thus a field of theoretical sciences aimed at discovering and modeling the relational patterns essential to life that has only a partial overlap with complex systems theory,[7] and also with the systems approach to biology called systems biology; this is because the latter is restricted primarily to simplified models of biological organization and organisms, as well as to only a general consideration of philosophical or semantic questions related to complexity in biology.[citation needed] Moreover, a wide range of abstract theoretical complex systems are studied as a field of applied mathematics, with or without relevance to biology, chemistry or physics.
Complex systems biology (CSB) is a branch or subfield of mathematical and theoretical biology concerned with complexity of both structure and function in biological organisms, as well as the emergence and evolution of organisms and species, with emphasis being placed on the complex interactions of, and within, bionetworks, and on the fundamental relations and relational patterns that are essential to life. CSB is thus a field of theoretical sciences aimed at discovering and modeling the relational patterns essential to life that has only a partial overlap with complex systems theory, and also with the systems approach to biology called systems biology; this is because the latter is restricted primarily to simplified models of biological organization and organisms, as well as to only a general consideration of philosophical or semantic questions related to complexity in biology. Moreover, a wide range of abstract theoretical complex systems are studied as a field of applied mathematics, with or without relevance to biology, chemistry or physics.
复杂系统生物学(Complex system biology, CSB)是数学和理论生物学的一个分支或子领域,研究生物有机体结构和功能的复杂性,以及生物和物种的出现与进化,重点研究生物网络及其内部的复杂相互作用,以及对生命至关重要的基本关系和关系模式。因此,CBS是一个理论科学领域,旨在发现和建模生命所必需的关系模式,它只与复杂系统理论有部分重叠,也与生物学的系统方法称为系统生物学;这是因为后者主要局限于生物组织和有机体的简化模型,以及对与生物学复杂性相关的哲学或语义问题的一般性考虑。此外,广泛的抽象理论复杂系统被作为应用数学的一个领域进行研究,无论其是否与生物学、化学或物理相关。
--[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“以及对生命至关重要的基本关系和关系模式”改为“以及生命所必须的基本关系与关系模式” --[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“它只与复杂系统理论有部分重叠,也与生物学的系统方法称为系统生物学”改为“它与复杂系统理论以及被称为系统生物学的系统生物学方法只有部分重叠”
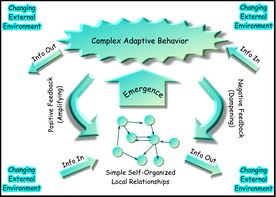
Network Representation of a Complex Adaptive System
一个复杂自适应系统的网络表示
Complexity of organisms and biosphere
生物体和生物圈的复杂性
A complete definition of complexity for individual organisms, species, ecosystems, biological evolution and the biosphere has eluded researchers, and still is an ongoing issue.[3][8]
A complete definition of complexity for individual organisms, species, ecosystems, biological evolution and the biosphere has eluded researchers, and still is an ongoing issue.right
单个生物体、物种、生态系统、生物进化和生物圈的复杂性的完整定义一直困扰着研究人员,而且仍然是一个悬而未决的问题。
Most complex system models are often formulated in terms of concepts drawn from statistical physics, information theory and non-linear dynamics; however, such approaches are not focused on, or do not include, the conceptual part of complexity related to organization and topological attributes or algebraic topology, such as network connectivity of genomes, interactomes and biological organisms that are very important.[6][9][10] Recently, the two complementary approaches based both on information theory, network topology/abstract graph theory concepts are being combined for example in the fields of neuroscience and human cognition.[7][11] It is generally agreed that there is a hierarchy of complexity levels of organization that should be considered as distinct from that of the levels of reality in ontology.[7][12][13] The hierarchy of complexity levels of organization in the biosphere is also recognized in modern classifications
Most complex system models are often formulated in terms of concepts drawn from statistical physics, information theory and non-linear dynamics; however, such approaches are not focused on, or do not include, the conceptual part of complexity related to organization and topological attributes or algebraic topology, such as network connectivity of genomes, interactomes and biological organisms that are very important. Recently, the two complementary approaches based both on information theory, network topology/abstract graph theory concepts are being combined for example in the fields of neuroscience and human cognition. It is generally agreed that there is a hierarchy of complexity levels of organization that should be considered as distinct from that of the levels of reality in ontology. The hierarchy of complexity levels of organization in the biosphere is also recognized in modern classifications
大多数复杂系统模型通常是根据统计物理学、信息论和非线性动力学的概念来制定的;然而,这些方法并不关注或不包括与组织、拓扑属性或代数拓扑有关的复杂性的概念部分,如非常重要的基因组、交互体和生物有机体的网络连通性。近年来,以信息论、网络拓扑/抽象图论概念为基础的两种互补方法在神经科学和人类认知领域得到了结合。人们普遍认为,组织的复杂程度存在一种层次结构,应与本体论的现实层次相区别。生物圈的复杂层次结构在现代分类等级分类中也得到承认,例如:生物领域和生物圈、生物界、门、生物纲、目、科、属和种。由于生物体具有动态性和组成的可变性、内在的“模糊性”、自生属性、自我繁殖的能力等等,生物体不符合一般系统的“标准”定义,因此它们在功能和结构上都是“超级复杂”的;因此,在CSB中,生物体只能被定义为简单动态系统的“元系统”。这样一个有机体、物种、“生态系统”等等的元系统定义,并不等同于Autopoietic系统理论中对系统中的系统的定义。它也不同于k·d·帕尔默在元系统工程中提出的定义,即生物体不同于具有固定输入输出转换函数的机器和自动机,或不同于具有固定相空间的连续动力系统,这与笛卡尔哲学思想相反;因此,尽管“非确定性自动机”和“模糊自动机”也被定义了,但生物体不能仅仅用五组a(状态、启动状态、输入和输出集/字母、转换函数)来定义。然而,棋盘自动机(tessellation automata)或元胞自动机(cellular automata)提供了一种直观的、可视化的/计算的视角来洞察较低层次的复杂性,因此已经成为一种越来越流行的离散模型,研究领域包括可计算理论、应用数学、物理、计算机科学、理论生物学/系统生物学、癌症模拟和微观结构建模。利用遗传算法实现元胞自动机也是一个新兴的领域,试图在CSB中填补棋盘自动机与更高层次复杂性方法之间的空白。
--[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“k·d·帕尔默”改为“K·D·帕尔默” --[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“生物体只能被定义为简单动态系统的“元系统””改为“生物体只能被定义为一种简单动态系统,“元系统”” --[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“并不等同于Autopoietic系统理论“改为”并不等同于自生系统理论“ --[[用户:Ebteddy|Ebteddy] (讨论) 【审校】“利用遗传算法实现元胞自动机也是一个新兴的领域,试图在CSB中填补棋盘自动机与更高层次复杂性方法之间的空白。”修改为“利用遗传算法实现元胞自动机是一个桥接棋盘自动机和CSB中的高层次复杂性方法之间差距的新兴领域。”
of taxonomic ranks, such as: biological domain and biosphere, biological kingdom, Phylum, biological class, order, family, genus and species. Because of their dynamic and composition variability, intrinsic "fuzziness", autopoietic attributes, ability to self-reproduce, and so on, organisms do not fit into the 'standard' definition of general systems, and they are therefore 'super-complex' in both their function and structure; organisms can be thus be defined in CSB only as 'meta-systems' of simpler dynamic systems[7][14] Such a meta-system definition of organisms, species, 'ecosystems', and so on, is not equivalent to the definition of a system of systems as in Autopoietic Systems Theory,;[15] it also differs from the definition proposed for example by K.D. Palmer in meta-system engineering,[16] organisms being quite different from machines and automata with fixed input-output transition functions, or a continuous dynamical system with fixed phase space,引用错误:没有找到与</ref>对应的<ref>标签 contrary to the Cartesian philosophical thinking; thus, organisms cannot be defined merely in terms of a quintuple A of (states, startup state, input and output sets/alphabet, transition function),[17] although 'non-deterministic automata', as well as 'fuzzy automata' have also been defined. Tessellation or cellular automata provide however an intuitive, visual/computational insight into the lower levels of complexity, and have therefore become an increasingly popular, discrete model studied in computability theory, applied mathematics, physics, computer science, theoretical biology/systems biology, cancer simulations and microstructure modeling. Evolving cellular automata using genetic algorithms[18][19] contrary to the Cartesian philosophical thinking; thus, organisms cannot be defined merely in terms of a quintuple A of (states, startup state, input and output sets/alphabet, transition function), although 'non-deterministic automata', as well as 'fuzzy automata' have also been defined. Tessellation or cellular automata provide however an intuitive, visual/computational insight into the lower levels of complexity, and have therefore become an increasingly popular, discrete model studied in computability theory, applied mathematics, physics, computer science, theoretical biology/systems biology, cancer simulations and microstructure modeling. Evolving cellular automata using genetic algorithms[20][21] is also an emerging field attempting to bridge the gap between the tessellation automata and the higher level complexity approaches in CSB.
Evolving Cellular Automata with Genetic Algorithms: A Review of Recent Work, Melanie Mitchell, James P. Crutchfeld, Rajarshi Das (In Proceedings of the First International Conference on Evolutionary Computation and Its Applications (EvCA'96). Moscow, Russia: Russian Academy of Sciences, 1996.)</ref> is also an emerging field attempting to bridge the gap between the tessellation automata and the higher level complexity approaches in CSB.
Topics in complex systems biology
复杂系统生物学的主题
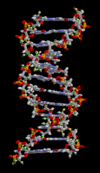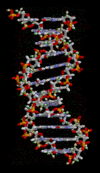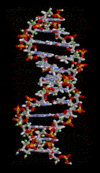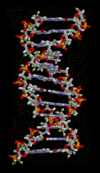
Animated Molecular Model of a DNA double helix
一个DNA双螺旋的动画分子模型
Telomerase structure and function
端粒酶的结构与功能
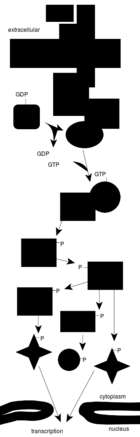
A Complex Signal Transduction Pathway
一个复杂的信号转导途径
The following is only a partial list of topics covered in complex systems biology:
The following is only a partial list of topics covered in complex systems biology:
以下只是复杂系统生物学所涵盖的部分主题列表:
生物与物种的关系与进化
物种间的相互作用
- Evolution theories and population genetics
进化论和种群遗传学
- Population genetics models
群体遗传学模型
表观遗传学
- Molecular evolution theories
分子进化理论
量子生物计算学
量子遗传
关系生物学
- Self-reproduction[26] (also called self-replication in a more general context)
自我繁殖(在更一般的情况下也称为自我复制)
计算基因模型
DNA拓扑学
DNA测序理论
进化发育生物学
自我生产
蛋白质折叠
- Telomerase conformations and functions in vivo
端粒酶在体内的构象和功能
表观遗传学
- Interactomics[27][28][28] < ref name ="hayete2007">
{{cite journal
{{cite journal
{引用期刊
|author1=Hayete, B |author2=Gardner, TS |author3=Collins, JJ | year = 2007
|author1=Hayete, B |author2=Gardner, TS |author3=Collins, JJ | year = 2007
1 = Hayete,b | author2 = Gardner,TS | author3 = Collins,JJ | year = 2007
| title = Size matters: network inference tackles the genome scale
| title = Size matters: network inference tackles the genome scale
| title = 规模问题: 网络推理解决了基因组规模
| journal = Molecular Systems Biology
| journal = Molecular Systems Biology
分子系统生物学
| volume = 3 |issue = 1| pages = 77
| volume = 3 |issue = 1| pages = 77
3 | issue = 1 | pages = 77
| doi = 10.1038/msb4100118
| doi = 10.1038/msb4100118
| doi = 10.1038/msb4100118
| pmid = 17299414
| pmid = 17299414
17299414
| pmc = 1828748
| pmc = 1828748
1828748
}}</ref>
}}</ref>
} </ref >
细胞信号传导
信号转导网络
复杂神经网络
基因网络
形态发生
数字形态发生
复杂自适应系统
形态形成的拓扑模型
渔业种群动态
流行病学
理论生态学
- Immune system
免疫系统
See also
另请参阅 模板:Portal
数学和理论生物学
抽象关系生物学
复杂
复杂系统
生物系统
系统理论
动力系统
动力系统理论
自动机理论
元胞自动机
系统生物学
人类学中的系统理论
自组织
非线性度
生成科学
紧急情况
生物圈
脱氧核糖核酸
量子生物学
量子遗传学
量子生物化学
量子化学
量子分子动力学
蛋白质折叠
交互组学
基因组学
蛋白质组学
表观遗传学
数字形态
复杂自适应系统
多代理系统
认知科学
面向模式的建模
波动性,不确定性,复杂性和歧义性
蓝色基因
折叠@家
端粒酶
生命是什么
Biographies
传记 模板:Colbegin
Notes
笔记
- ↑ Sprites, P; Glymour, C; Scheines, R (2000). Causation, Prediction, and Search: Adaptive Computation and Machine Learning (2nd ed.). MIT Press.
- ↑ Donald Snooks, Graeme, "A general theory of complex living systems: Exploring the demand side of dynamics", Complexity, vol. 13, no. 6, July/August 2008.
- ↑ 3.0 3.1 Bonner, J. T. 1988. The Evolution of Complexity by Means of Natural Selection. Princeton: Princeton University Press.
- ↑ 4.0 4.1 Rosen, R. (1958a). "A Relational Theory of Biological Systems". Bulletin of Mathematical Biophysics. 20 (3): 245–260. doi:10.1007/bf02478302.
- ↑ Baianu, I. C. (2006). "Robert Rosen's Work and Complex Systems Biology". Axiomathes. 16 (1–2): 25–34. doi:10.1007/s10516-005-4204-z.
- ↑ 6.0 6.1 Rosen, R. (1958b). "The Representation of Biological Systems from the Standpoint of the Theory of Categories". Bulletin of Mathematical Biophysics. 20 (4): 317–341. doi:10.1007/bf02477890.
- ↑ 7.0 7.1 7.2 7.3 Baianu, I. C.; Brown, R.; Glazebrook, J. F. (2007). "Categorical Ontology of Complex Spacetime Structures: The Emergence of Life and Human Consciousness". Axiomathes. 17 (3–4): 223–352. CiteSeerX 10.1.1.145.9486. doi:10.1007/s10516-007-9011-2.
- ↑ Heylighen, Francis (2008). "Complexity and Self-Organization". In Bates, Marcia J.; Maack, Mary Niles. Encyclopedia of Library and Information Sciences. CRC.
- ↑ ^ Heylighen, Francis (2008). "Complexity and Self-Organization". In Bates, Marcia J.; Maack, Mary Niles. Encyclopedia of Library and Information Sciences. CRC.
- ↑ "abstract relational biology (ARB)". PlanetPhysics. Retrieved 2010-03-17.
- ↑ http://hdl.handle.net/10101/npre.2011.6115.1 Wallace, Rodrick. When Spandrels Become Arches: Neural crosstalk and the evolution of consciousness. Available from Nature Precedings (2011)
- ↑ Poli R (2001a). "The Basic Problem of the Theory of Levels of Reality". Axiomathes. 12 (3–4): 261–283. doi:10.1023/A:1015845217681.
- ↑ Poli R (1998). "Levels". Axiomathes. 9 (1–2): 197–211. doi:10.1007/bf02681712. PMID 8053082.
- ↑ Metasystem Transition Theory, Valentin Turchin, Cliff Joslyn, 1993-1997
- ↑ Reflexive Autopoietic Systems Theory
- ↑ Meta-system Engineering, Kent D. Palmer, 1996
- ↑ John E. Hopcroft, Rajeev Motwani, Jeffrey D. Ullman.2000. Introduction to Automata Theory, Languages, and Computation (2nd Edition)Pearson Education.
- ↑ The Evolutionary Design of Collective Computation in Cellular Automata, James P. Crutchfeld, Melanie Mitchell, Rajarshi Das (In J. P. Crutchfield and P. K. Schuster (editors), Evolutionary Dynamics|Exploring the Interplay of Selection, Neutrality, Accident, and Function. New York: Oxford University Press, 2002.)
- ↑ Framework for Systems with Dynamic Topology. International Journal of Computing Anticipatory System's 14:259–270
- ↑ Evolving Cellular Automata with Genetic Algorithms: A Review of Recent Work, Melanie Mitchell, James P. Crutchfeld, Rajarshi Das (In Proceedings of the First International Conference on Evolutionary Computation and Its Applications (EvCA'96). Moscow, Russia: Russian Academy of Sciences, 1996.)
- ↑ Peak, West; Messinger, Mott; Messinger, SM; Mott, KA (2004). "Evidence for complex, collective dynamics and emergent, distributed computation in plants". Proceedings of the National Academy of Sciences of the USA. 101 (4): 918–922. Bibcode:2004PNAS..101..918P. doi:10.1073/pnas.0307811100. PMC 327117. PMID 14732685.
- ↑ Rosen, R. 1960. (1960). "A quantum-theoretic approach to genetic problems". Bulletin of Mathematical Biophysics. 22 (3): 227–255. doi:10.1007/BF02478347.
- ↑ Baianu, I. C.: 2006 (2006). "Robert Rosen's Work and Complex Systems Biology". Axiomathes. 16 (1–2): 25–34. doi:10.1007/s10516-005-4204-z.
- ↑ Rosen, R.: 1958b (1958). "The Representation of Biological Systems from the Standpoint of the Theory of Categories". Bulletin of Mathematical Biophysics. 20 (4): 317–341. doi:10.1007/BF02477890.
- ↑ PlanetMath
- ↑ "PlanetMath". PlanetMath. Retrieved 2010-03-17.
- ↑ 27.0 27.1 交互组学 {{cite journal {{cite journal {引用期刊 | author = Faith, JJ | author = Faith, JJ 作者 = Faith,JJ | year = 2007 | year = 2007 2007年 | title = Large-Scale Mapping and Validation of Escherichia coli Transcriptional Regulation from a Compendium of Expression Profiles | title = Large-Scale Mapping and Validation of Escherichia coli Transcriptional Regulation from a Compendium of Expression Profiles | title = 大比例绘制和验证表达式概要中的大肠桿菌转录调控 | journal = PLOS Biology | journal = PLOS Biology | journal = PLOS Biology | volume = 5 | issue = 1 | pages = 54–66 | volume = 5 | issue = 1 | pages = 54–66 5 | issue = 1 | pages = 54-66 | doi = 10.1371/journal.pbio.0050008 | doi = 10.1371/journal.pbio.0050008 10.1371/journal.pbio. 0050008 | pmid = 17214507 | pmid = 17214507 17214507 | pmc = 1764438 | pmc = 1764438 1764438 | display-authors = 1 | display-authors = 1 | display-authors = 1 | last2 = Hayete | last2 = Hayete 2 = Hayete | first2 = Boris | first2 = Boris 2 = Boris | last3 = Thaden | last3 = Thaden 3 = Thaden | first3 = Joshua T. | first3 = Joshua T. 3 = Joshua t. | last4 = Mogno | last4 = Mogno 4 = Mogno | first4 = Ilaria | first4 = Ilaria 4 = Ilaria | last5 = Wierzbowski | last5 = Wierzbowski 5 = Wierzbowski | first5 = Jamey | first5 = Jamey 5 = Jamey | last6 = Cottarel | last6 = Cottarel 6 = Cottarel | first6 = Guillaume | first6 = Guillaume 6 = Guillaume | last7 = Kasif | last7 = Kasif 7 = Kasif | first7 = Simon | first7 = Simon 7 = Simon | last8 = Collins | last8 = Collins 8 = Collins | first8 = James J. | first8 = James J. 8 = James j. | last9 = Gardner | last9 = Gardner 9 = Gardner | first9 = Timothy S. | first9 = Timothy S. 9 = Timothy s. }}
- ↑ 28.0 28.1 28.2
}} 引用错误:无效
<ref>标签;name属性“Hayete2007”使用不同内容定义了多次
References cited
参考文献引用
- Ahmed, E. (2006). "On modeling the immune system as a complex system". Theor. BioSci. 124 (3–4): 413–8. arXiv:0801.0847. Bibcode:2008arXiv0801.0847A. doi:10.1016/j.thbio.2005.07.001. PMID 17046369.
- Baianu, I. C., Computer Models and Automata Theory in Biology and Medicine., Monograph, Ch.11 in M. Witten (Editor), Mathematical Models in Medicine, vol. 7., Vol. 7: 1513-1577 (1987),Pergamon Press:New York, (updated by Hsiao Chen Lin in 2004
- Bonner, J. T. 1988. The Evolution of Complexity by Means of Natural Selection. Princeton: Princeton University Press.
- Donald Snooks, Graeme, "A general theory of complex living systems: Exploring the demand side of dynamics", Complexity, vol. 13, no. 6, July/August 2008.
- Drazin, P.G., Nonlinear systems. C.U.P., 1992.
- Edelstein-Keshet, L., Mathematical Models in Biology. SIAM, 2004.
- Forgacs, G.; S. A. Newman, Biological Physics of the Developing Embryo. C.U.P., 2005.
- Israel G (1988). "On the contribution of Volterra and Lotka to the development of modern biomathematics". History and Philosophy of the Life Sciences. 10 (1): 37–49. PMID 3045853.
- Israel, G., 2005, "Book on mathematical biology" in Grattan-Guinness, I., ed., Landmark Writings in Western Mathematics. Elsevier: 936-44.
- Jordan, D.W.; Smith, P., Nonlinear ordinary differential equations, 2nd ed. O.U.P., 1987.
- Kampen, N.G. van. Stochastic Processes in Physics and Chemistry, North Holland., 3rd ed. 2001,
- Murray, J.D., Mathematical Biology. Springer-Verlag, 3rd ed. in 2 vols.: Mathematical Biology: I. An Introduction, 2002 ; Mathematical Biology: II. Spatial Models and Biomedical Applications, 2003 .
- Nicolas Rashevsky. (1938)., Mathematical Biophysics. Chicago: University of Chicago Press.
- Preziosi, L., Cancer Modelling and Simulation. Chapman Hall/CRC Press, 2003. .
- Renshaw, E., Modelling biological populations in space and time. C.U.P., 1991.
- Rosen, Robert.1991, Life Itself: A Comprehensive Inquiry into the Nature, Origin, and Fabrication of Life, Columbia University Press, published posthumously:
- Rosen, Robert .1970. Dynamical system theory in biology. New York, Wiley-Interscience.
- Rosen, Robert. 2000, Essays on Life Itself, Columbia University Press.
- Rosen, Robert. 2003, "Anticipatory Systems; Philosophical, Mathematical, and Methodolical Foundations", Rosen Enterprises publs.
- Rubinow, S.I., Introduction to mathematical biology. John Wiley, 1975.
- Scudo FM (March 1971). "Vito Volterra and theoretical ecology". Theoretical Population Biology. 2 (1): 1–23. doi:10.1016/0040-5809(71)90002-5. PMID 4950157.
- Segel, L.A., Modeling dynamic phenomena in molecular and cellular biology. C.U.P., 1984. .
- Strogatz, S.H., Nonlinear dynamics and Chaos: Applications to Physics, Biology, Chemistry, and Engineering. Perseus, 2001,
- Thompson, D'Arcy W., 1992. On Growth and Form. Dover reprint of 1942, 2nd ed. (1st ed., 1917).
Further reading
进一步阅读
- Drawing the Line Between Theoretical and Basic Biology, a forum article by Isidro A. T. Savillo
- Kurata, Hiroyuki; Taira, K; Kitano, H (1999). "Synthesis and Analysis of a Biological System". Genome Informatics Series (Sers 10): 352–353. OCLC 203735966.
Category:Mathematical and theoretical biology
类别: 数学和理论生物学
Category:Bioinformatics
类别: 生物信息学
Category:Epidemiology
类别: 流行病学
Category:Biostatistics
类别: 生物统计学
Category:Biotechnology
类别: 生物技术
Category:Complex systems theory
范畴: 复杂系统理论
Category:Systems science
类别: 系统科学
This page was moved from wikipedia:en:Complex systems biology. Its edit history can be viewed at 复杂系统生物学/edithistory
- Thompson, D'Arcy W., 1992. On Growth and Form. Dover reprint of 1942, 2nd ed. (1st ed., 1917).
- Strogatz, S.H., Nonlinear dynamics and Chaos: Applications to Physics, Biology, Chemistry, and Engineering. Perseus, 2001,
- Renshaw, E., Modelling biological populations in space and time. C.U.P., 1991.
- Murray, J.D., Mathematical Biology. Springer-Verlag, 3rd ed. in 2 vols.: Mathematical Biology: I. An Introduction, 2002 ; Mathematical Biology: II. Spatial Models and Biomedical Applications, 2003 .
- Kampen, N.G. van. Stochastic Processes in Physics and Chemistry, North Holland., 3rd ed. 2001,
- Forgacs, G.; S. A. Newman, Biological Physics of the Developing Embryo. C.U.P., 2005.
- Edelstein-Keshet, L., Mathematical Models in Biology. SIAM, 2004.