“热力学第二定律”的版本间的差异
| 第21行: | 第21行: | ||
'''热力学第二定律'''也是热力学的四条基本定律之一,它表述了热力学过程的不可逆性——孤立系统自发地朝着热力学平衡方向(即最大熵状态)演化,另一种表述为:第二类永动机永不可能实现。 | '''热力学第二定律'''也是热力学的四条基本定律之一,它表述了热力学过程的不可逆性——孤立系统自发地朝着热力学平衡方向(即最大熵状态)演化,另一种表述为:第二类永动机永不可能实现。 | ||
| − | The second law has been expressed in many ways. Its first formulation is credited to the French scientist Sadi Carnot, who in 1824 showed that there is an upper limit to the efficiency of conversion of heat to work in a heat engine. This aspect of the second law is often named after Carnot. | + | The second law has been expressed in many ways. Its first formulation is credited to the French scientist Sadi Carnot, who in 1824 showed that there is an upper limit to the efficiency of conversion of heat to work in a heat engine. This aspect of the second law is often named after Carnot. |
| − | |||
==Introduction引言== | ==Introduction引言== | ||
2020年10月19日 (一) 09:42的版本
--嘉树(讨论) 全文审校中,heat一般统一译为“热”,caloric 或者 caloric of heat 译为“热量”。这一点是否准确,有待各位同学和专家的批评指正
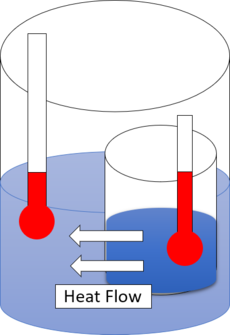
热力学第二定律 Second Law Of Thermodynamics指出,孤立系统 Isolated System的总熵永远不会随着时间而减少,且当且仅当所有过程都是可逆时,总熵才恒定。[1]孤立系统自发地到达到热力学平衡状态,此时为熵最大的状态。
系统及其周围环境的总熵在理想情况下可以保持不变,在这种情况下,系统处于热力学平衡状态,或者正在经历一个假想的可逆过程。所有过程中,包括自发过程 Spontaneous Processes,es,[2] 系统及其周围环境的总熵增加,这一过程在热力学意义上是不可逆的。熵的增加解释了自然过程的不可逆性,以及未来和过去之间的不对称性[3]
从历史上看,第二定律是一个经验性的发现,它被认为是热力学理论中的公理。经典统计力学、量子统计力学都可以解释这个定律的微观起源。
热力学第二定律可以使用多种方法表述。它的第一个公式归功于法国科学家卡诺 Sadi Carnot,卡诺在1824年证明了在热机中将热转化为功的效率有一个上限。第二定律的这个方面也被称为卡诺规则 Carnot's Rule或卡诺限制 Carnot's Limit。[4]
热力学第二定律也是热力学的四条基本定律之一,它表述了热力学过程的不可逆性——孤立系统自发地朝着热力学平衡方向(即最大熵状态)演化,另一种表述为:第二类永动机永不可能实现。
The second law has been expressed in many ways. Its first formulation is credited to the French scientist Sadi Carnot, who in 1824 showed that there is an upper limit to the efficiency of conversion of heat to work in a heat engine. This aspect of the second law is often named after Carnot.
Introduction引言
热力学第一定律 First Law Of Thermodynamics定义了热力学系统所涉及到的内能 Internal Energy,[5][6]并体现了能量守恒定律。热力学第二定律与自然过程Natural Processes[7]的方向有关。它断言自然过程只在一种意义上进行,且不可逆 。例如,当有了传导和辐射的(传播)路径时,热总是自发地从一个较热的物体流向一个较冷的物体。 这种现象可以用[8][9] 熵Entropy来解释。
--大白(讨论)这里是不是少了一段原文?
若一个孤立系统Isolated System最初在具有隔热内壁的系统内维持热力学平衡,通过一些操作使内壁透热,则该系统可自发地演变,最终达到一个新的内部热力学平衡,且其总熵S增加。If an isolated system is held initially in internal thermodynamic equilibrium by internal partitioning impermeable walls, and then some operation makes the walls more permeable, then the system spontaneously evolves to reach a final new internal thermodynamic equilibrium, and its total entropy, S, increases.
在假想的可逆过程中,系统的熵的无穷小增量[math]\displaystyle{ dS }[/math]被定义为由无穷小的热[math]\displaystyle{ δQ }[/math]传递到一个封闭系统(允许能量进入或出去,但不允许物质传递的系统)除以该平衡系统和提供热的环境的共同温度 [math]\displaystyle{ T }[/math]:[10]
- [math]\displaystyle{ \mathrm dS = \frac{\delta Q}{T} }[/math] system, actually possible, irreversible process 封闭系统中理想状态下的可逆过程).}</math>
用不同的符号δ和d表示无穷小量的热和无穷小量的熵,因为熵是状态函数,而热和功一样,并不是状态函数。对于实际上可能存在的不与环境发生物质交换的无穷小过程,第二定律要求系统熵增量满足不等式: [11][12]
- [math]\displaystyle{ \mathrm dS = \frac{\delta Q}{T} }[/math] system, actually possible, irreversible process 封闭系统中理想状态下的可逆过程).}</math>
这是因为在这种情况下的一般过程可能包括周围环境对系统所做的功,该功在系统内部会产生摩擦或粘滞效应,此时一个化学反应可能正在系统内部进行,或因为热传递实际上是不可逆地发生,系统温度[math]\displaystyle{ T }[/math]和周围环境温度[math]\displaystyle{ T_surr }[/math]之间的差异驱动了热传递的发生。[13][14] 注意该等式也适用于纯热流Pure Heat Flow[15]
- [math]\displaystyle{ \mathrm dS = \frac{\delta Q}{T} \,\, \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \text {(actually possible quasistatic irreversible process without composition change 实际上可能的,不改变成分的准静态不可逆性).} }[/math]
这种方法是通过测量热容曲线 Heat Capacity Curves和相变中熵的变化,来准确测定纯物质的绝对熵的基础,[16] [11]比如量热法calorimetry。 为了描述一个热力学系统在物理平衡状态下(要求有明确定义的等压P和等温T)偏离化学平衡状态,引入一组内部变量[math]\displaystyle{ x_i }[/math],[15] 可以用该等式
- [math]\displaystyle{ \mathrm dS = \frac{\delta Q}{T} - \frac{1}{T} \sum_{j} \, \Xi_{j} \,\delta \xi_j \,\, \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \text {(closed system, actually possible quasistatic irreversible process 封闭系统,实际可能的准静态不可逆过程).} }[/math]
第二项代表内部变量的功,这些内部变量可能受到外部影响的干扰,但是系统不能通过内部变量做任何正功。这种说法介绍了热力学系统在时间上演化不可能逆转的性质,并且可以被认为是热力学第二原理的另外一种相当于熵原理的表述。该公式可以被看作使用熵Entropy的热力学第二定律等价表述[17][18]
热力学第零定律是指如果两个热力学系统都与第三个热力学系统处于热平衡(温度相同)状态,则它们彼此也必定处于热平衡状态。热力学第零定律在它这个简短叙述中让人们认识到热平衡关系中的两个物体具有相同的温度,特别是当一个被测物体与一个参考测温物体具有相同的温度时,[19]对于两个处于热平衡状态的物体,有无限多的经验温标empirical temperature scales,这通常取决于特定参考温度体的性质。热力学第二定律允许区分温度标度temperature scale,它定义了一个绝对的热力学温度,与任何特定的参考温度体的性质无关。[20][21]
Various statements of the law热力学第二定律的不同表述
热力学第二定律可以用许多特定的方式来表达,[22] 最突出的经典陈述是 克劳修斯 Rudolf Clausius (1854)表述,开尔文 克劳修斯 Kelvin Clausius (1851)表述,以及康斯坦丁·卡拉西奥多里 Constantin Carathéodory(1909)在公理化热力学axiomatic thermodynamics中的表述。这些表述用一般的物理术语来描述定律,引用某些过程的不可能性。克劳修斯和开尔文表述被证明是等价的。
Carnot's principle卡诺原理
热力学第二定律的历史起源[23]是卡诺原理Carnot's principle。 它指的是卡诺热机Carnot heat engine的一个循环,卡诺热机以准静态quasi-static的极限慢速运转,因此热和功在子系统之间进行传递,子系统总是处于它们自己内部的热力学平衡状态。 卡诺热机是研究热机效率的工程师特别感兴趣的理想装置。 当卡诺发现卡诺原理时,热量理论caloric theory of heat还没有得到重视,热力学第一定律还没有得到承认,熵的概念还没有数学表达。 根据第一定律的解释,它在物理上等同于热力学第二定律,并沿用至今。 在热力学第一定律被发现之前,卡诺最初的论点是从热量理论的观点出发的。 下面是他书中的一些例子:
- ...wherever there exists a difference of temperature, motive power can be produced.[24]
...wherever there exists a difference of temperature, motive power can be produced.
……只要有温差,就能产生动力。
动力的产生不是由于蒸汽机实际消耗的热量,而是由于它从一个较热的物体转移到一个较冷的物体……。[25]
热的原动力与实现热的媒介无关,热的量完全取决于两个物体之间的温度,最后是热量的传递。[26]
用现代术语,卡诺原理可能更为准确:
准静态卡诺循环或可逆卡诺循环的效率只取决于两种热源的温度,而且无论工作物质是什么,效率是相同的。使用两个热源与工作物质交换热源(一个高温热源温度[math]\displaystyle{ T_1 }[/math]和一个低温热源温度[math]\displaystyle{ T_2 }[/math])的卡诺热机是最有效的热机。[27][28][29][30][31][32]
使用两个热源与工作物质交换热源(一个高温热源温度[math]\displaystyle{ T_1 }[/math]和一个低温热源温度[math]\displaystyle{ T_2 }[/math])的卡诺热机是最有效的热机。 A Carnot engine operated in this way is the most efficient possible heat engine using those two temperatures.
Clausius statement克劳修斯表述
1850年,德国科学家鲁道夫·克劳修斯 Rudolf Clausius 通过研究热传递和功之间的关系,为热力学第二定律奠定了基础。他在1854年用德语发表的论文中所提及的热力学第二定律定义被称为克劳修斯表述:
不可能把热从低温物体传递到高温物体而不产生其他影响。
克劳修斯的表述使用了“热通道Passage Of Heat”的概念。 在热力学的讨论中,通常这意味着“能量作为热的形式的净转移” ,而不是指其他方式的转移。
如果不对系统外部做功,热就不能自发地从冷区流向热区,这一点从制冷的普通经验中也可以看出。在冰箱中,只有在外部媒介也就是制冷系统的强制作用下热才会从冷区流到热区。
Kelvin statements开尔文表述
Lord Kelvin expressed the second law in several wordings.
Lord Kelvin expressed the second law in several wordings.
开尔文勋爵 Lord Kelvin 表述了热力学第二定律。
不可能从单一热源取热使之完全转换为有用的功而不产生其他影响。
不可能通过无生命物质的作用,将物质的任何部分冷却到低于周围物体最低的温度并产生机械效应 Mechanical Effect。
Equivalence of the Clausius and the Kelvin statements克劳修斯和开尔文表述的等价性
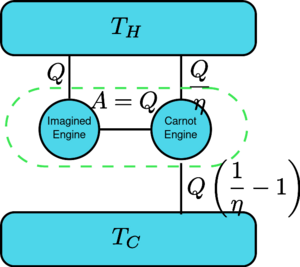
假设有一个热机违反了开尔文定理: 也就是说,这个热机以循环的方式吸收热并将其完全转化为功,而且不产生任何影响。现在将其与反向卡诺机相比较,如图所示。
普通热机的效率为η,反向热机的效率为1/η。这对联合热机的净效应和唯一效应是[math]\displaystyle{ \Delta Q=Q\left(\frac{1}{\eta}-1\right) }[/math] 将热从较冷热源转移到较热热源,这违反了克劳修斯表述。(这是能量守恒定律的结果,因为系统的总能量保持不变[math]\displaystyle{ \text{Input}+\text{Output}=0 \implies Q-\frac{Q}{\eta} = -Q_c }[/math],所以[math]\displaystyle{ Q_c=Q\left( \frac{1}{\eta}-1\right) }[/math]。)因此,违反开尔文表述意味着违反克劳修斯表述,即克劳修斯表述暗示了开尔文表述。我们可以用类似的方式证明开尔文表述暗示了克劳修斯表述,因此两者是等价的。
Planck's proposition普朗克命题
普朗克提出了直接来自经验的如下命题。这有时被认为是他对热力学第二定律的表述,但他认为这是热力学第二定律推导的起点。
不可能建造一台发动机,使其在一个完整的循环中工作,并且除了提高重量和冷却热储以外不会产生任何效果。[33][34]
Relation between Kelvin's statement and Planck's proposition 开尔文表述与普朗克命题的关系
教科书中几乎总是用“开尔文-普朗克表述 Kelvin-Planck Statement”来称呼该定律,例如德克·特哈尔 Diek ter Haar 和哈拉尔德·沃格兰 Harald Wergeland 就是这样表述的。[35]
热力学第二定律的开尔文-普朗克表述(或称“热机表述 Heat Engine Statement”)指出
设计一种唯一效果是从单一热源吸收热并提供等量的功的循环运行装置是不可能的。[36]
Planck's statement 普朗克表述
普朗克表述第二定律如下。
自然界中发生的任一过程都是沿参与其中的所有物体的熵之和增加的方向进行的。在极限情况中,即对于可逆过程,熵的总和保持不变。[37][38][39]
与普朗克表述非常相似的是乌伦贝克 Uhlenbeck和福特 Ford关于不可逆现象的表述。
……在从一个平衡态到另一个平衡态的不可逆或自发的变化中(例如,当两个物体 A 和 B 接触时的温度平衡过程),熵总是增加。[40]
Principle of Carathéodory 卡拉西奥多里原理
—— caratheodory 的原理在这里重新定向——
康斯坦丁·卡拉西奥多里 Constantin Carathéodory在纯数学公理的基础上进行了热力学公理化formulated阐明。他对第二定律的陈述被称为卡拉西奥多里原理 Principle of Carathéodory,可以这样表述:[41]
在绝热封闭系统的任意状态 S 附近,总有从 S 出发不可达的状态。[42]
通过这个阐明,他首次描述了绝热可达性 Adiabatic Accessibility的概念,并为经典热力学的一个新的子领域,即通常所说的几何热力学 Geometrical Thermodynamics奠定了基础。由卡拉西奥多里原理可以推出,作为热的能量的准静态转移是一个 完整的过程函数holonomic process function即[math]\displaystyle{ \delta Q=TdS }[/math]。[43]
--Dorr(讨论)准静态转移的热量值是一个可积过程函数 存疑 --嘉树(讨论)本句的修改供讨论。可积函数的英文应该是Integrable function。但是什么是完整的函数存疑,是连续的函数? --小头盔(讨论)这里建议标注一下 完整的过程函数 holonomic process function
--Dorr(讨论)奠定了基础为何要删???????????????????????????????????????? --嘉树(讨论) 不好意思,不应该删,已经改回来了
尽管在教科书几乎惯称卡拉西奥多里原理也是第二定律的一种表述,并认为其与克劳修斯表述或开尔文-普朗克表述等价,但事实并非如此。为了得到第二定律的所有内容,需要对卡拉西奥多里原理补充普朗克表述,即等量功总是增加一个最初处于自身内部热力学平衡的封闭系统的内部能量。[14]模板:Sfnp[44][45]
Planck's principle 普朗克原理
1926年,马克斯·普朗克 Max Planck写了一篇关于热力学基础的重要论文。[44][46] 他指出了以下原理
一个封闭系统的内部能量因绝热过程增加,在整个过程中,系统的体积保持不变。[14]
这个公式没有提到热,没有提到温度,甚至没有提到熵,也不一定隐含地依赖于这些概念,但它暗示了第二定律的内容。一个密切相关的表述为,“摩擦力从来不做正功。”[47]普朗克写道: “摩擦生热是不可逆的。”[48][49]
这个普朗克定理没有提到熵,是用物理术语来表述的。它与上面给出的开尔文表述密切相关。[50]相关的是,对于恒定体积和摩尔数的系统,熵是内能的单调函数。然而,普朗克的这个原理实际上并不是普朗克对第二定律的首选表述(见前面小节),而是依赖于熵的概念。
Borgnakke 和 Sonntag 提出的以下表述在某种意义上是对普朗克原理的补充。他们没有将其作为第二定律的完整表述:
……只有一种方法可以减少(封闭)系统的熵,将热从系统中转移出去。[51]
与普朗克之前提出的原理不同,这一原理明确地用熵的变化来表示。从系统中去除物质也可以减少系统的熵。
Statement for a system that has a known expression of its internal energy as a function of its extensive state variables 一个其内能有已知表达式(其扩展状态变量的函数)的系统的表述
写成广泛性质(质量,体积,熵……)的函数时,第二定律被证明等价于弱凸函数内能 U。The second law has been shown to be equivalent to the internal energy U being a weakly convex function, when written as a function of extensive properties (mass, volume, entropy, ...).[52][53]
Corollaries 推论
Perpetual motion of the second kind 第二类永动机
卡诺定理 Carnot's Theorem(1824)是一条限制任何可能的热机的最大效率的原理。效率完全取决于热源中热和冷之间的温差。 卡诺定理指出:
- 所有在两个热源之间工作的不可逆热机的效率低于在
两相同同一个热源之间工作的卡诺热机 Carnot Engine。 - 所有在两个热源之间工作的可逆热机的效率与在
两相同同一个热源之间工作的卡诺机相等。
所有在两个热源之间工作的可逆热机的效率与在两相同热源之间工作的卡诺机相等。All irreversible heat engines between two heat reservoirs are less efficient than a Carnot engine operating between the same reservoirs.
--Dorr(讨论)改成同一个正确吗?????????????????????????? --嘉树(讨论)我不太理解什么叫between the same reservoirs。我的理解是效率不取决于热源这个实体,而取决于温差,所以就按照两个热源和一个相同的热源相对应地翻译了。但是这一句我仍然有疑问,因为两个热源之间工作和两相同热源之间工作,似乎还不构成相互比较的关系。已经标绿
在他的理想模型中,热转化为功的过程可以通过逆转循环的运动而恢复,这个概念后来被称为热力学可逆性 Thermodynamic Reversibility。然而,卡诺进一步假定,一些热量损失了,并没有转化为机械功。因此,没有一个真实的热机能够实现卡诺循环 Carnot Cycle的可逆性,并且被认为效率较低。
该理论尽管是用热量表述的(见被取代的热质说),而不是熵,但是它是对第二定律的早期认识。
Clausius inequality 克劳修斯不等式
克劳修斯定理 Clausius Theorem(1854)指出,在一个循环的过程中
- [math]\displaystyle{ \oint \frac{\delta Q}{T} \leq 0. }[/math]
等号在可逆情况下成立,[54]严格不等号在不可逆情况下成立。可逆情况下引入状态函数熵。这是因为在循环过程中,状态功能的变化相对于状态功能为零。This is because in cyclic processes the variation of a state function is zero from state functionality.
--Dorr(讨论)末句存疑 --嘉树(讨论) 我也没看懂,按照字面意思修改了一下 --小头盔(讨论)感觉修改后的句子还是怪怪的,这里标成疑难句吧 这是因为在循环过程中,状态功能的变化相对于状态功能为零。This is because in cyclic processes the variation of a state function is zero from state functionality.
Thermodynamic temperature 热力学温度
对于任意热机,效率为:
其中 Wn 表示每个循环所做的净功。因此效率只取决于 qC/qH。
卡诺定理指出,所有在相同热源间运行的可逆热机的效率是相同的。
因此,任何在温度T1和T2之间运行的可逆热机必须具有相同的效率,也就是说,效率只是温度的函数:
[math]\displaystyle{ \frac{q_C}{q_H} = f(T_H,T_C)\qquad (2). }[/math]
另外,在温度 T1 和 T3 之间工作的可逆热机必须具有与由分别在温度T1 和(中间)温度 T2之间、在 T2 和 T3 之间的两个循环组成的系统效率相同。只有下式成立才会出现这种情况:
- [math]\displaystyle{ f(T_1,T_3) = \frac{q_3}{q_1} = \frac{q_2 q_3} {q_1 q_2} = f(T_1,T_2)f(T_2,T_3). }[/math]
现在考虑如下情形,[math]\displaystyle{ T_1 }[/math] 是一个固定的参考温度: 水的三相点 Triple Point的温度。则对于任意 T2 和 T3,
- [math]\displaystyle{ f(T_2,T_3) = \frac{f(T_1,T_3)}{f(T_1,T_2)} = \frac{273.16 \cdot f(T_1,T_3)}{273.16 \cdot f(T_1,T_2)}. }[/math]
因此,如果热力学温度被定义为
- [math]\displaystyle{ T = 273.16 \cdot f(T_1,T) \, }[/math]
那么函数 f 作为热力学温度的函数,为
- [math]\displaystyle{ f(T_2,T_3) = \frac{T_3}{T_2}, }[/math]
参考温度 T1 的值为273.16。(任何参考温度和任何正值均可用——此处的选择对应开尔文标度。)
Entropy 熵
根据克劳修斯等式,对可逆过程有
- [math]\displaystyle{ \oint \frac{\delta Q}{T}=0 }[/math]
这意味着线积分 [math]\displaystyle{ \int_L \frac{\delta Q}{T} }[/math] 对于可逆过程是路径无关的。
所以我们可以定义一个叫做熵的状态函数 S,对于可逆过程或者纯热传递满足
- [math]\displaystyle{ dS = \frac{\delta Q}{T} \! }[/math]
据此,只有对上述公式进行积分,才能得到熵的差值。为了获得绝对值,我们需要热力学第三定律 Third Law of Thermodynamics,它指出绝对零度 Absolute Zero下完美晶体的 S = 0。
对于任意不可逆过程,由于熵是一个状态函数,我们总是可以将初始状态和最终状态与一个虚拟的可逆过程联系起来,并在这条路径上积分以计算熵的差值。
现在把可逆过程逆过来,将其与上述不可逆过程结合。把克劳修斯不等式应用到这个循环,
- [math]\displaystyle{ -\Delta S+\int\frac{\delta Q}{T}=\oint\frac{\delta Q}{T}\lt 0 }[/math]
Thus,
Thus,
故,
- [math]\displaystyle{ \Delta S \ge \int \frac{\delta Q}{T} \,\! }[/math]
如果变换可逆,等号成立。
注意,若该过程是一个绝热过程 Adiabatic Process,则[math]\displaystyle{ \delta Q=0 }[/math],故[math]\displaystyle{ \Delta S\ge 0 }[/math]。
Energy, available useful work
Energy, available useful work 能量,可用的有用工作
考虑将第二定律应用于孤立系统(称为整体系统或宇宙)是一个重要而具有启发性的理想情形。该系统由两部分组成:研究关心的子系统和子系统的周围环境。因为想象的环境非常大,以至于它们可以被视为一个温度为 TR 且压力为 PR 的无限热源,因此无论有多少热被转移到(或来自)子系统,周围的温度将保持TR;无论子系统的体积膨胀(或收缩)有多大,周围环境的压力都将保持不变。
无论子系统和周围环境单独地发生什么熵的变化 dS和dSR,根据第二定律,孤立总体系统的熵Stot不能减小。
- [math]\displaystyle{ dS_{\mathrm{tot}}= dS + dS_R \ge 0 }[/math]
[math]\displaystyle{ dS_{\mathrm{tot}}= dS + dS_R \ge 0 }[/math]
[math]\displaystyle{ dS_{\mathrm{tot}}= dS + dS_R \ge 0 }[/math]
根据热力学第一定律,子系统内能的变化 dU 是加在子系统上的热δq的和,减去子系统所做的任何功w,再加上进入子系统的任何净化学能 d ∑μiRNi,因此
- [math]\displaystyle{ dU = \delta q - \delta w + d(\sum \mu_{iR}N_i) \, }[/math]
[math]\displaystyle{ dU = \delta q - \delta w + d(\sum \mu_{iR}N_i) \, }[/math]
[math]\displaystyle{ dU = \delta q - \delta w + d(\sum \mu_{iR}N_i) \, }[/math]
其中μiR是外部环境中化学形态 chemical species的化学势。
现在热量离开热源进入子系统是
数学△ qtr (- dS r) le tr dS / math
在这个过程中,首先使用了经典热力学中熵的定义(在统计热力学中,熵变、温度和吸收热量之间的关系可以将其推导出来 can be derived) ,然后从上面的公式可以推导出第二定律的不等式。
因此,子系统所做的任何净功δw必须服从
数学增量 w le-dU + tds + sum mu { iR } dN i,/ math
将子系统所做的功δw划分为子系统可以完成的有用功δwu ,除了子系统在周围外部压力下膨胀所做的功pR dV外,给出以下可用功(有用能 exergy)关系式:
- [math]\displaystyle{ \delta w_u \le -d (U - T_R S + p_R V - \sum \mu_{iR} N_i )\, }[/math]
[math]\displaystyle{ \delta w_u \le -d (U - T_R S + p_R V - \sum \mu_{iR} N_i )\, }[/math]
[math]\displaystyle{ \delta w_u \le -d (U - T_R S + p_R V - \sum \mu_{iR} N_i )\, }[/math]
为方便起见,可以把右边定义为热力学势的精确导数,称为子系统的可用性 Availability 或有用能 exergy
- [math]\displaystyle{ E = U - T_R S + p_R V - \sum \mu_{iR} N_i }[/math]
[math]\displaystyle{ E = U - T_R S + p_R V - \sum \mu_{iR} N_i }[/math]
[math]\displaystyle{ E = U - T_R S + p_R V - \sum \mu_{iR} N_i }[/math]
因此,第二定律意味着,对于任何可以简单地被视为分为一个子系统和一个与之接触的无限温度和压力热源 Reservoir 的过程,The Second Law therefore implies that for any process which can be considered as divided simply into a subsystem, and an unlimited temperature and pressure reservoir with which it is in contact,
- [math]\displaystyle{ dE + \delta w_u \le 0 \, }[/math]
[math]\displaystyle{ dE + \delta w_u \le 0 \, }[/math]
[math]\displaystyle{ dE + \delta w_u \le 0 \, }[/math]
也就是。子系统有用能的变化加上子系统所做的有用功(或者,子系统有用能的变化减去除了压力热源外任何对系统做的功)必须小于或等于零。
总之,如果选择一个合适的类似于无限库的参考状态作为现实世界中的系统环境, 则第二定律预测不可逆过程的E值减少,可逆过程的E值不变。
- [math]\displaystyle{ dS_{tot} \ge 0 }[/math] Is equivalent to [math]\displaystyle{ dE + \delta w_u \le 0 }[/math]
[math]\displaystyle{ dS_{tot} \ge 0 }[/math] Is equivalent to [math]\displaystyle{ dE + \delta w_u \le 0 }[/math]
[math]\displaystyle{ dS_{tot} \ge 0 }[/math] Is equivalent to [math]\displaystyle{ dE + \delta w_u \le 0 }[/math]
这个表达式和相关的参考状态允许设计工程师在宏观尺度(高于热力学极限)下使用第二定律,而无需直接测量或考虑整个孤立系统中的熵变。(另见工艺工程师process engineer)。这些变化已经在假设中被考虑到,该假设认为所考虑的系统可以在不改变参考状态的情况下与参考状态达到平衡。将其与可逆理想状态进行比较,还可以找到一个过程或过程集合的效率(见第二定律效率second law efficiency)
第二定律的这种方法被广泛应用于工程实践engineering practice、环境会计environmental accounting、系统生态学systems ecology等其他学科。
The second law in chemical thermodynamics 化学热力学的第二定律
对于一个恒温恒压封闭系统中的自发化学过程,在没有 non-PV 功的情况下,克劳修斯不等式ΔS > Q/Tsurr由于吉布斯自由能Gibbs free energy的变化而转化为:
- [math]\displaystyle{ \Delta G \lt 0 }[/math]
[math]\displaystyle{ \Delta G \lt 0 }[/math]
[math]\displaystyle{ \Delta G \lt 0 }[/math]
或者 dG < 0。对于一个相似的恒温恒压过程,亥姆霍兹自由能 Helmholtz free energy 的变化一定是负的, [math]\displaystyle{ \Delta A \lt 0 }[/math]。因此,一个负的自由能(G 或 A)变化是过程自发的必要条件。这是热力学第二定律在化学中最有用的形式,其中自由能的变化可以通过表列生成焓 Tabulated Enthalpies of Formation和反应物及产物的标准摩尔熵来计算。[11] 在温度和压力不变的情况下,化学平衡条件是 dG = 0。
History历史
尼古拉·莱昂纳尔·萨迪·卡诺穿着传统的巴黎综合理工学院制服
卡诺在1824年提出热转化为机械功的第一个理论。他是第一个正确认识到了转换效率取决于发动机和环境之间的温差的人。
克劳修斯Rudolf Clausius 认识到焦耳James Prescott Joule在能量守恒方面工作的重要性后,在1850年提出了第二定律的第一个公式,在这个公式中: 热不会自发地从冷物体流向热物体。虽然现在这是常识,但是这与当时流行的热理论相反,当时的热理论认为热是一种流体。从这些他推断出了 萨迪卡诺定律the principle of Sadi Carnot和熵的定义(1865年)。
19世纪提出的开尔文-普朗克第二陈述(Kelvin-Planck)表示:“任何循环运行的设备都不可能从单个热源接收热并产生净功。”这被证明与克劳修斯的陈述等价。
遍历假设ergodic hypothesis对玻尔兹曼方法Boltzmann approach也很重要。遍历假设认为在很长一段时间内,在具有相同能量的微观态相空间的某些区域所花费的时间与这个区域的体积成正比,即在很长一段时间内,所有可访问的微观状态出现/成立的可能性都是一样的。等价于说,它表明时间平均值和统计集合的平均值是相同的。
--嘉树(讨论) 觉得加上出现/成立好一点?
克劳修斯还提出了一种传统的学说,他认为熵可以被理解为宏观系统中的分子“无序”molecular 'disorder',但这种学说已经过时了。[55][56][57]
Account given by Clausius作者: 克劳修斯
鲁道夫 · 克劳修斯
1856年,德国物理学家鲁道夫 • 克劳修斯Rudolf Clausius 阐述了他所谓的“热力学理论中的第二个基本定理second fundamental theorem in the mechanical theory of heat” ,其形式如下:
- [math]\displaystyle{ \int \frac{\delta Q}{T} = -N }[/math]
[math]\displaystyle{ \int \frac{\delta Q}{T} = -N }[/math]
[math]\displaystyle{ \int \frac{\delta Q}{T} = -N }[/math]
其中 Q 是热,T 是温度,N 是一个循环过程中所有非补偿的相变的“等价值”。后来在1865年,克劳修斯将“等价值”定义为熵。基于这个理论,同一年,第二定律最著名的版本在4月24日苏黎世哲学学会的一次演讲中被提出,在演讲的最后克劳修斯总结道:
宇宙的熵趋于最大
这句话是第二定律最著名的陈述。由于其语言松散模糊,如“宇宙universe”,和缺乏具体的条件,如“开放open”,“封闭closed”,或“孤立isolated”,许多人认为这一简单的陈述意味着热力学第二定律几乎适用于每一个可以想象的主题。这不是真的;这句话只是一个更广泛和更精确的描述的简化版本。
就时间变化而言,一个经历任意变换的孤立系统的第二定律的数学表述是:
- [math]\displaystyle{ \frac{dS}{dt} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} \ge 0 }[/math]
where: S is the entropy of the system and
S is the entropy of the system and
- t is time.
t is time.
where
这里S 是系统的熵,T是时间
!-- 可逆过程需要与周围环境保持平衡。这对于一个孤立的系统是不可能的。因此,关于可逆过程的讨论已经转移到对封闭系统的分析----
平衡后用等号。另一种表述孤立系统第二定律的方法是:
- [math]\displaystyle{ \frac{dS}{dt} = \dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} = \dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} = \dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
用[math]\displaystyle{ \dot S_{i} }[/math]表示系统内所有进程熵产生Entropy Production的速率之和。这个公式的优点是它显示了熵产生的效果。熵产生率是一个非常重要的概念,因为它决定(或限制)热机的效率。乘以环境温度[math]\displaystyle{ T_{a} }[/math],它给出所谓的耗散能[math]\displaystyle{ P_{diss}=T_{a}\dot S_{i} }[/math]。
封闭系统的第二定律(允许热量交换和移动边界,但不允许物质交换)的表达式是:
- [math]\displaystyle{ \frac{dS}{dt} = \frac{\dot Q}{T}+\dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} = \frac{\dot Q}{T}+\dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
Here : [math]\displaystyle{ \dot Q }[/math] is the heat flow into the system
[math]\displaystyle{ \dot Q }[/math] is the heat flow into the system
[math]\displaystyle{ \dot Q }[/math]
- [math]\displaystyle{ T }[/math] is the temperature at the point where the heat enters the system.
[math]\displaystyle{ T }[/math] is the temperature at the point where the heat enters the system.
Here
这里[math]\displaystyle{ \dot Q }[/math]是进入系统的热流,[math]\displaystyle{ T }[/math] 是热量进入系统时的温度。
只有在系统内发生可逆过程的情况下等号才成立如果发生不可逆过程(在实际操作系统中就是这种情况),则“>”成立。如果系统有多处供热,必须求相应项的代数和。
--嘉树(讨论) 因为是中文环境所以统一用中文标点
对于开放系统(也允许物质交换) :
- [math]\displaystyle{ \frac{dS}{dt} = \frac{\dot Q}{T}+\dot S+\dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
[math]\displaystyle{ \frac{dS}{dt} = \frac{\dot Q}{T}+\dot S+\dot S_{i} }[/math] with [math]\displaystyle{ \dot S_{i} \ge 0 }[/math]
这里[math]\displaystyle{ \dot S }[/math] 是进入系统的熵流,与进入系统的物质流有关。它不应该与熵的时间导数混淆。如果物质在几个地方被供给,需要取这些贡献的代数和。
Statistical mechanics
统计力学
统计力学通过假设物质是由不断运动的原子和分子组成的,来对第二定律给出了解释。系统中每个粒子的一组特定的位置和速度称为系统的微观状态,由于系统的不断运动,系统不断地改变其微观状态。统计力学假设,在平衡状态下,系统处于的每个微观状态的可能性是相等的。这个假设的提出直接导致第二定律必须在统计学意义上成立,也就是说,第二定律在平均意义上成立,其中统计学变异取决于数量级1/模板:Radic,其中 N 是系统中粒子数。在日常(宏观)情况下,违反第二定律的概率几乎为零。然而,对于粒子数量很少的系统,热力学参数,包括熵,可能显示出与第二定律预测结果的显著的统计偏差。经典热力学理论不处理这些统计变量。
(by Jie XIANG) 在通过假定一种物质由不断运动的原子和分子组成的前提下,统计力学对热力学第二定律进行了解释。系统中每个粒子其特定的位置和速度组成为系统的微观状态,由于(原子和分子的)不断运动,系统也持续改变其微观状态。统计力学假设,在系统力平衡的情况下,其微观状态其实是等可能发生的,进而能够得出结论:热力学第二定律必须在统计学意义上成立。也就是说,第二定律将保持均值不变, 其统计变化为1 /√N,N为系统中的粒子数。在日常(宏观)情况下,违反热力学第二定律的概率几乎为零,但是,对于粒子数量非常少的系统,其热力学参数(包括熵)可能会显示出与第二定律所预测的明显统计偏差。然而经典热力学理论并不处理这些统计变量。
--嘉树(讨论)当前结果已经参考了两种翻译,第二个翻译暂作删除处理。
Derivation from statistical mechanics
从统计力学导出
气体动力学Kinetic theory of gases理论的第一个力学论证由麦克斯韦James Clerk Maxwell在1860年给出,指出分子碰撞引起温度均衡化,因此整体趋向于平衡 Equilibrium ; 玻尔兹曼Ludwig Boltzmann[58]在1872年提出的 H 定理H-theorem也认为,气体由于碰撞应该随着时间的推移趋向于麦克斯韦-波兹曼分布Maxwell–Boltzmann distribution。
由于洛施密特悖论Loschmidt's paradox,第二定律的导出必须对过去做出一个假设,即系统在过去的某个时刻是不相关的;这样的假设允许进行简单的概率处理。这个假设通常被认为是一个边界条件boundary condition,因此热力学第二定律最终是过去某个地方的初始条件的结果,可能是在宇宙的开始(大爆炸 the Big Bang) ,尽管也有人提出了其他场景。.[59][60][61]
基于这些假设,在统计力学中,第二定律不是一个假设,而是统计力学基本假设Statistical mechanics#Fundamental postulate|fundamental postulate的一个结果,也被称为等先验概率假设 equal prior probability postulate。这个基本假设表明,只要一个人清楚地知道,简单的概率论证只适用于未来,而对于过去,有辅助的信息来源告诉我们,它是低熵的。如果我们把熵的概念限制在热平衡系统中,那么热力学第二定律的第一部分即热孤立系统的熵只能增加,是等先验概率假设的一个显然结果。处于热平衡状态的孤立系统且具有能量[math]\displaystyle{ E }[/math]的熵表示为:
- [math]\displaystyle{ S = k_{\mathrm B} \ln\left[\Omega\left(E\right)\right]\, }[/math]
[math]\displaystyle{ S = k_{\mathrm B} \ln\left[\Omega\left(E\right)\right]\, }[/math]
其中[math]\displaystyle{ \Omega\left(E\right) }[/math] 是处于 [math]\displaystyle{ E }[/math]和[math]\displaystyle{ E +\delta E\lt /math这个小区间内的量子态数目。这里的 \lt math\gt \delta E }[/math] 是一个宏观上很小的固定能量区间。严格地说,这意味着熵取决于对[math]\displaystyle{ \delta E }[/math]的选择。然而在热力学极限下(例如无穷大系统的极限),狭义的熵(单位体积或单位质量的熵)不依赖于 [math]\displaystyle{ \delta E }[/math]。
假设我们有一个孤立系统,其宏观状态由许多变量指定。这些宏观变量可以是总体积、活塞在系统中的位置等。从而[math]\displaystyle{ \Omega }[/math]将取决于这些变量的值。如果某个变量不是固定的(我们不会在某个位置夹住活塞) ,那么因为在平衡状态下到达所有可到达状态的可能性是相等的,平衡状态下的自由变量会使 [math]\displaystyle{ \Omega }[/math] 最大,因为这是平衡状态下最可能的情况。
如果该变量最初固定到某个值然后释放,当达到新的平衡时,变量将自我调整使得 [math]\displaystyle{ \Omega }[/math] 最大,这意味着熵将增加或保持不变(如果变量初始固定的值恰好是平衡值)。
假设我们初始位于一个平衡状态,突然移除了对一个变量的约束。我们做完这件事的时候,可达到的微观状态的数为[math]\displaystyle{ \Omega }[/math],但是系统还没有达到平衡,所以系统处于某些可达到的状态的实际概率还不等于先验概率 [math]\displaystyle{ 1/\Omega }[/math]。我们已经知道,最终的平衡状态相对于之前的平衡状态,熵会增加或者保持不变。然而,玻耳兹曼的H定理H-theorem证明系统在不处于平衡态的期间,那个量作为时间的函数单调增加。
Derivation of the entropy change for reversible processes
可逆过程熵变的推导
热力学第二定律的第二部分指出,经历可逆过程的系统的熵变为:
- [math]\displaystyle{ dS =\frac{\delta Q}{T} }[/math]
[math]\displaystyle{ dS =\frac{\delta Q}{T} }[/math]
其中温度定义为:
- [math]\displaystyle{ \frac{1}{k_{\mathrm B} T}\equiv\beta\equiv\frac{d\ln\left[\Omega\left(E\right)\right]}{dE} }[/math]
[math]\displaystyle{ \frac{1}{k_{\mathrm B} T}\equiv\beta\equiv\frac{d\ln\left[\Omega\left(E\right)\right]}{dE} }[/math]
请参阅此处Microcanonical ensemble|here查看该定义的正当性。假设系统有一些可以改变的外部参数 x。一般来说,系统的能量本征态将依赖于 x。根据量子力学的绝热定理adiabatic theorem,在系统哈密顿量无限缓慢变化的极限下,系统将保持在相同的能量本征态 energy eigenstate,因此系统的能量会随着其所在能量本征态的能量变化而变化。
--嘉树(讨论) 能量本征态 不懂翻译是否正确 --Jxzhou(讨论) 2020年8月17日 (一) 19:29 (CST)我觉得是这样的--Jxzhou(讨论) 2020年8月17日 (一) 19:29 (CST)
对于外部变量 x 可以定义广义力generalized force X ,因此如果 x 增加 dx,那么[math]\displaystyle{ X dx }[/math] 就是系统所做的功。例如,如果 x 是体积,那么 X 就是压强。一个已知处于能量本征态[math]\displaystyle{ E_{r} }[/math]的系统的广义力为:
- [math]\displaystyle{ X = -\frac{dE_{r}}{dx} }[/math]
[math]\displaystyle{ X = -\frac{dE_{r}}{dx} }[/math]
由于系统可以在[math]\displaystyle{ \delta E }[/math] 区间内的任意能量本征态内,我们将系统的广义力定义为上述表达式的期望值:
- [math]\displaystyle{ X = -\left\langle\frac{dE_{r}}{dx}\right\rangle\, }[/math]
[math]\displaystyle{ X = -\left\langle\frac{dE_{r}}{dx}\right\rangle\, }[/math]
为了计算平均值,我们按照能量本征态来划分[math]\displaystyle{ \Omega\left(E\right) }[/math],通过计算有多少能量本征态的[math]\displaystyle{ \frac{dE_{r}}{dx} }[/math]值在区间在[math]\displaystyle{ Y }[/math] 到 [math]\displaystyle{ Y + \delta Y }[/math]之间。把这个数字叫做为 [math]\displaystyle{ \Omega_{Y}\left(E\right) }[/math],我们有:
- [math]\displaystyle{ \Omega\left(E\right)=\sum_{Y}\Omega_{Y}\left(E\right)\, }[/math]
[math]\displaystyle{ \Omega\left(E\right)=\sum_{Y}\Omega_{Y}\left(E\right)\, }[/math]
根据平均值定义的广义力现在可以写成:
我们可以把它和由恒定能量 E 下的 x 导出来的熵联系起来。假定我们把 x 改变至 x + dx。然后因为能量本征态依赖于 x, [math]\displaystyle{ \Omega\left(E\right) }[/math] 将会改变,这导致能量本征态进入或超出[math]\displaystyle{ E }[/math] 和[math]\displaystyle{ E+\delta E }[/math] 之间的范围。让我们再次关注[math]\displaystyle{ \frac{dE_{r}}{dx} }[/math] 处于 [math]\displaystyle{ Y }[/math] 和 [math]\displaystyle{ Y + \delta Y }[/math] 之间的能量本征态。由于这些能量本征态的能量增加了 Y dx,所有这些在 E-Y dx 到 E 之间能量本征态都从 E 以下移动到 E 以上。 因此有
- [math]\displaystyle{ N_{Y}\left(E\right)=\frac{\Omega_{Y}\left(E\right)}{\delta E} Y dx\, }[/math]
[math]\displaystyle{ N_{Y}\left(E\right)=\frac{\Omega_{Y}\left(E\right)}{\delta E} Y dx\, }[/math]
这些的能量本征态。如果[math]\displaystyle{ Y dx\leq\delta E }[/math],则所有这些能量本征态将移动到 [math]\displaystyle{ E }[/math] 到 [math]\displaystyle{ E+\delta E }[/math]的范围内,使得[math]\displaystyle{ \Omega }[/math]增加。从[math]\displaystyle{ E+\delta E }[/math]以下移动到[math]\displaystyle{ E+\delta E }[/math]以上的能量本征态数目为 [math]\displaystyle{ N_{Y}\left(E+\delta E\right) }[/math]。它们的差
- [math]\displaystyle{ N_{Y}\left(E\right) - N_{Y}\left(E+\delta E\right)\, }[/math]
[math]\displaystyle{ N_{Y}\left(E\right) - N_{Y}\left(E+\delta E\right)\, }[/math]
因此是 [math]\displaystyle{ \Omega }[/math]增长的净贡献。请注意如果 Y dx 大于 [math]\displaystyle{ \delta E }[/math],那么将会有能量本征态从 E 以下移动到[math]\displaystyle{ E+\delta E }[/math]以上。它们在[math]\displaystyle{ N_{Y}\left(E\right) }[/math] 和 [math]\displaystyle{ N_{Y}\left(E+\delta E\right) }[/math]中都有计数,因此上述表达式在这种情况下也是有效的。
将上面的表达式表示为对 E 的导数,并且对 Y 求和得到表达式:
- [math]\displaystyle{ \left(\frac{\partial\Omega}{\partial x}\right)_{E} = -\sum_{Y}Y\left(\frac{\partial\Omega_{Y}}{\partial E}\right)_{x}= \left(\frac{\partial\left(\Omega X\right)}{\partial E}\right)_{x}\, }[/math]
[math]\displaystyle{ \left(\frac{\partial\Omega}{\partial x}\right)_{E} = -\sum_{Y}Y\left(\frac{\partial\Omega_{Y}}{\partial E}\right)_{x}= \left(\frac{\partial\left(\Omega X\right)}{\partial E}\right)_{x}\, }[/math]
[math]\displaystyle{ \Omega }[/math]的对数关于x的导数由以下方式给出:
- [math]\displaystyle{ \left(\frac{\partial\ln\left(\Omega\right)}{\partial x}\right)_{E} = \beta X +\left(\frac{\partial X}{\partial E}\right)_{x}\, }[/math]
[math]\displaystyle{ \left(\frac{\partial\ln\left(\Omega\right)}{\partial x}\right)_{E} = \beta X +\left(\frac{\partial X}{\partial E}\right)_{x}\, }[/math]
第一项是集约型的,即它不能根据系统大小进行缩放。相反,最后一项跟随逆系统的大小而缩放,因此将在热力学极限中消失。因此我们发现:
- [math]\displaystyle{ \left(\frac{\partial S}{\partial x}\right)_{E} = \frac{X}{T}\, }[/math]
[math]\displaystyle{ \left(\frac{\partial S}{\partial x}\right)_{E} = \frac{X}{T}\, }[/math]
将这个和下面式子结合
- [math]\displaystyle{ \left(\frac{\partial S}{\partial E}\right)_{x} = \frac{1}{T}\, }[/math]
[math]\displaystyle{ \left(\frac{\partial S}{\partial E}\right)_{x} = \frac{1}{T}\, }[/math]
给出:
- [math]\displaystyle{ dS = \left(\frac{\partial S}{\partial E}\right)_{x}dE+\left(\frac{\partial S}{\partial x}\right)_{E}dx = \frac{dE}{T} + \frac{X}{T} dx=\frac{\delta Q}{T}\, }[/math]
[math]\displaystyle{ dS = \left(\frac{\partial S}{\partial E}\right)_{x}dE+\left(\frac{\partial S}{\partial x}\right)_{E}dx = \frac{dE}{T} + \frac{X}{T} dx=\frac{\delta Q}{T}\, }[/math]
Derivation for systems described by the canonical ensemble
正则系综系统的推导
如果一个系统与某个温度为T的热浴热接触,那么在平衡状态下,关于能量本征值的概率分布值由正则系综canonical ensemble给出:
- [math]\displaystyle{ P_{j}=\frac{\exp\left(-\frac{E_{j}}{k_{\mathrm B} T}\right)}{Z} }[/math]
[math]\displaystyle{ P_{j}=\frac{\exp\left(-\frac{E_{j}}{k_{\mathrm B} T}\right)}{Z} }[/math]
这里 Z 是一个使所有概率之和归一化到 1 的因子,这个函数被称为配分函数Partition function (statistical mechanics)|partition function。现在我们考虑对温度和能级所依赖的外部参数的无限小的可逆改变。它遵循熵的一般公式:
--嘉树(讨论) 能级依赖温度吗?即“现在我们考虑……的可逆改变”当中,“on which the energy levels depend”修饰(1)in the external parameters,还是(2)in the temperature and in the external parameters --小头盔(讨论)修饰in the external parameters
- [math]\displaystyle{ S = -k_{\mathrm B}\sum_{j}P_{j}\ln\left(P_{j}\right) }[/math]
[math]\displaystyle{ S = -k_{\mathrm B}\sum_{j}P_{j}\ln\left(P_{j}\right) }[/math]
that
that
以及
- [math]\displaystyle{ dS = -k_{\mathrm B}\sum_{j}\ln\left(P_{j}\right)dP_{j} }[/math]
[math]\displaystyle{ dS = -k_{\mathrm B}\sum_{j}\ln\left(P_{j}\right)dP_{j} }[/math]
把正则系综的数学形式 [math]\displaystyle{ P_{j} }[/math] 插入,可以得到:
- [math]\displaystyle{ dS = \frac{1}{T}\sum_{j}E_{j}dP_{j}=\frac{1}{T}\sum_{j}d\left(E_{j}P_{j}\right) - \frac{1}{T}\sum_{j}P_{j}dE_{j}= \frac{dE + \delta W}{T}=\frac{\delta Q}{T} }[/math]
[math]\displaystyle{ dS = \frac{1}{T}\sum_{j}E_{j}dP_{j}=\frac{1}{T}\sum_{j}d\left(E_{j}P_{j}\right) - \frac{1}{T}\sum_{j}P_{j}dE_{j}= \frac{dE + \delta W}{T}=\frac{\delta Q}{T} }[/math]
生命体
表述热力学主要有两种方式:(a)描述从一种热力学平衡状态到另一种状态的路径,(b)通过系统保持不变但周围环境的总熵增加的循环过程。这两种方法有助于理解生命过程。这个话题大大超出了本文的范围,但是已经有一些研究者考虑过这个问题,比如薛定谔Erwin Schrödinger、布里渊Léon Brillouin[62]和阿西莫夫Isaac Asimov。这也是当前研究的主题。
从近似的角度来看,生命体可以被认为是(b)的一个例子。近似地,一只动物的身体状态每天循环,使得它几乎没有什么变化。动物吸收食物、水和氧气,经过新陈代谢metabolism,输出分解的产物和热量。植物吸收来自太阳的辐射能量,这可以认为是热量,以及二氧化碳和水,然后它们释放氧气。它们就是这样生长的。它们最终会死亡,尸体腐烂,大部分重新变成二氧化碳和水。这可以看作是一个循环过程。总的来说,阳光来自一个高温的源——太阳,它的能量被传递到一个较低的温度源,即向太空辐射。这个过程使得植物周围环境的熵增加。因此从循环过程的角度来看,动物和植物服从热力学第二定律。简单的热机效率概念很难适用于这个问题,因为它们假定系统是封闭的。
从(a)的热力学观点考虑,即考虑从一个平衡状态到另一个平衡状态的路径,只会产生一个粗略近似的图像,因为生命体从来不会处于热力学平衡状态。生物体经常必须被认为是开放的系统,因为它们吸收营养物质并排出废弃物。开放系统热力学目前通常从一个热力学平衡状态到另一个状态的路径的角度来考虑,或者是考虑局部热力学平衡近似下的流。生命体的问题可以通过假定一个流不变的稳态的近似来进一步简化。对于这样的近似,目前有很多关于熵产生的一般原理的未解决的争论或研究。尽管如此,从热力学第二定律的这个角度出发衍生出来的想法对生物是有启发意义的。
Gravitational systems
Gravitational systems 重力系统
在不需要对其广义相对论解释的系统中,物体始终具有正热容,这意味着温度随能量而升高。因此,当能量从高温物体流向低温物体时,发射源温度降低而冷源温度升高;因此,温差会随着时间的流逝而减小。对于引力很重要并且需要广义相对论的系统而言,情况并非总是如此。这样的系统可以自发地向质量和能量不均匀的分布转变。这适用于大规模的宇宙,因此可能很难或不可能对它应用第二定律。[63]除此之外,广义相对论描述的系统的热力学超出了本文的范围。
Non-equilibrium states
Non-equilibrium states 非平衡状态
经典热力学或平衡热力学理论是理想化的。其主要假定或假设(通常甚至没有明确地陈述),是基于系统处在自身内部的热力学平衡状态。通常,在自然界可能发现的,在给定时间包含物理系统的空间区域,其热力学平衡状态并不是能用最严格的术语来表示。用较宽泛的术语来说,整个宇宙中没有任何东西是或曾经是真正处于精确的热力学平衡状态中。[64]
为了进行物理分析,通常做一个热力学平衡的假设就足够方便可行。 这样的假设可能依赖于反复试验和试错来证明其合理性。如果假设是合理的,那么它通常会非常有价值,因为它使热力学理论成为可能。平衡假设的要素是,观察到的系统会无限期地保持不变,并且因为系统中有很多粒子,以至于完全可以忽略其微粒的本质。通常在这种平衡假设下,不存在一个宏观上可检测到的波动。临界状态是一个例外,肉眼可以观察到临界乳光现象opalescence。对于临界状态的实验室研究,需要非常长的观察时间。
在所有情况下,一旦做出了热力学平衡的假设,则作为一个结果意味着假定中的能改变系统熵的候选波动不存在。
物理系统很容易发生内部宏观变化,其速度之快足以使熵的恒定性假设失效。或物理系统中的粒子太少,以至于微粒的本质在可观测的波动中显而易见。那么热力学平衡的假设就会被放弃。对于非平衡态,熵没有绝对的通俗定义。[65]
还有一些中间情况,其中局部热力学平衡的假设是一个非常好的近似,</ref>[66][67][68]但严格地说它仍然是一个近似值,不是理论上的理想值。
对于一般的非平衡状态,考虑其他量的统计力学定义可能是有用的,这些量可以方便地称为“熵”,但是它们不应该与第二定律正确定义的熵混淆或合并。这些其他量确实属于统计力学,而不属于第二定律的主要领域——热力学。
宏观上可观察到的波动的物理学超出了本文的范围。
时间之箭
时间中的热力学不对称性
热力学第二定律是在时间方向上不对称的物理定律。这与在物理学基本定律中观察到的对称性概念(特别是CPT对称性)并没有冲突,因为第二定律在统计上适用于时间非对称边界条件。第二定律与时间向前和向后移动之间的差异有关,或者与原因先于结果的原理(时间的因果关系或因果关系)有关。[69]
第二定律被提出来侧面解释时间向前和向后移动的区别,例如为什么原因先于结果(因果关系的时间箭头)。.[70]
Irreversibility 不可逆性
热力学过程中的不可逆性是热力学作用不对称特性的结果,而不是物体内部任何不可逆的微观性质的结果。热力学作用是对参与体施加的宏观外部干预,并非来自其内部属性。由于没有认识到这一点,产生了被称为“悖论”的说法。
Loschmidt's paradox 洛施密特悖论
洛施密特悖论 Loschmidt's paradox,也被称为可逆性悖论reversibility paradox,它反驳说,从描述宏观系统微观演化的时间对称动力学中,不可能推导出不可逆过程。
薛定谔的认为:“现在很明显,您必须以何种方式重新制定熵定律(亦或者所有其他不可逆陈述)以使它们能够从可逆模型中得出。你不能只谈论一个孤立的系统,而应该至少谈论两个。你可以暂时认为它们与世界其它地方是孤立的,但并不总是相互孤立的。”这两个系统被隔离内壁彼此分开,[71]直到被热力学(由热力学定律设想出来的)作用而拆除。热力学作用是从外部施加的,不受支配系统组成部分的可逆微观动力学定律的制约。这是不可逆转的原因。本文中第二定律的陈述符合薛定谔的的建议。 因果关系在逻辑上先于第二定律,而不是由第二定律推导而来。
Poincaré recurrence theorem 庞加莱始态复现定理
庞加莱始态复现定理考虑了孤立物理系统的理论微观描述。
--嘉树(讨论)又叫递归定理吗?存疑
经过热力学作用去除隔离内壁之后,便可以认为其是热力学系统的模型。在足够长的时间后,系统将返回到非常接近初始状态的微观定义状态。庞加莱复现时间是指回归前经过的时间长度。它极其漫长,可能比宇宙的寿命还要长,并且对那个被热力学作用拆除的内壁的几何形状敏感。复现定理可能被认为是明显与热力学第二定律相矛盾的。但是,更显而易见的是,它只是通过移除两个系统之间的内壁而形成的隔离系统中热力学平衡的微观模型。对所有典型的热力学系统的实际目的,人们无法观察到如此之长的复现时间很长(比宇宙的寿命长很多倍)。尽管如此,还是有人会想像一个机会可以等待庞加莱复现的出现,然后重新插入被热力学作用去除的内壁。然后很明显,不可逆性的出现是由于庞加莱复现完全不可预测的特性,因为仅仅给出了初始状态是热力学平衡之一,就像宏观热力学的情况一样。即使一个人可以等那么长时间,他也没有实际操作的可能性来选择合适的时间重新插入内壁。庞加莱始态复现定理为洛施密特悖论提供了一个解决方案。如果一个孤立的热力学系统能以多倍于平均庞加莱复现时间的长度下被监控,则该系统的热力学行为在时间反转下将变得恒定。
James Clerk Maxwell
詹姆斯·克拉克·麦克斯韦
Maxwell's demon 麦克斯韦妖
麦克斯韦 James Clerk Maxwell设想一个容器分为A和B两部分。两部分均在相同温度下充满相同的气体,并彼此相邻由内壁隔开。一个虚构的妖精看守着一个内壁上的微型陷门,并观察者内壁两边的分子。当来自A的速度快于平均水平的分子飞向门时,妖精将门打开,该分子将从A飞向B。B中分子的平均速度将增加,而A中分子的平均速度将降低。由于平均分子速度与温度相对应,因此与热力学第二定律相反,温度在A中降低,在B中升高。
1929年,Leó Szilárd和后来的 Léon Brillouin 对这个问题提出了一个答案。Szilárd指出,现实生活中的麦克斯韦妖需要一些测量分子速度的方法,而获取信息的过程需要消耗能量。
麦克斯韦的“妖”重复地改变 A 和 B 之间壁的热透性。因此,它是在微观尺度上执行热力学操作,而不仅仅是观察普通的自发或自然的宏观热力学过程。
语录
我认为,熵总是增加的定律在自然定律中占有至高无上的地位。如果有人指出您的宇宙宠物理论杜撰的宇宙理论与麦克斯韦方程不符,那么麦克斯韦方程就遇到麻烦了。如果发现您的理论与观察不符,那么,可能是这些实验学家搞砸了。但是,如果发现您的理论违反了热力学第二定律,那么就没有希望了。除了在深深的耻辱中崩溃,别无他法。
—亚瑟·斯坦利·爱丁顿爵士,《自然世界》(1927年)
关于第二定律的公式几乎与关于第二定律的讨论一样多。
—哲学家/物理学家 布里奇曼(1941)
克劳修斯(Sir Clausius)是西伯利亚文“宇宙的能量是恒定的;宇宙的熵趋于最大。”的作者。连续热力学的目标远远不能解释“宇宙”,但是在该理论内,我们可以很容易地以某种类似于克劳修斯的方式得出明确的陈述,只是它仅仅指代一个简单的物体:一个有限大小的孤立物体。
— Truesdell, C., Muncaster, R. G. (1980). Fundamentals of Maxwell's Kinetic Theory of a Simple Monatomic Gas, Treated as a Branch of Rational Mechanics, Academic Press, New York, ISBN 0-12-701350-4, p. 17. 特雷斯德尔,C.,蒙卡斯特,R.G.(1980)。麦克斯韦的单原子气体动力学理论的基本原理,被作为“理性力学”的一个分支,纽约学术出版社,ISBN 0-12-701350-4,第1页. 17。
See also
See also
参见
References
References
参考资料
- ↑ http://web.mit.edu/16.unified/www/FALL/thermodynamics/notes/node38.html#SECTION05224000000000000000
- ↑ Atkins and de Paula, p.78
- ↑ Zohuri, Bahman (2016). Dimensional Analysis Beyond the Pi Theorem. Springer. p. 111. ISBN 978-3-319-45726-0. https://books.google.com/books?id=pRVuDQAAQBAJ.
- ↑ Jaffe, R.L., Taylor, W. (2018). The Physics of Energy, Cambridge University Press, Cambridge UK, pages 150, 151, 259, 772, 743.
- ↑ Planck, M. (1897/1903), pp. 40–41.
- ↑ Munster A. (1970), pp. 8–9, 50–51.
- ↑ Mandl 1988
- ↑ Planck, M. (1897/1903), pp. 79–107.
- ↑ Bailyn, M. (1994), Section 71, pp. 113–154.
- ↑ Bailyn, M. (1994), p. 120.
- ↑ 11.0 11.1 11.2 Mortimer, R. G. Physical Chemistry. 3rd ed., p. 120, Academic Press, 2008.
- ↑ Fermi, E. Thermodynamics, footnote on p. 48, Dover Publications,1956 (still in print).
- ↑ Adkins, C.J. (1968/1983), p. 75.
- ↑ 14.0 14.1 14.2 Münster, A. (1970), p. 45.
- ↑ 15.0 15.1 Schmidt-Rohr, K. (2014). "Expansion Work without the External Pressure, and Thermodynamics in Terms of Quasistatic Irreversible Processes" J. Chem. Educ. 91: 402-409. https://dx.doi.org/10.1021/ed3008704
- ↑ Oxtoby, D. W; Gillis, H.P., Butler, L. J. (2015).Principles of Modern Chemistry, Brooks Cole. p. 617.
- ↑ Pokrovskii V.N. (2005) Extended thermodynamics in a discrete-system approach, Eur. J. Phys. vol. 26, 769–781.
- ↑ Pokrovskii, Vladimir N. (2013). "A Derivation of the Main Relations of Nonequilibrium Thermodynamics". ISRN Thermodynamics. 2013: 1–9. doi:10.1155/2013/906136.
- ↑ J. S. Dugdale (1996). Entropy and its Physical Meaning. Taylor & Francis. p. 13. ISBN 978-0-7484-0569-5. "This law is the basis of temperature."
- ↑ Zemansky, M.W. (1968), pp. 207–209.
- ↑ Quinn, T.J. (1983), p. 8.
- ↑ "Concept and Statements of the Second Law". web.mit.edu. Retrieved 2010-10-07.
- ↑ Carnot, S. (1824/1986).
- ↑ Carnot, S. (1824/1986), p. 51.
- ↑ Carnot, S. (1824/1986), p. 46.
- ↑ Carnot, S. (1824/1986), p. 68.
- ↑ Truesdell, C. (1980), Chapter 5.
- ↑ Adkins, C.J. (1968/1983), pp. 56–58.
- ↑ Münster, A. (1970), p. 11.
- ↑ Kondepudi, D., Prigogine, I. (1998), pp.67–75.
- ↑ Lebon, G., Jou, D., Casas-Vázquez, J. (2008), p. 10.
- ↑ Eu, B.C. (2002), pp. 32–35.
- ↑ Planck, M. (1897/1903), p. 86.
- ↑ Roberts, J.K., Miller, A.R. (1928/1960), p. 319.
- ↑ ter Haar, D., Wergeland, H. (1966), p. 17.
- ↑ Rao, Y. V. C. (1997). Chemical Engineering Thermodynamics. Universities Press. p. 158. ISBN 978-81-7371-048-3.
- ↑ Planck, M. (1897/1903), p. 100.
- ↑ Planck, M. (1926), p. 463, translation by Uffink, J. (2003), p. 131.
- ↑ Roberts, J.K., Miller, A.R. (1928/1960), p. 382. This source is partly verbatim from Planck's statement, but does not cite Planck. This source calls the statement the principle of the increase of entropy.
- ↑ Uhlenbeck, G.E., Ford, G.W. (1963), p. 16.
- ↑ Carathéodory, C. (1909).
- ↑ Buchdahl, H.A. (1966), p. 68.
- ↑ Sychev, V. V. (1991). The Differential Equations of Thermodynamics. Taylor & Francis. ISBN 978-1-56032-121-7.
- ↑ 44.0 44.1 Planck, M. (1926).
- ↑ Buchdahl, H.A. (1966), p. 69.
- ↑ Uffink, J. (2003), pp. 129–132.
- ↑ Truesdell, C., Muncaster, R.G. (1980). Fundamentals of Maxwell's Kinetic Theory of a Simple Monatomic Gas, Treated as a Branch of Rational Mechanics, Academic Press, New York, , p. 15.
- ↑ Planck, M. (1897/1903), p. 81.
- ↑ Planck, M. (1926), p. 457, Wikipedia editor's translation.
- ↑ Lieb, E.H., Yngvason, J. (2003), p. 149.
- ↑ Borgnakke, C., Sonntag., R.E. (2009), p. 304.
- ↑ van Gool, W.; Bruggink, J.J.C. (Eds) (1985). Energy and time in the economic and physical sciences. North-Holland. pp. 41–56. ISBN 978-0-444-87748-2.
- ↑ Grubbström, Robert W. (2007). "An Attempt to Introduce Dynamics Into Generalised Exergy Considerations". Applied Energy. 84 (7–8): 701–718. doi:10.1016/j.apenergy.2007.01.003.
- ↑ Clausius theorem at Wolfram Research
- ↑ Denbigh, K.G., Denbigh, J.S. (1985). Entropy in Relation to Incomplete Knowledge, Cambridge University Press, Cambridge UK, , pp. 43–44.
- ↑ Grandy, W.T., Jr (2008). Entropy and the Time Evolution of Macroscopic Systems, Oxford University Press, Oxford, , pp. 55–58.
- ↑ Entropy Sites — A Guide Content selected by Frank L. Lambert
- ↑ Gyenis, Balazs (2017). "Maxwell and the normal distribution: A colored story of probability, independence, and tendency towards equilibrium". Studies in History and Philosophy of Modern Physics. 57: 53–65. arXiv:1702.01411. Bibcode:2017SHPMP..57...53G. doi:10.1016/j.shpsb.2017.01.001.
- ↑ Hawking, SW (1985). "Arrow of time in cosmology". Phys. Rev. D. 32 (10): 2489–2495. Bibcode:1985PhRvD..32.2489H. doi:10.1103/PhysRevD.32.2489. PMID 9956019.
- ↑ Greene, Brian (2004). The Fabric of the Cosmos. Alfred A. Knopf. p. 171. ISBN 978-0-375-41288-2. https://archive.org/details/fabricofcosmossp00gree.
- ↑ Lebowitz, Joel L. (September 1993). "Boltzmann's Entropy and Time's Arrow" (PDF). Physics Today. 46 (9): 32–38. Bibcode:1993PhT....46i..32L. doi:10.1063/1.881363. Retrieved 2013-02-22.
- ↑ Léon Brillouin Science and Information Theory (Academic Press, 1962) (Dover, 2004)
- ↑ Grandy, W.T. (Jr) (2008), p. 151.
- ↑ Callen, H.B. (1960/1985), p. 15.
- ↑ Lieb, E.H., Yngvason, J. (2003), p. 190.
- ↑ Glansdorff, P., Prigogine, I. (1971).
- ↑ Müller, I. (1985).
- ↑ Müller, I. (2003).
- ↑ Callender, Craig (29 July 2011). "Thermodynamic Asymmetry in Time".
{{cite web}}: Unknown parameter|encyclopedia=ignored (help) - ↑ Halliwell, J.J. (1994). Physical Origins of Time Asymmetry. Cambridge. ISBN 978-0-521-56837-1. chapter 6
- ↑ Schrödinger, E. (1950), p. 192.
Sources
Sources
资料来源
- Adkins, C.J. (1968/1983). Equilibrium Thermodynamics, (1st edition 1968), third edition 1983, Cambridge University Press, Cambridge UK, .
- Atkins, P.W., de Paula, J. (2006). Atkins' Physical Chemistry, eighth edition, W.H. Freeman, New York, .
- Attard, P. (2012). Non-equilibrium Thermodynamics and Statistical Mechanics: Foundations and Applications, Oxford University Press, Oxford UK, .
- Baierlein, R. (1999). Thermal Physics, Cambridge University Press, Cambridge UK, .
- Bailyn, M. (1994). A Survey of Thermodynamics, American Institute of Physics, New York, .
- Blundell, Stephen J.; Blundell, Katherine M. (2010). Concepts in thermal physics (2nd ed.). Oxford: Oxford University Press. doi:10.1093/acprof:oso/9780199562091.001.0001. ISBN 9780199562107. OCLC 607907330. http://cds.cern.ch/record/1235139.
- Boltzmann, L. (1896/1964). Lectures on Gas Theory, translated by S.G. Brush, University of California Press, Berkeley.
- Borgnakke, C., Sonntag., R.E. (2009). Fundamentals of Thermodynamics, seventh edition, Wiley, .
- Buchdahl, H.A. (1966). The Concepts of Classical Thermodynamics, Cambridge University Press, Cambridge UK.
- Bridgman, P.W. (1943). The Nature of Thermodynamics, Harvard University Press, Cambridge MA.
- Callen, H.B. (1960/1985). Thermodynamics and an Introduction to Thermostatistics, (1st edition 1960) 2nd edition 1985, Wiley, New York, .
- C. Carathéodory (1909). [http://gdz.sub.uni-goettingen.de/index.php?id=11&PPN=PPN235181684_0067&DMDID=DMDLOG_0033&L=1
2010年3月15日 "Untersuchungen über die Grundlagen der Thermodynamik"]. Mathematische Annalen. 67 (3): 355–386. doi:10.1007/bf01450409.
Axiom II: In jeder beliebigen Umgebung eines willkürlich vorgeschriebenen Anfangszustandes gibt es Zustände, die durch adiabatische Zustandsänderungen nicht beliebig approximiert werden können. (p.363)
{{cite journal}}: Check|url=value (help); Text "第355-386页" ignored (help); line feed character in|url=at position 93 (help). A translation may be found here. Also a mostly reliable translation is to be found at Kestin, J. (1976). The Second Law of Thermodynamics, Dowden, Hutchinson & Ross, Stroudsburg PA.|quote=Axiom II: In jeder beliebigen Umgebung eines willkürlich vorgeschriebenen Anfangszustandes gibt es Zustände, die durch adiabatische Zustandsänderungen nicht beliebig approximiert werden können. (p.363)|doi=10.1007/bf01450409}}. A translation may be found here. Also a mostly reliable translation is to be found at Kestin, J. (1976). The Second Law of Thermodynamics, Dowden, Hutchinson & Ross, Stroudsburg PA.
|quote=Axiom II: In jeder beliebigen Umgebung eines willkürlich vorgeschriebenen Anfangszustandes gibt es Zustände, die durch adiabatische Zustandsänderungen nicht beliebig approximiert werden können.(p. 363) | doi 10.1007 / bf01450409}.译文可以在这里找到[ http://neo-classical-physics.info/uploads/3/0/6/5/3065888/caratheodory_-_thermodynamics.pdf ]。同时,在 Kestin,j。(1976).热力学第二定律,Dowden,Hutchinson & Ross,Stroudsburg PA. 。
- Carnot, S. (1824/1986). Reflections on the motive power of fire, Manchester University Press, Manchester UK, . Also here.
- Chapman, S., Cowling, T.G. (1939/1970). The Mathematical Theory of Non-uniform gases. An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases, third edition 1970, Cambridge University Press, London.
- Clausius, R. (1850). "Ueber Die Bewegende Kraft Der Wärme Und Die Gesetze, Welche Sich Daraus Für Die Wärmelehre Selbst Ableiten Lassen". Annalen der Physik. 79 (4): 368–397, 500–524. Bibcode:1850AnP...155..500C. doi:10.1002/andp.18501550403. hdl:2027/uc1.$b242250. Retrieved 26 June 2012.
{{cite journal}}: Invalid|ref=harv(help) Translated into English: Clausius, R. (July 1851). "On the Moving Force of Heat, and the Laws regarding the Nature of Heat itself which are deducible therefrom". London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science. 4th. 2 (VIII): 1–21, 102–119. doi:10.1080/14786445108646819. Retrieved 26 June 2012.
|doi=10.1080/14786445108646819}}
10.1080 / 14786445108646819}
- Clausius, R. (1854). "Über eine veränderte Form des zweiten Hauptsatzes der mechanischen Wärmetheorie" (PDF). Annalen der Physik. xciii (12): 481–506. Bibcode:1854AnP...169..481C. doi:10.1002/andp.18541691202. Retrieved 24 March 2014.
{{cite journal}}: Invalid|ref=harv(help) Translated into English: Clausius, R. (July 1856). "On a Modified Form of the Second Fundamental Theorem in the Mechanical Theory of Heat". London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science. 4th. 2: 86. Bibcode:1854AnP...169..481C. Retrieved 24 March 2014. Translated into English: Clausius, R. (July 1856). "On a Modified Form of the Second Fundamental Theorem in the Mechanical Theory of Heat". London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science. 4th. 2: 86. Retrieved 242014年3月.{{cite journal}}: Check date values in:|accessdate=(help); Text "bibcode 1854AnP... 169. 1856年7月" ignored (help); Text "https://www.biodiversitylibrary.org/item/20044#page/100/mode/1up" ignored (help); Text "第2卷" ignored (help); Text "第4页" ignored (help); Text "第86页" ignored (help) Reprinted in: Clausius, R. (1867). The Mechanical Theory of Heat – with its Applications to the Steam Engine and to Physical Properties of Bodies. London: John van Voorst. https://archive.org/details/mechanicaltheor04claugoog. Retrieved 19 June 2012. "editions:PwR_Sbkwa8IC." Reprinted in: Clausius, R. (1867). The Mechanical Theory of Heat – with its Applications to the Steam Engine and to Physical Properties of Bodies. London: John van Voorst. https://archive.org/details/mechanicaltheor04claugoog. Retrieved 19 June 2012
热力学理论及其在蒸汽机和物体物理性质中的应用. "editions:pwr_sbkwa8ic。 2012年6月19日"
}}
}}
- Denbigh, K. (1954/1981). The Principles of Chemical Equilibrium. With Applications in Chemistry and Chemical Engineering, fourth edition, Cambridge University Press, Cambridge UK, .
- Eu, B.C. (2002). Generalized Thermodynamics. The Thermodynamics of Irreversible Processes and Generalized Hydrodynamics, Kluwer Academic Publishers, Dordrecht, .
- Gibbs, J.W. (1876/1878). On the equilibrium of heterogeneous substances, Trans. Conn. Acad., 3: 108-248, 343-524, reprinted in The Collected Works of J. Willard Gibbs, Ph.D, LL. D., edited by W.R. Longley, R.G. Van Name, Longmans, Green & Co., New York, 1928, volume 1, pp. 55–353.
- Griem, H.R. (2005). Principles of Plasma Spectroscopy (Cambridge Monographs on Plasma Physics), Cambridge University Press, New York .
- Glansdorff, P., Prigogine, I. (1971). Thermodynamic Theory of Structure, Stability, and Fluctuations, Wiley-Interscience, London, 1971, .
- Grandy, W.T., Jr (2008). Entropy and the Time Evolution of Macroscopic Systems. Oxford University Press. .
- Greven, A., Keller, G., Warnecke (editors) (2003). Entropy, Princeton University Press, Princeton NJ, .
- Guggenheim, E.A. (1949). 'Statistical basis of thermodynamics', Research, 2: 450–454.
- Guggenheim, E.A. (1967). Thermodynamics. An Advanced Treatment for Chemists and Physicists, fifth revised edition, North Holland, Amsterdam.
- Gyarmati, I. (1967/1970) Non-equilibrium Thermodynamics. Field Theory and Variational Principles, translated by E. Gyarmati and W.F. Heinz, Springer, New York.
- Kittel, C., Kroemer, H. (1969/1980). Thermal Physics, second edition, Freeman, San Francisco CA, .
- Kondepudi, D., Prigogine, I. (1998). Modern Thermodynamics: From Heat Engines to Dissipative Structures, John Wiley & Sons, Chichester, .
- Lebon, G., Jou, D., Casas-Vázquez, J. (2008). Understanding Non-equilibrium Thermodynamics: Foundations, Applications, Frontiers, Springer-Verlag, Berlin, .
- Lieb, E. H.; Yngvason, J. (1999). "The Physics and Mathematics of the Second Law of Thermodynamics". Physics Reports. 310 (1): 1–96. arXiv:cond-mat/9708200. Bibcode:1999PhR...310....1L. doi:10.1016/S0370-1573(98)00082-9.
{{cite journal}}: Invalid|ref=harv(help)
- Lieb, E.H., Yngvason, J. (2003). The Entropy of Classical Thermodynamics, pp. 147–195, Chapter 8 of Entropy, Greven, A., Keller, G., Warnecke (editors) (2003).
- Mandl, F. (1988). Statistical physics (second ed.). Wiley & Sons. ISBN 978-0-471-91533-1.
- Maxwell, J.C. (1867). "On the dynamical theory of gases". Phil. Trans. R. Soc. Lond. 157: 49–88. doi:10.1098/rstl.1867.0004.
- Müller, I. (1985). Thermodynamics, Pitman, London, .
- Müller, I. (2003). Entropy in Nonequilibrium, pp. 79–109, Chapter 5 of Entropy, Greven, A., Keller, G., Warnecke (editors) (2003).
- Münster, A. (1970), Classical Thermodynamics, translated by E.S. Halberstadt, Wiley–Interscience, London, .
- Pippard, A.B. (1957/1966). Elements of Classical Thermodynamics for Advanced Students of Physics, original publication 1957, reprint 1966, Cambridge University Press, Cambridge UK.
- Planck, M. (1897/1903). Treatise on Thermodynamics, translated by A. Ogg, Longmans Green, London, p. 100.
- Planck. M. (1914). The Theory of Heat Radiation, a translation by Masius, M. of the second German edition, P. Blakiston's Son & Co., Philadelphia.
- Planck, M. (1926). Über die Begründung des zweiten Hauptsatzes der Thermodynamik, Sitzungsberichte der Preussischen Akademie der Wissenschaften: Physikalisch-mathematische Klasse: 453–463.
- Pokrovskii V.N. (2005) Extended thermodynamics in a discrete-system approach, Eur. J. Phys. vol. 26, 769-781.
- Pokrovskii, Vladimir N. (2013). "A Derivation of the Main Relations of Nonequilibrium Thermodynamics". ISRN Thermodynamics. 2013: 1–9. doi:10.1155/2013/906136.
- Quinn, T.J. (1983). Temperature, Academic Press, London, .
- Rao, Y.V.C. (2004). An Introduction to thermodynamics. Universities Press. p. 213. ISBN 978-81-7371-461-0. https://books.google.com/books?id=iYWiCXziWsEC&pg=PA213.
- Roberts, J.K., Miller, A.R. (1928/1960). Heat and Thermodynamics, (first edition 1928), fifth edition, Blackie & Son Limited, Glasgow.
- Schrödinger, E. (1950). Irreversibility, Proc. R. Ir. Acad., A53: 189–195.
- ter Haar, D., Wergeland, H. (1966). Elements of Thermodynamics, Addison-Wesley Publishing, Reading MA.
- Thomson, W. (1851). "On the Dynamical Theory of Heat, with numerical results deduced from Mr Joule's equivalent of a Thermal Unit, and M. Regnault's Observations on Steam". Transactions of the Royal Society of Edinburgh. XX (part II): 261–268, 289–298.
{{cite journal}}: Invalid|ref=harv(help) Also published in Thomson, W. (December 1852). "On the Dynamical Theory of Heat, with numerical results deduced from Mr Joule's equivalent of a Thermal Unit, and M. Regnault's Observations on Steam". Philos. Mag. 4. IV (22): 13. Retrieved 25 June 2012.
- Thomson, W. (1852). On the universal tendency in nature to the dissipation of mechanical energy Philosophical Magazine, Ser. 4, p. 304.
- Tisza, L. (1966). Generalized Thermodynamics, M.I.T Press, Cambridge MA.
- Truesdell, C. (1980). The Tragicomical History of Thermodynamics 1822–1854, Springer, New York, .
- Uffink, J. (2001). Bluff your way in the second law of thermodynamics, Stud. Hist. Phil. Mod. Phys., 32(3): 305–394.
- Uffink, J. (2003). Irreversibility and the Second Law of Thermodynamics, Chapter 7 of Entropy, Greven, A., Keller, G., Warnecke (editors) (2003), Princeton University Press, Princeton NJ, .
- Uhlenbeck, G.E., Ford, G.W. (1963). Lectures in Statistical Mechanics, American Mathematical Society, Providence RI.
- Zemansky, M.W. (1968). Heat and Thermodynamics. An Intermediate Textbook, fifth edition, McGraw-Hill Book Company, New York.
Further reading
Further reading
进一步阅读
- Goldstein, Martin, and Inge F., 1993. The Refrigerator and the Universe. Harvard Univ. Press. Chpts. 4–9 contain an introduction to the Second Law, one a bit less technical than this entry.
- Leff, Harvey S., and Rex, Andrew F. (eds.) 2003. Maxwell's Demon 2 : Entropy, classical and quantum information, computing. Bristol UK; Philadelphia PA: Institute of Physics.
- Halliwell, J.J. (1994). Physical Origins of Time Asymmetry. Cambridge. ISBN 978-0-521-56837-1.(technical).
- Carnot, Sadi (1890). Thurston, Robert Henry. ed. Reflections on the Motive Power of Heat and on Machines Fitted to Develop That Power. New York: J. Wiley & Sons. (full text of 1897 ed.) (html)
- Stephen Jay Kline (1999). The Low-Down on Entropy and Interpretive Thermodynamics, La Cañada, CA: DCW Industries. .
- Kostic, M (2011). Revisiting The Second Law of Energy Degradation and Entropy Generation: From Sadi Carnot's Ingenious Reasoning to Holistic Generalization. AIP Conference Proceedings. 1411. pp. 327–350. Bibcode 2011AIPC.1411..327K. doi:10.1063/1.3665247. ISBN 978-0-7354-0985-9. also at [1].
External links
External links
外部链接
- Stanford Encyclopedia of Philosophy: "Philosophy of Statistical Mechanics" – by Lawrence Sklar.
- Second law of thermodynamics in the MIT Course Unified Thermodynamics and Propulsion from Prof. Z. S. Spakovszky
- E.T. Jaynes, 1988, "The evolution of Carnot's principle," in G. J. Erickson and C. R. Smith (eds.)Maximum-Entropy and Bayesian Methods in Science and Engineering, Vol 1: p. 267.
- The Second Law of Thermodynamics, BBC Radio 4 discussion with John Gribbin, Peter Atkins & Monica Grady (In Our Time, Dec. 16, 2004)
- Entropy (journal), 2004
Category:Concepts in physics
分类: 物理概念
2
2
Category:Non-equilibrium thermodynamics
类别: 非平衡态热力学
Category:Philosophy of thermal and statistical physics
类别: 热力学和统计物理学哲学
This page was moved from wikipedia:en:Second law of thermodynamics. Its edit history can be viewed at 热力学第二定律/edithistory
- Leff, Harvey S., and Rex, Andrew F. (eds.) 2003. Maxwell's Demon 2 : Entropy, classical and quantum information, computing. Bristol UK; Philadelphia PA: Institute of Physics.
- Lieb, E. H.; Yngvason, J. (1999). "The Physics and Mathematics of the Second Law of Thermodynamics". Physics Reports. 310 (1): 1–96. arXiv:cond-mat/9708200. Bibcode:1999PhR...310....1L. doi:10.1016/S0370-1573(98)00082-9.
- Lebon, G., Jou, D., Casas-Vázquez, J. (2008). Understanding Non-equilibrium Thermodynamics: Foundations, Applications, Frontiers, Springer-Verlag, Berlin, .
- Kondepudi, D., Prigogine, I. (1998). Modern Thermodynamics: From Heat Engines to Dissipative Structures, John Wiley & Sons, Chichester, .
- Greven, A., Keller, G., Warnecke (editors) (2003). Entropy, Princeton University Press, Princeton NJ, .
- Grandy, W.T., Jr (2008). Entropy and the Time Evolution of Macroscopic Systems. Oxford University Press. .
- Glansdorff, P., Prigogine, I. (1971). Thermodynamic Theory of Structure, Stability, and Fluctuations, Wiley-Interscience, London, 1971, .
- Eu, B.C. (2002). Generalized Thermodynamics. The Thermodynamics of Irreversible Processes and Generalized Hydrodynamics, Kluwer Academic Publishers, Dordrecht, .
- Bailyn, M. (1994). A Survey of Thermodynamics, American Institute of Physics, New York, .
- Baierlein, R. (1999). Thermal Physics, Cambridge University Press, Cambridge UK, .
- Attard, P. (2012). Non-equilibrium Thermodynamics and Statistical Mechanics: Foundations and Applications, Oxford University Press, Oxford UK, .
- Atkins, P.W., de Paula, J. (2006). Atkins' Physical Chemistry, eighth edition, W.H. Freeman, New York, .
- CS1 errors: unsupported parameter
- 调用重复模板参数的页面
- Articles with hatnote templates targeting a nonexistent page
- CS1 errors: unrecognized parameter
- CS1 errors: invisible characters
- CS1 errors: URL
- CS1 errors: invalid parameter value
- CS1: long volume value
- CS1 errors: dates
- Concepts in physics
- Laws of thermodynamics
- Non-equilibrium thermodynamics
- Philosophy of thermal and statistical physics
- 待整理页面