“量子计算”的版本间的差异

2020年11月5日 (四) 21:02的版本

此词条暂由水流心不竞初译，未经审校，带来阅读不便，请见谅。

Quantum computing is the use of quantum phenomena such as superposition and entanglement to perform computation. Computers that perform quantum computations are known as quantum computers.^[1]^:I-5 Quantum computers are believed to be able to solve certain computational problems, such as integer factorization (which underlies RSA encryption), substantially faster than classical computers. The study of quantum computing is a subfield of quantum information science.

Quantum computing is the use of quantum phenomena such as superposition and entanglement to perform computation. Computers that perform quantum computations are known as quantum computers. Quantum computers are believed to be able to solve certain computational problems, such as integer factorization (which underlies RSA encryption), substantially faster than classical computers. The study of quantum computing is a subfield of quantum information science.

量子计算Quantum computing是利用量子现象，如叠加和纠缠来执行计算。执行量子计算的计算机被称为量子计算机。量子计算机被认为能够解决某些计算问题，比如 RSA 加密的基础整数分解，比传统计算机快得多。 量子计算的研究是量子信息科学的一个分支。

< ! -- 历史 -- >

Quantum computing began in the early 1980s, when physicist Paul Benioff proposed a quantum mechanical model of the Turing machine.^[2] Richard Feynman and Yuri Manin later suggested that a quantum computer had the potential to simulate things that a classical computer could not.^[3]^[4] In 1994, Peter Shor developed a quantum algorithm for factoring integers that had the potential to decrypt RSA-encrypted communications.^[5] Despite ongoing experimental progress since the late 1990s, most researchers believe that "fault-tolerant quantum computing [is] still a rather distant dream."^[6] In recent years, investment into quantum computing research has increased in both the public and private sector.^[7]^[8] On 23 October 2019, Google AI, in partnership with the U.S. National Aeronautics and Space Administration (NASA), claimed to have performed a quantum computation that is infeasible on any classical computer.^[9]

Quantum computing began in the early 1980s, when physicist Paul Benioff proposed a quantum mechanical model of the Turing machine. Richard Feynman and Yuri Manin later suggested that a quantum computer had the potential to simulate things that a classical computer could not. In 1994, Peter Shor developed a quantum algorithm for factoring integers that had the potential to decrypt RSA-encrypted communications. Despite ongoing experimental progress since the late 1990s, most researchers believe that "fault-tolerant quantum computing [is] still a rather distant dream." In recent years, investment into quantum computing research has increased in both the public and private sector. On 23 October 2019, Google AI, in partnership with the U.S. National Aeronautics and Space Administration (NASA), claimed to have performed a quantum computation that is infeasible on any classical computer.

量子计算始于20世纪80年代早期，当时物理学家保罗 · 贝尼奥夫提出了图灵机的量子力学模型。理查德 · 费曼和尤里 · 曼宁后来提出，量子计算机具有模拟传统计算机所不具备的潜力。1994年，Peter Shor 开发了一种量子算法，用于分解整数，这种算法有可能解密 rsa 加密的通信。尽管自20世纪90年代后期以来，实验取得了进展，但大多数研究人员认为，“容错量子计算机仍然是一个相当遥远的梦想。”近年来，量子计算研究的投资在公共和私营部门都有所增加。2019年10月23日，谷歌人工智能与美国宇航局合作，声称已经完成了在任何传统计算机上都不可能完成的量子计算。

< ! -- 量子计算的基本原理 -- >

There are several models of quantum computing, including the quantum circuit model, quantum Turing machine, adiabatic quantum computer, one-way quantum computer, and various quantum cellular automata. The most widely used model is the quantum circuit. Quantum circuits are based on the quantum bit, or "qubit", which is somewhat analogous to the bit in classical computation. Qubits can be in a 1 or 0 quantum state, or they can be in a superposition of the 1 and 0 states. However, when qubits are measured the result of the measurement is always either a 0 or a 1; the probabilities of these two outcomes depend on the quantum state that the qubits were in immediately prior to the measurement. Computation is performed by manipulating qubits with quantum logic gates, which are somewhat analogous to classical logic gates.

量子计算有几种模型，包括量子电路模型、量子图灵机、绝热量子计算机、单向量子计算机和各种量子细胞自动机。使用最广泛的模型是 量子电路Quantum circuits 。量子电路是基于量子比特或 “量子比特”"qubit"的，它在某种程度上类似于经典计算中的比特。 量子比特可以处于1或0的量子态，也可以处于1和0的叠加态。然而，当量子比特被测量时，测量结果总是0或1; 这两个结果的概率取决于量子比特在测量之前所处的量子状态。计算是通过 量子逻辑门Quantum logic gates操纵量子比特来完成的，这在某种程度上类似于经典逻辑门。

< ! -- 物理实现 -- >

There are currently two main approaches to physically implementing a quantum computer: analog and digital. Analog approaches are further divided into quantum simulation, quantum annealing, and adiabatic quantum computation. Digital quantum computers use quantum logic gates to do computation. Both approaches use qubits.^[1]^:2–13 There are currently a number of significant obstacles in the way of constructing useful quantum computers. In particular, it is difficult to maintain the quantum states of qubits as they are prone to quantum decoherence, and quantum computers require significant error correction as they are far more prone to errors than classical computers.^[10]^[11]

There are currently two main approaches to physically implementing a quantum computer: analog and digital. Analog approaches are further divided into quantum simulation, quantum annealing, and adiabatic quantum computation. Digital quantum computers use quantum logic gates to do computation. Both approaches use qubits.

目前实现量子计算机主要有两种方法: 模拟和数字。模拟方法进一步分为量子模拟、量子退火模拟和绝热量子计算。数字量子计算机使用量子逻辑门进行计算。两种方法都使用量子位。

< ! -- 可计算性和复杂性 -- >

Any computational problem that can be solved by a classical computer can also, in principle, be solved by a quantum computer. Conversely, quantum computers obey the Church–Turing thesis; that is, any computational problem that can be solved by a quantum computer can also be solved by a classical computer. While this means that quantum computers provide no additional advantages over classical computers in terms of computability, they do in theory enable the design of algorithms for certain problems that have significantly lower time complexities than known classical algorithms. Notably, quantum computers are believed to be able to quickly solve certain problems that no classical computer could solve in any feasible amount of time—a feat known as "quantum supremacy." The study of the computational complexity of problems with respect to quantum computers is known as quantum complexity theory.

Any computational problem that can be solved by a classical computer can also, in principle, be solved by a quantum computer. Conversely, quantum computers obey the Church–Turing thesis; that is, any computational problem that can be solved by a quantum computer can also be solved by a classical computer. While this means that quantum computers provide no additional advantages over classical computers in terms of computability, they do in theory enable the design of algorithms for certain problems that have significantly lower time complexities than known classical algorithms. Notably, quantum computers are believed to be able to quickly solve certain problems that no classical computer could solve in any feasible amount of time—a feat known as "quantum supremacy." The study of the computational complexity of problems with respect to quantum computers is known as quantum complexity theory.

任何可以由经典计算机解决的计算问题问题，原则上也可以由量子计算机解决。相反，量子计算机遵循 Church-Turing 理论; 也就是说，任何可以由量子计算机解决的计算问题问题也可以由经典计算机解决。虽然这意味着量子计算机在可计算性方面没有比传统计算机提供额外的优势，但在理论上，它们确实能够为某些问题设计算法，这些问题的时间复杂性明显低于已知的经典算法。值得注意的是，人们相信量子计算机能够快速解决某些问题，而这些问题是任何传统计算机都无法在任何可行的时间内解决的——这一壮举被称为“量子优势”研究量子计算机问题的计算复杂性被称为量子复杂性理论。

Quantum operations量子计算

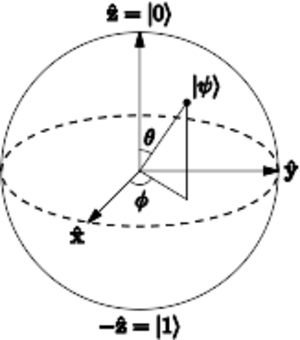
The Bloch sphere is a representation of a qubit, the fundamental building block of quantum computers.

布洛赫球体是量子计算机的基本构件——量子比特的表示。

The prevailing model of quantum computation describes the computation in terms of a network of quantum logic gates.^[12]

The prevailing model of quantum computation describes the computation in terms of a network of quantum logic gates.

当前流行的量子计算模型用量子逻辑门网络来描述计算。

A memory consisting of [math]\displaystyle{ n }[/math] bits of information has [math]\displaystyle{ 2^n }[/math] possible states. A vector representing all memory states thus has [math]\displaystyle{ 2^n }[/math] entries (one for each state). This vector is viewed as a probability vector and represents the fact that the memory is to be found in a particular state.

A memory consisting of [math]\displaystyle{ n }[/math] bits of information has [math]\displaystyle{ 2^n }[/math] possible states. A vector representing all memory states thus has [math]\displaystyle{ 2^n }[/math] entries (one for each state). This vector is viewed as a probability vector and represents the fact that the memory is to be found in a particular state.

一个由[math]\displaystyle{ n }[/math] 比特信息组成的内存有 [math]\displaystyle{ 2^n }[/math] 可能的状态。因此，一个代表所有内存状态的向量具有 [math]\displaystyle{ 2^n }[/math] 条目(每个状态一个)。这个向量被看作是一个概率向量，它代表了一个事实，即内存将在一个特定的状态下被找到。

In the classical view, one entry would have a value of 1 (i.e. a 100% probability of being in this state) and all other entries would be zero.

在经典的观点中，一个条目的值为1(即。100% 的概率处于这种状态) ，所有其他条目都是零。

In quantum mechanics, probability vectors are generalized to density operators. This is the technically rigorous mathematical foundation for quantum logic gates, but the intermediate quantum state vector formalism is usually introduced first because it is conceptually simpler. This article focuses on the quantum state vector formalism for simplicity.

在量子力学中，概率向量被推广到 密度算子Density operators。这是技术上严格的量子逻辑门的数学基础，但中间量子态向量形式通常首先介绍，因为它在概念上比较简单。为了简单起见，本文着重讨论量子态向量形式。

We begin by considering a simple memory consisting of only one bit. This memory may be found in one of two states: the zero state or the one state. We may represent the state of this memory using Dirac notation so that

我们首先考虑一个只包含一个位的简单内存。这种记忆可以在两种状态中的一种中找到: 零状态或一种状态。我们可以用 狄拉克符号Dirac notation来表示这段记忆的状态

[math]\displaystyle{ \lt math display="block"\gt “数学显示屏” |0\rangle := \begin{pmatrix} 1 \\ 0 \end{pmatrix};\quad |0\rangle := \begin{pmatrix} 1 \\ 0 \end{pmatrix};\quad 0 rangle: = begin { pmatrix }10 end { pmatrix } ; quad |1\rangle := \begin{pmatrix} 0 \\ 1 \end{pmatrix} |1\rangle := \begin{pmatrix} 0 \\ 1 \end{pmatrix} 1 rangle: = begin { pmatrix }01 end { pmatrix } }[/math]

</math>

数学

A quantum memory may then be found in any quantum superposition [math]\displaystyle{ |\psi\rangle }[/math] of the two classical states [math]\displaystyle{ |0\rangle }[/math] and [math]\displaystyle{ |1\rangle }[/math]:

一个量子内存可能会在任何一个经典状态下的量子态叠加原理中被发现:

[math]\displaystyle{ \lt math display="block"\gt “数学显示屏” |\psi\rangle := \alpha\,|0\rangle + \beta\,|1\rangle |\psi\rangle := \alpha\,|0\rangle + \beta\,|1\rangle | psi rangle: = alpha，| 0 rangle + beta，| 1 rangle = \begin{pmatrix} \alpha \\ \beta \end{pmatrix};\quad = \begin{pmatrix} \alpha \\ \beta \end{pmatrix};\quad 2.1.2.2.2.2.3.3.3.3.3.3.3.3.3.3.3.3.3.4.3.3.3.4.3.3.3.3.3.3.3.3.3.4.3.3.3.3.3.3.3.3.3.3.3.3.3.3.3.3 |\alpha|^2 + |\beta|^2 = 1. |\alpha|^2 + |\beta|^2 = 1. | alpha | ^ 2 + | beta | ^ 2 = 1. }[/math]

</math>

数学

In general, the coefficients [math]\displaystyle{ \alpha }[/math] and [math]\displaystyle{ \beta }[/math] are complex numbers. In this scenario, one qubit of information is said to be encoded into the quantum memory. The state [math]\displaystyle{ |\psi\rangle }[/math] is not itself a probability vector but can be connected with a probability vector via a measurement operation. If the quantum memory is measured to determine if the state is [math]\displaystyle{ |0\rangle }[/math] or [math]\displaystyle{ |1\rangle }[/math] (this is known as a computational basis measurement), the zero state would be observed with probability [math]\displaystyle{ |\alpha|^2 }[/math] and the one state with probability [math]\displaystyle{ |\beta|^2 }[/math]. The numbers [math]\displaystyle{ \alpha }[/math] and [math]\displaystyle{ \beta }[/math] are called quantum amplitudes.

一般来说，系数 [math]\displaystyle{ \alpha }[/math] 和 [math]\displaystyle{ \beta }[/math]都是复数。在这种情况下，一个量子比特的信息被称为被编码到量子存储器中。状态[math]\displaystyle{ |\psi\rangle }[/math]本身不是一个概率向量，但可以通过一个测量操作与一个概率向量相连。如果量子内存被测量以确定其状态是否为 [math]\displaystyle{ |0\rangle }[/math] 或[math]\displaystyle{ |1\rangle }[/math](这被称为计算基础测量) ，那么零状态将被观察到概率 [math]\displaystyle{ |\alpha|^2 }[/math]和概率 [math]\displaystyle{ |\beta|^2 }[/math] 。数字 [math]\displaystyle{ \alpha }[/math] 和 [math]\displaystyle{ \beta }[/math]被称为量子幅值。

The state of this one-qubit quantum memory can be manipulated by applying quantum logic gates, analogous to how classical memory can be manipulated with classical logic gates. One important gate for both classical and quantum computation is the NOT gate, which can be represented by a matrix

这种单比特量子存储器的状态可以通过量子逻辑门来控制，类似于用经典逻辑门来控制经典存储器。对经典和量子计算都重要的一个门是 非门NOT gate，它可以用矩阵表示

[math]\displaystyle{ X := \begin{pmatrix} 0 & 1 \\ 1 & 0 \end{pmatrix}. }[/math]

数学显示 = “ block” > x: = begin { pmatrix }0 & 11 & 0 end { pmatrix }

Mathematically, the application of such a logic gate to a quantum state vector is modelled with matrix multiplication. Thus [math]\displaystyle{ X|0\rangle = |1\rangle }[/math] and [math]\displaystyle{ X|1\rangle = |0\rangle }[/math].

在数学上，这种逻辑门应用于量子态矢量是用矩阵乘法模型来建模的。因此 [math]\displaystyle{ X|0\rangle = |1\rangle }[/math] 和 [math]\displaystyle{ X|1\rangle = |0\rangle }[/math]。

The mathematics of single qubit gates can be extended to operate on multiqubit quantum memories in two important ways. One way is simply to select a qubit and apply that gate to the target qubit whilst leaving the remainder of the memory unaffected. Another way is to apply the gate to its target only if another part of the memory is in a desired state. These two choices can be illustrated using another example. The possible states of a two-qubit quantum memory are

单个量子比特门的数学可以通过两种重要的方式扩展到对多量子比特量子存储器的操作。一种方法是简单地选择一个量子位并将该门应用于目标量子位，同时不影响其余的内存。另一种方法是，只有当内存的另一部分处于所需状态时，才将门应用于目标。这两种选择可以用另一个例子来说明。两比特量子存储器的可能状态是

[math]\displaystyle{ \lt math display="block"\gt “数学显示屏” |00\rangle := \begin{pmatrix} 1 \\ 0 \\ 0 \\ 0 \end{pmatrix};\quad |00\rangle := \begin{pmatrix} 1 \\ 0 \\ 0 \\ 0 \end{pmatrix};\quad 1000 end { pmatrix } ; quad |01\rangle := \begin{pmatrix} 0 \\ 1 \\ 0 \\ 0 \end{pmatrix};\quad |01\rangle := \begin{pmatrix} 0 \\ 1 \\ 0 \\ 0 \end{pmatrix};\quad 01 rangle: = begin { pmatrix }0100 end { pmatrix } ; quad |10\rangle := \begin{pmatrix} 0 \\ 0 \\ 1 \\ 0 \end{pmatrix};\quad |10\rangle := \begin{pmatrix} 0 \\ 0 \\ 1 \\ 0 \end{pmatrix};\quad 10 rangle: = begin { pmatrix }010 end { pmatrix } ; quad |11\rangle := \begin{pmatrix} 0 \\ 0 \\ 0 \\ 1 \end{pmatrix}. |11\rangle := \begin{pmatrix} 0 \\ 0 \\ 0 \\ 1 \end{pmatrix}. 11 rangle: = begin { pmatrix }001 end { pmatrix }. }[/math]

</math>

数学

The CNOT gate can then be represented using the following matrix:

然后， 量子受控非门CNOT gate可以用以下矩阵表示:

[math]\displaystyle{ \lt math display="block"\gt “数学显示屏” CNOT := CNOT := = \begin{pmatrix} \begin{pmatrix} 开始{ pmatrix } 1 & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 1 \\ 0 & 0 & 0 & 1 \\ 0 & 0 & 0 & 1 \\ 0 & 0 & 1 & 0 0 & 0 & 1 & 0 0 & 0 & 1 & 0 \end{pmatrix}. \end{pmatrix}. 结束{ pmatrix }。 }[/math]

</math>

数学

As a mathematical consequence of this definition, [math]\displaystyle{ CNOT|00\rangle = |00\rangle }[/math], [math]\displaystyle{ CNOT|01\rangle = |01\rangle }[/math], [math]\displaystyle{ CNOT|10\rangle = |11\rangle }[/math], and [math]\displaystyle{ CNOT|11\rangle = |10\rangle }[/math]. In other words, the CNOT applies a NOT gate ([math]\displaystyle{ X }[/math] from before) to the second qubit if and only if the first qubit is in the state [math]\displaystyle{ |1\rangle }[/math]. If the first qubit is [math]\displaystyle{ |0\rangle }[/math], nothing is done to either qubit.

作为这个定义的数学推论，[math]\displaystyle{ CNOT|00\rangle = |00\rangle }[/math], [math]\displaystyle{ CNOT|01\rangle = |01\rangle }[/math], [math]\displaystyle{ CNOT|10\rangle = |11\rangle }[/math], 和[math]\displaystyle{ CNOT|11\rangle = |10\rangle }[/math]。换句话说，当且仅当第一个量子位处于状态 [math]\displaystyle{ |1\rangle }[/math] 时，CNOT 对第二个量子位应用 NOT 门([math]\displaystyle{ X }[/math])。如果第一个量子位是 [math]\displaystyle{ |0\rangle }[/math]，则对任何一个量子位都不做处理。

In summary, a quantum computation can be described as a network of quantum logic gates and measurements. Any measurement can be deferred to the end of a quantum computation, though this deferment may come at a computational cost. Because of this possibility of deferring a measurement, most quantum circuits depict a network consisting only of quantum logic gates and no measurements. More information can be found in the following articles: universal quantum computer, Shor's algorithm, Grover's algorithm, Deutsch–Jozsa algorithm, amplitude amplification, quantum Fourier transform, quantum gate, quantum adiabatic algorithm and quantum error correction.

总之， 量子计算可以描述为一个由量子逻辑门和测量组成的网络。任何测量都可以推迟到 量子计算结束时进行，尽管这种推迟可能会带来计算成本。由于这种延迟测量的可能性，大多数量子电路描述的网络只有量子逻辑门而没有测量。更多信息可以在以下文章中找到: 通用量子计算机，Shor 算法，Grover 算法，Deutsch-Jozsa 算法，振幅放大，量子傅里叶变换Quantum Fourier transform，量子门，量子绝热算法和量子误差修正Quantum error correction。

Any quantum computation can be represented as a network of quantum logic gates from a fairly small family of gates. A choice of gate family that enables this construction is known as a universal gate set. One common such set includes all single-qubit gates as well as the CNOT gate from above. This means any quantum computation can be performed by executing a sequence of single-qubit gates together with CNOT gates. Though this gate set is infinite, it can be replaced with a finite gate set by appealing to the Solovay-Kitaev theorem. The representation of multiple qubits can be shown as Qsphere.

任何 量子计算都可以表示为一个量子逻辑门网络，它来自一个相当小的量子门家族。使这种结构成为可能的门系列的选择被称为通用门系列。一个常见的这样的集合包括所有的单量子比特门以及上面的量子受控非门CNOT 门。这意味着任何量子计算都可以通过执行一系列带有量子受控非门CNOT 门的单量子比特门来完成。虽然这个门集合是无限的，但是它可以通过引用 Solovay-Kitaev 定理用一个有限的门集合来代替。多个量子位的表示可以用 Qsphere 来表示。

Potential applications 潜在应用

Cryptography 密码学

Integer factorization, which underpins the security of public key cryptographic systems, is believed to be computationally infeasible with an ordinary computer for large integers if they are the product of few prime numbers (e.g., products of two 300-digit primes).^[13] By comparison, a quantum computer could efficiently solve this problem using Shor's algorithm to find its factors. This ability would allow a quantum computer to break many of the cryptographic systems in use today, in the sense that there would be a polynomial time (in the number of digits of the integer) algorithm for solving the problem. In particular, most of the popular public key ciphers are based on the difficulty of factoring integers or the discrete logarithm problem, both of which can be solved by Shor's algorithm. In particular, the RSA, Diffie–Hellman, and elliptic curve Diffie–Hellman algorithms could be broken. These are used to protect secure Web pages, encrypted email, and many other types of data. Breaking these would have significant ramifications for electronic privacy and security.

Integer factorization, which underpins the security of public key cryptographic systems, is believed to be computationally infeasible with an ordinary computer for large integers if they are the product of few prime numbers (e.g., products of two 300-digit primes). By comparison, a quantum computer could efficiently solve this problem using Shor's algorithm to find its factors. This ability would allow a quantum computer to break many of the cryptographic systems in use today, in the sense that there would be a polynomial time (in the number of digits of the integer) algorithm for solving the problem. In particular, most of the popular public key ciphers are based on the difficulty of factoring integers or the discrete logarithm problem, both of which can be solved by Shor's algorithm. In particular, the RSA, Diffie–Hellman, and elliptic curve Diffie–Hellman algorithms could be broken. These are used to protect secure Web pages, encrypted email, and many other types of data. Breaking these would have significant ramifications for electronic privacy and security.

整数因式分解是公钥密码系统安全性的基础，如果大整数是几个素数的乘积（例如，两个300位素数的乘积），那么在普通计算机上计算大整数是不可行的。相比之下，量子计算机可以有效地解决这个问题，使用 肖尔Shor算法来寻找它的因子。这种能力将使量子计算机能够破解目前使用的许多密码系统，在这个意义上，将有一个多项式时间（整数位数）算法来解决这个问题。特别是目前流行的公钥密码算法大多是基于整数的因式分解困难或离散对数问题，这两个问题都可以用Shor算法来解决。特别是RSA、Diffie-Hellman和椭圆曲线Diffie-Hellman算法可能会被打破。它们用于保护安全网页、加密电子邮件和许多其他类型的数据。破坏这些将对电子隐私和安全产生重大影响。

However, other cryptographic algorithms do not appear to be broken by those algorithms.^[14]^[15] Some public-key algorithms are based on problems other than the integer factorization and discrete logarithm problems to which Shor's algorithm applies, like the McEliece cryptosystem based on a problem in coding theory.^[14]^[16] Lattice-based cryptosystems are also not known to be broken by quantum computers, and finding a polynomial time algorithm for solving the dihedral hidden subgroup problem, which would break many lattice based cryptosystems, is a well-studied open problem.^[17] It has been proven that applying Grover's algorithm to break a symmetric (secret key) algorithm by brute force requires time equal to roughly 2^n/2 invocations of the underlying cryptographic algorithm, compared with roughly 2ⁿ in the classical case,^[18] meaning that symmetric key lengths are effectively halved: AES-256 would have the same security against an attack using Grover's algorithm that AES-128 has against classical brute-force search (see Key size).

However, other cryptographic algorithms do not appear to be broken by those algorithms. Some public-key algorithms are based on problems other than the integer factorization and discrete logarithm problems to which Shor's algorithm applies, like the McEliece cryptosystem based on a problem in coding theory. Lattice-based cryptosystems are also not known to be broken by quantum computers, and finding a polynomial time algorithm for solving the dihedral hidden subgroup problem, which would break many lattice based cryptosystems, is a well-studied open problem. It has been proven that applying Grover's algorithm to break a symmetric (secret key) algorithm by brute force requires time equal to roughly 2^n/2 invocations of the underlying cryptographic algorithm, compared with roughly 2ⁿ in the classical case, meaning that symmetric key lengths are effectively halved: AES-256 would have the same security against an attack using Grover's algorithm that AES-128 has against classical brute-force search (see Key size).

然而，其他密码算法似乎并没有被这些算法破解。有些公钥算法是基于除整数分解和离散对数问题以外的问题，Shor算法适用于这些问题，例如McEliece密码体制基于编码理论中的一个问题。基于格的密码体制也不被量子计算机破解，寻找一个多项式时间算法来解决二面体隐子群问题，这将打破许多基于格的密码体制，是一个研究得很好的开放性问题。已经证明，应用Grover算法以暴力破解对称（密钥）算法所需的时间大约相当于基础加密算法的2次调用^n/2，而在经典情况下大约需要2ⁿ，这意味着对称密钥长度有效地减半：AES-256对于使用Grover算法的攻击的安全性与AES-128针对经典暴力搜索的安全性相同（参见密钥大小）。

Quantum cryptography could potentially fulfill some of the functions of public key cryptography. Quantum-based cryptographic systems could, therefore, be more secure than traditional systems against quantum hacking.^[19]

Quantum cryptography could potentially fulfill some of the functions of public key cryptography. Quantum-based cryptographic systems could, therefore, be more secure than traditional systems against quantum hacking.

量子密码学可以实现公开密钥加密的一些功能。因此，基于量子的加密系统可能比传统系统更安全，可以抵御量子黑客攻击。

Quantum search 量子搜索

Besides factorization and discrete logarithms, quantum algorithms offering a more than polynomial speedup over the best known classical algorithm have been found for several problems,^[20] including the simulation of quantum physical processes from chemistry and solid state physics, the approximation of Jones polynomials, and solving Pell's equation. No mathematical proof has been found that shows that an equally fast classical algorithm cannot be discovered, although this is considered unlikely.^[21] However, quantum computers offer polynomial speedup for some problems. The most well-known example of this is quantum database search, which can be solved by Grover's algorithm using quadratically fewer queries to the database than that are required by classical algorithms. In this case, the advantage is not only provable but also optimal, it has been shown that Grover's algorithm gives the maximal possible probability of finding the desired element for any number of oracle lookups. Several other examples of provable quantum speedups for query problems have subsequently been discovered, such as for finding collisions in two-to-one functions and evaluating NAND trees.^{[citation needed]}

}}模板:Self-published inline</ref>然而，量子计算机为某些问题提供了多项式加速。这方面最著名的例子是“量子数据库搜索”，它可以通过格罗夫算法使用比经典算法要求的更少的对数据库的查询来解决。在这种情况下，这种优势不仅是可证明的，而且是最优的，已经证明Grover的算法为任何数量的oracle查询提供了找到所需元素的最大可能概率。随后又发现了其他几个可证明的量子加速的例子，例如在两对一函数中寻找碰撞和评估NAND树。^{[citation needed]}

There is no searchable structure in the collection of possible answers,

在可能的答案的集合中没有可搜索的结构,

The number of possible answers to check is the same as the number of inputs to the algorithm, and

要检查的可能答案的数量与算法的输入数量相同，以及

Problems that can be addressed with Grover's algorithm have the following properties:^{[citation needed]}

There exists a boolean function which evaluates each input and determines whether it is the correct answer

存在一个布尔函数，它评估每个输入，并确定它是否是正确的答案

There is no searchable structure in the collection of possible answers,

在可能的答案集合中没有可搜索的结构，

The number of possible answers to check is the same as the number of inputs to the algorithm, and

要检查的可能答案的数量与算法的输入数量相同，并且 For problems with all these properties, the running time of Grover's algorithm on a quantum computer will scale as the square root of the number of inputs (or elements in the database), as opposed to the linear scaling of classical algorithms. A general class of problems to which Grover's algorithm can be applied is Boolean satisfiability problem. In this instance, the database through which the algorithm is iterating is that of all possible answers. An example (and possible) application of this is a password cracker that attempts to guess the password or secret key for an encrypted file or system. Symmetric ciphers such as Triple DES and AES are particularly vulnerable to this kind of attack. This application of quantum computing is a major interest of government agencies.

对于具有所有这些性质的问题，Grover算法在量子计算机上的运行时间将按输入（或数据库中元素）数量的平方根来缩放，而不是经典算法的线性缩放。Grover算法可以应用的一类问题是布尔可满足性问题。在此例中，算法迭代使用的数据库是所有可能答案的数据库。这方面的一个例子（也是可能的）是一个密码破解程序，它试图猜测加密文件或系统的密码或密钥。对称密码如三重DES和AES特别容易受到这种攻击。量子计算的这种应用是政府机构的主要兴趣。

There exists a boolean function which evaluates each input and determines whether it is the correct answer

存在一个布尔函数，它计算每个输入并确定它是否是正确的答案

For problems with all these properties, the running time of Grover's algorithm on a quantum computer will scale as the square root of the number of inputs (or elements in the database), as opposed to the linear scaling of classical algorithms. A general class of problems to which Grover's algorithm can be applied^[22] is Boolean satisfiability problem. In this instance, the database through which the algorithm is iterating is that of all possible answers. An example (and possible) application of this is a password cracker that attempts to guess the password or secret key for an encrypted file or system. Symmetric ciphers such as Triple DES and AES are particularly vulnerable to this kind of attack.^{[citation needed]} This application of quantum computing is a major interest of government agencies.^[23]

对于具有所有这些性质的问题，Grover算法在量子计算机上的运行时间将按输入（或数据库中元素）数量的平方根进行缩放，而不是经典算法的线性缩放。一类可以应用Grover算法的一般问题是布尔可满足性问题。在本例中，算法迭代使用的“数据库”是所有可能答案的数据库。这方面的一个例子（也是可能的）应用是一个密码破解器试图猜测加密文件或系统的密码或密钥。对称密码例如Triple DES和 AES特别容易受到此类攻击。{{引文需要{日期=2019年11月}}量子计算的这一应用是政府机构的主要兴趣。

Since chemistry and nanotechnology rely on understanding quantum systems, and such systems are impossible to simulate in an efficient manner classically, many believe quantum simulation will be one of the most important applications of quantum computing. Quantum simulation could also be used to simulate the behavior of atoms and particles at unusual conditions such as the reactions inside a collider.

由于化学和纳米技术依赖于对量子系统的理解，而这样的系统是不可能以有效的经典方式进行模拟的，许多人相信 量子模拟将是量子计算最重要的应用之一。 量子模拟也可以用来模拟原子和粒子在非正常条件下的行为，比如对撞机内部的反应。

Quantum simulation 量子模拟

Since chemistry and nanotechnology rely on understanding quantum systems, and such systems are impossible to simulate in an efficient manner classically, many believe quantum simulation will be one of the most important applications of quantum computing.^[24] Quantum simulation could also be used to simulate the behavior of atoms and particles at unusual conditions such as the reactions inside a collider.^[25]

由于化学和纳米技术依赖于对量子系统的理解，而这种系统不可能以经典的方式进行有效的模拟，许多人相信量子模拟将是量子计算最重要的应用之一。量子模拟也可以用来模拟原子和粒子在异常条件下的行为，例如对撞机内部的反应。

Quantum annealing or Adiabatic quantum computation relies on the adiabatic theorem to undertake calculations. A system is placed in the ground state for a simple Hamiltonian, which is slowly evolved to a more complicated Hamiltonian whose ground state represents the solution to the problem in question. The adiabatic theorem states that if the evolution is slow enough the system will stay in its ground state at all times through the process.

量子退火或绝热量子计算依赖于绝热定理进行计算。对于一个简单的 哈密顿量，一个系统被放置在基态，这个 哈密顿量慢慢演化成一个更复杂的哈密顿量，它的基态代表问题的解。绝热定理指出，如果演化足够慢，系统在整个演化过程中将始终处于基态。

Quantum annealing and adiabatic optimization量子退火与绝热优化

Quantum annealing or Adiabatic quantum computation relies on the adiabatic theorem to undertake calculations. A system is placed in the ground state for a simple Hamiltonian, which is slowly evolved to a more complicated Hamiltonian whose ground state represents the solution to the problem in question. The adiabatic theorem states that if the evolution is slow enough the system will stay in its ground state at all times through the process.

量子退火或绝热量子计算依赖绝热定理进行计算。一个系统被置于基态，简单的哈密顿量慢慢演化成一个更复杂的哈密顿量，其基态代表问题的解决方案。绝热定理指出，如果进化足够慢，系统将在整个过程中始终保持在基态。

The Quantum algorithm for linear systems of equations, or "HHL Algorithm", named after its discoverers Harrow, Hassidim, and Lloyd, is expected to provide speedup over classical counterparts.

以其发现者 哈罗Harrow，哈西迪姆Hassidim和劳埃德Lloyd命名的线性方程组的量子算法，或称 “ HHL 算法”，预计将提供比经典算法更快的速度。

Solving linear equations 求解线性方程

The Quantum algorithm for linear systems of equations, or "HHL Algorithm", named after its discoverers Harrow, Hassidim, and Lloyd, is expected to provide speedup over classical counterparts.^[26]

以其发现者哈罗、哈西迪姆和劳埃德命名的用于线性方程组的量子算法或“HHL算法”，有望提供比经典算法更快的速度。

John Preskill has introduced the term quantum supremacy to refer to the hypothetical speedup advantage that a quantum computer would have over a classical computer in a certain field. Google announced in 2017 that it expected to achieve quantum supremacy by the end of the year though that did not happen. IBM said in 2018 that the best classical computers will be beaten on some practical task within about five years and views the quantum supremacy test only as a potential future benchmark. Although skeptics like Gil Kalai doubt that quantum supremacy will ever be achieved, in October 2019, a Sycamore processor created in conjunction with Google AI Quantum was reported to have achieved quantum supremacy, with calculations more than 3,000,000 times as fast as those of Summit, generally considered the world's fastest computer. Bill Unruh doubted the practicality of quantum computers in a paper published back in 1994. Paul Davies argued that a 400-qubit computer would even come into conflict with the cosmological information bound implied by the holographic principle.

约翰 · 普雷斯基尔提出了 量子优势Quantum supremacy这一术语，指的是量子计算机在某一领域相对于经典计算机的假设加速优势。谷歌在2017年宣布，它希望在今年年底前实现 量子优势，尽管这一目标没有实现。IBM 在2018年表示，最好的经典计算机将在大约5年内在某些实际任务上被击败，并将 量子优势测试视为未来的潜在基准。尽管像吉尔 · 卡莱这样的怀疑者对量子优势的实现持怀疑态度，但在2019年10月，据报道，与谷歌人工智能量子公司合作开发的 Sycamore 处理器已经取得了量子优势，其计算速度是顶峰计算机的300万倍以上，顶峰计算机被公认为世界上最快的计算机。比尔 · 安鲁在1994年发表的一篇论文中对量子计算机的实用性表示怀疑。认为一台400量子位的计算机甚至会与全息原理宇宙理论暗示的宇宙学信息发生冲突。

Quantum supremacy 量子至上

John Preskill has introduced the term quantum supremacy to refer to the hypothetical speedup advantage that a quantum computer would have over a classical computer in a certain field.^[27] Google announced in 2017 that it expected to achieve quantum supremacy by the end of the year though that did not happen. IBM said in 2018 that the best classical computers will be beaten on some practical task within about five years and views the quantum supremacy test only as a potential future benchmark.^[28] Although skeptics like Gil Kalai doubt that quantum supremacy will ever be achieved,^[29]^[30] in October 2019, a Sycamore processor created in conjunction with Google AI Quantum was reported to have achieved quantum supremacy,^[31] with calculations more than 3,000,000 times as fast as those of Summit, generally considered the world's fastest computer.^[32] Bill Unruh doubted the practicality of quantum computers in a paper published back in 1994.^[33] Paul Davies argued that a 400-qubit computer would even come into conflict with the cosmological information bound implied by the holographic principle.^[34]

John Preskill引入了“量子至上”一词来指量子计算机在某一领域相对于经典计算机所具有的假设加速优势。Google在2017年宣布，它预计将在今年年底实现量子霸权，但这并没有实现。IBM在2018年表示，最好的经典计算机将在大约五年内完成一些实际任务，并将量子优势测试视为未来潜在的基准。尽管像Gil Kalai这样的怀疑论者怀疑量子霸权是否会实现，据报道，2019年10月，与谷歌AI Quantum联合创建的Sycamore processor实现了量子优势，它的计算速度是世界上最快的计算机 Summit的300多万倍。Paul Davies认为，一台400 量子比特的计算机甚至会与[[全息原理]所隐含的宇宙信息界发生冲突。

There are a number of technical challenges in building a large-scale quantum computer. Physicist David DiVincenzo has listed the following requirements for a practical quantum computer:

建造大型量子计算机存在许多技术挑战。物理学家 David DiVincenzo 列出了实用量子计算机的下列要求:

Obstacles

There are a number of technical challenges in building a large-scale quantum computer.^[35] Physicist David DiVincenzo has listed the following requirements for a practical quantum computer:^[36]

Scalable physically to increase the number of qubits

Qubits that can be initialized to arbitrary values

Quantum gates that are faster than decoherence time

Universal gate set

Sourcing parts for quantum computers is also very difficult. Many quantum computers, like those constructed by Google and IBM, need Helium-3, a nuclear research byproduct, and special superconducting cables that are only made by the Japanese company Coax Co.

寻找量子计算机的零部件也非常困难。许多量子计算机，比如谷歌和 IBM 制造的计算机，需要核研究的副产品氦 -3，以及只有日本 Coax 公司制造的特殊超导电缆。

Qubits that can be read easily

The control of multi qubit systems requires the generation and coordination of a large number of electrical signals with tight and deterministic timing resolution. This had led to the development of quantum controllers which enable interfacing the qubit. Scaling these systems to support a growing number of qubits is an additional challenge in the scaling of quantum computers.

多量子比特系统的控制需要产生和协调大量具有紧密和确定性定时分辨率的电信号。这导致了量子控制器的发展，使接口的量子位。扩展这些系统以支持越来越多的量子比特是量子计算机扩展中的一个额外挑战。

Sourcing parts for quantum computers is also very difficult. Many quantum computers, like those constructed by Google and IBM, need Helium-3, a nuclear research byproduct, and special superconducting cables that are only made by the Japanese company Coax Co.^[37]

The control of multi qubit systems requires the generation and coordination of a large number of electrical signals with tight and deterministic timing resolution. This had led to the development of quantum controllers which enable interfacing the qubit. Scaling these systems to support a growing number of qubits is an additional challenge in the scaling of quantum computers.^{[citation needed]}

Quantum decoherence

One of the greatest challenges involved with constructing quantum computers is controlling or removing quantum decoherence. This usually means isolating the system from its environment as interactions with the external world cause the system to decohere. However, other sources of decoherence also exist. Examples include the quantum gates, and the lattice vibrations and background thermonuclear spin of the physical system used to implement the qubits. Decoherence is irreversible, as it is effectively non-unitary, and is usually something that should be highly controlled, if not avoided. Decoherence times for candidate systems in particular, the transverse relaxation time T₂ (for NMR and MRI technology, also called the dephasing time), typically range between nanoseconds and seconds at low temperature. Currently, some quantum computers require their qubits to be cooled to 20 millikelvins in order to prevent significant decoherence. A 2020 study argues that ionizing radiation such as cosmic rays can nevertheless cause certain systems to decohere within millisections.

构建量子计算机的最大挑战之一是控制或移除量子退相干。这通常意味着将系统与其环境隔离开来，因为与外部世界的相互作用会导致系统解码。然而，退相干的其他来源也存在。例子包括量子门、晶格振动和用于实现量子位的物理系统的背景热核自旋。退相干是不可逆的，因为它实际上是非幺正的，而且通常应该受到高度控制，如果不能避免的话。特别是候选系统的退相干时间，横向弛豫时间 t 2 (对于 NMR 和 MRI 技术，也称为退相时间) ，通常在低温下纳秒到秒之间。目前，一些量子计算机需要将它们的量子比特冷却到20毫升以防止明显的退相干。2020年的一项研究认为，像宇宙射线这样的电离辐射可以引起某些系统在毫秒内退相干。

As a result, time-consuming tasks may render some quantum algorithms inoperable, as maintaining the state of qubits for a long enough duration will eventually corrupt the superpositions.

因此，耗时的任务可能会使一些量子算法无法运行，因为维持量子位的状态足够长的时间最终会破坏这些叠加。

One of the greatest challenges involved with constructing quantum computers is controlling or removing quantum decoherence. This usually means isolating the system from its environment as interactions with the external world cause the system to decohere. However, other sources of decoherence also exist. Examples include the quantum gates, and the lattice vibrations and background thermonuclear spin of the physical system used to implement the qubits. Decoherence is irreversible, as it is effectively non-unitary, and is usually something that should be highly controlled, if not avoided. Decoherence times for candidate systems in particular, the transverse relaxation time T₂ (for NMR and MRI technology, also called the dephasing time), typically range between nanoseconds and seconds at low temperature.^[38] Currently, some quantum computers require their qubits to be cooled to 20 millikelvins in order to prevent significant decoherence.^[39] A 2020 study argues that ionizing radiation such as cosmic rays can nevertheless cause certain systems to decohere within millisections.^[40]

These issues are more difficult for optical approaches as the timescales are orders of magnitude shorter and an often-cited approach to overcoming them is optical pulse shaping. Error rates are typically proportional to the ratio of operating time to decoherence time, hence any operation must be completed much more quickly than the decoherence time.

这些问题对于光学方法来说更加困难，因为光学方法的时间数量级更短，而克服这些问题的常用方法是光脉冲整形。错误率通常与操作时间与去相干时间的比例成正比，因此任何操作必须比去相干时间更快地完成。

As a result, time-consuming tasks may render some quantum algorithms inoperable, as maintaining the state of qubits for a long enough duration will eventually corrupt the superpositions.^[41]

As described in the Quantum threshold theorem, if the error rate is small enough, it is thought to be possible to use quantum error correction to suppress errors and decoherence. This allows the total calculation time to be longer than the decoherence time if the error correction scheme can correct errors faster than decoherence introduces them. An often cited figure for the required error rate in each gate for fault-tolerant computation is 10⁻³, assuming the noise is depolarizing.

正如量子阈值定理所描述的那样，如果误差率足够小，人们认为可以利用量子误差修正来抑制误差和退相干。这使得总计算时间比消相干时间更长，如果纠错方案能够比消相干引入的误差更快地纠正误差。假设噪声是去极化的，容错计算中每个门所需的错误率经常被引用的数字是10 -3 。

These issues are more difficult for optical approaches as the timescales are orders of magnitude shorter and an often-cited approach to overcoming them is optical pulse shaping. Error rates are typically proportional to the ratio of operating time to decoherence time, hence any operation must be completed much more quickly than the decoherence time.

Meeting this scalability condition is possible for a wide range of systems. However, the use of error correction brings with it the cost of a greatly increased number of required qubits. The number required to factor integers using Shor's algorithm is still polynomial, and thought to be between L and L², where L is the number of qubits in the number to be factored; error correction algorithms would inflate this figure by an additional factor of L. For a 1000-bit number, this implies a need for about 10⁴ bits without error correction. With error correction, the figure would rise to about 10⁷ bits. Computation time is about L² or about 10⁷ steps and at 1 MHz, about 10 seconds.

对于很多系统来说，满足这种可伸缩性条件是可能的。然而，错误校正的使用带来了大大增加所需量子位数的成本。使用 Shor 算法对整数进行因子分解所需要的数字仍然是多项式的，并且被认为介于 l 和 l 2 之间，其中 l 是要分解的数字中的量子位数; 错误校正算法将使这个数字增加一个额外的因子 l，对于一个1000位的数字，这意味着需要大约10 < > 4 位而不进行错误校正。经过纠错，这个数字将上升到大约10 7 位。计算时间是大约 l 2 或大约10 7 步骤，在1mhz，大约10秒。

As described in the Quantum threshold theorem, if the error rate is small enough, it is thought to be possible to use quantum error correction to suppress errors and decoherence. This allows the total calculation time to be longer than the decoherence time if the error correction scheme can correct errors faster than decoherence introduces them. An often cited figure for the required error rate in each gate for fault-tolerant computation is 10⁻³, assuming the noise is depolarizing.

A very different approach to the stability-decoherence problem is to create a topological quantum computer with anyons, quasi-particles used as threads and relying on braid theory to form stable logic gates.

稳定性退相干问题的另一种不同的方法是用任意子、准粒子作为线程，依靠辫子理论形成稳定的逻辑门，创建一个拓扑量子计算机。

Meeting this scalability condition is possible for a wide range of systems. However, the use of error correction brings with it the cost of a greatly increased number of required qubits. The number required to factor integers using Shor's algorithm is still polynomial, and thought to be between L and L², where L is the number of qubits in the number to be factored; error correction algorithms would inflate this figure by an additional factor of L. For a 1000-bit number, this implies a need for about 10⁴ bits without error correction.^[42] With error correction, the figure would rise to about 10⁷ bits. Computation time is about L² or about 10⁷ steps and at 1 MHz, about 10 seconds.

Physicist Mikhail Dyakonov has expressed skepticism of quantum computing as follows:

物理学家米哈伊尔 · 迪亚科诺夫对量子计算表示怀疑，他说:

A very different approach to the stability-decoherence problem is to create a topological quantum computer with anyons, quasi-particles used as threads and relying on braid theory to form stable logic gates.^[43]^[44]

Physicist Mikhail Dyakonov has expressed skepticism of quantum computing as follows:

"So the number of continuous parameters describing the state of such a useful quantum computer at any given moment must be... about 10³⁰⁰... Could we ever learn to control the more than 10³⁰⁰ continuously variable parameters defining the quantum state of such a system? My answer is simple. No, never."^[45]^[46]

Developments

Quantum computing models

There are a number of quantum computing models, distinguished by the basic elements in which the computation is decomposed. The four main models of practical importance are:

Quantum gate array (computation decomposed into a sequence of few-qubit quantum gates)

One-way quantum computer (computation decomposed into a sequence of one-qubit measurements applied to a highly entangled initial state or cluster state)

Adiabatic quantum computer, based on quantum annealing (computation decomposed into a slow continuous transformation of an initial Hamiltonian into a final Hamiltonian, whose ground states contain the solution)^[47]

Topological quantum computer^[48] (computation decomposed into the braiding of anyons in a 2D lattice)

Conversely, any problem solvable by a quantum computer is also solvable by a classical computer; or more formally, any quantum computer can be simulated by a Turing machine. In other words, quantum computers provide no additional power over classical computers in terms of computability. This means that quantum computers cannot solve undecidable problems like the halting problem and the existence of quantum computers does not disprove the Church–Turing thesis.

相反，任何量子计算机可以解决的问题也可以用经典计算机来解决; 或者更正式地说，任何量子计算机都可以用图灵机来模拟。换句话说，就可计算性而言，量子计算机并不比传统计算机提供额外的能力。这意味着量子计算机不能解决不可判定的问题，例如停机问题和量子计算机的存在并不能否定丘奇-图灵论点。

The quantum Turing machine is theoretically important but the physical implementation of this model is not feasible. All four models of computation have been shown to be equivalent; each can simulate the other with no more than polynomial overhead.

As of yet, quantum computers do not satisfy the strong Church thesis. While hypothetical machines have been realized, a universal quantum computer has yet to be physically constructed. The strong version of Church's thesis requires a physical computer, and therefore there is no quantum computer that yet satisfies the strong Church thesis.

到目前为止，量子计算机还不能满足教会强有力的论点。虽然假想的机器已经被实现，但是通用的量子计算机还有待物理建造。切奇论点的强势版本需要一台物理计算机，因此没有量子计算机能够满足切奇论点的强势。

Physical realizations

For physically implementing a quantum computer, many different candidates are being pursued, among them (distinguished by the physical system used to realize the qubits):

Superconducting quantum computing^[49]^[50] (qubit implemented by the state of small superconducting circuits (Josephson junctions)

< ! -- 量子计算机的能力和极限 -- >

Trapped ion quantum computer (qubit implemented by the internal state of trapped ions)

While quantum computers cannot solve any problems that classical computers cannot already solve, it is suspected that they can solve many problems faster than classical computers. For instance, it is known that quantum computers can efficiently factor integers, while this is not believed to be the case for classical computers. However, the capacity of quantum computers to accelerate classical algorithms has rigid upper bounds, and the overwhelming majority of classical calculations cannot be accelerated by the use of quantum computers.

虽然量子计算机不能解决任何传统计算机已经不能解决的问题，人们怀疑它们能比传统计算机更快地解决许多问题。例如，众所周知量子计算机可以有效地对整数进行因子分解，而经典计算机则不然。然而，量子计算机加速经典算法的能力具有严格的上限，绝大多数经典计算不能被量子计算机加速。

Neutral atoms in Optical lattices (qubit implemented by internal states of neutral atoms trapped in an optical lattice)^[51]^[52]

Quantum dot computer, spin-based (e.g. the Loss-DiVincenzo quantum computer^[53]) (qubit given by the spin states of trapped electrons)

< ! -- bqp 的基本定义 -- >

Quantum dot computer, spatial-based (qubit given by electron position in double quantum dot)^[54]

The class of problems that can be efficiently solved by a quantum computer with bounded error is called BQP, for "bounded error, quantum, polynomial time". More formally, BQP is the class of problems that can be solved by a polynomial-time quantum Turing machine with error probability of at most 1/3. As a class of probabilistic problems, BQP is the quantum counterpart to BPP ("bounded error, probabilistic, polynomial time"), the class of problems that can be solved by polynomial-time probabilistic Turing machines with bounded error. It is known that BPP[math]\displaystyle{ \subseteq }[/math]BQP and is widely suspected that BQP[math]\displaystyle{ \nsubseteq }[/math]BPP, which intuitively would mean that quantum computers are more powerful than classical computers in terms of time complexity.

有界误差的量子计算机能够有效地求解的一类问题称为 BQP，即“有界误差，量子，多项式时间”问题。更正式地说，BQP 是一类问题，可以用多项式时间量子图灵机来解决，错误概率最多为1/3。作为一类概率问题，BQP 是 BPP (“有界误差，概率，多项式时间”)的量子对应物，BPP 是一类可用多项式时间概率图灵机解决的有界误差问题。众所周知，量子计算机比传统计算机在时间复杂度方面更强大，人们普遍怀疑量子计算机比传统计算机更强大。

Quantum computing using engineered quantum wells, which could in principle enable the construction of quantum computers that operate at room temperature^[55]^[56]

Coupled Quantum Wire (qubit implemented by a pair of Quantum Wires coupled by a Quantum Point Contact)^[57]^[58]^[59]

< ! -- bqp 与基本复杂类的关系 -- >

Nuclear magnetic resonance quantum computer (NMRQC) implemented with the nuclear magnetic resonance of molecules in solution, where qubits are provided by nuclear spins within the dissolved molecule and probed with radio waves

The suspected relationship of BQP to several classical complexity classes.

BQP 与几个经典复杂类的可疑关系。

Solid-state NMR Kane quantum computers (qubit realized by the nuclear spin state of phosphorus donors in silicon)

The exact relationship of BQP to P, NP, and PSPACE is not known. However, it is known that P[math]\displaystyle{ \subseteq }[/math]BQP[math]\displaystyle{ \subseteq }[/math]PSPACE; that is, all problems that can be efficiently solved by a deterministic classical computer can also be efficiently solved by a quantum computer, and all problems that can be efficiently solved by a quantum computer can also be solved by a deterministic classical computer with polynomial space resources. It is further suspected that BQP is a strict superset of P, meaning there are problems that are efficiently solvable by quantum computers that are not efficiently solvable by deterministic classical computers. For instance, integer factorization and the discrete logarithm problem are known to be in BQP and are suspected to be outside of P. On the relationship of BQP to NP, little is known beyond the fact that some NP problems that are believed not to be in P are also in BQP (integer factorization and the discrete logarithm problem are both in NP, for example). It is suspected that NP[math]\displaystyle{ \nsubseteq }[/math]BQP; that is, it is believed that there are efficiently checkable problems that are not efficiently solvable by a quantum computer. As a direct consequence of this belief, it is also suspected that BQP is disjoint from the class of NP-complete problems (if an NP-complete problem were in BQP, then it would follow from NP-hardness that all problems in NP are in BQP).

BQP 与 p、 NP 和 PSPACE 的确切关系尚不清楚。然而，众所周知，p < math > subseteq </math > BQP < math > subseteq </math > PSPACE; 也就是说，所有可以由确定性经典计算机有效地解决的问题也可以由量子计算机有效地解决，所有可以由量子计算机有效地解决的问题也可以由具有 PSPACE 资源的确定性经典计算机解决。进一步怀疑 BQP 是 p 的严格超集，这意味着有些问题可以通过量子计算机有效地解决，而这些问题不能通过确定性经典计算机有效地解决。例如，整数分解和离散对数问题已知在 BQP 中，并且被怀疑在 p 之外。关于 BQP 与 NP 的关系，除了一些被认为不在 p 中的 NP 问题也在 BQP 中之外，我们知之甚少(例如，整数分解和离散对数问题都在 NP 中)。人们怀疑 NP < math > nsubseteq </math > BQP; 也就是说，人们相信存在有效的检验问题，而量子计算机无法有效地解决这些问题。作为这一信念的直接结果，我们还怀疑 BQP 是与 NP 完全问题类不相交的(如果一个 NP 完全问题在 BQP 中，那么它可以从 NP 难度推出所有 NP 完全问题在 BQP 中)。

Electrons-on-helium quantum computers (qubit is the electron spin)

Cavity quantum electrodynamics (CQED) (qubit provided by the internal state of trapped atoms coupled to high-finesse cavities)

< ! -- 本质复杂性类的关系总结 -- >

Molecular magnet^[60] (qubit given by spin states)

The relationship of BQP to the basic classical complexity classes can be summarized as follows:

BQP 与基本经典复杂性类的关系可以概括如下:

Fullerene-based ESR quantum computer (qubit based on the electronic spin of atoms or molecules encased in fullerenes)^[61]

[math]\displaystyle{ \mathsf{P \subseteq BPP \subseteq BQP \subseteq PP \subseteq PSPACE} }[/math]

{ p subseteq BPP subseteq subseteq PP subseteq PSPACE } </math >

Nonlinear optical quantum computer (qubits realized by processing states of different modes of light through both linear and nonlinear elements)^[62]^[63]

It is also known that BQP is contained in the complexity class #P (or more precisely in the associated class of decision problems P^#P), Theories of quantum gravity, such as M-theory and loop quantum gravity, may allow even faster computers to be built. However, defining computation in these theories is an open problem due to the problem of time; that is, within these physical theories there is currently no obvious way to describe what it means for an observer to submit input to a computer at one point in time and then receive output at a later point in time.

众所周知，BQP 包含在复杂性等级 # p 中(或者更准确地说是相关类型的决策问题 p # p ) ，量子引力理论，如 m 理论和回圈量子重力理论，可能允许建立更快的计算机。然而，由于时间问题，在这些理论中定义计算是一个开放的问题; 也就是说，在这些物理理论中，目前没有明显的方法来描述观察者在一个时间点向计算机提交输入，然后在稍后的时间点接收输出意味着什么。

Linear optical quantum computer (qubits realized by processing states of different modes of light through linear elements e.g. mirrors, beam splitters and phase shifters)^[64]

Diamond-based quantum computer^[65]^[66]^[67] (qubit realized by the electronic or nuclear spin of nitrogen-vacancy centers in diamond)

Bose-Einstein condensate-based quantum computer^[68]

Transistor-based quantum computer – string quantum computers with entrainment of positive holes using an electrostatic trap

Rare-earth-metal-ion-doped inorganic crystal based quantum computers^[69]^[70] (qubit realized by the internal electronic state of dopants in optical fibers)

Metallic-like carbon nanospheres based quantum computers^[71]

A large number of candidates demonstrates that quantum computing, despite rapid progress, is still in its infancy.^{[citation needed]}

Relation to computability and complexity theory

Computability theory

Any computational problem solvable by a classical computer is also solvable by a quantum computer.^[72] Intuitively, this is because it is believed that all physical phenomena, including the operation of classical computers, can be described using quantum mechanics, which underlies the operation of quantum computers.

Category:Models of computation

类别: 计算模型

Category:Quantum cryptography

类别: 量子密码学

Conversely, any problem solvable by a quantum computer is also solvable by a classical computer; or more formally, any quantum computer can be simulated by a Turing machine. In other words, quantum computers provide no additional power over classical computers in terms of computability. This means that quantum computers cannot solve undecidable problems like the halting problem and the existence of quantum computers does not disprove the Church–Turing thesis.^[73]

Category:Information theory

范畴: 信息论

Category:Computational complexity theory

类别: 计算复杂性理论

As of yet, quantum computers do not satisfy the strong Church thesis. While hypothetical machines have been realized, a universal quantum computer has yet to be physically constructed. The strong version of Church's thesis requires a physical computer, and therefore there is no quantum computer that yet satisfies the strong Church thesis.

Category:Classes of computers

类别: 电脑类别

Category:Theoretical computer science

类别: 理论计算机科学

Quantum complexity theory

Category:Open problems

类别: 开放性问题

Category:Computer-related introductions in 1980

类别: 1980年与计算机有关的介绍

Category:Emerging technologies

类别: 新兴技术

This page was moved from wikipedia:en:Quantum computing. Its edit history can be viewed at 量子计算/edithistory

↑ ^1.0 ^1.1 The National Academies of Sciences, Engineering, and Medicine (2019). Quantum Computing : Progress and Prospects (2018). Washington, DC: National Academies Press. p. I-5. doi:10.17226/25196. ISBN 978-0-309-47969-1. OCLC 1081001288.
↑ Benioff, Paul (1980). "The computer as a physical system: A microscopic quantum mechanical Hamiltonian model of computers as represented by Turing machines". Journal of Statistical Physics. 22 (5): 563–591. Bibcode:1980JSP....22..563B. doi:10.1007/bf01011339. S2CID 122949592.
↑ Feynman, Richard (June 1982). "Simulating Physics with Computers" (PDF). International Journal of Theoretical Physics. 21 (6/7): 467–488. Bibcode:1982IJTP...21..467F. doi:10.1007/BF02650179. S2CID 124545445. Archived from the original (PDF) on 8 January 2019. Retrieved 28 February 2019.
↑ Manin, Yu. I. (1980) (in Russian). Vychislimoe i nevychislimoe. Sov.Radio. pp. 13–15. Archived from the original on 2013-05-10. https://web.archive.org/web/20130510173823/http://publ.lib.ru/ARCHIVES/M/MANIN_Yuriy_Ivanovich/Manin_Yu.I._Vychislimoe_i_nevychislimoe.(1980).%5Bdjv%5D.zip. Retrieved 2013-03-04.
↑ 模板:Cite document
↑ John Preskill (2018). "Quantum Computing in the NISQ era and beyond". Quantum. 2: 79. arXiv:1801.00862. doi:10.22331/q-2018-08-06-79. S2CID 44098998.
↑ Gibney, Elizabeth (2 October 2019). "Quantum gold rush: the private funding pouring into quantum start-ups". Nature. 574 (7776): 22–24. Bibcode:2019Natur.574...22G. doi:10.1038/d41586-019-02935-4. PMID 31578480.
↑ Rodrigo, Chris Mills (12 February 2020). "Trump budget proposal boosts funding for artificial intelligence, quantum computing". The Hill.{{cite news}}: CS1 maint: url-status (link)
↑ "On "Quantum Supremacy"". IBM Research Blog (in English). 2019-10-22. Retrieved 2020-01-21.
↑ Franklin, Diana; Chong, Frederic T. (2004). "Challenges in Reliable Quantum Computing". Nano, Quantum and Molecular Computing. pp. 247–266. doi:10.1007/1-4020-8068-9_8. ISBN 1-4020-8067-0.
↑ Pakkin, Scott; Coles, Patrick (10 June 2019). "The Problem with Quantum Computers". Scientific American.
↑ Nielsen, Michael A.; Chuang, Isaac L. (2010). Quantum Computation and Quantum Information: 10th Anniversary Edition. Cambridge: Cambridge University Press. doi:10.1017/cbo9780511976667. ISBN 9780511976667. http://cds.cern.ch/record/465953.
↑ Lenstra, Arjen K. (2000). "Integer Factoring" (PDF). Designs, Codes and Cryptography. 19 (2/3): 101–128. doi:10.1023/A:1008397921377. S2CID 9816153. Archived from the original (PDF) on 2015-04-10.
↑ ^14.0 ^14.1 Bernstein, Daniel J. (2009). "Introduction to post-quantum cryptography". Post-Quantum Cryptography. 549. pp. 1–14. doi:10.1007/978-3-540-88702-7_1. ISBN 978-3-540-88701-0. PMID 28905891.
↑ See also pqcrypto.org, a bibliography maintained by Daniel J. Bernstein and Tanja Lange on cryptography not known to be broken by quantum computing.
↑ McEliece, R. J. (January 1978). "A Public-Key Cryptosystem Based On Algebraic Coding Theory" (PDF). DSNPR. 44: 114–116. Bibcode:1978DSNPR..44..114M.
↑ Kobayashi, H.; Gall, F.L. (2006). "Dihedral Hidden Subgroup Problem: A Survey". Information and Media Technologies. 1 (1): 178–185. doi:10.2197/ipsjdc.1.470.
↑ Bennett, Charles H.; Bernstein, Ethan; Brassard, Gilles; Vazirani, Umesh (October 1997). "Strengths and Weaknesses of Quantum Computing". SIAM Journal on Computing. 26 (5): 1510–1523. arXiv:quant-ph/9701001. Bibcode:1997quant.ph..1001B. doi:10.1137/s0097539796300933. S2CID 13403194.
↑ Katwala, Amit (5 March 2020). "Quantum computers will change the world (if they work)". Wired UK.
↑ Quantum Algorithm Zoo -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期2018-04-29. – Stephen Jordan's Homepage
↑ Schiller, Jon (2009-06-19 Problems that can be addressed with Grover's algorithm have the following properties: 可以通过 Grover 的算法解决的问题有以下属性:). [https://books.google.com/books?id=l217ma2sWkoC&pg=PA11 Besides factorization and discrete logarithms, quantum algorithms offering a more than polynomial speedup over the best known classical algorithm have been found for several problems, including the simulation of quantum physical processes from chemistry and solid state physics, the approximation of Jones polynomials, and solving Pell's equation. No mathematical proof has been found that shows that an equally fast classical algorithm cannot be discovered, although this is considered unlikely. However, quantum computers offer polynomial speedup for some problems. The most well-known example of this is quantum database search, which can be solved by Grover's algorithm using quadratically fewer queries to the database than that are required by classical algorithms. In this case, the advantage is not only provable but also optimal, it has been shown that Grover's algorithm gives the maximal possible probability of finding the desired element for any number of oracle lookups. Several other examples of provable quantum speedups for query problems have subsequently been discovered, such as for finding collisions in two-to-one functions and evaluating NAND trees. 除了因式分解和离散对数外，量子算法比最著名的经典算法提供了超过多项式的加速比，在一些问题上被发现，包括从化学和固态物理模拟量子物理过程， 琼斯多项式Jones polynomials的近似，和解 佩尔方程Pell's equation。目前还没有发现一个同样快速的经典算法无法被发现的数学证明，尽管这被认为是不可能的。然而，量子计算机为某些问题提供了多项式加速。最著名的例子是量子数据库搜索，它可以通过格罗夫Grover算法来解决，使用比经典算法所需的查询更少的数据库查询。在这种情况下，这种优势不仅是可证明的，而且是最优的，已经证明Grover的算法为任何数量的oracle查找提供了找到所需元素的最大可能概率。随后又发现了其他几个可证明的量子加速的例子，例如在两对一函数中寻找碰撞和评估NAND树。 Quantum Computers]. ISBN 9781439243497. https://books.google.com/books?id=l217ma2sWkoC&pg=PA11 Besides factorization and discrete logarithms, quantum algorithms offering a more than polynomial speedup over the best known classical algorithm have been found for several problems, including the simulation of quantum physical processes from chemistry and solid state physics, the approximation of Jones polynomials, and solving Pell's equation. No mathematical proof has been found that shows that an equally fast classical algorithm cannot be discovered, although this is considered unlikely. However, quantum computers offer polynomial speedup for some problems. The most well-known example of this is quantum database search, which can be solved by Grover's algorithm using quadratically fewer queries to the database than that are required by classical algorithms. In this case, the advantage is not only provable but also optimal, it has been shown that Grover's algorithm gives the maximal possible probability of finding the desired element for any number of oracle lookups. Several other examples of provable quantum speedups for query problems have subsequently been discovered, such as for finding collisions in two-to-one functions and evaluating NAND trees. 除了因式分解和离散对数外，量子算法比最著名的经典算法提供了超过多项式的加速比，在一些问题上被发现，包括从化学和固态物理模拟量子物理过程， 琼斯多项式Jones polynomials的近似，和解 佩尔方程Pell's equation。目前还没有发现一个同样快速的经典算法无法被发现的数学证明，尽管这被认为是不可能的。然而，量子计算机为某些问题提供了多项式加速。最著名的例子是量子数据库搜索，它可以通过格罗夫Grover算法来解决，使用比经典算法所需的查询更少的数据库查询。在这种情况下，这种优势不仅是可证明的，而且是最优的，已经证明Grover的算法为任何数量的oracle查找提供了找到所需元素的最大可能概率。随后又发现了其他几个可证明的量子加速的例子，例如在两对一函数中寻找碰撞和评估NAND树。. 模板:Self-published inline
↑ Ambainis, Ambainis (June 2004). "Quantum search algorithms". ACM SIGACT News. 35 (2): 22–35. arXiv:quant-ph/0504012. Bibcode:2005quant.ph..4012A. doi:10.1145/992287.992296. S2CID 11326499.
↑ Rich, Steven; Gellman, Barton (2014-02-01). "NSA seeks to build quantum computer that could crack most types of encryption". Washington Post.
↑ Norton, Quinn (2007-02-15). "The Father of Quantum Computing". Wired.
↑ Ambainis, Andris (Spring 2014). "What Can We Do with a Quantum Computer?". Institute for Advanced Study.
↑ Harrow, Aram; Hassidim, Avinatan; Lloyd, Seth (2009). "Quantum algorithm for solving linear systems of equations". Physical Review Letters. 103 (15): 150502. arXiv:0811.3171. Bibcode:2009PhRvL.103o0502H. doi:10.1103/PhysRevLett.103.150502. PMID 19905613. S2CID 5187993.
↑ Boixo, Sergio; Isakov, Sergei V.; Smelyanskiy, Vadim N.; Babbush, Ryan; Ding, Nan; Jiang, Zhang; Bremner, Michael J.; Martinis, John M.; Neven, Hartmut (2018). "Characterizing Quantum Supremacy in Near-Term Devices". Nature Physics. 14 (6): 595–600. arXiv:1608.00263. Bibcode:2018NatPh..14..595B. doi:10.1038/s41567-018-0124-x. S2CID 4167494.
↑ Savage, Neil. "Quantum Computers Compete for "Supremacy"".
↑ "Quantum Supremacy and Complexity". 23 April 2016.
↑ Kalai, Gil. "The Quantum Computer Puzzle" (PDF). AMS.
↑ Arute, Frank; Arya, Kunal; Babbush, Ryan; Bacon, Dave; Bardin, Joseph C.; Barends, Rami; Biswas, Rupak; Boixo, Sergio; Brandao, Fernando G. S. L.; Buell, David A.; Burkett, Brian; Chen, Yu; Chen, Zijun; Chiaro, Ben; Collins, Roberto; Courtney, William; Dunsworsth, Andrew; Farhi, Edward; Foxen, Brooks; Fowler, Austin; Gidney, Craig; Giustina, Marissa; Graff, Rob; Guerin, Keith; Habegger, Steve; Harrigan, Matthew P.; Hartmann, Michael J.; Ho, Alan; Hoffman, Markus; Huang, Trent; Humble, Travis S.; Isakov, Sergei V.; Jeffery, Evan; Jiang, Zhang; Kafri, Dvir; Kechedzhi, Kostyantyn; Kelly, Julian; Klimov, Paul V.; Knysh, Sergey; Korotov, Alexander; Kostritsa, Fedor; Landhuis, David; Lindmark, Mike; Lucero, Erik; Lyakh, Dmitry; Mandrà, Salvatore; McClean, Jarrod R.; McEwen, Matthew; Megrant, Anthony; Mi, Xiao; Michielsen, Kristel; Mohseni, Masoud; Mutus, Josh; Naaman, Ofer; Neeley, Matthew; Neill, Charles; Niu, Murphy Yuezhen; Ostby, Eric; Petukhov, Andre; Platt, John C.; Quintana, Chris; Rieffel, Eleanor G.; Roushan, Pedram; Rubin, Nicholas C.; Sank, Daniel; Satzinger, Kevin J.; Smelyanskiy, Vadim; Sung, Kevin J.; Trevithick, Matthew D.; Vainsencher, Amit; Villalonga, Benjamin; White, Theodore; Yao, Z. Jamie; Yeh, Ping; Zalcman, Adam; Neven, Hartmut; Martinis, John M. (23 October 2019). "Quantum supremacy using a programmable superconducting processor". Nature. 574 (7779): 505–510. arXiv:1910.11333. Bibcode:2019Natur.574..505A. doi:10.1038/s41586-019-1666-5. PMID 31645734. S2CID 204836822.
↑ "Google researchers have reportedly achieved "quantum supremacy"". MIT Technology Review.
↑ Unruh, Bill (1995). "Maintaining coherence in Quantum Computers". Physical Review A. 51 (2): 992–997. arXiv:hep-th/9406058. Bibcode:1995PhRvA..51..992U. doi:10.1103/PhysRevA.51.992. PMID 9911677. S2CID 13980886.
↑ Davies, Paul. "The implications of a holographic universe for quantum information science and the nature of physical law" (PDF). Macquarie University.
↑ Dyakonov, Mikhail (2018-11-15). "The Case Against Quantum Computing". IEEE Spectrum.
↑ DiVincenzo, David P. (2000-04-13). "The Physical Implementation of Quantum Computation". Fortschritte der Physik. 48 (9–11): 771–783. arXiv:quant-ph/0002077. Bibcode:2000ForPh..48..771D. doi:10.1002/1521-3978(200009)48:9/11<771::AID-PROP771>3.0.CO;2-E.
↑ Giles, Martin (17 January 2019). "We'd have more quantum computers if it weren't so hard to find the damn cables". MIT Technology Review.
↑ DiVincenzo, David P. (1995). "Quantum Computation". Science. 270 (5234): 255–261. Bibcode:1995Sci...270..255D. CiteSeerX 10.1.1.242.2165. doi:10.1126/science.270.5234.255. S2CID 220110562. (subscription required)
↑ Jones, Nicola (19 June 2013). "Computing: The quantum company". Nature. 498 (7454): 286–288. Bibcode:2013Natur.498..286J. doi:10.1038/498286a. PMID 23783610.
↑ Vepsäläinen, Antti P.; Karamlou, Amir H.; Orrell, John L.; Dogra, Akshunna S.; Loer, Ben; et al. (August 2020). "Impact of ionizing radiation on superconducting qubit coherence". Nature (in English). 584 (7822): 551–556. arXiv:2001.09190. doi:10.1038/s41586-020-2619-8. ISSN 1476-4687. PMID 32848227. S2CID 210920566.
↑ Amy, Matthew; Matteo, Olivia; Gheorghiu, Vlad; Mosca, Michele; Parent, Alex; Schanck, John (November 30, 2016). "Estimating the cost of generic quantum pre-image attacks on SHA-2 and SHA-3". arXiv:1603.09383 [quant-ph].
↑ Dyakonov, M. I. (2006-10-14). S. Luryi; J. Xu; A. Zaslavsky (eds.). "Is Fault-Tolerant Quantum Computation Really Possible?". Future Trends in Microelectronics. Up the Nano Creek: 4–18. arXiv:quant-ph/0610117. Bibcode:2006quant.ph.10117D.
↑ {{cite journal "So the number of continuous parameters describing the state of such a useful quantum computer at any given moment must be... about 10³⁰⁰... Could we ever learn to control the more than 10³⁰⁰ continuously variable parameters defining the quantum state of such a system? My answer is simple. No, never." “因此，描述这样一个有用的量子计算机在任何给定时刻的状态的连续参数的数量必须是... ... 大约10 300 ... ... 我们能否学会控制定义这样一个系统的量子态的超过10 300 连续可变参数？我的答案很简单。不，永远不会。” | last1 = Freedman | first1 = Michael H. | author1-link = Michael Freedman | last2 = Kitaev | first2 = Alexei | author2-link = Alexei Kitaev | last3 = Larsen | first3 = Michael J. | author3-link = Michael J. Larsen | last4 = Wang | first4 = Zhenghan There are a number of quantum computing models, distinguished by the basic elements in which the computation is decomposed. The four main models of practical importance are: 有许多量子计算模型，以计算被分解的基本元素来区分。具有实际重要性的四个主要模式是: | arxiv = quant-ph/0101025 | doi = 10.1090/S0273-0979-02-00964-3 | issue = 1 | journal = Bulletin of the American Mathematical Society | mr = 1943131 The quantum Turing machine is theoretically important but the physical implementation of this model is not feasible. All four models of computation have been shown to be equivalent; each can simulate the other with no more than polynomial overhead. 量子图灵机在理论上是重要的，但是这个模型的实际实施是不可行的。所有四种计算模型都被证明是等价的; 每种模型只需要不超过多项式的开销就可以模拟另一种模型。 | pages = 31–38 | title = Topological quantum computation | volume = 40 For physically implementing a quantum computer, many different candidates are being pursued, among them (distinguished by the physical system used to realize the qubits): 为了实现量子计算机的物理实现，许多不同的候选者正在被追求，其中包括(区别于用于实现量子比特的物理系统) : | year = 2003}}
↑ Monroe, Don (2008-10-01). "Anyons: The breakthrough quantum computing needs?". New Scientist.
↑ Dyakonov, Mikhail. "The Case Against Quantum Computing". IEEE Spectrum. Retrieved 3 December 2019.
↑ Dyakonov, Mikhail (24 March 2020). Will We Ever Have a Quantum Computer?. Springer. ISBN 9783030420185. https://www.springer.com/gp/book/9783030420185. Retrieved 22 May 2020. 模板:Page needed
↑ Das, A.; Chakrabarti, B. K. (2008). "Quantum Annealing and Analog Quantum Computation". Rev. Mod. Phys. 80 (3): 1061–1081. arXiv:0801.2193. Bibcode:2008RvMP...80.1061D. CiteSeerX 10.1.1.563.9990. doi:10.1103/RevModPhys.80.1061. S2CID 14255125.
↑ Nayak, Chetan; Simon, Steven; Stern, Ady A large number of candidates demonstrates that quantum computing, despite rapid progress, is still in its infancy. 大量的候选者表明，量子计算，尽管进展迅速，仍然处于初级阶段。; Das Sarma, Sankar (2008). "Nonabelian Anyons and Quantum Computation". Reviews of Modern Physics. 80 (3): 1083–1159. arXiv:0707.1889. Bibcode:[https://ui.adsabs.harvard.edu/abs/2008RvMP...80.1083N Any computational problem solvable by a classical computer is also solvable by a quantum computer. Intuitively, this is because it is believed that all physical phenomena, including the operation of classical computers, can be described using quantum mechanics, which underlies the operation of quantum computers. 任何经典计算机可解的计算问题也可以用量子计算机来解。直观地说，这是因为人们相信所有的物理现象，包括经典计算机的运算，都可以用量子计算机运算的基础---- 量子力学来描述。 2008RvMP...80.1083N Any computational problem solvable by a classical computer is also solvable by a quantum computer. Intuitively, this is because it is believed that all physical phenomena, including the operation of classical computers, can be described using quantum mechanics, which underlies the operation of quantum computers. 任何经典计算机可解的计算问题也可以用量子计算机来解。直观地说，这是因为人们相信所有的物理现象，包括经典计算机的运算，都可以用量子计算机运算的基础---- 量子力学来描述。]. doi:10.1103/RevModPhys.80.1083. S2CID 119628297. {{cite journal}}: Check |bibcode= length (help); line feed character in |bibcode= at position 20 (help); line feed character in |first3= at position 4 (help)
↑ Clarke, John; Wilhelm, Frank K. (18 June 2008). "Superconducting quantum bits". Nature. 453 (7198): 1031–1042. Bibcode:2008Natur.453.1031C. doi:10.1038/nature07128. PMID 18563154. S2CID 125213662.
↑ Kaminsky, William M.; Lloyd, Seth; Orlando, Terry P. (12 March 2004). "Scalable Superconducting Architecture for Adiabatic Quantum Computation". arXiv:quant-ph/0403090. Bibcode:2004quant.ph..3090K. {{cite journal}}: Cite journal requires |journal= (help)
↑ Khazali, Mohammadsadegh; Mølmer, Klaus (2020-06-11). "Fast Multiqubit Gates by Adiabatic Evolution in Interacting Excited-State Manifolds of Rydberg Atoms and Superconducting Circuits". Physical Review X. 10 (2): 021054. Bibcode:2020PhRvX..10b1054K. doi:10.1103/PhysRevX.10.021054.
↑ Henriet, Loic; Beguin, Lucas; Signoles, Adrien; Lahaye, Thierry; Browaeys, Antoine; Reymond, Georges-Olivier; Jurczak, Christophe (2020-06-22). "Quantum computing with neutral atoms". Quantum. 4: 327. arXiv:2006.12326. doi:10.22331/q-2020-09-21-327. S2CID 219966169.
↑ Imamog¯lu, A.; Awschalom, D. D.; Burkard, G.; DiVincenzo, D. P.; Loss, D.; Sherwin, M.; Small, A. (15 November 1999). "Quantum Information Processing Using Quantum Dot Spins and Cavity QED". Physical Review Letters. 83 (20): 4204–4207. arXiv:quant-ph/9904096. Bibcode:1999PhRvL..83.4204I. doi:10.1103/PhysRevLett.83.4204. S2CID 18324734.
↑ Fedichkin, L.; Yanchenko, M.; Valiev, K. A. (June 2000). "Novel coherent quantum bit using spatial quantization levels in semiconductor quantum dot". Quantum Computers and Computing. 1: 58. arXiv:quant-ph/0006097. Bibcode:2000quant.ph..6097F.
↑ Ivády, Viktor; Davidsson, Joel; Delegan, Nazar; Falk, Abram L.; Klimov, Paul V.; Whiteley, Samuel J.; Hruszkewycz, Stephan O.; Holt, Martin V.; Heremans, F. Joseph; Son, Nguyen Tien; Awschalom, David D.; Abrikosov, Igor A.; Gali, Adam (6 December 2019). "Stabilization of point-defect spin qubits by quantum wells". Nature Communications. 10 (1): 5607. arXiv:1905.11801. Bibcode:2019NatCo..10.5607I. doi:10.1038/s41467-019-13495-6. PMC 6898666. PMID 31811137.
↑ "Scientists Discover New Way to Get Quantum Computing to Work at Room Temperature". interestingengineering.com. 24 April 2020.
↑ Bertoni, A.; Bordone, P.; Brunetti, R.; Jacoboni, C.; Reggiani, S. (19 June 2000). "Quantum Logic Gates based on Coherent Electron Transport in Quantum Wires". Physical Review Letters. 84 (25): 5912–5915. Bibcode:2000PhRvL..84.5912B. doi:10.1103/PhysRevLett.84.5912. hdl:11380/303796. PMID 10991086.
↑ Ionicioiu, Radu; Amaratunga, Gehan; Udrea, Florin (20 January 2001). "Quantum Computation with Ballistic Electrons". International Journal of Modern Physics B. 15 (2): 125–133. arXiv:quant-ph/0011051. Bibcode:2001IJMPB..15..125I. CiteSeerX 10.1.1.251.9617. doi:10.1142/S0217979201003521. S2CID 119389613.
↑ Ramamoorthy, A; Bird, J P; Reno, J L (11 July 2007). "Using split-gate structures to explore the implementation of a coupled-electron-waveguide qubit scheme". Journal of Physics: Condensed Matter. 19 (27): 276205. Bibcode:2007JPCM...19A6205R. doi:10.1088/0953-8984/19/27/276205.
↑ Leuenberger, Michael N.; Loss, Daniel (April 2001). "Quantum computing in molecular magnets". Nature. 410 (6830): 789–793. arXiv:cond-mat/0011415. Bibcode:2001Natur.410..789L. doi:10.1038/35071024. PMID 11298441. S2CID 4373008.
↑ Harneit, Wolfgang (27 February 2002). "Fullerene-based electron-spin quantum computer". Physical Review A. 65 (3): 032322. Bibcode:2002PhRvA..65c2322H. doi:10.1103/PhysRevA.65.032322.https://www.researchgate.net/publication/257976907_Fullerene-based_electron-spin_quantum_computer
↑ K. Igeta and Y. Yamamoto. "Quantum mechanical computers with single atom and photon fields." International Quantum Electronics Conference (1988) https://www.osapublishing.org/abstract.cfm?uri=IQEC-1988-TuI4
↑ I.L. Chuang and Y. Yamamoto. "Simple quantum computer." Physical Review A 52, 5, 3489 (1995) https://doi.org/10.1103/PhysRevA.52.3489
↑ Knill, G. J.; Laflamme, R.; Milburn, G. J. (2001). "A scheme for efficient quantum computation with linear optics". Nature. 409 (6816): 46–52. Bibcode:2001Natur.409...46K. doi:10.1038/35051009. PMID 11343107. S2CID 4362012.
↑ Optics and Spectroscopy < ! -- 新链接请按字母顺序排列 -- >. August 2005
{ columns-list
↑ Dutt, M. V. G.; Childress, L.; Jiang, L.; Togan, E.; Maze, J.; Jelezko, F.; Zibrov, A. S.; Hemmer, P. R.; Lukin, M. D. (1 June 2007). "Quantum Register Based on Individual Electronic and Nuclear Spin Qubits in Diamond". Science. 316 (5829): 1312–1316. Bibcode:2007Sci...316.....D. doi:10.1126/science.1139831. PMID 17540898. S2CID 20697722. Lay summary. {{cite journal}}: Cite uses deprecated parameter |lay-url= (help)
↑ Neumann, P.; et al. (June 6, 2008). "Multipartite Entanglement Among Single Spins in Diamond". Science. 320 (5881): 1326–1329. Bibcode:2008Sci...320.1326N. doi:10.1126/science.1157233. PMID 18535240.. {{cite journal}}: Check date values in: |date= (help); External link in |date= (help); Missing or empty |title= (help); line feed character in |date= at position 12 (help); line feed character in |journal= at position 24 (help) |first7 = V. |last8 = Gaebel |first8 = T. |last9 = Jelezko |first9 = F. |s2cid = 8892596 }}
↑ Anderlini, Marco; Lee, Patricia J.; Brown, Benjamin L.; Sebby-Strabley, Jennifer; Phillips, William D.; Porto, J. V. (July 2007). "Controlled exchange interaction between pairs of neutral atoms in an optical lattice". Nature. 448 (7152): 452–456. arXiv:0708.2073. Bibcode:2007Natur.448..452A. doi:10.1038/nature06011. PMID 17653187. S2CID 4410355. Lay summary. {{cite journal}}: Cite uses deprecated parameter |lay-url= (help)
↑ Ohlsson, N.; Mohan, R. K.; Kröll, S. (January 1, 2002). "Quantum computer hardware based on rare-earth-ion-doped inorganic crystals". Opt. Commun. 201 (1–3): 71–77. Bibcode:2002OptCo.201...71O. doi:10.1016/S0030-4018(01)01666-2.
↑ Longdell, J. J.; Sellars, M. J.; Manson, N. B. Lectures 讲座 (September 23, 2004). "Demonstration of conditional quantum phase shift between ions in a solid". Phys. Rev. Lett. 93 (13): 130503. arXiv:quant-ph/0404083. Bibcode:2004PhRvL..93m0503L. doi:10.1103/PhysRevLett.93.130503. PMID 15524694. S2CID 41374015. {{cite journal}}: line feed character in |first3= at position 6 (help)
↑ Náfrádi, Bálint; Choucair, Mohammad; Dinse, Klaus-Peter; Forró, László (18 July 2016). "Room temperature manipulation of long lifetime spins in metallic-like carbon nanospheres". Nature Communications. 7 (1): 12232. arXiv:1611.07690. Bibcode:2016NatCo...712232N. doi:10.1038/ncomms12232. PMC 4960311. PMID 27426851.
↑ Nielsen, p. 29
↑ Nielsen, p. 126

[2018Report-1] 1.0 ^1.1 The National Academies of Sciences, Engineering, and Medicine (2019). Quantum Computing : Progress and Prospects (2018). Washington, DC: National Academies Press. p. I-5. doi:10.17226/25196. ISBN 978-0-309-47969-1. OCLC 1081001288.

[The_computer_as_a_physical_system-2] Benioff, Paul (1980). "The computer as a physical system: A microscopic quantum mechanical Hamiltonian model of computers as represented by Turing machines". Journal of Statistical Physics. 22 (5): 563–591. Bibcode:1980JSP....22..563B. doi:10.1007/bf01011339. S2CID 122949592.

[3] Feynman, Richard (June 1982). "Simulating Physics with Computers" (PDF). International Journal of Theoretical Physics. 21 (6/7): 467–488. Bibcode:1982IJTP...21..467F. doi:10.1007/BF02650179. S2CID 124545445. Archived from the original (PDF) on 8 January 2019. Retrieved 28 February 2019.

[manin1980vychislimoe-4] Manin, Yu. I. (1980) (in Russian). Vychislimoe i nevychislimoe. Sov.Radio. pp. 13–15. Archived from the original on 2013-05-10. https://web.archive.org/web/20130510173823/http://publ.lib.ru/ARCHIVES/M/MANIN_Yuriy_Ivanovich/Manin_Yu.I._Vychislimoe_i_nevychislimoe.(1980).%5Bdjv%5D.zip. Retrieved 2013-03-04.

[5] 模板:Cite document

[preskill2018-6] John Preskill (2018). "Quantum Computing in the NISQ era and beyond". Quantum. 2: 79. arXiv:1801.00862. doi:10.22331/q-2018-08-06-79. S2CID 44098998.

[7] Gibney, Elizabeth (2 October 2019). "Quantum gold rush: the private funding pouring into quantum start-ups". Nature. 574 (7776): 22–24. Bibcode:2019Natur.574...22G. doi:10.1038/d41586-019-02935-4. PMID 31578480.

[8] Rodrigo, Chris Mills (12 February 2020). "Trump budget proposal boosts funding for artificial intelligence, quantum computing". The Hill.{{cite news}}: CS1 maint: url-status (link)

[9] "On "Quantum Supremacy"". IBM Research Blog (in English). 2019-10-22. Retrieved 2020-01-21.

[10] Franklin, Diana; Chong, Frederic T. (2004). "Challenges in Reliable Quantum Computing". Nano, Quantum and Molecular Computing. pp. 247–266. doi:10.1007/1-4020-8068-9_8. ISBN 1-4020-8067-0.

[11] Pakkin, Scott; Coles, Patrick (10 June 2019). "The Problem with Quantum Computers". Scientific American.

[Nielson-Chuang-12] Nielsen, Michael A.; Chuang, Isaac L. (2010). Quantum Computation and Quantum Information: 10th Anniversary Edition. Cambridge: Cambridge University Press. doi:10.1017/cbo9780511976667. ISBN 9780511976667. http://cds.cern.ch/record/465953.

[13] Lenstra, Arjen K. (2000). "Integer Factoring" (PDF). Designs, Codes and Cryptography. 19 (2/3): 101–128. doi:10.1023/A:1008397921377. S2CID 9816153. Archived from the original (PDF) on 2015-04-10.

[pqcrypto_survey-14] 14.0 ^14.1 Bernstein, Daniel J. (2009). "Introduction to post-quantum cryptography". Post-Quantum Cryptography. 549. pp. 1–14. doi:10.1007/978-3-540-88702-7_1. ISBN 978-3-540-88701-0. PMID 28905891.

[15] See also pqcrypto.org, a bibliography maintained by Daniel J. Bernstein and Tanja Lange on cryptography not known to be broken by quantum computing.

[16] McEliece, R. J. (January 1978). "A Public-Key Cryptosystem Based On Algebraic Coding Theory" (PDF). DSNPR. 44: 114–116. Bibcode:1978DSNPR..44..114M.

[17] Kobayashi, H.; Gall, F.L. (2006). "Dihedral Hidden Subgroup Problem: A Survey". Information and Media Technologies. 1 (1): 178–185. doi:10.2197/ipsjdc.1.470.

[bennett_1997-18] Bennett, Charles H.; Bernstein, Ethan; Brassard, Gilles; Vazirani, Umesh (October 1997). "Strengths and Weaknesses of Quantum Computing". SIAM Journal on Computing. 26 (5): 1510–1523. arXiv:quant-ph/9701001. Bibcode:1997quant.ph..1001B. doi:10.1137/s0097539796300933. S2CID 13403194.

[19] Katwala, Amit (5 March 2020). "Quantum computers will change the world (if they work)". Wired UK.

[20] Quantum Algorithm Zoo -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期2018-04-29. – Stephen Jordan's Homepage

[21] Schiller, Jon (2009-06-19 Problems that can be addressed with Grover's algorithm have the following properties: 可以通过 Grover 的算法解决的问题有以下属性:). [https://books.google.com/books?id=l217ma2sWkoC&pg=PA11 Besides factorization and discrete logarithms, quantum algorithms offering a more than polynomial speedup over the best known classical algorithm have been found for several problems, including the simulation of quantum physical processes from chemistry and solid state physics, the approximation of Jones polynomials, and solving Pell's equation. No mathematical proof has been found that shows that an equally fast classical algorithm cannot be discovered, although this is considered unlikely. However, quantum computers offer polynomial speedup for some problems. The most well-known example of this is quantum database search, which can be solved by Grover's algorithm using quadratically fewer queries to the database than that are required by classical algorithms. In this case, the advantage is not only provable but also optimal, it has been shown that Grover's algorithm gives the maximal possible probability of finding the desired element for any number of oracle lookups. Several other examples of provable quantum speedups for query problems have subsequently been discovered, such as for finding collisions in two-to-one functions and evaluating NAND trees. 除了因式分解和离散对数外，量子算法比最著名的经典算法提供了超过多项式的加速比，在一些问题上被发现，包括从化学和固态物理模拟量子物理过程， 琼斯多项式Jones polynomials的近似，和解 佩尔方程Pell's equation。目前还没有发现一个同样快速的经典算法无法被发现的数学证明，尽管这被认为是不可能的。然而，量子计算机为某些问题提供了多项式加速。最著名的例子是量子数据库搜索，它可以通过格罗夫Grover算法来解决，使用比经典算法所需的查询更少的数据库查询。在这种情况下，这种优势不仅是可证明的，而且是最优的，已经证明Grover的算法为任何数量的oracle查找提供了找到所需元素的最大可能概率。随后又发现了其他几个可证明的量子加速的例子，例如在两对一函数中寻找碰撞和评估NAND树。 Quantum Computers]. ISBN 9781439243497. https://books.google.com/books?id=l217ma2sWkoC&pg=PA11 Besides factorization and discrete logarithms, quantum algorithms offering a more than polynomial speedup over the best known classical algorithm have been found for several problems, including the simulation of quantum physical processes from chemistry and solid state physics, the approximation of Jones polynomials, and solving Pell's equation. No mathematical proof has been found that shows that an equally fast classical algorithm cannot be discovered, although this is considered unlikely. However, quantum computers offer polynomial speedup for some problems. The most well-known example of this is quantum database search, which can be solved by Grover's algorithm using quadratically fewer queries to the database than that are required by classical algorithms. In this case, the advantage is not only provable but also optimal, it has been shown that Grover's algorithm gives the maximal possible probability of finding the desired element for any number of oracle lookups. Several other examples of provable quantum speedups for query problems have subsequently been discovered, such as for finding collisions in two-to-one functions and evaluating NAND trees. 除了因式分解和离散对数外，量子算法比最著名的经典算法提供了超过多项式的加速比，在一些问题上被发现，包括从化学和固态物理模拟量子物理过程， 琼斯多项式Jones polynomials的近似，和解 佩尔方程Pell's equation。目前还没有发现一个同样快速的经典算法无法被发现的数学证明，尽管这被认为是不可能的。然而，量子计算机为某些问题提供了多项式加速。最著名的例子是量子数据库搜索，它可以通过格罗夫Grover算法来解决，使用比经典算法所需的查询更少的数据库查询。在这种情况下，这种优势不仅是可证明的，而且是最优的，已经证明Grover的算法为任何数量的oracle查找提供了找到所需元素的最大可能概率。随后又发现了其他几个可证明的量子加速的例子，例如在两对一函数中寻找碰撞和评估NAND树。. 模板:Self-published inline

[22] Ambainis, Ambainis (June 2004). "Quantum search algorithms". ACM SIGACT News. 35 (2): 22–35. arXiv:quant-ph/0504012. Bibcode:2005quant.ph..4012A. doi:10.1145/992287.992296. S2CID 11326499.

[23] Rich, Steven; Gellman, Barton (2014-02-01). "NSA seeks to build quantum computer that could crack most types of encryption". Washington Post.

[24] Norton, Quinn (2007-02-15). "The Father of Quantum Computing". Wired.

[25] Ambainis, Andris (Spring 2014). "What Can We Do with a Quantum Computer?". Institute for Advanced Study.

[Quantum_algorithm_for_solving_linear_systems_of_equations_by_Harrow_et_al.-26] Harrow, Aram; Hassidim, Avinatan; Lloyd, Seth (2009). "Quantum algorithm for solving linear systems of equations". Physical Review Letters. 103 (15): 150502. arXiv:0811.3171. Bibcode:2009PhRvL.103o0502H. doi:10.1103/PhysRevLett.103.150502. PMID 19905613. S2CID 5187993.

[27] Boixo, Sergio; Isakov, Sergei V.; Smelyanskiy, Vadim N.; Babbush, Ryan; Ding, Nan; Jiang, Zhang; Bremner, Michael J.; Martinis, John M.; Neven, Hartmut (2018). "Characterizing Quantum Supremacy in Near-Term Devices". Nature Physics. 14 (6): 595–600. arXiv:1608.00263. Bibcode:2018NatPh..14..595B. doi:10.1038/s41567-018-0124-x. S2CID 4167494.

[28] Savage, Neil. "Quantum Computers Compete for "Supremacy"".

[29] "Quantum Supremacy and Complexity". 23 April 2016.

[30] Kalai, Gil. "The Quantum Computer Puzzle" (PDF). AMS.

[31] Arute, Frank; Arya, Kunal; Babbush, Ryan; Bacon, Dave; Bardin, Joseph C.; Barends, Rami; Biswas, Rupak; Boixo, Sergio; Brandao, Fernando G. S. L.; Buell, David A.; Burkett, Brian; Chen, Yu; Chen, Zijun; Chiaro, Ben; Collins, Roberto; Courtney, William; Dunsworsth, Andrew; Farhi, Edward; Foxen, Brooks; Fowler, Austin; Gidney, Craig; Giustina, Marissa; Graff, Rob; Guerin, Keith; Habegger, Steve; Harrigan, Matthew P.; Hartmann, Michael J.; Ho, Alan; Hoffman, Markus; Huang, Trent; Humble, Travis S.; Isakov, Sergei V.; Jeffery, Evan; Jiang, Zhang; Kafri, Dvir; Kechedzhi, Kostyantyn; Kelly, Julian; Klimov, Paul V.; Knysh, Sergey; Korotov, Alexander; Kostritsa, Fedor; Landhuis, David; Lindmark, Mike; Lucero, Erik; Lyakh, Dmitry; Mandrà, Salvatore; McClean, Jarrod R.; McEwen, Matthew; Megrant, Anthony; Mi, Xiao; Michielsen, Kristel; Mohseni, Masoud; Mutus, Josh; Naaman, Ofer; Neeley, Matthew; Neill, Charles; Niu, Murphy Yuezhen; Ostby, Eric; Petukhov, Andre; Platt, John C.; Quintana, Chris; Rieffel, Eleanor G.; Roushan, Pedram; Rubin, Nicholas C.; Sank, Daniel; Satzinger, Kevin J.; Smelyanskiy, Vadim; Sung, Kevin J.; Trevithick, Matthew D.; Vainsencher, Amit; Villalonga, Benjamin; White, Theodore; Yao, Z. Jamie; Yeh, Ping; Zalcman, Adam; Neven, Hartmut; Martinis, John M. (23 October 2019). "Quantum supremacy using a programmable superconducting processor". Nature. 574 (7779): 505–510. arXiv:1910.11333. Bibcode:2019Natur.574..505A. doi:10.1038/s41586-019-1666-5. PMID 31645734. S2CID 204836822.

[32] "Google researchers have reportedly achieved "quantum supremacy"". MIT Technology Review.

[33] Unruh, Bill (1995). "Maintaining coherence in Quantum Computers". Physical Review A. 51 (2): 992–997. arXiv:hep-th/9406058. Bibcode:1995PhRvA..51..992U. doi:10.1103/PhysRevA.51.992. PMID 9911677. S2CID 13980886.

[34] Davies, Paul. "The implications of a holographic universe for quantum information science and the nature of physical law" (PDF). Macquarie University.

[35] Dyakonov, Mikhail (2018-11-15). "The Case Against Quantum Computing". IEEE Spectrum.

[36] DiVincenzo, David P. (2000-04-13). "The Physical Implementation of Quantum Computation". Fortschritte der Physik. 48 (9–11): 771–783. arXiv:quant-ph/0002077. Bibcode:2000ForPh..48..771D. doi:10.1002/1521-3978(200009)48:9/11<771::AID-PROP771>3.0.CO;2-E.

[37] Giles, Martin (17 January 2019). "We'd have more quantum computers if it weren't so hard to find the damn cables". MIT Technology Review.

[DiVincenzo_1995-38] DiVincenzo, David P. (1995). "Quantum Computation". Science. 270 (5234): 255–261. Bibcode:1995Sci...270..255D. CiteSeerX 10.1.1.242.2165. doi:10.1126/science.270.5234.255. S2CID 220110562. (subscription required)

[39] Jones, Nicola (19 June 2013). "Computing: The quantum company". Nature. 498 (7454): 286–288. Bibcode:2013Natur.498..286J. doi:10.1038/498286a. PMID 23783610.

[40] Vepsäläinen, Antti P.; Karamlou, Amir H.; Orrell, John L.; Dogra, Akshunna S.; Loer, Ben; et al. (August 2020). "Impact of ionizing radiation on superconducting qubit coherence". Nature (in English). 584 (7822): 551–556. arXiv:2001.09190. doi:10.1038/s41586-020-2619-8. ISSN 1476-4687. PMID 32848227. S2CID 210920566.

[41] Amy, Matthew; Matteo, Olivia; Gheorghiu, Vlad; Mosca, Michele; Parent, Alex; Schanck, John (November 30, 2016). "Estimating the cost of generic quantum pre-image attacks on SHA-2 and SHA-3". arXiv:1603.09383 [quant-ph].

[42] Dyakonov, M. I. (2006-10-14). S. Luryi; J. Xu; A. Zaslavsky (eds.). "Is Fault-Tolerant Quantum Computation Really Possible?". Future Trends in Microelectronics. Up the Nano Creek: 4–18. arXiv:quant-ph/0610117. Bibcode:2006quant.ph.10117D.

[43] {{cite journal "So the number of continuous parameters describing the state of such a useful quantum computer at any given moment must be... about 10³⁰⁰... Could we ever learn to control the more than 10³⁰⁰ continuously variable parameters defining the quantum state of such a system? My answer is simple. No, never." “因此，描述这样一个有用的量子计算机在任何给定时刻的状态的连续参数的数量必须是... ... 大约10 < sup > 300 ... ... 我们能否学会控制定义这样一个系统的量子态的超过10 < sup > 300 连续可变参数？我的答案很简单。不，永远不会。” | last1 = Freedman | first1 = Michael H. | author1-link = Michael Freedman | last2 = Kitaev | first2 = Alexei | author2-link = Alexei Kitaev | last3 = Larsen | first3 = Michael J. | author3-link = Michael J. Larsen | last4 = Wang | first4 = Zhenghan There are a number of quantum computing models, distinguished by the basic elements in which the computation is decomposed. The four main models of practical importance are: 有许多量子计算模型，以计算被分解的基本元素来区分。具有实际重要性的四个主要模式是: | arxiv = quant-ph/0101025 | doi = 10.1090/S0273-0979-02-00964-3 | issue = 1 | journal = Bulletin of the American Mathematical Society | mr = 1943131 The quantum Turing machine is theoretically important but the physical implementation of this model is not feasible. All four models of computation have been shown to be equivalent; each can simulate the other with no more than polynomial overhead. 量子图灵机在理论上是重要的，但是这个模型的实际实施是不可行的。所有四种计算模型都被证明是等价的; 每种模型只需要不超过多项式的开销就可以模拟另一种模型。 | pages = 31–38 | title = Topological quantum computation | volume = 40 For physically implementing a quantum computer, many different candidates are being pursued, among them (distinguished by the physical system used to realize the qubits): 为了实现量子计算机的物理实现，许多不同的候选者正在被追求，其中包括(区别于用于实现量子比特的物理系统) : | year = 2003}}

[44] Monroe, Don (2008-10-01). "Anyons: The breakthrough quantum computing needs?". New Scientist.

[45] Dyakonov, Mikhail. "The Case Against Quantum Computing". IEEE Spectrum. Retrieved 3 December 2019.

[46] Dyakonov, Mikhail (24 March 2020). Will We Ever Have a Quantum Computer?. Springer. ISBN 9783030420185. https://www.springer.com/gp/book/9783030420185. Retrieved 22 May 2020. 模板:Page needed

[Das_2008_1061.E2.80.931081-47] Das, A.; Chakrabarti, B. K. (2008). "Quantum Annealing and Analog Quantum Computation". Rev. Mod. Phys. 80 (3): 1061–1081. arXiv:0801.2193. Bibcode:2008RvMP...80.1061D. CiteSeerX 10.1.1.563.9990. doi:10.1103/RevModPhys.80.1061. S2CID 14255125.

[Nayaketal2008-48] Nayak, Chetan; Simon, Steven; Stern, Ady A large number of candidates demonstrates that quantum computing, despite rapid progress, is still in its infancy. 大量的候选者表明，量子计算，尽管进展迅速，仍然处于初级阶段。; Das Sarma, Sankar (2008). "Nonabelian Anyons and Quantum Computation". Reviews of Modern Physics. 80 (3): 1083–1159. arXiv:0707.1889. Bibcode:[https://ui.adsabs.harvard.edu/abs/2008RvMP...80.1083N Any computational problem solvable by a classical computer is also solvable by a quantum computer. Intuitively, this is because it is believed that all physical phenomena, including the operation of classical computers, can be described using quantum mechanics, which underlies the operation of quantum computers. 任何经典计算机可解的计算问题也可以用量子计算机来解。直观地说，这是因为人们相信所有的物理现象，包括经典计算机的运算，都可以用量子计算机运算的基础---- 量子力学来描述。 2008RvMP...80.1083N Any computational problem solvable by a classical computer is also solvable by a quantum computer. Intuitively, this is because it is believed that all physical phenomena, including the operation of classical computers, can be described using quantum mechanics, which underlies the operation of quantum computers. 任何经典计算机可解的计算问题也可以用量子计算机来解。直观地说，这是因为人们相信所有的物理现象，包括经典计算机的运算，都可以用量子计算机运算的基础---- 量子力学来描述。]. doi:10.1103/RevModPhys.80.1083. S2CID 119628297. {{cite journal}}: Check |bibcode= length (help); line feed character in |bibcode= at position 20 (help); line feed character in |first3= at position 4 (help)

[ClarkeWilhelm2008-49] Clarke, John; Wilhelm, Frank K. (18 June 2008). "Superconducting quantum bits". Nature. 453 (7198): 1031–1042. Bibcode:2008Natur.453.1031C. doi:10.1038/nature07128. PMID 18563154. S2CID 125213662.

[50] Kaminsky, William M.; Lloyd, Seth; Orlando, Terry P. (12 March 2004). "Scalable Superconducting Architecture for Adiabatic Quantum Computation". arXiv:quant-ph/0403090. Bibcode:2004quant.ph..3090K. {{cite journal}}: Cite journal requires |journal= (help)

[51] Khazali, Mohammadsadegh; Mølmer, Klaus (2020-06-11). "Fast Multiqubit Gates by Adiabatic Evolution in Interacting Excited-State Manifolds of Rydberg Atoms and Superconducting Circuits". Physical Review X. 10 (2): 021054. Bibcode:2020PhRvX..10b1054K. doi:10.1103/PhysRevX.10.021054.

[52] Henriet, Loic; Beguin, Lucas; Signoles, Adrien; Lahaye, Thierry; Browaeys, Antoine; Reymond, Georges-Olivier; Jurczak, Christophe (2020-06-22). "Quantum computing with neutral atoms". Quantum. 4: 327. arXiv:2006.12326. doi:10.22331/q-2020-09-21-327. S2CID 219966169.

[53] Imamog¯lu, A.; Awschalom, D. D.; Burkard, G.; DiVincenzo, D. P.; Loss, D.; Sherwin, M.; Small, A. (15 November 1999). "Quantum Information Processing Using Quantum Dot Spins and Cavity QED". Physical Review Letters. 83 (20): 4204–4207. arXiv:quant-ph/9904096. Bibcode:1999PhRvL..83.4204I. doi:10.1103/PhysRevLett.83.4204. S2CID 18324734.

[54] Fedichkin, L.; Yanchenko, M.; Valiev, K. A. (June 2000). "Novel coherent quantum bit using spatial quantization levels in semiconductor quantum dot". Quantum Computers and Computing. 1: 58. arXiv:quant-ph/0006097. Bibcode:2000quant.ph..6097F.

[55] Ivády, Viktor; Davidsson, Joel; Delegan, Nazar; Falk, Abram L.; Klimov, Paul V.; Whiteley, Samuel J.; Hruszkewycz, Stephan O.; Holt, Martin V.; Heremans, F. Joseph; Son, Nguyen Tien; Awschalom, David D.; Abrikosov, Igor A.; Gali, Adam (6 December 2019). "Stabilization of point-defect spin qubits by quantum wells". Nature Communications. 10 (1): 5607. arXiv:1905.11801. Bibcode:2019NatCo..10.5607I. doi:10.1038/s41467-019-13495-6. PMC 6898666. PMID 31811137.

[56] "Scientists Discover New Way to Get Quantum Computing to Work at Room Temperature". interestingengineering.com. 24 April 2020.

[57] Bertoni, A.; Bordone, P.; Brunetti, R.; Jacoboni, C.; Reggiani, S. (19 June 2000). "Quantum Logic Gates based on Coherent Electron Transport in Quantum Wires". Physical Review Letters. 84 (25): 5912–5915. Bibcode:2000PhRvL..84.5912B. doi:10.1103/PhysRevLett.84.5912. hdl:11380/303796. PMID 10991086.

[58] Ionicioiu, Radu; Amaratunga, Gehan; Udrea, Florin (20 January 2001). "Quantum Computation with Ballistic Electrons". International Journal of Modern Physics B. 15 (2): 125–133. arXiv:quant-ph/0011051. Bibcode:2001IJMPB..15..125I. CiteSeerX 10.1.1.251.9617. doi:10.1142/S0217979201003521. S2CID 119389613.

[59] Ramamoorthy, A; Bird, J P; Reno, J L (11 July 2007). "Using split-gate structures to explore the implementation of a coupled-electron-waveguide qubit scheme". Journal of Physics: Condensed Matter. 19 (27): 276205. Bibcode:2007JPCM...19A6205R. doi:10.1088/0953-8984/19/27/276205.

[60] Leuenberger, Michael N.; Loss, Daniel (April 2001). "Quantum computing in molecular magnets". Nature. 410 (6830): 789–793. arXiv:cond-mat/0011415. Bibcode:2001Natur.410..789L. doi:10.1038/35071024. PMID 11298441. S2CID 4373008.

[61] Harneit, Wolfgang (27 February 2002). "Fullerene-based electron-spin quantum computer". Physical Review A. 65 (3): 032322. Bibcode:2002PhRvA..65c2322H. doi:10.1103/PhysRevA.65.032322.https://www.researchgate.net/publication/257976907_Fullerene-based_electron-spin_quantum_computer

[qc1988-62] K. Igeta and Y. Yamamoto. "Quantum mechanical computers with single atom and photon fields." International Quantum Electronics Conference (1988) https://www.osapublishing.org/abstract.cfm?uri=IQEC-1988-TuI4

[chuang1995-63] I.L. Chuang and Y. Yamamoto. "Simple quantum computer." Physical Review A 52, 5, 3489 (1995) https://doi.org/10.1103/PhysRevA.52.3489

[KLM2001-64] Knill, G. J.; Laflamme, R.; Milburn, G. J. (2001). "A scheme for efficient quantum computation with linear optics". Nature. 409 (6816): 46–52. Bibcode:2001Natur.409...46K. doi:10.1038/35051009. PMID 11343107. S2CID 4362012.

[Nizovtsevetal2004-65] Optics and Spectroscopy < ! -- 新链接请按字母顺序排列 -- >. August 2005
{ columns-list

[66] Dutt, M. V. G.; Childress, L.; Jiang, L.; Togan, E.; Maze, J.; Jelezko, F.; Zibrov, A. S.; Hemmer, P. R.; Lukin, M. D. (1 June 2007). "Quantum Register Based on Individual Electronic and Nuclear Spin Qubits in Diamond". Science. 316 (5829): 1312–1316. Bibcode:2007Sci...316.....D. doi:10.1126/science.1139831. PMID 17540898. S2CID 20697722. Lay summary. {{cite journal}}: Cite uses deprecated parameter |lay-url= (help)

[Neumannetal2008-67] Neumann, P.; et al. (June 6, 2008). "Multipartite Entanglement Among Single Spins in Diamond". Science. 320 (5881): 1326–1329. Bibcode:2008Sci...320.1326N. doi:10.1126/science.1157233. PMID 18535240.. {{cite journal}}: Check date values in: |date= (help); External link in |date= (help); Missing or empty |title= (help); line feed character in |date= at position 12 (help); line feed character in |journal= at position 24 (help) |first7 = V. |last8 = Gaebel |first8 = T. |last9 = Jelezko |first9 = F. |s2cid = 8892596 }}

[68] Anderlini, Marco; Lee, Patricia J.; Brown, Benjamin L.; Sebby-Strabley, Jennifer; Phillips, William D.; Porto, J. V. (July 2007). "Controlled exchange interaction between pairs of neutral atoms in an optical lattice". Nature. 448 (7152): 452–456. arXiv:0708.2073. Bibcode:2007Natur.448..452A. doi:10.1038/nature06011. PMID 17653187. S2CID 4410355. Lay summary. {{cite journal}}: Cite uses deprecated parameter |lay-url= (help)

[Ohlsson2002-69] Ohlsson, N.; Mohan, R. K.; Kröll, S. (January 1, 2002). "Quantum computer hardware based on rare-earth-ion-doped inorganic crystals". Opt. Commun. 201 (1–3): 71–77. Bibcode:2002OptCo.201...71O. doi:10.1016/S0030-4018(01)01666-2.

[Longdell2004-70] Longdell, J. J.; Sellars, M. J.; Manson, N. B. Lectures 讲座 (September 23, 2004). "Demonstration of conditional quantum phase shift between ions in a solid". Phys. Rev. Lett. 93 (13): 130503. arXiv:quant-ph/0404083. Bibcode:2004PhRvL..93m0503L. doi:10.1103/PhysRevLett.93.130503. PMID 15524694. S2CID 41374015. {{cite journal}}: line feed character in |first3= at position 6 (help)

[Nafradi2016-71] Náfrádi, Bálint; Choucair, Mohammad; Dinse, Klaus-Peter; Forró, László (18 July 2016). "Room temperature manipulation of long lifetime spins in metallic-like carbon nanospheres". Nature Communications. 7 (1): 12232. arXiv:1611.07690. Bibcode:2016NatCo...712232N. doi:10.1038/ncomms12232. PMC 4960311. PMID 27426851.

[72] Nielsen, p. 29

[73] Nielsen, p. 126

[1]

[2]

[3]

[4]

[5]

[6]

[7]

[8]

[9]

[10]

[11]

[12]

[13]

[14]

[15]

[16]

[17]

[18]

[19]

[20]

[21]

[22]

[23]

[24]

[25]

[26]

[27]

[28]

[29]

[30]

[31]

[32]

[33]

[34]

[35]

[36]

[37]

[38]

[39]

[40]

[41]

[42]

[43]

[44]

[45]

[46]

[47]

[48]

[49]

[50]

[51]

[52]

[53]

[54]

[55]

[56]

[57]

[58]

[59]

[60]

[61]

[62]

[63]

[64]

[65]

[66]

[67]

[68]

[69]

[70]

[71]

[72]

[73]

@@ 第458行： / 第458行： @@
 约翰 · 普雷斯基尔提出了'''<font color="#ff8000"> 量子优势Quantum supremacy</font>'''这一术语，指的是量子计算机在某一领域相对于经典计算机的假设加速优势。谷歌在2017年宣布，它希望在今年年底前实现'''<font color="#ff8000"> 量子优势</font>'''，尽管这一目标没有实现。IBM 在2018年表示，最好的经典计算机将在大约5年内在某些实际任务上被击败，并将'''<font color="#ff8000"> 量子优势</font>'''测试视为未来的潜在基准。尽管像吉尔 · 卡莱这样的怀疑者对量子优势的实现持怀疑态度，但在2019年10月，据报道，与谷歌人工智能量子公司合作开发的 Sycamore 处理器已经取得了量子优势，其计算速度是顶峰计算机的300万倍以上，顶峰计算机被公认为世界上最快的计算机。比尔 · 安鲁在1994年发表的一篇论文中对量子计算机的实用性表示怀疑。认为一台400量子位的计算机甚至会与全息原理宇宙理论暗示的宇宙学信息发生冲突。
-=== Quantum supremacy ===
+=== Quantum supremacy 量子至上===
 {{Main|Quantum supremacy}}
 [[John Preskill]] has introduced the term ''[[quantum supremacy]]'' to refer to the hypothetical speedup advantage that a quantum computer would have over a classical computer in a certain field.<ref>{{Cite journal|title=Characterizing Quantum Supremacy in Near-Term Devices|journal=Nature Physics|volume=14|issue=6|pages=595–600|first1=Sergio|last1=Boixo|first2=Sergei V.|last2=Isakov|first3=Vadim N.|last3=Smelyanskiy|first4=Ryan|last4=Babbush|first5=Nan|last5=Ding|first6=Zhang|last6=Jiang|first7=Michael J.|last7=Bremner|first8=John M.|last8=Martinis|first9=Hartmut|last9=Neven|year=2018|arxiv=1608.00263|doi=10.1038/s41567-018-0124-x|bibcode=2018NatPh..14..595B|s2cid=4167494}}</ref> [[Google]] announced in 2017 that it expected to achieve quantum supremacy by the end of the year though that did not happen. [[IBM]] said in 2018 that the best classical computers will be beaten on some practical task within about five years and views the quantum supremacy test only as a potential future benchmark.<ref>{{cite web|url=https://www.scientificamerican.com/article/quantum-computers-compete-for-supremacy/|title=Quantum Computers Compete for "Supremacy"|first=Neil|last=Savage}}</ref> Although skeptics like [[Gil Kalai]] doubt that quantum supremacy will ever be achieved,<ref>{{cite web|url=https://rjlipton.wordpress.com/2016/04/22/quantum-supremacy-and-complexity/|title=Quantum Supremacy and Complexity|date=23 April 2016}}</ref><ref>{{cite web|last1=Kalai|first1=Gil|title=The Quantum Computer Puzzle|url=http://www.ams.org/journals/notices/201605/rnoti-p508.pdf|publisher=AMS}}</ref> in October 2019, a [[Sycamore processor]] created in conjunction with Google AI Quantum was reported to have achieved quantum supremacy,<ref>{{cite journal|last1=Arute|first1=Frank|last2=Arya|first2=Kunal|last3=Babbush|first3=Ryan|last4=Bacon|first4=Dave|last5=Bardin|first5=Joseph C.|last6=Barends|first6=Rami|last7=Biswas|first7=Rupak|last8=Boixo|first8=Sergio|last9=Brandao|first9=Fernando G. S. L.|last10=Buell|first10=David A.|last11=Burkett|first11=Brian|date=23 October 2019|title=Quantum supremacy using a programmable superconducting processor|journal=Nature|volume=574|issue=7779|first15=Roberto|first57=Murphy Yuezhen|last64=Rubin|first63=Pedram|last63=Roushan|first62=Eleanor G.|last62=Rieffel|first61=Chris|last61=Quintana|first60=John C.|last60=Platt|first59=Andre|last59=Petukhov|first58=Eric|last58=Ostby|last57=Niu|last65=Sank|first56=Charles|last56=Neill|first55=Matthew|last55=Neeley|first54=Ofer|last54=Naaman|first53=Josh|last53=Mutus|first52=Masoud|last52=Mohseni|first51=Kristel|last51=Michielsen|first50=Xiao|last50=Mi|first64=Nicholas C.|first65=Daniel|last49=Megrant|last74=Yeh|last12=Chen|first12=Yu|last13=Chen|first13=Zijun|last14=Chiaro|first14=Ben|first77=John M.|last77=Martinis|first76=Hartmut|last76=Neven|first75=Adam|last75=Zalcman|first74=Ping|first73=Z. Jamie|last66=Satzinger|last73=Yao|first72=Theodore|last72=White|first71=Benjamin|last71=Villalonga|first70=Amit|last70=Vainsencher|first69=Matthew D.|last69=Trevithick|first68=Kevin J.|last68=Sung|first67=Vadim|last67=Smelyanskiy|first66=Kevin J.|first49=Anthony|first48=Matthew|last16=Courtney|last24=Guerin|first30=Trent|last30=Huang|first29=Markus|last29=Hoffman|first28=Alan|last28=Ho|first27=Michael J.|last27=Hartmann|first26=Matthew P.|last26=Harrigan|first25=Steve|last25=Habegger|first24=Keith|first23=Rob|first31=Travis S.|last23=Graff|first22=Marissa|last22=Giustina|first21=Craig|last21=Gidney|first20=Austin|last20=Fowler|first19=Brooks|last19=Foxen|first18=Edward|last18=Farhi|first17=Andrew|last17=Dunsworsth|first16=William|last31=Humble|last32=Isakov|last48=McEwen|first40=Alexander|first47=Jarrod R.|last47=McClean|first46=Salvatore|last46=Mandrà|first45=Dmitry|last45=Lyakh|first44=Erik|last44=Lucero|first43=Mike|last43=Lindmark|first42=David|last42=Landhuis|first41=Fedor|last15=Collins|last40=Korotov|first32=Sergei V.|first39=Sergey|last39=Knysh|first38=Paul V.|last38=Klimov|first37=Julian|last37=Kelly|first36=Kostyantyn|last36=Kechedzhi|first35=Dvir|last35=Kafri|first34=Zhang|last34=Jiang|first33=Evan|last33=Jeffery|last41=Kostritsa|doi=10.1038/s41586-019-1666-5|pmid=31645734|pages=505–510|bibcode=2019Natur.574..505A|arxiv=1910.11333|s2cid=204836822}}</ref> with calculations more than 3,000,000 times as fast as those of [[Summit (supercomputer)|Summit]], generally considered the world's fastest computer.<ref>{{Cite web|url=https://www.technologyreview.com/f/614416/google-researchers-have-reportedly-achieved-quantum-supremacy/|title=Google researchers have reportedly achieved "quantum supremacy"|website=MIT Technology Review}}</ref> [[Bill Unruh]] doubted the practicality of quantum computers in a paper published back in 1994.<ref>{{Cite journal|last1=Unruh|first1=Bill|title=Maintaining coherence in Quantum Computers|journal=Physical Review A|volume=51|issue=2|pages=992–997|arxiv=hep-th/9406058|bibcode=1995PhRvA..51..992U|year=1995|doi=10.1103/PhysRevA.51.992|pmid=9911677|s2cid=13980886}}</ref> [[Paul Davies]] argued that a 400-qubit computer would even come into conflict with the cosmological information bound implied by the [[holographic principle]].<ref>{{cite web|last1=Davies|first1=Paul|title=The implications of a holographic universe for quantum information science and the nature of physical law|url=http://power.itp.ac.cn/~mli/pdavies.pdf|publisher=Macquarie University}}</ref>
+[[John Preskill]]引入了“[[量子至上]]”一词来指量子计算机在某一领域相对于经典计算机所具有的假设加速优势。[[Google]]在2017年宣布，它预计将在今年年底实现量子霸权，但这并没有实现。[[IBM]]在2018年表示，最好的经典计算机将在大约五年内完成一些实际任务，并将量子优势测试视为未来潜在的基准。尽管像[[Gil Kalai]]这样的怀疑论者怀疑量子霸权是否会实现，据报道，2019年10月，与谷歌AI Quantum联合创建的[[Sycamore processor]]实现了量子优势，它的计算速度是世界上最快的计算机[[Summit（supercomputer）| Summit]]的300多万倍。[[Paul Davies]]认为，一台400 量子比特的计算机甚至会与[[全息原理]所隐含的宇宙信息界发生冲突。
 There are a number of technical challenges in building a large-scale quantum computer. Physicist David DiVincenzo has listed the following requirements for a practical quantum computer:

“量子计算”的版本间的差异

2020年11月5日 (四) 21:02的版本

目录

Quantum operations量子计算

Potential applications 潜在应用

Cryptography 密码学

Quantum search 量子搜索

Quantum simulation 量子模拟

Quantum annealing and adiabatic optimization量子退火与绝热优化

Solving linear equations 求解线性方程

Quantum supremacy 量子至上

Obstacles

Quantum decoherence

Developments

Quantum computing models

Physical realizations

Relation to computability and complexity theory

Computability theory

Quantum complexity theory

导航菜单

搜索