“路径 path”的版本间的差异

来自集智百科 - 复杂系统|人工智能|复杂科学|复杂网络|自组织
跳到导航 跳到搜索
第23行： 第23行：
 
Let  be a graph. A finite walk is a sequence of edges  for which there is a sequence of vertices  such that ϕ(e<sub>i</sub>) = {v<sub>i</sub>, v<sub>i + 1</sub>} for .  is the vertex sequence of the walk. This walk is  closed if v<sub>1</sub> = v<sub>n</sub>, and open else. An infinite walk is a sequence of edges of the same type described here, but with no first or last vertex, and a semi-infinite walk (or ray) has a first vertex but no last vertex.
 
Let  be a graph. A finite walk is a sequence of edges  for which there is a sequence of vertices  such that ϕ(e<sub>i</sub>) = {v<sub>i</sub>, v<sub>i + 1</sub>} for .  is the vertex sequence of the walk. This walk is  closed if v<sub>1</sub> = v<sub>n</sub>, and open else. An infinite walk is a sequence of edges of the same type described here, but with no first or last vertex, and a semi-infinite walk (or ray) has a first vertex but no last vertex.
  
−
以一个图为例{{nowrap|1=''G'' = (''V'', ''E'', ''ϕ'')}} 。'''有限步道 finite walk'''是一系列的边{{nowrap|(''e''<sub>1</sub>, ''e''<sub>2</sub>, …, ''e''<sub>''n'' − 1</sub>)}}，其顶点序列{{nowrap|(''v''<sub>1</sub>, ''v''<sub>2</sub>, …, ''v''<sub>''n''</sub>)}}。 {{nowrap begin}}''ϕ''(''e''<sub>''i''</sub>) = {''v''<sub>''i''</sub>, ''v''<sub>''i'' + 1</sub>}{{nowrap end}}对于{{nowrap|1=''i'' = 1, 2, …, ''n'' − 1}}. {{nowrap|(''v''<sub>1</sub>, ''v''<sub>2</sub>, …, ''v''<sub>''n''</sub>)}} 是移动的顶点序列。如果 {{nowrap begin}}''v''<sub>1</sub> = ''v''<sub>''n''</sub>{{nowrap end}} ，则此步道封闭，反之则开放。'''无限步道 infinite walk'''是由一系列边组成的，它们的类型与这里描述的相同，但没有起点或终点，而一个半无限步道(或光线)则有起点但是没有终点。
+
:以一个图为例 ''G'' = (''V'', ''E'', ''ϕ'') 。'''有限步道 finite walk'''是一系列的边 (''e''<sub>1</sub>, ''e''<sub>2</sub>, …, ''e''<sub>''n'' − 1</sub>)，其顶点序列(''v''<sub>1</sub>, ''v''<sub>2</sub>, …, ''v''<sub>''n''</sub>) 。 ''ϕ''(''e''<sub>''i''</sub>) = {''v''<sub>''i''</sub>, ''v''<sub>''i'' + 1</sub>}对于''i'' = 1, 2, …, ''n'' − 1 . (''v''<sub>1</sub>, ''v''<sub>2</sub>, …, ''v''<sub>''n''</sub>) 是移动的顶点序列。如果 ''v''<sub>1</sub> = ''v''<sub>''n''</sub> ，则此步道封闭，反之则开放。'''无限步道 infinite walk'''是由一系列边组成的，它们的类型与这里描述的相同，但没有起点或终点，而一个半无限步道(或光线)则有起点但是没有终点。
  
  
−
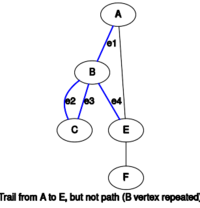
* '''轨迹 trail'''是所有的边缘都清晰可见的步道。{{sfn|Bender|Williamson|2010|p=162}}
+
* '''轨迹 trail'''是所有的边缘都清晰可见的步道。
  
−
* '''路径 path'''是一条所有顶点（包括所有边）都是不同的轨迹。{{sfn|Bender|Williamson|2010|p=162}}
+
* '''路径 path'''是一条所有顶点（包括所有边）都是不同的轨迹。
  
  

2020年12月3日 (四) 23:25的版本

本词条由Ryan初步翻译 由CecileLi初步审校；于2020.11.19再次审校，若有遗漏敬请谅解。

图1：一个三维的超立方体图表显示一个红色的哈密顿图，和一个黑色的最长诱导路径

在图论 Graph theory中，图中的路径 path是一个有限或无限的边序列，这些边连接着一系列顶点，这些顶点在大多数定义中都是不同的(又因为这些顶点是不同的，所以图中的边也是不同的)。有向图 directed path（有时也称为dipath[1]）中的有向路径，在具有附加条件：所有的边都方向相同时是一个有限或无限的边序列，它连接一系列不同的顶点。


路径是图论的基本概念，在大多数图论文本的导论部分都有描述，见Bondy and Murty（1976） ， Gibbons（1985），或Diestel（2005）。 Korte（1990）等人著作中，涵盖了更多关于图中路径的高级算法的话题。


定义

步道 轨迹 路径 Walk, trail, path

图2：Trail from A to E，but not path 从A到E的轨迹而非路径


  • 步道 Walk：是连接一系列顶点形成的有限或无限的边序列。

Let G = (V, E, ϕ) be a graph. A finite walk is a sequence of edges (e1, e2, …, en − 1) for which there is a sequence of vertices (v1, v2, …, vn) such that 模板:Nowrap beginϕ(ei) = {vi, vi + 1}模板:Nowrap end for i = 1, 2, …, n − 1. (v1, v2, …, vn) is the vertex sequence of the walk. This walk is closed if 模板:Nowrap beginv1 = vn模板:Nowrap end, and open else. An infinite walk is a sequence of edges of the same type described here, but with no first or last vertex, and a semi-infinite walk (or ray) has a first vertex but no last vertex.

Let be a graph. A finite walk is a sequence of edges for which there is a sequence of vertices such that ϕ(ei) = {vi, vi + 1} for . is the vertex sequence of the walk. This walk is closed if v1 = vn, and open else. An infinite walk is a sequence of edges of the same type described here, but with no first or last vertex, and a semi-infinite walk (or ray) has a first vertex but no last vertex.

以一个图为例 G = (V, E, ϕ) 。有限步道 finite walk是一系列的边 (e1, e2, …, en − 1)，其顶点序列(v1, v2, …, vn) 。 ϕ(ei) = {vi, vi + 1}对于i = 1, 2, …, n − 1 . (v1, v2, …, vn) 是移动的顶点序列。如果 v1 = vn ，则此步道封闭，反之则开放。无限步道 infinite walk是由一系列边组成的，它们的类型与这里描述的相同，但没有起点或终点，而一个半无限步道(或光线)则有起点但是没有终点。


  • 轨迹 trail是所有的边缘都清晰可见的步道。
  • 路径 path是一条所有顶点（包括所有边）都是不同的轨迹。


If w = (e1, e2, …, en − 1) is a finite walk with vertex sequence (v1, v2, …, vn) then w is said to be a walk from v1 to vn. Similarly for a trail or a path. If there is a finite walk between two distinct vertices then there is also a finite trail and a finite path between them.

If is a finite walk with vertex sequence then w is said to be a walk from v1 to vn. Similarly for a trail or a path. If there is a finite walk between two distinct vertices then there is also a finite trail and a finite path between them.

如果 w = (e1, e2, …, en − 1)是有顶点的序列 (v1, v2, …, vn)，那么 w 就是从 v1 到 vn的有限步道。同样，对于一条轨迹或者一条路径也是如此。如果两个不同的顶点之间有一个有限步道，那么在它们之间也有一条有限的轨迹和一条有限的路径。


有些学者对路径的定义中并不要求它的所有顶点都是不同的，而是使用术语“简单路径”来指代这样的路径。


一个加权图 weighted graph将一个值（权重）与图中的每条边相关联。一个加权图中的步道（或轨迹或路径）的权是所有边的权之和。有时，“成本 cost”或“长度 length”这两个词可以用来指代“权重”这一概念。


有向的步道，轨迹，路径 Directed walk, trail, path

  • 有向步道 directed walk是指由连接一系列顶点的边沿相同方向定向形成的有限或无限序列。模板:Sfn

Let G = (V, E, ϕ) be a directed graph. A finite directed walk is a sequence of edges (e1, e2, …, en − 1) for which there is a sequence of vertices (v1, v2, …, vn) such that ϕ(ei) = (vi, vi + 1) for i = 1, 2, …, n − 1. (v1, v2, …, vn) is the vertex sequence of the directed walk. An infinite directed walk is a sequence of edges of the same type described here, but with no first or last vertex, and a semi-infinite directed walk (or ray) has a first vertex but no last vertex.

以一个有向图G = (V, E, ϕ)为例。有限有向步道由一系列的边组成 (e1, e2, …, en − 1)，而对于这些边，又存在一系列的顶点 (v1, v2, …, vn)。ϕ(ei) = (vi, vi + 1)对于i = 1, 2, …, n − 1. (v1, v2, …, vn)是有向步道的顶点序列。无限有向步道是一个边序列，其类型与本文描述的相同，但起点或终点，而半无限有向步道(或射线)有起点，但没有终点。

  • A directed trail is a directed walk in which all edges are distinct.

有向轨迹 directed trail是指所有边都可见的轨迹。模板:Sfn

  • A directed path is a directed trail in which all vertices are distinct.

有向路径 directed path是指所有顶点都不同的有向路径。模板:Sfn


If w = (e1, e2, …, en − 1) is a finite directed walk with vertex sequence (v1, v2, …, vn) then w is said to be a walk from v1 to vn. Similarly for a directed trail or a path. If there is a finite directed walk between two distinct vertices then there is also a finite directed trail and a finite directed path between them.

假设一个步道w = (e1, e2, …, en − 1)是顶点序列有限的(v1, v2, …, vn)有向步道，那么 w 就是从 v1 到 vn的步道。同样，对于有向轨迹或路径也是如此。如果在两个不同的顶点之间存在有限有向步道，那么在它们之间也存在有限有向轨迹和有限有向路径。


有些学者并不要求有向路径的所有顶点都是不同的，因此他们用“简单有向路径 simple directed path”这个术语来表示它。


A weighted directed graph associates a value (weight) with every edge in the directed graph. The weight of a directed walk (or trail or path) in a weighted directed graph is the sum of the weights of the traversed edges. Sometimes the words cost or length are used instead of weight.

A weighted directed graph associates a value (weight) with every edge in the directed graph. The weight of a directed walk (or trail or path) in a weighted directed graph is the sum of the weights of the traversed edges. Sometimes the words cost or length are used instead of weight.

一个加权有向图将一个值（权重）与有向图中的每条边相关联。加权有向图中有向步道（或路径）的权是有向边权重的和。有时，“成本”或“长度”这两个词可以用来指代“权重”这一概念。

例子

  • 如果路径包含每一对顶点，那么图就是连通的。
  • A directed graph is strongly connected if there are oppositely oriented directed paths containing each pair of vertices.

如果一个有向图中存在包含每对顶点的相对有向路径，那么这个有向图就是紧密连通的。

  • 没有边连接两个不连续的路径顶点的路径称为诱导路径 induced path。
  • 包含图的每个顶点的路径称为哈密顿路径 Hamiltonian Path。
  • Two paths are vertex-independent (alternatively, internally vertex-disjoint) if they do not have any internal vertex in common. Similarly, two paths are edge-independent (or edge-disjoint) if they do not have any internal edge in common. Two internally vertex-disjoint paths are edge-disjoint, but the converse is not necessarily true.

如果两条路径没有任何共同的内部顶点，那么它们与顶点无关（换言之，内部顶点不相交）。类似地，如果两条路径没有任何共同的内边，那么它们是边独立的（或边不相交）。两条内部顶点不相交的路径的边也不相交，但反之未必正确的。

  • 图中两个顶点之间，如果最短路径存在，那么它们的的距离是最短路径的长度，否则该距离是无穷大。
  • 连接图的直径是图的顶点对之间的最大距离（如上定义）。


路径寻找 Finding paths

Several algorithms exist to find shortest and longest paths in graphs, with the important distinction that the former problem is computationally much easier than the latter.

Several algorithms exist to find shortest and longest paths in graphs, with the important distinction that the former problem is computationally much easier than the latter.

求解图的最短路径和最长路径的均有多种算法，但是前者的计算比后者简单得多。


Dijkstra's algorithm produces a list of shortest paths from a source vertex to every other vertex in directed and undirected graphs with non-negative edge weights (or no edge weights), whilst the Bellman–Ford algorithm can be applied to directed graphs with negative edge weights. The Floyd–Warshall algorithm can be used to find the shortest paths between all pairs of vertices in weighted directed graphs.

Dijkstra's algorithm produces a list of shortest paths from a source vertex to every other vertex in directed and undirected graphs with non-negative edge weights (or no edge weights), whilst the Bellman–Ford algorithm can be applied to directed graphs with negative edge weights. The Floyd–Warshall algorithm can be used to find the shortest paths between all pairs of vertices in weighted directed graphs.

Dijkstra 算法产生了一个从源顶点到无向或有向图中每个顶点的最短路径列表，而Bellman-Ford 算法 可以应用于具有负边权重的有向图。利用 Floyd-Warshall 算法可以求出加权有向图中所有顶点对之间的最短路径。

参见


References 引用

  1. ↑ Graph Structure Theory: Proceedings of the AMS-IMS-SIAM Joint Summer Research Conference on Graph Minors, Held June 22 to July 5, 1991, p.205
  • Gibbons, A. (1985). Algorithmic Graph Theory. Cambridge University Press. p. 5-6. ISBN 0-521-28881-9. 

Category:Graph theory objects

范畴: 图论对象

Category:Graph connectivity

类别: 图形连接性


模板:Interwiki extra


This page was moved from wikipedia:en:Path (graph theory). Its edit history can be viewed at 路径/edithistory

取自“https://wiki.swarma.org/index.php?title=路径_path&oldid=19628”