奇点

在数学中，奇点 singularity一般是一个给定数学对象没有定义的点，或一个数学对象在某些特定方面不再表现良好的点，例如缺乏可微性或可分析性。

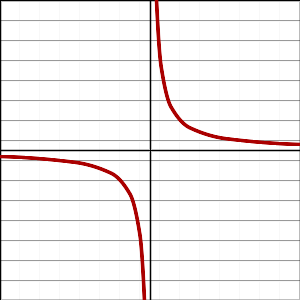
例如，实值函数

[math]\displaystyle{ f(x)=\frac{1}{x} }[/math]

在[math]\displaystyle{ x = 0 }[/math]处有一个奇点，在这里它似乎“爆炸”到±∞，因此没有定义。绝对值函数[math]\displaystyle{ g(x) = |x| }[/math]在[math]\displaystyle{ x = 0 }[/math]处也有奇点，因为它在那里不可微。

在 [math]\displaystyle{ (x, y) }[/math] 坐标系中由 [math]\displaystyle{ \{(x,y):y^3-x^2=0\} }[/math] 定义的代数曲线在[math]\displaystyle{ (0, 0) }[/math]处有一个奇点（称为尖点）。关于代数几何中的奇点，参见代数簇中的奇点。关于微分几何中的奇点，参见奇点理论。

实际分析

在实际分析中，奇点要么是不连续的，要么是导数的不连续（有时也是高阶导数的不连续）。有四种不连续：I型，有两种亚型；II型，也可分为两种亚型（尽管通常不是）。

为了描述这两种极限的使用方式，假设[math]\displaystyle{ f(x) }[/math]是实参数[math]\displaystyle{ x }[/math]的函数，对于其自变量的任意值，比如[math]\displaystyle{ c }[/math]，则左极限 [math]\displaystyle{ f(c^-) }[/math]和右极限[math]\displaystyle{ f(c^+) }[/math]的定义如下：

[math]\displaystyle{ f(c^-) = \lim_{x \to c}f(x) }[/math], 当[math]\displaystyle{ x \lt c }[/math]，

[math]\displaystyle{ f(c^+) = \lim_{x \to c}f(x) }[/math], 当[math]\displaystyle{ x \gt c }[/math]。

值[math]\displaystyle{ f(c^-) }[/math] 是函数[math]\displaystyle{ f(x) }[/math]在值[math]\displaystyle{ x }[/math]从下接近[math]\displaystyle{ c }[/math]时趋向的值，而值[math]\displaystyle{ f(c^+) }[/math]是函数[math]\displaystyle{ f(x) }[/math] 在值[math]\displaystyle{ x }[/math]从上接近[math]\displaystyle{ c }[/math]时趋向的值，而不管函数在[math]\displaystyle{ x = c }[/math]点处的实际值如何

有些函数根本不存在这些限制。例如，函数

[math]\displaystyle{ g(x) = \sin\left(\frac{1}{x}\right) }[/math]

在[math]\displaystyle{ x }[/math]趋于[math]\displaystyle{ c = 0 }[/math]时不趋向任何值。在这种情况下，极限不是无限的，而是没有定义的：[math]\displaystyle{ g(x) }[/math]没有确定的值。借用复分析，这有时被称为本质奇点（本性奇点）essential singularity。

参数为给定值[math]\displaystyle{ c }[/math]时的可能情况如下。

• 连续性点是[math]\displaystyle{ c }[/math]的一个值，在这点[math]\displaystyle{ f(c^-) = f(c) = f(c^+) }[/math],就像人们期望的光滑函数一样。所有的值必须是有限的。如果[math]\displaystyle{ c }[/math]不是连续点，则在[math]\displaystyle{ c }[/math]处发生不连续。

• 当[math]\displaystyle{ f(c^-) }[/math]和[math]\displaystyle{ f(c^+) }[/math]同时存在且为有限时，即出现I型不连续，但是也至少适用以下三个条件中的一个：

• • [math]\displaystyle{ f(c^-) \neq f(c^+) }[/math]
  • 当[math]\displaystyle{ x = c }[/math]时，[math]\displaystyle{ f(x) }[/math] 没有定义；
  • 或[math]\displaystyle{ f(c) }[/math]有一个定义值，但它与两个极限的值不匹配。

I型不连续性可以进一步区分为下列亚型之一:

• 当[math]\displaystyle{ f(c^-) \neq f(c^+) }[/math]时，无论是否定义了[math]\displaystyle{ f(c) }[/math]，也不管是否定义了[math]\displaystyle{ f(c) }[/math]的值，都会出现跳跃不连续。

• 当[math]\displaystyle{ f(c^-) = f(c^+) }[/math]时，无论是否定义了[math]\displaystyle{ f(c) }[/math]，也不管是否已定义它的值（但不匹配两个极限）时，都会出现可移动的不连续性。

• 当[math]\displaystyle{ f(c^-) }[/math]或[math]\displaystyle{ f(c^+) }[/math] 不存在时（可能两者都不存在），就会出现II型不连续性。这有两个亚型，通常不单独考虑：
  • 无限不连续是当左极限或右极限不存在时的特例，特别是因为它是无限的，而另一个极限要么是无限的，要么是某种定义良好的有限数。换句话说，当函数的图形有一个垂直渐近线时，函数具有无限的不连续性。
  • 本质奇点（本性奇点）是从复分析中借用的一个术语（见下文）。当极限 [math]\displaystyle{ f(c^-) }[/math]或[math]\displaystyle{ f(c^+) }[/math]两者中的任意一者不存在时，情况就会如此，但不是因为它是一个“无限不连续性”。”本质奇点（本性奇点）“接近无限制，即使有效解扩展到包括[math]\displaystyle{ \pm\infty }[/math]。

在实际分析中，奇点或不连续是函数本身的一个性质。任何可能存在于函数导数中的奇点都被认为是属于导数，而不是原函数。

坐标奇点 Coordinate singularities

当在一个坐标系中出现明显的奇异性或不连续性时，就会出现坐标奇点 coordinate singularity，可以通过选择不同的坐标系来消除。这方面的一个例子是在球面坐标系中90度纬度处的明显奇异性。在球体表面正北方移动的物体（例如，沿经度为0度的直线）将突然在极点处经历经度的瞬时变化（在本例中，从经度0跳到经度180度）。然而，这种不连续性只是显而易见的；它是所选坐标系的一个伪影，在极点处是奇异的。不同的坐标系将消除明显的不连续性（例如，用矢量表示代替经纬度表示法）。

复分析 Complex analysis

在复分析中，有几类奇点。其中包括孤立奇点 isolated singularities、非孤立奇点 nonisolated singularities 和分支点 branch points。

孤立奇点 Isolated singularities

假设 U 是复数 C 的一个开子集，点 a 是 U 的一个元素，而 f 是定义在 a 周围某个邻域上的复可微函数，除了 a: U \ {a} ，那么:

• 如果存在一个定义在所有U上的全纯函数g，使得对于U \ {a}中的所有z,f(z) = g(z)，那么点a是f的一个可去奇点。函数g是函数f的连续替换。
• 如果存在定义在 U上的全纯函数 g，且g(a) 非零，且存在一个自然数n，使得对所有z属于U \ {a},f(z) = g(z) / (z − a)ⁿ，则点a为极点（复分析）或f的非本质奇点。最小的这个数n称为“极序”。非本质奇点处的导数本身也有一个非本质奇点，当n增加1时（除非n为0，因此奇点可移除）。
• 如果点a既不是可去奇点，也不是极点，则它是f的非本质奇点。点a是非本质奇点当且仅当洛朗级数具有无穷多个负次幂。

非孤立奇点 Nonisolated singularities

除孤立奇点外，一个变量的复变函数还可能表现出其他奇异行为。这些称为非孤立奇点，其中有两种类型:

• 簇点 Cluster points：孤立奇点的极限点 limit points。如果它们都是极点，尽管在每个极点上都有劳伦级数 Laurent series展开式，那么在极限条件下，这样的展开是不可能的。
• 自然边界 Natural boundaries：函数不能 解析连续在其周围（或在其外部，如果它们是黎曼球面上的闭合曲线）的任何非孤立集合（如曲线）。

分支点 Branch points

分支点 Branch point通常是一个多值函数的结果，如[math]\displaystyle{ \sqrt{z} }[/math]或[math]\displaystyle{ \log(z) }[/math]，它们被定义在一个特定的限定域中，这样函数就可以在该域中成为单值函数。切线是被排除在域之外的一条线或曲线，用于在不连续的函数值之间引入技术分离。当真正需要切割时，该函数在分支切割的每一边都有明显不同的值。分枝切割的形状是一个选择的问题，即使它必须连接两个不同的分支点(如[math]\displaystyle{ \log(z) }[/math]的[math]\displaystyle{ z=0 }[/math]和[math]\displaystyle{ z=\infty }[/math])，这两个分支点是固定的。

有限时间奇点 Finite-time singularity

倒数函数 The reciprocal function，显示双曲增长 hyperbolic growth.

当一个输入变量为时间时，而一个输出变量在有限时间趋于无穷大时，就会出现有限时间奇点 finite-time singularity。这些在运动学和偏微分方程中很重要——无穷大在物理上并不存在，但在奇点附近的行为通常是令人感兴趣的。在数学上，最简单的有限时间奇点是[math]\displaystyle{ x^{-\alpha} }[/math]形式的各种指数的幂律，其中最简单的是双曲增长，其中指数为（负）1：[math]\displaystyle{ x^{-1} }[/math] 。更准确地说，为了随着时间的推移在正时间处获得奇点（因此输出增长到无穷大），可以使用 [math]\displaystyle{ (t_0-t)^{-\alpha} }[/math]（使用t表示时间，将方向反转为[math]\displaystyle{ -t }[/math]，以便时间增加到无穷大，并将奇点从0向前移动到固定时间[math]\displaystyle{ t_0 }[/math]）。

一个例子是一个非弹性球在平面上的反弹运动。如果考虑理想化的运动，即每次弹跳动能损失的比例相同，反弹的频率就变得无限大，因为球在有限时间内静止。有限时间奇点的其他例子包括潘列夫悖论 Painlevé paradox的各种形式（例如，在黑板上拖动粉笔时，粉笔会跳跃的趋势），以及在平面上旋转的硬币的进动率如何在突然停止之前加速到无限大（正如使用欧拉圆盘 Euler's Disk玩具所研究的那样）。

假设的例子包括海因茨·冯·福斯特 Heinz von Foerster滑稽的“世界末日方程 Doomsday's equation”（简单化模型在有限时间内产生无限多的人口）。

代数几何与交换代数 Algebraic geometry and commutative algebra

在代数几何中，代数簇的奇点是簇中切线空间可能没有规则定义的一点。奇点最简单的例子就是它们自己交叉的曲线。但是还有其他类型的奇点，比如尖点。例如，方程 [math]\displaystyle{ y^2 − x^3 = 0 }[/math]定义了一条在原点[math]\displaystyle{ x= y = 0 }[/math]有一个尖点的曲线。可以将x轴定义为这一点的切线，但这个定义不能与其他点的定义相同。实际上，在这种情况下,-轴是一个“双切线”。

对于仿射簇 affine variety和射影簇 projective variety，奇点是指雅可比矩阵 Jacobian matrix的秩低于簇中其他点的秩的点。

可以给出一个关于交换代数 commutative algebra的等价定义，它扩展到抽象的簇和方案: 如果局部环在这一点上不是一个正则局部环，那么该点为奇点。

参见

• 突变理论 Catastrophe theory
• 定义和未定义 Defined and undefined
• 退化（数学） Degeneracy (mathematics)
• 除以零 Division by zero
• 双曲线增长 Hyperbolic growth
• 病理学（数学） Pathological (mathematics)
• 奇异解 Singular solution
• 可移动奇点 Removable singularity

参考文献

---

本中文词条由Henry翻译，Bai审校，薄荷欢迎在讨论页面留言。

本词条内容源自公开资料，遵守 CC3.0协议。