伯努瓦·曼德布洛特 Benoit Mandelbrot

来自集智百科 - 复杂系统|人工智能|复杂科学|复杂网络|自组织
跳到导航 跳到搜索

此词条Jie翻译。

See also: Mandelbrot set

伯努瓦 曼德布洛特
Born1924年11月20日
波兰华沙
Died2010年10月14日
美国马萨诸塞州剑桥
Nationality法国，美国，波兰
Alma mater巴黎综合理工学院，加州理工学院，巴黎大学
Known for曼德布罗集，混沌理论，分形，Zipf –曼德尔布洛特法则
Spouse(s)阿利耶特·卡甘（1955-2010）
Awards法国荣誉军团勋章（1990年侠士·2006年警官），2003日本国际奖，1993沃尔夫奖，1989年哈维奖，1986年富兰克林奖章，1985年巴纳德奖章
Scientific career
Fields数学，空气动力学
Institutions耶鲁大学，IBM公司，太平洋西北国家实验室
Doctoral advisor保罗·皮埃尔·莱维
Doctoral studentsL-E. 卡维，尤金·法玛，肯·穆斯格雷夫，穆拉德·塔克
Influences约翰内斯·开普勒，保罗·列维，索莱姆·曼德布罗特
Influenced纳西姆·尼古拉斯·塔勒布


伯努瓦[n 1] 曼德布洛特[1]|group=n}}（1924年11月20日至2010年10月14日）是波兰裔法国裔美国数学家和博学家，对实用科学有着广泛的研究。[2][3][4]他热衷于研究物理现象的“粗糙艺术”和“生活中不受控制的元素”，并且将自己称为“分形主义者”，因其对分形几何学领域的贡献而受到认可，其中包括创造了“ 分形 Fractal”一词，[5]并发展了自然界中的“ 粗糙度Roughness和 自相似性Self-similarity ”理论。[6]

1936年，当曼德布罗特还是个孩子时，一家人从波兰华沙移民到了法国。第二次世界大战结束后，曼德布洛特学习了数学，从巴黎和美国的大学毕业，并获得了加州理工学院的航空硕士学位。他的职业生涯大部分时间都是在美国和法国度过，拥有法国和美国双重国籍。1958年，他在IBM开始了35年的职业生涯，并在那里成为了IBM院士，定期请假到哈佛大学任教。在哈佛大学发表关于棉花期货的美国商品市场研究之后，他开始教授经济学和应用科学。


Because of his access to IBM's computers, Mandelbrot was one of the first to use computer graphics to create and display fractal geometric images, leading to his discovery of the Mandelbrot set in 1980. He showed how visual complexity can be created from simple rules. He said that things typically considered to be "rough", a "mess", or "chaotic", such as clouds or shorelines, actually had a "degree of order".[7] His math and geometry-centered research career included contributions to such fields as statistical physics, meteorology, hydrology, geomorphology, anatomy, taxonomy, neurology, linguistics, information technology, computer graphics, economics, geology, medicine, physical cosmology, engineering, chaos theory, econophysics, metallurgy, and the social sciences.[8]

由于曼德布罗特可以使用IBM的计算机，他是最早使用计算机图形来创建和显示分形几何图像的人之一。他于1980年发现了曼德布洛特集合，展示了如何从简单的规则图形创建出视觉复杂的图形。他认为那些通常被认为是“粗糙”，“杂乱”或“混乱”的事物，例如云层或海岸线，实际上都具有“ 有序度Degree of order ”的性质。他以数学和几何学为中心的延申研究领域包括了统计物理学，气象学，水文学，地貌学，解剖学，分类学，神经学，语言学，信息技术，计算机图形学，经济学，地质学，医学，物理宇宙学，工程学，混沌理论等领域的贡献 ，经济物理学，冶金学和社会科学。


Toward the end of his career, he was Sterling Professor of Mathematical Sciences at Yale University, where he was the oldest professor in Yale's history to receive tenure.[9] Mandelbrot also held positions at the Pacific Northwest National Laboratory, Université Lille Nord de France, Institute for Advanced Study and Centre National de la Recherche Scientifique. During his career, he received over 15 honorary doctorates and served on many science journals, along with winning numerous awards. His autobiography, The Fractalist: Memoir of a Scientific Maverick, was published posthumously in 2012. 在他职业生涯后期，他担任耶鲁大学数学科学系的斯特林教授，并且被授予了耶鲁历史上最年长的终身教授职位。曼德布罗特还在西北太平洋国家实验室，里尔-北法兰西院校联盟，普林斯顿高等研究院和法国国家科学研究中心担任过职务。他的自传《分形主义者:一个科学特立独行者的回忆录The Fractalist: Memoir of a Scientific Maveric》于2012年死后出版。


Early years 早年生活

家庭背景和早期教育，（4:11）伯努瓦 曼德布洛特访谈，《Web of Stories》 144第1部分

Mandelbrot was born in a Lithuanian Jewish family, in Warsaw during the Second Polish Republic.[10] His father made his living trading clothing; his mother was a dental surgeon. During his first two school years, he was tutored privately by an uncle who despised rote learning: "Most of my time was spent playing chess, reading maps and learning how to open my eyes to everything around me."[11] In 1936, when he was 11, the family emigrated from Poland to France. The move, the war, and his acquaintance with his father's brother, the mathematician Szolem Mandelbrojt (who had moved to Paris around 1920), further prevented a standard education. "The fact that my parents, as economic and political refugees, joined Szolem in France saved our lives," he writes.[5]:17[12] 曼德布洛特出生于波兰第二共和国时期，华沙的一个立陶宛犹太家庭。父亲以服装贸易为生，母亲是一名牙科医生。他入学后的前两年，一直由他叔叔私下辅导，这位叔叔尤其鄙视死记硬背的学习方法：“我的大部分时间都花在下棋，阅读地图和学习如何打开我的视角观察周围的一切。”后来1936年，他11岁时，一家人从波兰移民到了法国。这次移民，战争，和他父亲兄弟Szolem Mandelbrojt（数学家斯佐勒姆·曼德尔贝罗亚特，1920年左右移居巴黎）的接触，更进一步阻碍了他受到规范的教育。他曾写道：“我的父母作为经济和政治难民，在法国投靠了佐勒姆，因此而挽救了我们的生命。”


Mandelbrot attended the Lycée Rolin in Paris until the start of World War II, when his family moved to Tulle, France. He was helped by Rabbi David Feuerwerker, the Rabbi of Brive-la-Gaillarde, to continue his studies.[5]:62–63[13] Much of France was occupied by the Nazis at the time, and Mandelbrot recalls this period:

Mandelbrot attended the Lycée Rolin in Paris until the start of World War II, when his family moved to Tulle, France. He was helped by Rabbi David Feuerwerker, the Rabbi of Brive-la-Gaillarde, to continue his studies.[8]:62–63[17] Much of France was occupied by the Nazis at the time, and Mandelbrot recalls this period:

曼德布罗特在巴黎罗兰公立中学学习，一直到第二次世界大战开始，后来他的家人搬到了法国的蒂勒。后来犹太教教士大卫·菲尔韦克Rabbi David Feuerwerker（布里夫拉盖勒德犹太教教士the Rabbi of Brive-la-Gaillarde）帮助他继续学业。当时法国大部分地区都被纳粹占领，曼德布罗特回忆起这段时期：


Our constant fear was that a sufficiently determined foe might report us to an authority and we would be sent to our deaths. This happened to a close friend from Paris, Zina Morhange, a physician in a nearby county seat. Simply to eliminate the competition, another physician denounced her ... We escaped this fate. Who knows why?


我们一直担心的是，一旦敌人下定决心将我们报告给当局，等待我们的就是死刑。类似的事情就发生在巴黎的一位密友Zina Morhange深上，她曾是附近某个县城的医生。当时只是为了解决竞争对手，另一位医生就举报了她...而我们逃过了一劫。谁知道是为什么？


In 1944, Mandelbrot returned to Paris, studied at the Lycée du Parc in Lyon, and in 1945 to 1947 attended the École Polytechnique, where he studied under Gaston Julia and Paul Lévy. From 1947 to 1949 he studied at California Institute of Technology, where he earned a master's degree in aeronautics.[14] Returning to France, he obtained his PhD degree in Mathematical Sciences at the University of Paris in 1952.[11]


1944年，曼德布洛特回到巴黎，在里昂的帕克中学学习，并于1945年至1947年考上了巴黎综合理工学院，在加斯顿·朱莉亚Gaston Julia和保罗·列维Paul Lévy的指导下学习。之后的1947年到1949年，他就读于加利福尼亚理工学院，在那里获得了航空硕士学位。返回法国后，他于1952年在巴黎大学获得数学科学博士学位。

Research career 科研生涯

From 1949 to 1958, Mandelbrot was a staff member at the Centre National de la Recherche Scientifique. During this time he spent a year at the Institute for Advanced Study in Princeton, New Jersey, where he was sponsored by John von Neumann. In 1955 he married Aliette Kagan and moved to Geneva, Switzerland (to collaborate with Jean Piaget at the International Centre for Genetic Epistemology) and later to the Université Lille Nord de France.[15] In 1958 the couple moved to the United States where Mandelbrot joined the research staff at the IBM Thomas J. Watson Research Center in Yorktown Heights, New York.[15] He remained at IBM for 35 years, becoming an IBM Fellow, and later Fellow Emeritus.[11]


自1949年到1958年，曼德布洛特任职于法国国家科学研究中心。在此期间，他得到了约翰·冯·诺伊曼John von Neumann的赞助，在新泽西州普林斯顿的高级研究学院度过了一年。1955年，他与阿利耶特·卡甘结婚，并搬到瑞士日内瓦（与国际遗传认识论中心的让·皮亚杰Jean Piaget合作），后来又迁往法国里尔大学。到了1958年，这对夫妇搬到了美国，在那里曼德布洛特加入了位于纽约约克敦高地的IBM托马斯·沃森研究中心。他在IBM待了35年，并且成为IBM院士，后来成为了荣誉退休院士。


From 1951 onward, Mandelbrot worked on problems and published papers not only in mathematics but in applied fields such as information theory, economics, and fluid dynamics.

从1951年起，曼德布洛特开始致力于研究相关问题并发表论文，不仅在数学领域，而且在信息论，经济学和流体动力学等应用领域中也发表许多研究论文。


Randomness in financial markets 金融市场的随机性

Mandelbrot saw financial markets as an example of "wild randomness", characterized by concentration and long range dependence. He developed several original approaches for modelling financial fluctuations.[16] In his early work, he found that the price changes in financial markets did not follow a Gaussian distribution, but rather Lévy stable distributions having infinite variance. He found, for example, that cotton prices followed a Lévy stable distribution with parameter α equal to 1.7 rather than 2 as in a Gaussian distribution. "Stable" distributions have the property that the sum of many instances of a random variable follows the same distribution but with a larger scale parameter.[17]

曼德布洛特将金融市场视为“野生随机性”的很好案例，其特征是集中性和长相关性。为此他开发了几种可以模拟财务波动的创新方法。在他的早期工作中，他发现金融市场的价格变化并非遵循高斯分布，而是遵循具有无限方差的 列维稳定分布Lévy stable distributions 。他发现，例如棉花价格遵循列维稳定分布，其参数α等于1.7，而不是高斯分布中的2。该“稳定”分布具有以下性质：随机变量的许多实例总和遵循相同的分布，只是比例参数较大。


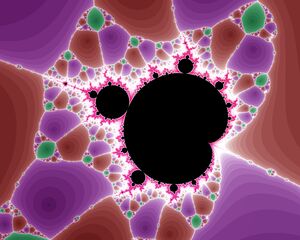
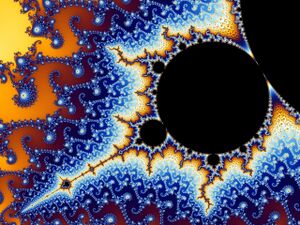
Developing "fractal geometry" and the Mandelbrot set “分形几何”和曼德布洛特集合的发展

As a visiting professor at Harvard University, Mandelbrot began to study fractals called Julia sets that were invariant under certain transformations of the complex plane. Building on previous work by Gaston Julia and Pierre Fatou, Mandelbrot used a computer to plot images of the Julia sets. While investigating the topology of these Julia sets, he studied the Mandelbrot set which was introduced by him in 1979. In 1982, Mandelbrot expanded and updated his ideas in The Fractal Geometry of Nature.[18] This influential work brought fractals into the mainstream of professional and popular mathematics, as well as silencing critics, who had dismissed fractals as "program artifacts".


作为哈佛大学的客座教授，曼德布洛特开始研究名为 朱莉娅集合Julia sets的分形，这些分形在复杂平面的变换下依旧保持不变。在加斯顿·朱莉娅Gaston Julia和皮埃尔·法图Pierre Fatou先前工作的基础上，曼德尔布洛特使用计算机绘制出了朱莉娅集合的图像。在他研究这些朱莉娅集的拓扑时，他于1979年提出的曼德布洛特集。1982年，他在《大自然的分形几何学The Fractal Geometry of Nature》一书中扩展并更新了他的思想。但是这项颇具影响力的著作不仅将分形技术引入到专业数学和大众数学中，也同时进入到了那些将分形技术仅视为“程序工件”的批评者眼中。


曼德布洛特在2006年法国荣誉军团勋章的提名演讲，当时他提到了曼德布洛特集合


In 1975, Mandelbrot coined the term fractal to describe these structures and first published his ideas, and later translated, Fractals: Form, Chance and Dimension.[19] According to computer scientist and physicist Stephen Wolfram, the book was a "breakthrough" for Mandelbrot, who until then would typically "apply fairly straightforward mathematics ... to areas that had barely seen the light of serious mathematics before".[7] Wolfram adds that as a result of this new research, he was no longer a "wandering scientist", and later called him "the father of fractals":


1975年，曼德布洛特创造了“分形”一词来描述这些结构，并首先发表了他的想法，其翻译为《分形：形式，机会和维度Fractals: Form, Chance and Dimension》。根据计算机科学家和物理学家斯蒂芬·沃尔夫拉姆Stephen Wolfram的说法，这本书对曼德尔布洛特来说是一个“突破”，他在那之前通常会“将相当简单的数学应用于……以前几乎没有看到过的严肃数学领域”。沃尔夫拉姆补充说，由于这项新研究，他不再是“流浪的科学家”，他被称为“分形之父”：


Mandelbrot ended up doing a great piece of science and identifying a much stronger and more fundamental idea—put simply, that there are some geometric shapes, which he called "fractals", that are equally "rough" at all scales. No matter how close you look, they never get simpler, much as the section of a rocky coastline you can see at your feet looks just as jagged as the stretch you can see from space.


事实证明曼德布洛特最终完成了一部伟大的科学著作。他建立了一个基本的概念，简单地说，有些几何形状（他称之为“分形”）在各个尺度上都同样“粗糙”。不管您凑多近去看，它们都永远不会变得简单，就像您在脚下看到的多岩石的海岸线与从太空中看到的伸展部分一样参差不齐。


Wolfram briefly describes fractals as a form of geometric repetition, "in which smaller and smaller copies of a pattern are successively nested inside each other, so that the same intricate shapes appear no matter how much you zoom in to the whole. Fern leaves and Romanesque broccoli are two examples from nature."[7] He points out an unexpected conclusion:


沃尔夫拉姆简要地将分形描述为几何重复的一种形式，“在其中，越来越少的相同复制图案相继被嵌套在彼此内部，因此无论您放大多少，同样的复杂形状都会展现出来。蕨叶和罗马式西兰花是自然界的两个例子。“他指出了一个出乎意料的结论：


One might have thought that such a simple and fundamental form of regularity would have been studied for hundreds, if not thousands, of years. But it was not. In fact, it rose to prominence only over the past 30 or so years—almost entirely through the efforts of one man, the mathematician Benoit Mandelbrot.


可能有人以为，这种简单而基本的规律已经被研究了数百年甚至数千年，但事实并非如此。实际上，它仅在过去30多年中才受到关注，而且几乎完全是通过一个人的努力，即数学家伯努瓦 曼德布洛特。


Mandelbrot used the term "fractal" as it derived from the Latin word "fractus", defined as broken or shattered glass. Using the newly developed IBM computers at his disposal, Mandelbrot was able to create fractal images using graphics computer code, images that an interviewer described as looking like "the delirious exuberance of the 1960s psychedelic art with forms hauntingly reminiscent of nature and the human body". He also saw himself as a "would-be Kepler", after the 17th-century scientist Johannes Kepler, who calculated and described the orbits of the planets.[20]

曼德布洛特使用“分形”一词，因为它源自拉丁语 fractus”，意为碎玻璃。通过使用新开发的IBM计算机和图形计算机代码，曼德布洛特创建了分形图像，这些图像被采访者描述为“1960年代迷幻艺术的疯狂，其形式令人不禁联想到自然和人体”。他还将自己视为“未来的开普勒”（在17世纪，科学家约翰尼斯·开普勒Johannes Kepler计算并描述了行星的轨道）。

一个德布洛特集合

Mandelbrot, however, never felt he was inventing a new idea. He describes his feelings in a documentary with science writer Arthur C. Clarke:

Mandelbrot, however, never felt he was inventing a new idea. He describes his feelings in a documentary with science writer Arthur C. Clarke:

但是，曼德布洛特从未觉得他在发明一个新概念。在他与科学作家亚瑟·克拉克Arthur C. Clarke的纪录片中他描述了自己的感受：


Exploring this set I certainly never had the feeling of invention. I never had the feeling that my imagination was rich enough to invent all those extraordinary things on discovering them. They were there, even though nobody had seen them before. It's marvelous, a very simple formula explains all these very complicated things. So the goal of science is starting with a mess, and explaining it with a simple formula, a kind of dream of science.

】 在探索这个集合的时候我并未感觉在发明一个新概念。我也从没有感觉到我的想象力足以发现所有这些非凡的事物。其实他们就一直呈现在那里，只是过去没有人注意过他们。一个如此简单的公式就可以解释所有这些异常复杂的事物，太难以置信了。因此，科学的目标是从一团乱开始，再由一个简单的公式来解释它。我想这是研究科学的梦想。


According to Clarke, "the Mandelbrot set is indeed one of the most astonishing discoveries in the entire history of mathematics. Who could have dreamed that such an incredibly simple equation could have generated images of literally infinite complexity?" Clarke also notes an "odd coincidence

the name Mandelbrot, and the word "mandala"—for a religious symbol—which I'm sure is a pure coincidence, but indeed the Mandelbrot set does seem to contain an enormous number of mandalas.[21]


克拉克Clarke说：“曼德布洛特集确实是整个数学史上最惊人的发现之一。谁能想到，如此难以置信的简单方程式就可以生成视觉上无限复杂的图像？“克拉克还注意到了一个奇怪的巧合”：我确信曼德布洛特的名称和“曼陀罗”（一个宗教象征）一词纯属巧合，但确实曼德布洛特集似乎包含了大量的曼陀罗。


Mandelbrot left IBM in 1987, after 35 years and 12 days, when IBM decided to end pure research in his division.[22] He joined the Department of Mathematics at Yale, and obtained his first tenured post in 1999, at the age of 75.[23] At the time of his retirement in 2005, he was Sterling Professor of Mathematical Sciences.


经过35年零12天的努力，曼德布洛特在1987年离开了IBM，当时IBM决定结束其部门的研究。随后他加入了耶鲁大学数学系，并于1999年以75岁的高龄获得了第一任终身职位。在2005年退休之时，他成为了斯特林数学科学教授。


Fractals and the "theory of roughness" 分形与“粗糙度理论”

Mandelbrot created the first-ever "theory of roughness", and he saw "roughness" in the shapes of mountains, coastlines and river basins; the structures of plants, blood vessels and lungs; the clustering of galaxies. His personal quest was to create some mathematical formula to measure the overall "roughness" of such objects in nature.[5]:xi He began by asking himself various kinds of questions related to nature:

曼德布洛特创造了第一个“粗糙度理论”，他看到了山脉，海岸线和河流盆地形状的“粗糙度”。植物，血管和肺的结构的“粗糙度”；还有星系聚集的“粗糙度”。他个人的追求是创建一些数学公式来测量此类物体在自然界中的整体“粗糙度”。他首先问自己各种与自然有关的问题：


Can geometry deliver what the Greek root of its name [geo-] seemed to promise—truthful measurement, not only of cultivated fields along the Nile River but also of untamed Earth?引用错误：没有找到与</ref>对应的<ref>标签[24]


曼德布洛特在1967年《科学》杂志上发表的论文《英国的海岸线有多长？统计自相似性和分形维数How Long Is the Coast of Britain? Statistical Self-Similarity and Fractional Dimension》中讨论了 豪斯多夫维数Hausdorff dimension的自相似曲线。这些都是分形的例子，尽管当时曼德布洛特在论文中并没有使用这个术语，因为他直到1975年才创造这个名词。该论文是曼德布洛特关于分形主题的第一批出版物之一。


Mandelbrot emphasized the use of fractals as realistic and useful models for describing many "rough" phenomena in the real world. He concluded that "real roughness is often fractal and can be measured."[5]:296 Although Mandelbrot coined the term "fractal", some of the mathematical objects he presented in The Fractal Geometry of Nature had been previously described by other mathematicians. Before Mandelbrot, however, they were regarded as isolated curiosities with unnatural and non-intuitive properties. Mandelbrot brought these objects together for the first time and turned them into essential tools for the long-stalled effort to extend the scope of science to explaining non-smooth, "rough" objects in the real world. His methods of research were both old and new:

Mandelbrot emphasized the use of fractals as realistic and useful models for describing many "rough" phenomena in the real world. He concluded that "real roughness is often fractal and can be measured."[8]:296 Although Mandelbrot coined the term "fractal", some of the mathematical objects he presented in The Fractal Geometry of Nature had been previously described by other mathematicians. Before Mandelbrot, however, they were regarded as isolated curiosities with unnatural and non-intuitive properties. Mandelbrot brought these objects together for the first time and turned them into essential tools for the long-stalled effort to extend the scope of science to explaining non-smooth, "rough" objects in the real world. His methods of research were both old and new:

曼德布洛特特地强调可以使用分形作为描述现实世界中多数“粗糙”现象的模型，因其能真实且有效地展现出来。他还总结道：“实际粗糙度通常都是分形的，是可以测量出来的。” 不过，尽管他创造了“分形”一词，但他在《大自然的分形几何学》中提出的一些数学现象之前曾被其他数学家描述过。只是在曼德布洛特之前，这些现象被视为不自然并且非直觉特性的特例存在。是曼德布洛特首次将这些现象或物体放在一起，并将它们变成了必要的工具，通过长期的努力，以科学的范畴去解释现实世界中这些非光滑的“粗糙”物体。


The form of geometry I increasingly favored is the oldest, most concrete, and most inclusive, specifically empowered by the eye and helped by the hand and, today, also by the computer ... bringing an element of unity to the worlds of knowing and feeling ... and, unwittingly, as a bonus, for the purpose of creating beauty.


我越来越喜欢的几何形式是最古老，最具体，最包容的几何形式，特别是由眼睛，由手，甚至由当今计算机提供协助去赋予其力量……为认识和感知世界带来统一的元素……并且，在不经意间，作为创造美的目的，其实这也相当于是额外奖赏。


Fractals are also found in human pursuits, such as music, painting, architecture, and stock market prices. Mandelbrot believed that fractals, far from being unnatural, were in many ways more intuitive and natural than the artificially smooth objects of traditional Euclidean geometry:

Clouds are not spheres, mountains are not cones, coastlines are not circles, and bark is not smooth, nor does lightning travel in a straight line.
  —Mandelbrot, in his introduction to The Fractal Geometry of Nature


分形也同样存在于人类的追求中，例如音乐，绘画，建筑和股票市场价格。曼德布洛特认为，分形超脱于自然，而且在许多方面比传统欧几里得几何形状的人工光滑物体更直观，更自然：

云不是球形，山不是圆锥形，海岸线不是圆形，树皮不是光滑的，闪电也不是直线传播的。

-来自于曼德布洛特的《大自然的分形几何学》介绍


曼德布洛特集合的部分

Mandelbrot has been called an artist, and a visionary[25] and a maverick.[26] His informal and passionate style of writing and his emphasis on visual and geometric intuition (supported by the inclusion of numerous illustrations) made The Fractal Geometry of Nature accessible to non-specialists. The book sparked widespread popular interest in fractals and contributed to chaos theory and other fields of science and mathematics.

Mandelbrot has been called an artist, and a visionary[29] and a maverick.[30] His informal and passionate style of writing and his emphasis on visual and geometric intuition (supported by the inclusion of numerous illustrations) made The Fractal Geometry of Nature accessible to non-specialists. The book sparked widespread popular interest in fractals and contributed to chaos theory and other fields of science and mathematics.

曼德布洛特被称为艺术家，他是一位有远见和特立独行的人。他非正式和热情的写作风格以及对视觉和几何直觉的重视（并辅以大量插图的支持）使非专业人士可以亲身体会《大自然的分形几何学》。这本书引起了大众对分形的广泛兴趣，并为混沌理论以及科学和数学的其他领域做出了贡献。


Mandelbrot also put his ideas to work in cosmology. He offered in 1974 a new explanation of Olbers' paradox (the "dark night sky" riddle), demonstrating the consequences of fractal theory as a sufficient, but not necessary, resolution of the paradox. He postulated that if the stars in the universe were fractally distributed (for example, like Cantor dust), it would not be necessary to rely on the Big Bang theory to explain the paradox. His model would not rule out a Big Bang, but would allow for a dark sky even if the Big Bang had not occurred.[27]


曼德布洛特也将他的想法运用到了宇宙学中。他在1974年对 奥伯斯佯谬Olbers' paradox （“夜空之谜”）提出了新的解释，证明了分形理论的结果是解决悖论的充分非必要条件。他推测，如果宇宙中的恒星是分形分布的（例如像 康托尔尘埃Cantor dust一样），则不必依靠大爆炸理论来解释这一悖论。他的模型不能排除“大爆炸”的发生，但是即使没有发生“大爆炸”，也可以允许黑暗的天空。

Awards and honors 奖项与荣誉

Mandelbrot's awards include the Wolf Prize for Physics in 1993, the Lewis Fry Richardson Prize of the European Geophysical Society in 2000, the Japan Prize in 2003,[28] and the Einstein Lectureship of the American Mathematical Society in 2006.

Mandelbrot's awards include the Wolf Prize for Physics in 1993, the Lewis Fry Richardson Prize of the European Geophysical Society in 2000, the Japan Prize in 2003,[32] and the Einstein Lectureship of the American Mathematical Society in 2006.

曼德布洛特获得了诸多奖项，包括1993年的沃尔夫物理学奖，2000年的欧洲地球物理学会的Lewis Fry Richardson奖，2003年的日本国际奖以及2006年的美国数学学会的爱因斯坦讲师称号。


The small asteroid 27500 Mandelbrot was named in his honor. In November 1990, he was made a Chevalier in France's Legion of Honour. In December 2005, Mandelbrot was appointed to the position of Battelle Fellow at the Pacific Northwest National Laboratory.[29] Mandelbrot was promoted to an Officer of the Legion of Honour in January 2006.[30] An honorary degree from Johns Hopkins University was bestowed on Mandelbrot in the May 2010 commencement exercises.[31]


后来以他的名字命名了小行星27500 曼德布洛特。1990年11月，他被授予法国荣誉军团勋章。2005年12月，他被任命为太平洋西北国家实验室的巴特尔研究所研究员。于2006年1月晋升为荣誉军团军官。在2010年5月，约翰·霍普金斯大学的毕业典礼上授予曼德布洛特荣誉学位。


A partial list of awards received by Mandelbrot:[32]

曼德布洛特获得的部分奖励清单：（建议这些奖项不要翻译成中文比较好）


Death and legacy 死亡与遗产

Mandelbrot died from pancreatic cancer at the age of 85 in a hospice in Cambridge, Massachusetts on 14 October 2010.[36][37] Reacting to news of his death, mathematician Heinz-Otto Peitgen said: "[I]f we talk about impact inside mathematics, and applications in the sciences, he is one of the most important figures of the last fifty years."[36]

Mandelbrot died from pancreatic cancer at the age of 85 in a hospice in Cambridge, Massachusetts on 14 October 2010.[1][40] Reacting to news of his death, mathematician Heinz-Otto Peitgen said: "[I]f we talk about impact inside mathematics, and applications in the sciences, he is one of the most important figures of the last fifty years."

曼德布洛特于2010年10月14日在马萨诸塞州剑桥市的一家救济院死于胰腺癌，享年85岁。数学家海因茨·奥托·皮特根Heinz-Otto Peitgen听闻他去世的消息后说道：“如果我们谈论数学领域的影响以及在科学界的应用，曼德布洛特毫无疑问是过去五十年来最重要的人物之一。”


Chris Anderson, TED conference curator, described Mandelbrot as "an icon who changed how we see the world".[38] Nicolas Sarkozy, President of France at the time of Mandelbrot's death, said Mandelbrot had "a powerful, original mind that never shied away from innovating and shattering preconceived notions [... h]is work, developed entirely outside mainstream research, led to modern information theory."[39] Mandelbrot's obituary in The Economist points out his fame as "celebrity beyond the academy" and lauds him as the "father of fractal geometry".[40]

Chris Anderson, TED conference curator, described Mandelbrot as "an icon who changed how we see the world".[41] Nicolas Sarkozy, President of France at the time of Mandelbrot's death, said Mandelbrot had "a powerful, original mind that never shied away from innovating and shattering preconceived notions [... h]is work, developed entirely outside mainstream research, led to modern information theory."[42] Mandelbrot's obituary in The Economist points out his fame as "celebrity beyond the academy" and lauds him as the "father of fractal geometry".

TED会议策展人克里斯·安德森Chris Anderson将曼德布洛特描述为“改变我们看待世界的标志性人物”。曼德布洛特逝世时法国总统尼古拉斯·萨科齐Nicolas Sarkozy表示，曼德布洛特具有“大胆创新的思想，从不回避改革并打破先入为主的观念……他的工作完全是在主流研究之外发展，从而催生了现代信息论。”在《经济学人》上发表的曼德布洛特讣告指出，他是“超越学院之外的名家”，并称赞他是“分形几何之父”。


Best-selling essayist-author Nassim Nicholas Taleb has remarked that Mandelbrot's book The (Mis)Behavior of Markets is in his opinion "The deepest and most realistic finance book ever published".[6]

Best-selling essayist-author Nassim Nicholas Taleb has remarked that Mandelbrot's book The (Mis)Behavior of Markets is in his opinion "The deepest and most realistic finance book ever published".

畅销的散文作家纳西姆·尼古拉斯·塔勒布Nassim Nicholas Taleb表示，曼德布洛特的《市场行为The（Mis）Behavior of Markets》在他看来是“有史以来最深，最现实的金融书”。

Bibliography 参考书目

in English 英文

  • Fractals: Form, Chance and Dimension, 1977, 2020
  • Fractals and Scaling in Finance: Discontinuity, Concentration, Risk. Selecta Volume E, 1997 by Benoit B. Mandelbrot and R.E. Gomory
  • Fractales, hasard et finance, 1959-1997, 1 November 1998
  • Multifractals and 1/ƒ Noise: Wild Self-Affinity in Physics (1963–1976) (Selecta; V.N) 18 January 1999 by J.M. Berger and Benoit B. Mandelbrot
  • Gaussian Self-Affinity and Fractals: Globality, The Earth, 1/f Noise, and R/S (Selected Works of Benoit B. Mandelbrot) 14 December 2001 by Benoit Mandelbrot and F.J. Damerau
  • Fractals and Chaos: The Mandelbrot Set and Beyond, 9 January 2004
  • The Misbehavior of Markets: A Fractal View of Financial Turbulence, 2006 by Benoit Mandelbrot and Richard L. Hudson
  • The Fractalist: Memoir of a Scientific Maverick, 2014


  • 分形：形式，机会和维度, 1977, 2020
  • 大自然的分形几何学, 1982
  • 金融分形和规模化：间断性，集中性，风险. Selecta Volume E, 1997 by Benoit B. Mandelbrot and R.E. Gomory
  • 分形，机会和财务, 1959-1997, 1 November 1998
  • 多重分形和1 /ƒ噪声：物理学中的自仿射性 (1963–1976) (Selecta; V.N) 18 January 1999 by J.M. Berger and Benoit B. Mandelbrot
  • 高斯自仿射性和分形：全局性，地球，1 / f噪声和R / S (Selected Works of Benoit B. Mandelbrot) 14 December 2001 by Benoit Mandelbrot and F.J. Damerau
  • 分形与混沌：曼德布洛特集及其他, 9 January 2004
  • 市场的不当行为：金融动荡的分形观点, 2006 by Benoit Mandelbrot and Richard L. Hudson
  • 分形主义者:一个科学特立独行者的回忆录, 2014


In French 法语

  • La forme d'une vie. Mémoires (1924-2010) by Benoît Mandelbrot (Author), Johan-Frédérik Hel Guedj (Translator)
  • 生活形式回忆录 (1924–2010) by Benoît Mandelbrot (Author), Johan-Frédérik Hel Guedj (Translator)


References in popular culture 流行文化参考

  • In 1992, author Piers Anthony wrote Fractal Mode where ideas of multiple universes being linked via fractals is a main point of the worldbuilding in the story.
  • 1992年，作家皮尔斯·安东尼Piers Anthony撰写了《分形模式》，其中通过分形将多个宇宙联系在一起的想法是故事中世界建构的重点。


  • In 2004, the American singer-songwriter Jonathan Coulton wrote "Mandelbrot Set". Formerly, it contained the lines "Mandelbrot's in heaven / at least he will be when he's dead / right now he's still alive and teaching math at Yale". Live performances after Mandelbrot's passing in 2010 feature only the first line and a brief rock instrumental.
  • 2004年，美国歌手兼作词人乔纳森·库尔顿Jonathan Coulton创作了《曼德布洛特集》。其歌词包含“曼德布洛特在天堂/至少是他死后的去向/现在他还活着并在耶鲁教数学”这句话。曼德布洛特在2010年过世后，该歌曲的现场表演仅以第一句歌词和简短的摇滚乐器来呈现。


  • In 2007, the author Laura Ruby published "The Chaos King," which includes a character named Mandelbrot and discussion of chaos theory.
  • 2007年，作者劳拉·鲁比Laura Ruby出版了《混沌之王》，其中包括一个名叫曼德尔布洛特的角色以及对混沌理论的讨论。


  • 2017年，扎克·韦纳史密斯Zach Weinersmith的网络漫画《星期六的谷物早餐》描绘了曼德布洛特。


  • In 2017, Liz Ziemska published a novella, Mandelbrot The Magnificent, a fictional account of how Mandelbrot saved his family during WWII.
  • 2017年，莉兹·齐姆斯卡Liz Ziemska出版了中篇小说《曼德尔布洛特的壮丽》（Mandelbrot The Magnificent），这是对曼德布洛特在第二次世界大战期间如何拯救家人的虚构描述。

See also 其他参考资料

  • 1 / f噪声1/f noise –幅度与频率成反比的信号类型
  • 分形维数Fractal dimension –一个比率，表示复杂度随比例变化的统计指标
  • 分数布朗运动Fractional Brownian motion
  • 英国海岸线有多长？– 伯努瓦 曼德布洛特的论文讨论了分形的性质（但内容未使用该术语）
  • 赫斯特指数Hurst exponent – 衡量时间序列的长期依赖性
  • 峰度风险Kurtosis risk – 决策理论术语
  • 间隙度Lacunarity – 几何和分形分析术语
  • 劳伦斯·巴施里耶Louis Bachelier – 法国数学经济学先驱
  • 曼德布洛特竞赛 – 高中数学竞赛
  • 多重分形系统Multifractal system – 具有多重分形维数的系统
  • 自相似性Self-similarity – 对象的整体在数学上与其自身相似
  • 七种随机状态Seven states of randomness – 随机性思想的概括
  • 偏度风险Skewness risk – 财务建模术语
  • Zipf–曼德布洛特定律 – 离散概率分布

Notes 注释

Category:Fellows of the Econometric Society

类别: 经济计量学会研究员

  1. ↑ 引用错误：无效<ref>标签；未给name属性为Mandelbrot's_name的引用提供文字

Category:Fellows of the American Physical Society

类别: 美国物理学会会员


Category:French emigrants to the United States

类别: 移居美国的法国移民

References 参考文献

Category:French scientists

分类: 法国科学家

  1. ↑ Recording of the ceremony on 11 September 2006 at which Mandelbrot received the insignia for an Officer of the Légion d'honneur.
  2. ↑ "Archived copy". Archived from the original on 8 January 2018. Retrieved 8 January 2018.{{cite web}}: CS1 maint: archived copy as title (link)
  3. ↑ Benoit Mandelbrot: Fractals and the art of roughness -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期14 April 2016.. ted.com (February 2010)
  4. ↑ Hudson & Mandelbrot, Prelude, page xviii
  5. ↑ 5.0 5.1 5.2 5.3 5.4 引用错误：无效<ref>标签；未给name属性为Mandelbrot的引用提供文字
  6. ↑ 6.0 6.1 Gomory, R. (2010). "Benoît Mandelbrot (1924–2010)". Nature. 468 (7322): 378. Bibcode:2010Natur.468..378G. doi:10.1038/468378a. PMID 21085164.
  7. ↑ 7.0 7.1 7.2 Wolfram, Stephen. "The Father of Fractals" -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期25 August 2017., Wall Street Journal, 22 November 2012
  8. ↑ list includes specific sciences mentioned in Hudson & Mandelbrot, the Prelude, p. xvi, and p. 26
  9. ↑ Steve Olson (November–December 2004). "The Genius of the Unpredictable". Yale Alumni Magazine. Archived from the original on 22 October 2014. Retrieved 22 July 2014.
  10. ↑ Hoffman, Jascha (16 October 2010). "Benoît Mandelbrot, Novel Mathematician, Dies at 85 (Published 2010)". The New York Times (in English). ISSN 0362-4331. Archived from the original on 21 January 2017. Retrieved 20 November 2020.
  11. ↑ 11.0 11.1 11.2 Mandelbrot, Benoît (2002). "The Wolf Prizes for Physics, A Maverick's Apprenticeship" (PDF). Imperial College Press. Archived (PDF) from the original on 3 December 2013. Retrieved 23 April 2012.
  12. ↑ "BBC News – 'Fractal' mathematician Benoît Mandelbrot dies aged 85". BBC Online. 17 October 2010. Archived from the original on 18 October 2010. Retrieved 17 October 2010.
  13. ↑ Hemenway P. (2005) Divine proportion: Phi in art, nature and science. Psychology Press.
  14. ↑ 引用错误：无效<ref>标签；未给name属性为guardian_obit的引用提供文字
  15. ↑ 15.0 15.1 Barcellos, Anthony (1984). "Mathematical People, Interview of B. B. Mandelbrot" (PDF). Birkhaüser. Archived (PDF) from the original on 27 April 2015. Retrieved 25 June 2013.
  16. ↑ Rama Cont (19 April 2010). "Mandelbrot, Benoit". Wiley. doi:10.1002/9780470061602.eqf01006. ISBN 9780470057568. 
  17. ↑ "New Scientist, 19 April 1997". Newscientist.com. 19 April 1997. Archived from the original on 21 April 2010. Retrieved 17 October 2010.
  18. ↑ The Fractal Geometry of Nature -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期30 November 2015., by Benoît Mandelbrot; W H Freeman & Co, 1982;
  19. ↑ Fractals: Form, Chance and Dimension, by Benoît Mandelbrot; W H Freeman and Co, 1977;
  20. ↑ Ivry, Benjamin. "Benoit Mandelbrot Influenced Art and Mathematics" -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期2 June 2013., Forward, 17 November 2012
  21. ↑ 引用错误：无效<ref>标签；未给name属性为Clarke的引用提供文字
  22. ↑ Mandelbrot, Benoît; Bernard Sapoval; Daniel Zajdenweber (May 1998). "Web of Stories • Benoît Mandelbrot • IBM: background and policies". Web of Stories. Archived from the original on 8 September 2011. Retrieved 17 October 2010.
  23. ↑ Tenner, Edward (16 October 2010). "Benoît Mandelbrot the Maverick, 1924–2010". The Atlantic. Archived from the original on 18 October 2010. Retrieved 16 October 2010.
  24. ↑ Mandelbrot, Benoit B. (5 May 1967). "How long is the coast of Britain? Statistical self-similarity and fractional dimension" (PDF). Science. 156 (3775): 636–638. Bibcode:1967Sci...156..636M. doi:10.1126/science.156.3775.636. PMID 17837158. S2CID 15662830. Archived from the original on 13 July 2015. Retrieved 11 January 2016.
  25. ↑ Devaney, Robert L. (2004). ""Mandelbrot's Vision for Mathematics" in Proceedings of Symposia in Pure Mathematics. Volume 72.1" (PDF). American Mathematical Society. Archived from the original (PDF) on 9 December 2006. Retrieved 5 January 2007.
  26. ↑ Jersey, Bill (24 April 2005). "A Radical Mind". Hunting the Hidden Dimension. NOVA/ PBS. Archived from the original on 22 August 2009. Retrieved 20 August 2009.
  27. ↑ Galaxy Map Hints at Fractal Universe, by Amanda Gefter; New Scientist; 25 June 2008
  28. ↑ Laureates of the Japan Prize -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期17 April 2016.. japanprize.jp
  29. ↑ "PNNL press release: Mandelbrot joins Pacific Northwest National Laboratory". Pnl.gov. 16 February 2006. Archived from the original on 12 January 2009. Retrieved 17 October 2010.
  30. ↑ "Légion d'honneur announcement of promotion of Mandelbrot to officier" (in français). Legifrance.gouv.fr. Archived from the original on 20 November 2020. Retrieved 17 October 2010.
  31. ↑ "Six granted honorary degrees, Society of Scholars inductees recognized". Gazette.jhu.edu. 7 June 2010. Archived from the original on 17 June 2010. Retrieved 17 October 2010.
  32. ↑ Mandelbrot, Benoit B. (2 February 2006). "Vita and Awards (Word document)". Retrieved 6 January 2007. Retrieved from Internet Archive 15 December 2013.
  33. ↑ View/Search Fellows of the ASA -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期16 June 2016., accessed 20 August 2016.
  34. ↑ "APS Fellow Archive". APS. Archived from the original on 20 November 2020. Retrieved 24 September 2020.
  35. ↑ "Gruppe 1: Matematiske fag" (in norsk). Norwegian Academy of Science and Letters. Archived from the original on 10 November 2013. Retrieved 7 October 2010.
  36. ↑ 36.0 36.1 引用错误：无效<ref>标签；未给name属性为nyt_obit的引用提供文字
  37. ↑ "Benoît Mandelbrot, fractals pioneer, dies". United Press International. 16 October 2010. Archived from the original on 25 June 2013. Retrieved 17 October 2010.
  38. ↑ "Mandelbrot, father of fractal geometry, dies". The Gazette. Archived from the original on 19 October 2010. Retrieved 16 October 2010.
  39. ↑ "Sarkozy rend hommage à Mandelbrot" [Sarkozy pays homage to Mandelbrot]. Le Figaro (in français). Archived from the original on 25 June 2013. Retrieved 17 October 2010.
  40. ↑ Benoît Mandelbrot's obituary -{zh-cn:互联网档案馆; zh-tw:網際網路檔案館; zh-hk:互聯網檔案館;}-的存檔，存档日期24 October 2010.. The Economist (21 October 2010)
  41. ↑ "Saturday Morning Breakfast Cereal – Mandelbrot". Archived from the original on 7 October 2017. Retrieved 6 October 2017.

Category:Harvard University people

类别: 哈佛大学的人


Category:IBM employees

类别: IBM 员工

Bibliography 参考书目

  • Hudson, Richard L.; Mandelbrot, Benoît B. (2004). 市场的（错误）行为：风险，破产和回报的分形观点 New York: Basic Books. ISBN 978-0-465-04355-2.


Further reading 拓展阅读

取自“https://wiki.swarma.org/index.php?title=伯努瓦·曼德布洛特_Benoit_Mandelbrot&oldid=22895”