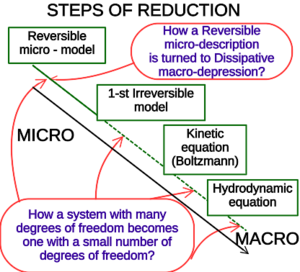
玻尔兹曼方程

此词条由栗子CUGB翻译整理。

The place of the Boltzmann kinetic equation on the stairs of model reduction from microscopic dynamics to macroscopic continuum dynamics (illustration to the content of the book) 玻耳兹曼动力学方程在从微观动力学到宏观连续动力学的模型简化阶梯上的位置(本书内容的说明)

The Boltzmann equation or Boltzmann transport equation (BTE) describes the statistical behaviour of a thermodynamic system not in a state of equilibrium, devised by Ludwig Boltzmann in 1872. The classic example of such a system is a fluid with temperature gradients in space causing heat to flow from hotter regions to colder ones, by the random but biased transport of the particles making up that fluid. In the modern literature the term Boltzmann equation is often used in a more general sense, referring to any kinetic equation that describes the change of a macroscopic quantity in a thermodynamic system, such as energy, charge or particle number.

玻尔兹曼方程或玻尔兹曼输运方程(Boltzmann transport equation, BTE)是描述非平衡状态的热力学系统统计行为的偏微分方程，由路德维希·玻尔兹曼 Ludwig Boltzmann于1872年提出。 这类系统的经典实例是：在空间中具有温度梯度的流体，组成该流体的粒子通过随机但具有偏向性的传输使得热量从较热的区域流向较冷的区域。在现代文献中，玻尔兹曼方程一词通常用于更一般的意义上，指描述热力学系统中宏观量（如能量、电荷或粒子数变化）的任何动力学方程。

The equation arises not by analyzing the individual positions and momenta of each particle in the fluid but rather by considering a probability distribution for the position and momentum of a typical particle—that is, the probability that the particle occupies a given very small region of space (mathematically the volume element [math]\displaystyle{ \mathrm{d}^3 \bf{r} }[/math]) centered at the position [math]\displaystyle{ \bf{r} }[/math], and has momentum nearly equal to a given momentum vector [math]\displaystyle{ \bf{p} }[/math] (thus occupying a very small region of momentum space [math]\displaystyle{ \mathrm{d}^3 \bf{p} }[/math]), at an instant of time.

玻尔兹曼方程并不分析流体中每个粒子的位置和动量，而是考虑特定粒子的位置和动量的概率分布，此类粒子某一时刻在几何空间占据以给定位置[math]\displaystyle{ \bf{r} }[/math]为中心的小邻域（数学上的体积元[math]\displaystyle{ \mathrm{d}^3 \bf{r} }[/math]），且其动量几乎与给定动量矢量[math]\displaystyle{ \bf{p} }[/math]相等，在动量空间占据非常小的区域[math]\displaystyle{ \mathrm{d}^3 \bf{p} }[/math]。

The Boltzmann equation can be used to determine how physical quantities change, such as heat energy and momentum, when a fluid is in transport. One may also derive other properties characteristic to fluids such as viscosity, thermal conductivity, and electrical conductivity (by treating the charge carriers in a material as a gas). See also convection–diffusion equation.

在流体运输过程中，玻尔兹曼方程可以用来确定物理量如何变化，比如热能和动量。人们还可以推导出流体的其他特性，如粘度、热导率和电导率(通过将材料中的载流子当作气体来处理)。 参见对流扩散方程 Convection–Diffusion Equation。

The equation is a nonlinear integro-differential equation, and the unknown function in the equation is a probability density function in six-dimensional space of a particle position and momentum. The problem of existence and uniqueness of solutions is still not fully resolved, but some recent results are quite promising.

玻尔兹曼方程是非线性积分微分方程，方程中的未知函数是位置和动量在六维空间中的概率密度函数。方程解的存在唯一性仍然是未完全解决的问题，但是一些研究显示解决这一问题是很有希望的。

Overview 概述

The phase space and density function 相空间和密度函数

The set of all possible positions r and momenta p is called the phase space of the system; in other words a set of three coordinates for each position coordinate x, y, z, and three more for each momentum component p_x, p_y, p_z. The entire space is 6-dimensional: a point in this space is (r, p) = (x, y, z, p_x, p_y, p_z), and each coordinate is parameterized by time t. The small volume ("differential volume element") is written

系统中所有可能的位置r和动量p的集合称为系统的相空间，集合中位置坐标记为 x，y，z，动量坐标记为p_x，p_y，p_z。整个空间是6维的：空间中一点可以表示为(r, p) = ( x, y, z, p_x, p_y, p_z )，每个坐标由时间 t 参数化。微元(即微分体积元)写作：

[math]\displaystyle{ \text{d}^3\mathbf{r}\,\text{d}^3\mathbf{p} = \text{d}x\,\text{d}y\,\text{d}z\,\text{d}p_x\,\text{d}p_y\,\text{d}p_z. }[/math].

Since the probability of N molecules which all have r and p within [math]\displaystyle{ \mathrm{d}^3\bf{r} }[/math] [math]\displaystyle{ \mathrm{d}^3\bf{p} }[/math] is in question, at the heart of the equation is a quantity f which gives this probability per unit phase-space volume, or probability per unit length cubed per unit momentum cubed, at an instant of time t. This is a probability density function: f (r, p, t), defined so that,

[math]\displaystyle{ \text{d}N = f (\mathbf{r},\mathbf{p},t)\,\text{d}^3\mathbf{r}\,\text{d}^3\mathbf{p} }[/math]

由于在[math]\displaystyle{ \mathrm{d}^3\bf{r} }[/math][math]\displaystyle{ \mathrm{d}^3\bf{p} }[/math]的N个分子都具有的概率都位置r和动量p存在疑问，玻尔兹曼方程的核心是f，它可以给出在某一时刻t单位相空间体积的概率。定义概率密度函数: f (r，p，t) 得到,

[math]\displaystyle{ \text{d}N = f (\mathbf{r},\mathbf{p},t)\,\text{d}^3\mathbf{r}\,\text{d}^3\mathbf{p} }[/math]

is the number of molecules which all have positions lying within a volume element [math]\displaystyle{ d^3\bf{r} }[/math] about r and momenta lying within a momentum space element [math]\displaystyle{ \mathrm{d}^3\bf{p} }[/math] about p, at time t. Integrating over a region of position space and momentum space gives the total number of particles which have positions and momenta in that region:

dN是在t时刻，关于（r，p）的微体积元[math]\displaystyle{ d^3\bf{r} }[/math]和微动量元[math]\displaystyle{ \mathrm{d}^3\bf{p} }[/math]内的分子数目。在位置空间和动量空间的一个区域上积分，得出在该区域中具有位置和动量的粒子总数：

[math]\displaystyle{ \begin{align} N &=\underset{momenta}\int\text{d}^{3}\mathbf{p}\underset{positions}\int\text{d}^{3}\mathbf{r}f(\mathbf{r},\mathbf{p},t)\\[5pt] &=\underset{momenta}\iiint\; \; \; \underset{positions}\iiint f(x,y,z,p_{x},p_{y},p_{z},t)\; \text{d}x\,\text{d}y\,\text{d}z\,\text{d}p_x\,\text{d}p_y\,\text{d}p_z \end{align} }[/math]

which is a 6-fold integral. While f is associated with a number of particles, the phase space is for one-particle (not all of them, which is usually the case with deterministic many-body systems), since only one r and p is in question. It is not part of the analysis to use r₁, p₁ for particle 1, r₂, p₂ for particle 2, etc. up to r_N, p_N for particle N.

这是一个六重积分。虽然f与一群粒子有关，但相空间是针对单一粒子进行讨论(对于所有粒子的分析通常是确定性多体系统 Many-Body的情况)，因为只有一个r和p是需要考虑的。使用r₁, p₁代表粒子1,r₂, p₂代表粒子2，......，直到r_N, p_N代表粒子N，都不在考虑范围之内。

It is assumed the particles in the system are identical (so each has an identical mass m). For a mixture of more than one chemical species, one distribution is needed for each, see below.

系统假设粒子都是相同的（因此每个粒子的质量m相同）。对于组成多于一种化学物质的混合物，其中每种物质都需要一种分布，见下文。

一般形式

The general equation can then be written as

玻尔兹曼方程的一般形式可以写作：

[math]\displaystyle{ \frac{df}{dt} = \left(\frac{\partial f}{\partial t}\right)_\text{force} + \left(\frac{\partial f}{\partial t}\right)_\text{diff} + \left(\frac{\partial f}{\partial t}\right)_\text{coll}, }[/math]

where the "force" term corresponds to the forces exerted on the particles by an external influence (not by the particles themselves), the "diff" term represents the diffusion of particles, and "coll" is the collision term – accounting for the forces acting between particles in collisions. Expressions for each term on the right side are provided below.

Note that some authors use the particle velocity v instead of momentum p; they are related in the definition of momentum by p = mv.

其中“force”一词指外界对粒子施加的力（而不是粒子间的作用），“diff”表示粒子扩散，“coll”表示粒子碰撞，指碰撞中粒子间相互的作用力。上述三项的具体形式将会在下文给出。注意，一些作者会使用 v 表示粒子的速度，而不是动量 p。这两个物理量可以通过动量的定义p = mv联系起来。

The force and diffusion terms “force”项与“diff”项

Consider particles described by [math]\displaystyle{ f }[/math] , each experiencing an external force [math]\displaystyle{ F }[/math] not due to other particles (see the collision term for the latter treatment).

考虑一群以 [math]\displaystyle{ f }[/math] 分布的粒子。每个粒子均受到外力[math]\displaystyle{ \mathbf{F} }[/math]的作用（不包括粒子间作用力。粒子间的作用见后面对“coll”项的处理)。

Suppose at time [math]\displaystyle{ t }[/math] some number of particles all have position [math]\displaystyle{ r }[/math] within element [math]\displaystyle{ d^3\bf{r} }[/math] and momentum [math]\displaystyle{ p }[/math] within [math]\displaystyle{ d^3\bf{p} }[/math]. If a force [math]\displaystyle{ F }[/math] instantly acts on each particle, then at time [math]\displaystyle{ t+\Delta t }[/math] their position will be [math]\displaystyle{ \mathbf{r}+\Delta \mathbf{r}= \textbf{r}+\frac{\textbf{p}}{m}\Delta t }[/math] and momentum [math]\displaystyle{ \mathbf{p}+\Delta \mathbf{p}= \mathbf{p}+\mathbf{F}\Delta t }[/math]. Then, in the absence of collisions, [math]\displaystyle{ f }[/math] must satisfy

假设在时间 [math]\displaystyle{ t }[/math]，一定数量的粒子都有位置 [math]\displaystyle{ \mathbf{r} }[/math]（于微元 [math]\displaystyle{ d^3\mathbf{r} }[/math] 内），和动量 [math]\displaystyle{ \mathbf{p} }[/math]（于微元 [math]\displaystyle{ d^3\mathbf{p} }[/math] 内）。如果此时有一个力[math]\displaystyle{ \mathbf{F} }[/math]在这一瞬作用在每个颗粒上，那么在时间 [math]\displaystyle{ t + \Delta\,t }[/math]，它们的位置将会是[math]\displaystyle{ \mathbf{r} + \Delta\,\mathbf{r} = \mathbf{r} + \mathbf{p} \Delta\,t/m }[/math]，动量将变成 [math]\displaystyle{ \mathbf{p} + \Delta\,\mathbf{p} = \mathbf{p} + \mathbf{F}\Delta\,t }[/math]。在没有碰撞的情况下，[math]\displaystyle{ f }[/math]必须满足

[math]\displaystyle{ f\left ( \textbf{r}+\frac{\textbf{p}}{m}\Delta t,\textbf{p}+\textbf{F}\Delta t,t+\Delta t \right )\, d^{3}\textbf{r}\, d^{3}\textbf{p}= f(\textbf{r},\textbf{p},t)\, d^{3}\textbf{r}\, d^{3}\textbf{p} }[/math]

Note that we have used the fact that the phase space volume element [math]\displaystyle{ d^3\bf{r} }[/math] [math]\displaystyle{ d^3\bf{p} }[/math] is constant, which can be shown using Hamilton's equations (see the discussion under Liouville's theorem). However, since collisions do occur, the particle density in the phase-space volume [math]\displaystyle{ d^3\bf{r} }[/math] '[math]\displaystyle{ d^3\bf{p} }[/math] changes, so

这里，注意到相空间元 [math]\displaystyle{ d^3\mathbf{r}\,d^3\mathbf{p} }[/math] 是恒定的这个事实可以从哈密顿方程 Hamilton's Equations（见刘维尔定理 Liouville's Theorem）得知。然而，由于存在碰撞，相空间元 [math]\displaystyle{ d^3\mathbf{r}\,d^3\mathbf{p} }[/math] 中的粒子密度是可变的，所以有：

[math]\displaystyle{ \begin{align} dN_{coll} &= \left ( \frac{\partial f}{\partial t} \right )_{coll}\Delta td^{3}\textbf{r}\, d^{3}\textbf{p}\\[5pt] & = f\left ( \textbf{r}+\frac{\textbf{p}}{m}\Delta t,\textbf{p}+\textbf{F}\Delta t,t+\Delta t \right )\, d^{3}\textbf{r}\, d^{3}\textbf{p}- f(\textbf{r},\textbf{p},t)\, d^{3}\textbf{r}\, d^{3}\textbf{p}\\[5pt] & =\Delta f d^{3}\textbf{r}\, d^{3}\textbf{p} \end{align} }[/math]

(1)

where Δf is the total change in f. Dividing (1) by [math]\displaystyle{ d^3\bf{r} }[/math] [math]\displaystyle{ d^3\bf{p} }[/math] Δt and taking the limits Δt → 0 and Δf → 0, we have 其中 [math]\displaystyle{ \Delta f }[/math] 指的是[math]\displaystyle{ f }[/math]的总变化量。（1）式除以 [math]\displaystyle{ d^3\mathbf{r}\,d^3\mathbf{p}\,\Delta t }[/math] 并取极限 [math]\displaystyle{ \Delta t\,\rightarrow 0 }[/math] 和 [math]\displaystyle{ \Delta f\,\rightarrow 0 }[/math] 可得：

[math]\displaystyle{ \frac{d f}{d t} = \left(\frac{\partial f}{\partial t} \right)_\mathrm{coll} }[/math]

(2)

The total differential of f is:

<math>f</math>的全微分为：

[math]\displaystyle{ \begin{align} d f & = \frac{\partial f}{\partial t} \, dt +\left(\frac{\partial f}{\partial x} \, dx +\frac{\partial f}{\partial y} \, dy +\frac{\partial f}{\partial z} \, dz \right) +\left(\frac{\partial f}{\partial p_x} \, dp_x +\frac{\partial f}{\partial p_y} \, dp_y +\frac{\partial f}{\partial p_z} \, dp_z \right)\\[5pt] & = \frac{\partial f}{\partial t}dt +\nabla f \cdot d\mathbf{r} + \frac{\partial f}{\partial \mathbf{p}}\cdot d\mathbf{p} \\[5pt] & = \frac{\partial f}{\partial t}dt +\nabla f \cdot \frac{\mathbf{p}}{m}dt + \frac{\partial f}{\partial \mathbf{p}}\cdot \mathbf{F} \, dt \end{align} }[/math]

(3)

where ∇ is the gradient operator, · is the dot product,

其中 ∇ 为梯度算符，· 为点积，

[math]\displaystyle{ \frac{\partial f}{\partial \mathbf{p}} = \mathbf{\hat{e}}_x\frac{\partial f}{\partial p_x} + \mathbf{\hat{e}}_y\frac{\partial f}{\partial p_y} + \mathbf{\hat{e}}_z \frac{\partial f}{\partial p_z}= \nabla_\mathbf{p}f }[/math]

is a shorthand for the momentum analogue of ∇, and ê_x, ê_y, ê_z are Cartesian unit vectors.

是∇的动量类比的一个简写，ê_x, ê_y, ê_z 为笛卡尔坐标系下的单位矢量。

Final statement 最终形式

Dividing (3) by dt and substituting into (2) gives:

对（3）两边同除以dt 并代入（2）可得：

[math]\displaystyle{ \frac{\partial f}{\partial t} + \frac{\mathbf{p}}{m}\cdot\nabla f + \mathbf{F} \cdot \frac{\partial f}{\partial \mathbf{p}} = \left(\frac{\partial f}{\partial t} \right)_\mathrm{coll} }[/math]

In this context, F(r, t) is the force field acting on the particles in the fluid, and m is the mass of the particles. The term on the right hand side is added to describe the effect of collisions between particles; if it is zero then the particles do not collide. The collisionless Boltzmann equation, where individual collisions are replaced with long-range aggregated interactions, e.g. Coulomb interactions, is often called the Vlasov equation.

这里，[math]\displaystyle{ F (\mathbf{r}, t) }[/math] 为流体中作用在粒子上的力场，[math]\displaystyle{ m }[/math]为粒子质量。 右边的一项用于描述粒子间相互碰撞产生的影响；如果此项为零，则说明粒子之间没有碰撞。无碰撞情况下,个体碰撞被长程聚合相互作用(例如库仑相互作用)所取代，此时的玻尔兹曼方程常被称为弗拉索夫方程式 Vlasov Equation。

This equation is more useful than the principal one above, yet still incomplete, since f cannot be solved unless the collision term in f is known. This term cannot be found as easily or generally as the others – it is a statistical term representing the particle collisions, and requires knowledge of the statistics the particles obey, like the Maxwell–Boltzmann, Fermi–Dirac or Bose–Einstein distributions.

这个方程比上一节“主要论述”中的一般形式更加有用。然而这个方程依旧是不完整的：除非已知[math]\displaystyle{ f }[/math]中的碰撞项，否则[math]\displaystyle{ f }[/math]是解不出来的。这一项并不像其他项一样可以简单地或一般地得到——这一项是表示粒子的碰撞的统计项，需要知道粒子遵守怎样的统计规律，例如麦克斯韦-玻尔兹曼分布 Maxwell–Boltzmann Distribution，费米-狄拉克分布 Fermi–Dirac Distribution或玻色–爱因斯坦分布 Bose–Einstein Distribution。

The collision term (Stosszahlansatz) and molecular chaos 碰撞项（Stosszahlansatz）和分子混沌

Two-body collision term 双体碰撞项

A key insight applied by Boltzmann was to determine the collision term resulting solely from two-body collisions between particles that are assumed to be uncorrelated prior to the collision. This assumption was referred to by Boltzmann as the "Stosszahlansatz " and is also known as the "molecular chaos assumption". Under this assumption the collision term can be written as a momentum-space integral over the product of one-particle distribution functions:

玻尔兹曼在确定碰撞项时所应用到的关键见解就是：他假设的碰撞项完全是由假定在碰撞前不相关的两个粒子的相互碰撞得到的。这个假设被波尔兹曼称为“Stosszahlansatz”，也叫做“分子混沌 Molecular Chaos假设”。根据这一假设，碰撞项可以被写作单粒子分布函数的乘积在动量空间上的积分：

[math]\displaystyle{ \left(\frac{\partial f}{\partial t}\right)_\text{coll} = \iint gI(g, \Omega)[f(\mathbf{r},\mathbf{p'}_A, t) f(\mathbf{r},\mathbf{p'}_B,t) - f(\mathbf{r},\mathbf{p}_A,t) f(\mathbf{r},\mathbf{p}_B,t)] \,d\Omega \,d^3\mathbf{p}_A \,d^3\mathbf{p}_B, }[/math]

where p_A and p_B are the momenta of any two particles (labeled as A and B for convenience) before a collision, p′_A and p′_B are the momenta after the collision, 其中 [math]\displaystyle{ \mathbf{p}_A }[/math] 和 [math]\displaystyle{ \mathbf{p}_B }[/math] 表示碰撞前任意两个粒子的动量（为了方便而标记为[math]\displaystyle{ A }[/math]和[math]\displaystyle{ B }[/math]）， [math]\displaystyle{ \mathbf{p}'_A }[/math] 和 [math]\displaystyle{ \mathbf{p}'_B }[/math] 表示碰撞后的动量，

[math]\displaystyle{ g = |\mathbf{p}_B - \mathbf{p}_A| = |\mathbf{p'}_B - \mathbf{p'}_A| }[/math]

is the magnitude of the relative momenta (see relative velocity for more on this concept), and I(g, Ω) is the differential cross section of the collision, in which the relative momenta of the colliding particles turns through an angle θ into the element of the solid angle dΩ, due to the collision.

指对应动量的大小（此概念参考相对速度 Relative Velocity），[math]\displaystyle{ I(g, \Omega) }[/math] 是碰撞的微分散射截面 Differential Cross Section，其中碰撞粒子的相对动量通过一个角θ变为实心角 Solid AngledΩ的元。

Simplifications to the collision term 对碰撞项的简化

Since much of the challenge in solving the Boltzmann equation originates with the complex collision term, attempts have been made to "model" and simplify the collision term. The best known model equation is due to Bhatnagar, Gross and Krook. The assumption in the BGK approximation is that the effect of molecular collisions is to force a non-equilibrium distribution function at a point in physical space back to a Maxwellian equilibrium distribution function and that the rate at which this occurs is proportional to the molecular collision frequency. The Boltzmann equation is therefore modified to the BGK form:

由于求解波尔兹曼方程时，许多挑战都来自于其复杂的碰撞项；因此人们会对碰撞项做一些建模和简化的尝试。现知最好的模型是由Bhatnagar，Gross和Krook作出的BGK近似。BGK近似假设分子碰撞的影响会迫使物理空间中某一点的非平衡分布函数回到麦克斯韦平衡分布函数，且其发生率正比于分子碰撞频率。于是，波尔兹曼方程可写作以下的BGK形式：

[math]\displaystyle{ \frac{\partial f}{\partial t} + \frac{\mathbf{p}}{m}\cdot\nabla f + \mathbf{F} \cdot \frac{\partial f}{\partial \mathbf{p}} = \nu (f_0 - f), }[/math]

where [math]\displaystyle{ \nu }[/math] is the molecular collision frequency, and [math]\displaystyle{ f_0 }[/math] is the local Maxwellian distribution function given the gas temperature at this point in space.

其中 [math]\displaystyle{ \nu }[/math] 是分子碰撞频率，和驰豫时间 [math]\displaystyle{ \tau }[/math] 具有倒数关系：[math]\displaystyle{ \nu = 1/\tau }[/math]。[math]\displaystyle{ f_0 }[/math]是局域麦克斯韦分布函数，由空间中这一点的气体温度给定。

通用方程（对于混合物）

For a mixture of chemical species labelled by indices i = 1, 2, 3, ..., n the equation for species i is

对于具有多种化学组分的混合物，我们以 i =1，2，3，……，n 标记各种成分。则对于组分i的方程是：

[math]\displaystyle{ \frac{\partial f_i}{\partial t} + \frac{\mathbf{p}_i}{m_i} \cdot \nabla f_i + \mathbf{F} \cdot \frac{\partial f_i}{\partial \mathbf{p}_i} = \left(\frac{\partial f_i}{\partial t} \right)_\text{coll}, }[/math]

where f_i = f_i(r, p_i, t), and the collision term is

其中 [math]\displaystyle{ f_i = f_i(\mathbf{r}, \mathbf{p_i}, t) }[/math]。碰撞项为

[math]\displaystyle{ \left(\frac{\partial f_i}{\partial t} \right)_{\mathrm{coll}} = \sum_{j=1}^n \iint g_{ij} I_{ij}(g_{ij}, \Omega)[f'_i f'_j - f_if_j] \,d\Omega\,d^3\mathbf{p'}, }[/math]

where f′ = f′(p′_i, t), the magnitude of the relative momenta is

其中 [math]\displaystyle{ f' = f'(\mathbf{p_i'}, t) }[/math]，相对动量的大小是

[math]\displaystyle{ g_{ij} = |\mathbf{p}_i - \mathbf{p}_j| = |\mathbf{p'}_i - \mathbf{p'}_j|, }[/math]

and I_ij is the differential cross-section, as before, between particles i and j. The integration is over the momentum components in the integrand (which are labelled i and j). The sum of integrals describes the entry and exit of particles of species i in or out of the phase-space element.

I_ij 是粒子i和粒子j之间的微分散射截面。此积分的和描述某一相空间元中，组分i粒子的进出。

应用与推广

Conservation equations 守恒方程

The Boltzmann equation can be used to derive the fluid dynamic conservation laws for mass, charge, momentum, and energy. For a fluid consisting of only one kind of particle, the number density n is given by

玻尔兹曼方程可用于推导流体动力学中的质量守恒，电量守恒，动量守恒，以及能量守恒定律 。对于只含有一种粒子的流体，粒子数密度 [math]\displaystyle{ n }[/math] 为：

[math]\displaystyle{ n = \int f \,d^3p. }[/math]

The average value of any function A is 算符 A 的期望值由下式给出：

[math]\displaystyle{ \langle A \rangle = \frac 1 n \int A f \,d^3p. }[/math]

Since the conservation equations involve tensors, the Einstein summation convention will be used where repeated indices in a product indicate summation over those indices. Thus [math]\displaystyle{ \mathbf{x} \mapsto x_i }[/math] and [math]\displaystyle{ \mathbf{p} \mapsto p_i = m w_i }[/math], where [math]\displaystyle{ w_i }[/math] is the particle velocity vector. Define [math]\displaystyle{ A(p_i) }[/math] as some function of momentum [math]\displaystyle{ p_i }[/math] only, which is conserved in a collision. Assume also that the force [math]\displaystyle{ F_i }[/math] is a function of position only, and that f is zero for [math]\displaystyle{ p_i \to \pm\infty }[/math]. Multiplying the Boltzmann equation by A and integrating over momentum yields four terms, which, using integration by parts, can be expressed as

由于守恒方程中包含张量，以下使用爱因斯坦求和约定 Einstein Summation Convention,乘积中的重复标记表示对这些带有标记量的求和。因此 [math]\displaystyle{ \mathbf{x}\rightarrow x_i }[/math] 且 [math]\displaystyle{ \mathbf{p}\rightarrow p_i = m w_i }[/math]，其中 [math]\displaystyle{ w_i }[/math] 为粒子速度矢量。定义某函数 [math]\displaystyle{ A(p_i) }[/math]，使得其唯一的自变量为动量 [math]\displaystyle{ p_i }[/math]（碰撞中动量守恒）。假设力 [math]\displaystyle{ F_i }[/math] 为位置的函数，且对于 [math]\displaystyle{ p_i\rightarrow\pm \infty }[/math]，[math]\displaystyle{ f }[/math] 为 0。对玻尔兹曼方程两边同乘 [math]\displaystyle{ A }[/math] ，并对动量积分，使用分部积分法可得四项。如下所示：

[math]\displaystyle{ \int A \frac{\partial f}{\partial t} \,d^3p = \frac{\partial }{\partial t} (n \langle A \rangle), }[/math]

[math]\displaystyle{ \int \frac{p_j A}{m}\frac{\partial f}{\partial x_j} \,d^3p = \frac{1}{m}\frac{\partial}{\partial x_j}(n\langle A p_j \rangle), }[/math]

[math]\displaystyle{ \int A F_j \frac{\partial f}{\partial p_j} \,d^3p = -nF_j\left\langle \frac{\partial A}{\partial p_j}\right\rangle, }[/math]

[math]\displaystyle{ \int A \left(\frac{\partial f}{\partial t}\right)_\text{coll} \,d^3p = 0, }[/math]

where the last term is zero, since A is conserved in a collision.

因为 [math]\displaystyle{ A }[/math] 在碰撞中守恒，所以最后一项为零。

Letting [math]\displaystyle{ A = m }[/math], the mass of the particle, the integrated Boltzmann equation becomes the conservation of mass equation:

令 [math]\displaystyle{ A=m }[/math]，即粒子质量，积分后的玻尔兹曼方程化为质量守恒方程：

[math]\displaystyle{ \frac{\partial}{\partial t}\rho + \frac{\partial}{\partial x_j}(\rho V_j) = 0, }[/math]

where [math]\displaystyle{ \rho = mn }[/math] is the mass density, and [math]\displaystyle{ V_i = \langle w_i\rangle }[/math] is the average fluid velocity.

[math]\displaystyle{ \rho=mn }[/math] 为质量密度，[math]\displaystyle{ V_i=\langle w_i\rangle }[/math] 为平均流体速度。

Letting [math]\displaystyle{ A = p_i }[/math], the momentum of the particle, the integrated Boltzmann equation becomes the conservation of momentum equation:

令 [math]\displaystyle{ A = p_i }[/math]，即粒子动量，积分后的玻尔兹曼方程化为动量守恒方程：

[math]\displaystyle{ \frac{\partial}{\partial t}(\rho V_i) + \frac{\partial}{\partial x_j}(\rho V_i V_j+P_{ij}) - nF_i = 0, }[/math]

where [math]\displaystyle{ P_{ij} = \rho \langle (w_i-V_i) (w_j-V_j) \rangle }[/math] is the pressure tensor (the viscous stress tensor plus the hydrostatic pressure).

[math]\displaystyle{ P_{ij}=\rho\langle (w_i-V_i) (w_j-V_j) \rangle }[/math] 为压强张量（粘性应力张量加上流体静力学压强）。

Letting [math]\displaystyle{ A =\frac{p_i p_i}{2m} }[/math], the kinetic energy of the particle, the integrated Boltzmann equation becomes the conservation of energy equation:

令 [math]\displaystyle{ A =\frac{p_i p_i}{2m} }[/math]，即粒子动能，积分后的玻尔兹曼方程化为能量守恒方程：

[math]\displaystyle{ \frac{\partial}{\partial t}(u + \tfrac{1}{2}\rho V_i V_i) + \frac{\partial}{\partial x_j} (uV_j + \tfrac{1}{2}\rho V_i V_i V_j + J_{qj} + P_{ij}V_i) - nF_iV_i = 0, }[/math]

where [math]\displaystyle{ u = \tfrac{1}{2} \rho \langle (w_i-V_i) (w_i-V_i) \rangle }[/math] is the kinetic thermal energy density, and [math]\displaystyle{ J_{qi} = \tfrac{1}{2} \rho \langle(w_i - V_i)(w_k - V_k)(w_k - V_k)\rangle }[/math] is the heat flux vector.

其中[math]\displaystyle{ u=\tfrac{1}{2}\rho\langle (w_i-V_i) (w_i-V_i) \rangle }[/math] 为动力热能密度（kinetic thermal energy density），[math]\displaystyle{ J_{qi}=\tfrac{1}{2}\rho\langle (w_i-V_i)(w_k-V_k)(w_k-V_k)\rangle }[/math] 热通量矢量。

Hamiltonian mechanics 哈密顿力学

In Hamiltonian mechanics, the Boltzmann equation is often written more generally as

在哈密顿力学中, 玻尔兹曼方程通常写作

[math]\displaystyle{ \hat{\mathbf{L}}[f]=\mathbf{C}[f], \, }[/math]

where L is the Liouville operator (there is an inconsistent definition between the Liouville operator as defined here and the one in the article linked) describing the evolution of a phase space volume and C is the collision operator. The non-relativistic form of L is 其中 L 是刘维尔算子(这里定义的刘维尔算子和链接文章中的定义不一致)，它描述了相空间体积的演化；C 是碰撞算子。非相对论下的L 写作

[math]\displaystyle{ \hat{\mathbf{L}}_\mathrm{NR} = \frac{\partial}{\partial t} + \frac{\mathbf{p}}{m} \cdot \nabla + \mathbf{F}\cdot\frac{\partial}{\partial \mathbf{p}}\,. }[/math]

Quantum theory and violation of particle number conservation 量子理论与粒子数守恒的违背

It is possible to write down relativistic quantum Boltzmann equations for relativistic quantum systems in which the number of particles is not conserved in collisions. This has several applications in physical cosmology, including the formation of the light elements in Big Bang nucleosynthesis, the production of dark matter and baryogenesis. It is not a priori clear that the state of a quantum system can be characterized by a classical phase space density f. However, for a wide class of applications a well-defined generalization of f exists which is the solution of an effective Boltzmann equation that can be derived from first principles of quantum field theory.

对于碰撞中粒子数不守恒的相对论量子系统，给出相对论量子玻尔兹曼方程是可能的。这在物理宇宙学中有一些应用，包括大爆炸核合成中轻元素的形成，暗物质的产生和重子发生。我们并不预先知道量子系统的状态可以用经典相空间密度f 来表征。然而，对于许多应用来说，定义良好的f 作为有效玻尔兹曼方程的解是存在的，可以由量子场论 Quantum_Field_Theory的第一原理推导得出。

General relativity and astronomy 广义相对论和天文学

The Boltzmann equation is of use in galactic dynamics. A galaxy, under certain assumptions, may be approximated as a continuous fluid; its mass distribution is then represented by f; in galaxies, physical collisions between the stars are very rare, and the effect of gravitational collisions can be neglected for times far longer than the age of the universe.

玻尔兹曼方程在星系动力学中也大有可为。在一定的假设下，星系可以近似为连续流体；其质量分布可以表示为 f 。在星系中，不同星体间的物理碰撞鲜有发生。在远长于宇宙年龄的时间内，引力碰撞的影响可以被忽略。

Its generalization in general relativity. is

方程在广义相对论中的推广为：

[math]\displaystyle{ \hat{\mathbf{L}}_\mathrm{GR}=p^\alpha\frac{\partial}{\partial x^\alpha} - \Gamma^\alpha{}_{\beta\gamma}p^\beta p^\gamma\frac{\partial}{\partial p^\alpha}, }[/math]

where Γ^α_βγ is the Christoffel symbol of the second kind (this assumes there are no external forces, so that particles move along geodesics in the absence of collisions), with the important subtlety that the density is a function in mixed contravariant-covariant (xⁱ, p_i) phase space as opposed to fully contravariant (xⁱ, pⁱ) phase space.

其中 Γ^α_βγ 代表第二类克里斯托费尔符号（这里假定没有外力，所以粒子在没有碰撞时沿着短程线运动），巧妙地传递出重要的讯息：密度是逆变-协变(xⁱ, p_i)混合相空间内的函数，而不是完全的逆变 (xⁱ, pⁱ)相空间。

In physical cosmology the fully covariant approach has been used to study the cosmic microwave background radiation. More generically the study of processes in the early universe often attempt to take into account the effects of quantum mechanics and general relativity. In the very dense medium formed by the primordial plasma after the Big Bang, particles are continuously created and annihilated. In such an environment quantum coherence and the spatial extension of the wavefunction can affect the dynamics, making it questionable whether the classical phase space distribution f that appears in the Boltzmann equation is suitable to describe the system. In many cases it is, however, possible to derive an effective Boltzmann equation for a generalized distribution function from first principles of quantum field theory. This includes the formation of the light elements in Big Bang nucleosynthesis, the production of dark matter and baryogenesis.

在物理宇宙学中，全协变方法已经应用于宇宙微波背景辐射研究。 更一般地说，对早期宇宙过程的研究常常试图考虑量子力学和广义相对论的影响。 大爆炸后，在非常稠密的原始等离子体介质中，粒子不断地产生和湮灭。在这样的环境中，量子相干性和波函数的空间扩展会影响动力学，使人们怀疑玻尔兹曼方程中出现的经典相空间分布f 是否适合描述这一系统。然而，在许多情况下，可以从量子场论的第一原理推导出广义分布函数的有效玻尔兹曼方程。

方程求解

Exact solutions to the Boltzmann equations have been proven to exist in some cases; this analytical approach provides insight, but is not generally usable in practical problems.

在某些情况下，可以证明玻尔兹曼方程存在精确解。这意味着，如果对服从波尔兹曼方程的系统施加一个微扰，此系统最终将回到平衡状态，而不是发散到无穷，或表现出其他的行为。然而，这种存在性证明无助于我们在现实问题中求解该方程。

Instead, numerical methods (including finite elements and lattice Boltzmann methods) are generally used to find approximate solutions to the various forms of the Boltzmann equation. Example applications range from hypersonic aerodynamics in rarefied gas flows to plasma flows. An application of the Boltzmann equation in electrodynamics is the calculation of the electrical conductivity - the result is in leading order identical with the semiclassical result.

相反，数值方法(包括有限元和格子玻尔兹曼方法)经常用来帮助人们寻找各种形式的玻尔兹曼方程的近似解。应用范围覆盖稀薄气流中的高超音速空气动力学到等离子流。电动力学中，玻尔兹曼方程可以应用于电导率的计算，其结果与半经典结果一致。

Close to local equilibrium, solution of the Boltzmann equation can be represented by an asymptotic expansion in powers of Knudsen number (the Chapman-Enskog expansion). The first two terms of this expansion give the Euler equations and the Navier-Stokes equations. The higher terms have singularities. The problem of developing mathematically the limiting processes, which lead from the atomistic view (represented by Boltzmann's equation) to the laws of motion of continua, is an important part of Hilbert's sixth problem.

在接近局部均衡的情况下，玻尔兹曼方程的解可以用克努森数幂的渐近展开式来表示(Chapman-Enskog 查普曼-恩斯科格展开式)。展开式的前两项给出了欧拉方程和纳维-斯托克斯方程。较高阶项有奇点。从原子观点(以玻尔兹曼方程为代表)到连续体运动定律的极限过程的数学推导问题，是希尔伯特第六问题的重要组成部分。

另见

• Vlasov equation
• H-theorem
• Fokker–Planck equation
• Williams-Boltzmann equation
• Navier–Stokes equations
• Vlasov–Poisson equation
• Lattice Boltzmann methods

参考文献

• Harris, Stewart (1971). An introduction to the theory of the Boltzmann equation. Dover Books. pp. 221. ISBN 978-0-486-43831-3. https://books.google.com/books?id=KfYK1lyq3VYC. . Very inexpensive introduction to the modern framework (starting from a formal deduction from Liouville and the Bogoliubov–Born–Green–Kirkwood–Yvon hierarchy (BBGKY) in which the Boltzmann equation is placed). Most statistical mechanics textbooks like Huang still treat the topic using Boltzmann's original arguments. To derive the equation, these books use a heuristic explanation that does not bring out the range of validity and the characteristic assumptions that distinguish Boltzmann's from other transport equations like Fokker–Planck or Landau equations.

• Arkeryd, Leif (1972). "On the Boltzmann equation part I: Existence". Arch. Rational Mech. Anal. 45 (1): 1–16. Bibcode:1972ArRMA..45....1A. doi:10.1007/BF00253392. S2CID 117877311.
• Arkeryd, Leif (1972). "On the Boltzmann equation part II: The full initial value problem". Arch. Rational Mech. Anal. 45 (1): 17–34. Bibcode:1972ArRMA..45...17A. doi:10.1007/BF00253393. S2CID 119481100.
• Arkeryd, Leif (1972). "On the Boltzmann equation part I: Existence". Arch. Rational Mech. Anal. 45 (1): 1–16. Bibcode:1972ArRMA..45....1A. doi:10.1007/BF00253392. S2CID 117877311.
• DiPerna, R. J.; Lions, P.-L. (1989). "On the Cauchy problem for Boltzmann equations: global existence and weak stability". Ann. of Math. 2. 130 (2): 321–366. doi:10.2307/1971423. JSTOR 1971423.

外部链接

• The Boltzmann Transport Equation by Franz Vesely
• Boltzmann gaseous behaviors solved