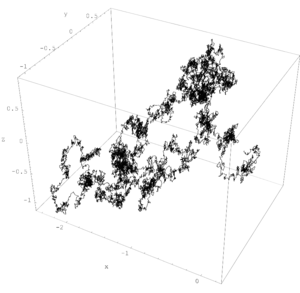
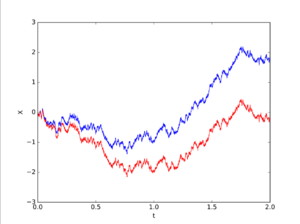
随机过程

来自集智百科 - 复杂系统|人工智能|复杂科学|复杂网络|自组织
Fernando（讨论 | 贡献）2022年1月14日 (五) 21:02的版本 （编辑到维纳过程）
跳到导航 跳到搜索


球体表面维纳或布朗运动过程的计算机模拟实现。维纳过程被广泛认为是概率论中研究最多和中心的随机过程。[1][2][3]


在概率论及相关领域中，随机过程 stochastic process（或random process）是一个数学对象，通常被定义为随机变量的集合。给出对一个随机过程的解释，该过程表示某个系统随机的数值随时间的变化，例如细菌种群的增长，电流由于热噪声而波动，或者一个气体分子的运动。[1][4][5][6]随机过程被广泛用作以随机方式变化的系统和现象的数学模型。它们在许多学科都有应用，比如生物学[7]，化学 [8] 生态学,[9] 神经科学[10], 物理学[11], 图像处理, 信号处理,[12] 控制理论, [13] 信息论,[14] 计算机科学,[15] 密码学[16] 和 电信学.[17] 此外，金融市场中看似随机的变化促进了随机过程在金融领域的广泛应用。[18][19][20]


应用和现象研究反过来又启发了新随机过程的提出。这种随机过程的例子包括维纳过程（Wiener process）或布朗运动过程（Brownian motion process，“布朗运动”可以指物理过程，也被称为“布朗运动”，以及随机过程，一个数学对象，但为了避免歧义，本文使用“布朗运动过程”或“维纳过程”来表示后者，其风格类似于：例如吉赫曼和斯科罗霍德 [21] 或罗森布拉特[22]）使用路易斯·巴切勒来研究巴黎证券交易所的价格变化，[23] 以及爱尔朗使用的泊松过程来研究某段时间内拨出的电话号码。[24]这两个随机过程被认为是随机过程理论中最重要和最核心的，[1][4][25] 并且被巴切勒和爱尔朗先后于不同的环境和国家多次被独立地发现[23][26]。


随机函数 Random function这个术语也用来指随机或随机过程，[27][28] 因为随机过程也可以被解释为函数空间中的随机元素。[29][30]stochastic和random process可以互换使用，通常没有专门的数学空间用于索引随机变量。[29][31]但是，当随机变量被整数或实数的一个区间索引时，通常使用这两个术语。[5][31]如果随机变量被笛卡尔平面或某些高维欧几里得空间索引，那么随机变量的集合通常被称为随机场 random field。[5][32]随机过程的值并不总是数字，也可以是向量或其他数学对象。[5][30]


根据随机过程的数学性质，随机过程可以分为不同的类别，包括随机游走，[33] 鞅（概率论）,[34] 马尔可夫过程,[35] 莱维过程,[36] 高斯过程,[37] 随机场，[38] 更新过程和分支过程[39]。随机过程的研究使用了概率、微积分、线性代数、集合论的数学知识和技术，以及拓扑学[40][41][42]和数学分析的分支，如实分析、测量理论、傅立叶分析和泛函分析。随机过程理论是对数学的重要贡献[43]，不论关于理论还是应用，它都是活跃的研究主题。[44][45][46]

简介

随机过程可以被定义为随机变量的集合，这些随机变量由一些数学集合构成索引，这意味着随机过程中的每个随机变量都与集合中的一个元素唯一关联。[4][5]用于索引随机变量的集合称为“索引集”。从历史上看，索引集是实数的一些子集，例如自然数，为索引集提供了对时间的解释。[1] 集合中的每个随机变量都取值于相同的数学空间中，称为“状态空间（state space）”。例如，这个状态空间可以是整数、实数或维欧几里德空间。[1] 增量是随机过程在两个索引值之间变化的量，通常被解释为两个时间点。[47][48]由于随机性，随机过程可以有许多结果，随机过程的单个结果被称为“抽样函数”或“实现”。[30][49]

单个计算机模拟时间0≤t≤2的三维维纳或布朗运动过程的“样本函数”或“实现”。这个随机过程的指标集是非负数，而其状态空间是三维欧几里德空间

分类

随机过程可以用不同的方法进行分类，例如，根据其状态空间、索引集或随机变量之间的相关性。一种常见的分类方法是通过索引集和状态空间的基数进行分类。[50][51][52]


当解释为时间时，如果随机过程的指标集有有限个或可数个元素，例如有限的一组数、一组整数或自然数，那么随机过程被认为在离散时间域上[53][54] 。如果索引集是实数上的某个区间，则时间被称为连续时间。这两类随机过程分别被称为离散时间随机过程和连续时间随机过程[47][55][56]。离散时间随机过程被认为更容易研究，因为连续时间过程需要更先进的数学技术和知识，特别当索引集不可数时。[57][58] 如果索引集是整数或整数的子集，则随机过程也可以称为随机序列 random sequence。[54]


如果状态空间是整数或自然数，则随机过程称为“离散随机过程”或“整值随机过程”。如果状态空间是实数，则随机过程被称为“实值随机过程”或“具有连续状态空间的过程”。如果状态空间是[math]\displaystyle{ n }[/math]-维欧几里德空间，则随机过程称为[math]\displaystyle{ n }[/math]-“维向量过程”或[math]\displaystyle{ n }[/math]—“向量过程”。[59][51]


词源学

在英语中，“随机”一词最初用作形容词，其定义是“与推测有关”，源于一个希腊语词，意思是“瞄准一个标记，猜测”，而牛津英语词典将1662年作为最早出现的年份。[60]雅各布·伯努利 Jakob Bernoulli在他关于概率“Ars conquectandi”的著作中使用了“Ars conquectandi istice”这个短语，最初于1713年以拉丁文出版，目前在译文中已经被翻译成“猜想或随机的艺术”。[61]这一短语根据伯努利的引用，是由拉迪斯劳斯-博特凯维茨在1917是用德语stochastic表示同样的意思“随机”时使用的。[62]。术语“随机过程”最早出现在1934年约瑟夫-杜布的一篇论文中。[60] 对于这个术语和明确的数学定义，杜布引用了另一篇1934年的论文，其中亚历山大-金钦在德语中使用了术语"stochastischer Prozeß ”，[63][64]尽管德语这个词在早些时候就被使用过，例如，安德烈-科尔莫戈罗夫在1931年就使用过。[65]

根据牛津英语词典的研究，英语中和随机含义相同的这个词的早期出现，可以追溯到16世纪，而早期记录的类似用法开始于14世纪，它是一个名词，意思是“浮躁、极速、力量或暴力(在骑马、奔跑、惊人等等)”。这个单词本身来自中世纪法语单词，意思是“速度，匆忙” ，它可能来源于法语动词，意思是“奔跑”或“疾驰”。随机（random）过程这个术语的第一次书面出现早于随机（stochastic）过程，牛津英语词典也把它作为同义词，并在弗朗西斯·埃奇沃思1888年发表的一篇文章中使用。


根据《牛津英语词典》，英语中“random”（随机）一词的最早出现时间可追溯到16世纪，而早期有记载的用法则始于14世纪，意思是“急躁、速度快、力量大或暴力（骑马、跑步、击打等）”。这个词本身来自法语中间的一个词，意思是“速度，匆忙”，它可能是从法语动词“奔跑”或“飞奔”衍生而来。术语“随机过程”的首次书面出现是在“随机过程”之前出现的，牛津英语词典也将其作为同义词出现，并被Francis Edgeworth于1888年发表的一篇文章中使用。[66]


术语

随机过程的定义是不同的，[67] 但是随机过程传统上被定义为由一些集合索引的随机变量的集族[68][69]。术语“随机（random）过程”和“随机（stochastic）过程”被视为同义词，可以互换使用，而无需精确指定索引集。[29][31][32][70][71][72]。两个“集族”[30][70]，或“族”都在使用着[4][73]，而除了“索引集”的叫法，还会叫做“参数集”[30] 或“参数空间”[32] 。


术语“随机函数”也用于指代随机或随机过程，[5][74][75]尽管有时它只在随机过程取实值时使用。[30][73]当索引集是数学空间而不是实数时，也使用这个术语，[5][76]，而术语“随机过程”和“随机过程”通常在索引集被解释为时间时使用，[5][76][77]其他的叫法，例如当索引集是[math]\displaystyle{ n }[/math]-维欧几里德空间[math]\displaystyle{ \mathbb{R}^n }[/math]或流形时，会称为随机场。[5][30][32]


记号

随机过程可以用[math]\displaystyle{ \{X(t)\}_{t\in T} }[/math]，[55] [math]\displaystyle{ \{X_t\}_{t\in T} }[/math],[69] [math]\displaystyle{ \{X_t\} }[/math][78]或简单地称为[math]\displaystyle{ X }[/math]或[math]\displaystyle{ X（t） }[/math]，尽管[math]\displaystyle{ X（t） }[/math]被视为函数表示法滥用。[79] 例如， [math]\displaystyle{ X(t) }[/math] 或 [math]\displaystyle{ X_t }[/math]表示具有索引[math]\displaystyle{ t }[/math]的随机变量，而不是整个随机过程。[78]如果索引集是[math]\displaystyle{ T=[0,\infty) }[/math]，那么我们可以这样写，例如，[math]\displaystyle{ (X_t , t \geq 0) }[/math]来表示随机过程。[31]


示例

伯努利过程

最简单的随机过程之一是伯努利过程，[80]它是独立同分布随机变量的序列，其中每个随机变量取值1或0，比如概率[math]\displaystyle{ p }[/math]的值为1，概率[math]\displaystyle{ 1-p }[/math]为零。这个过程可以与反复投一枚硬币关联，其中正面的概率为[math]\displaystyle{ p }[/math]，其值为1，而反面的值为零。[81]换句话说，伯努利过程是一系列独立同分布的伯努利随机变量，[82]每一次抛硬币都是伯努利试验的一个例子。[83]


随机游走

随机游走这样一类随机过程，通常定义为欧几里德空间中独立同分布的随机变量或者或随机向量的和，因此它们是在离散时间上变化的过程。[84][85][86][87][88]但是有些人也使用这个术语来指代在连续时间上变化的过程，[89]尤其是金融中使用的维纳过程，这导致了一些误解，从而招来了一些批评。[90]还有其他各种类型的随机游走，它们的状态空间可以是其他数学对象，例如格和群，一般来说，它们都是被充分研究的，在不同的学科中有许多应用。[91][92]


随机游走的一个经典例子被称为“简单随机游动”，它是一个离散时间上的随机过程，以整数为状态空间，基于伯努利过程，其中每个伯努利变量取+1或-1。换言之，简单随机游走发生在整数上，其值要么随概率[math]\displaystyle{ p }[/math]增加1，要么随着概率[math]\displaystyle{ 1-p }[/math]而减小1，因此这种随机游动的指标集是自然数，而其状态空间是整数。如果[math]\displaystyle{ p=0.5 }[/math]，这种随机游动称为对称随机游走。[93][94]


维纳过程

维纳过程是一个具有平稳独立增量并且基于增量大小呈正态分布的随机过程。[2][95]维纳过程是以诺伯特-维纳命名的，他证明了它的数学存在性，但是这个过程也被称为布朗运动过程或布朗运动，因为它和液体中的布朗运动有历史渊源。[96][97][97][98]

实现维纳Wiener过程（或布朗运动过程），具有漂移（蓝色）且不漂移（红色）。

Wiener process维纳过程在概率论中起着中心作用，通常被认为是最重要和研究的随机过程，并与其他随机过程联系在一起[1][2][3][99][100][101][102]其索引集和状态空间分别是非负数和实数，因此它既有连续索引集又有状态空间[103]但是过程可以定义得更广泛，这样它的状态空间可以是维欧几里德空间。[92][100][104]如果任何增量的平均值为零，则所得到的维纳或布朗运动过程称为零漂移。如果任意两个时间点的增量的平均值等于时间差乘以某个常数[math]\displaystyle{ \mu }[/math]，即实数，由此产生的随机过程被称为漂移。[105][106][107]


几乎可以肯定，维纳过程的样本路径处处连续，但无处可微。它可以看作是简单随机游走的一个连续版本。[48][106]当其他随机过程（如某些随机游动重新缩放）的数学极限时，该过程出现，[108][109]这是Donsker定理或不变性原理的主题，也被称为函数中心极限定理。[110][111][112]


维纳过程是一些重要的随机过程家族的成员，包括马尔可夫过程，Lévy过程和高斯过程。[2][48]该过程也有许多应用，是随机微积分中使用的主要随机过程。[113][114]它在数量金融中起着核心作用，[115][116]在Black-Scholes-Merton模型中使用它。[117]该过程也被用于不同的领域，包括大多数自然科学以及社会科学的一些分支，作为各种随机现象的数学模型。[3][118][119]


泊松过程 Poisson process

泊松过程是一个随机过程，有不同的形式和定义。[120][121]它可以定义为一个计数过程，它是一个随机过程，表示某个时间点或事件的随机数量。在从零到某个给定时间区间内的过程点的数目是一个泊松随机变量，它取决于该时间和某个参数。该过程以自然数为状态空间，非负数为索引集。此过程也称为泊松计数过程，因为它可以被解释为计数过程的一个示例。[122]


如果一个泊松过程是用一个正常数定义的，那么这个过程称为齐次泊松过程。[120][123]齐次泊松过程是随机过程的一个重要类，如马尔可夫过程和Lévy过程。[48]


齐次泊松过程可以用不同的方法定义和推广。它的指标集可以定义为实数，这个随机过程也被称为平稳泊松过程[124][125]如果泊松过程的参数常数被某个非负可积函数的[math]\displaystyle{ t }[/math]代替，则得到的过程称为非齐次或非齐次Poisson过程，其中过程点的平均密度不再是常数。[126]作为排队论中的一个基本过程，泊松过程是数学模型的一个重要过程，在这里，它找到了在特定时间窗口中随机发生的事件模型的应用程序。[127][128]


在实数上定义的泊松过程可以解释为一个随机过程，[48][129]等随机变量对象。[130][131]但是它可以定义在[math]\displaystyle{ n }[/math]维欧几里德空间或其他数学空间上，[132]其中它通常被解释为随机集或随机计数度量，而不是随机过程。[130][131]在此设置中，是泊松过程，也称为泊松点过程，是概率论中最重要的研究对象之一，无论是应用还是理论原因。[24][133]但有人指出，Poisson过程并没有得到应有的重视，部分原因是它经常被认为只是在实数上，而不是在其他数学空间中。[133][134]

定义

随机过程 Stochastic process

随机过程被定义为在一个公共概率空间[math]\displaystyle{ (\Omega, \mathcal{F}, P) }[/math]上定义的随机变量集合，其中[math]\displaystyle{ \Omega }[/math] 是样本空间，[math]\displaystyle{ \mathcal{F} }[/math]是一个[math]\displaystyle{ \sigma }[/math]-代数，[math]\displaystyle{ P }[/math]是概率测度；而随机变量，由某个集合[math]\displaystyle{ T }[/math]索引，所有值都取同一个数学空间[math]\displaystyle{ S }[/math]，对于某些[math]\displaystyle{ \sigma }[/math]-代数[math]\displaystyle{ \sigma }[/math][30]


换言之，对于给定的概率空间[math]\displaystyle{ （\Omega，\mathcal{F}，P） }[/math]和可测空间[math]\displaystyle{ （S，Sigma） }[/math]，随机过程是一个值为[math]\displaystyle{ S }[/math]的随机变量的集合，可以写成：[80]

[math]\displaystyle{ \{X(t):t\in T \}. }[/math]


历史上，在许多自然科学问题中，一个点[math]\displaystyle{ t\in T }[/math] 具有时间的意义，因此，[math]\displaystyle{ X(t) }[/math]表示是一个在时间[math]\displaystyle{ t }[/math]的随机变量。[135]随机过程也可以写成[math]\displaystyle{ \{X（t，omega）：t\ in t\} }[/math]来反映它实际上是两个变量的函数，[math]\displaystyle{ t\in t }[/math]和[math]\displaystyle{ \omega\in\omega }[/math][30][136]


还有其他方法可以考虑随机过程，上面的定义被认为是传统的。[68][69]例如，一个随机过程可以解释或定义为一个[math]\displaystyle{ S^T }[/math]值的随机变量，其中[math]\displaystyle{ S^T }[/math]是所有可能的[math]\displaystyle{ S }[/math]-值函数的空间T</math>从集合[math]\displaystyle{ T }[/math]到空间[math]\displaystyle{ S }[/math]。[29][68]


索引集 Index set

集合[math]\displaystyle{ T }[/math]称为“索引集”[4][50]或“参数集”[30][137]。通常，这个集合是实数的一个子集，例如自然数或一个区间，使集合[math]\displaystyle{ T }[/math]能够解释时间。[1]除了这些集合，索引集[math]\displaystyle{ T }[/math]可以是其他线性有序集或更一般的数学集，[1][53]例如笛卡尔平面[math]\displaystyle{ R^2 }[/math]或[math]\displaystyle{ n }[/math]维欧几里得空间，其中t中的元素可以表示空间中的一个点。[47][138]但一般情况下，当索引集有序时，随机过程可以得到更多的结果和定理。[139]


状态空间 State space

随机过程的数学空间[math]\displaystyle{ S }[/math]称为其“状态空间”。这个数学空间可以用整数、实数、[math]\displaystyle{ n }[/math]维欧几里得空间、复杂平面或更抽象的数学空间来定义。状态空间是用反映随机过程可以采用的不同值的元素来定义的进程。[1][5][30][50][55]


样本函数 Sample function

样本函数是随机过程的单个结果，因此，它是由随机过程中每个随机变量的一个可能值构成的。[30][140]更准确地说，如果[math]\displaystyle{ \{X（t，omega）：t\in t\} }[/math]是一个随机过程，那么对于任何点[math]\displaystyle{ \omega\in\omega }[/math]，映射

[math]\displaystyle{ X(\cdot,\omega): T \rightarrow S, }[/math]


称为样本函数，称为“实现”，或者，特别是当[math]\displaystyle{ T }[/math]被解释为时间时，随机过程的“样本路径”[math]\displaystyle{ \{X（T，omega）：T\in T\} }[/math]。[49]这意味着对于一个固定的[math]\displaystyle{ \omega\in\omega }[/math]，存在一个将索引集[math]\displaystyle{ T }[/math]映射到状态空间[math]\displaystyle{ S }[/math][30] 的示例函数的其他名称随机过程包括“轨迹”、“路径函数”[141]或“路径”.[142]


增量 Increment

随机过程的增量是同一随机过程的两个随机变量之间的差值。对于一个指数集可以解释为时间的随机过程，增量是随机过程在某个时间段内的变化量。例如，如果[math]\displaystyle{ \{X（t）：t\in t\} }[/math] 是具有状态空间的随机过程[math]\displaystyle{ S }[/math]且索引集[math]\displaystyle{ T=[0,\infty) }[/math]中的任意两个非负数[math]\displaystyle{ t_1\in [0,\infty) }[/math]和[math]\displaystyle{ t_2\in [0,\infty) }[/math]且[math]\displaystyle{ t_1\leq t_2 }[/math]，差异[math]\displaystyle{ X{tu 2}-X{t_1} }[/math]是一个称为增量的[math]\displaystyle{ S }[/math]值随机变量。[47][48]当对增量感兴趣时，通常状态空间[math]\displaystyle{ S }[/math]是实数或自然数，但它可以是[math]\displaystyle{ n }[/math]维欧几里德空间或更抽象的空间，如巴拿赫空间 Banach spaces。[48]


进一步定义

定律

对于定义在概率空间[math]\displaystyle{ （\Omega，\mathcal{F}，P） }[/math]上的随机过程[math]\displaystyle{ X\colon\Omega\rightarrow S^T }[/math]，随机过程X</math>的定律被定义为前推度量 Pushforward measure：

[math]\displaystyle{ \mu=P\circ X^{-1}, }[/math]


其中[math]\displaystyle{ P }[/math]是一个概率度量，符号[math]\displaystyle{ \circ }[/math]表示函数组合，[math]\displaystyle{ X^{-1} }[/math]是可测量函数的前映像，或者等价地，[math]\displaystyle{ S^T }[/math]值随机变量[math]\displaystyle{ X }[/math]，其中[math]\displaystyle{ S^T }[/math]是[math]\displaystyle{ t\in T }[/math]中所有可能的[math]\displaystyle{ S }[/math]值函数的空间，所以随机过程的规律就是一个概率测度。[29][68][143][144]


对于[math]\displaystyle{ S^T }[/math]的可测子集[math]\displaystyle{ B }[/math]，预图像[math]\displaystyle{ X }[/math]给出

[math]\displaystyle{ X^{-1}(B)=\{\omega\in \Omega: X(\omega)\in B \}, }[/math]


所以a[math]\displaystyle{ X }[/math]定律可以写成：[30]

[math]\displaystyle{ \mu(B)=P(\{\omega\in \Omega: X(\omega)\in B \}). }[/math]


随机过程或随机变量的规律也被称为“概率定律 probability law”，“概率分布 probability distribution”，或“分布”。[135][143][145][146][147]


有限维概率分布 Finite-dimensional probability distributions

对于随机过程[math]\displaystyle{ X }[/math]，其“有限维分布”定义为：

[math]\displaystyle{ \mu_{t_1,\dots,t_n} =P\circ (X({t_1}),\dots, X({t_n}))^{-1}, }[/math]


这项措施[math]\displaystyle{ \mu_{t_1,..,t_n} }[/math]是随机向量的联合分布 [math]\displaystyle{ (X({t_1}),\dots, X({t_n})) }[/math]；它可以被视为法律的“投影”[math]\displaystyle{ \mu }[/math]到一个有限子集[math]\displaystyle{ T }[/math]。[29][148]


对于[math]\displaystyle{ n }[/math]级笛卡尔幂[math]\displaystyle{ S^n=S\times\dots \times S }[/math]的任何可测子集[math]\displaystyle{ C }[/math]，[math]\displaystyle{ X }[/math]的有限维分布可以写成：[30]

[math]\displaystyle{ \mu_{t_1,\dots,t_n}(C) =P \Big(\big\{\omega\in \Omega: \big( X_{t_1}(\omega), \dots, X_{t_n}(\omega) \big) \in C \big\} \Big). }[/math]


随机过程的有限维分布满足两个称为一致性条件的数学条件。[56]


稳定性 Stationarity

“稳定性”是当随机过程的所有随机变量都是相同分布时随机过程所具有的数学性质。换言之，如果[math]\displaystyle{ X }[/math]是一个平稳随机过程，那么对于任何[math]\displaystyle{ t\in T }[/math]，随机变量[math]\displaystyle{ X_t }[/math]具有相同的分布，这意味着对于任何一组[math]\displaystyle{ n }[/math]索引集值[math]\displaystyle{ t_1,\dots, t_n }[/math]而言，对应的[math]\displaystyle{ n }[/math]随机变量

[math]\displaystyle{ X_{t_1}, \dots X_{t_n}, }[/math]


它们都有相同的概率分布。平稳随机过程的指标集通常被解释为时间，因此可以是整数或实数。[149][150] 但对于点过程和随机场也存在平稳性的概念，其中指标集不被解释为时间。[149][151][152]


当指标集[math]\displaystyle{ T }[/math]可以解释为时间时，如果随机过程的有限维分布在时间平移下是不变的，则称其为平稳过程。这种随机过程可以用来描述处于稳态的物理系统，但是仍然会经历随机波动。[149]平稳性背后的直觉是，随着时间的推移，平稳随机过程的分布保持不变。[153]只有当随机变量相同分布时，一系列随机变量才会形成平稳随机过程。[149]


具有上述平稳性定义的随机过程有时被称为严格平稳的，但也有其他形式的平稳性。一个例子是当离散时间或连续时间随机过程[math]\displaystyle{ X }[/math]被称为广义平稳时，那么这个过程[math]\displaystyle{ X }[/math]，有一个有限的第二时刻对于所有[math]\displaystyle{ t\in T }[/math]和两个随机变量的协方差 [math]\displaystyle{ X_t }[/math] 和 [math]\displaystyle{ X_{t+h} }[/math] 只取决于在[math]\displaystyle{ t\in T }[/math]时的数值[math]\displaystyle{ h }[/math][153][154] Khinchin介绍了“广义平稳性”的相关概念，其他名称包括“协方差平稳性”或“广义平稳性”。[154][155]


过滤 Filtration

过滤是定义在某个概率空间中的sigma代数的递增序列和具有某种总阶关系的索引集，例如在索引集是实数的某个子集的情况下。更为正式的是，如果随机过程有一个指数集总排序的随机过程，则如果随机过程有一个指数集的总序为总序，那么在概率空间[math]\displaystyle{ (\Omega, \mathcal{F}, P) }[/math]上的过滤[math]\displaystyle{ \{\mathcal{F}_t\}_{t\in T} }[/math] 是一个sigma代数族，使得[math]\displaystyle{ \mathcal{F}_s \subseteq \mathcal{F}_t \subseteq \mathcal{F} }[/math]对所有[math]\displaystyle{ s \leq t }[/math]，其中[math]\displaystyle{ t, s\in T }[/math]和[math]\displaystyle{ \leq }[/math]表示指标集[math]\displaystyle{ T }[/math]的总阶[50]通过过滤的概念，可以研究[math]\displaystyle{ t\in T }[/math]中随机过程[math]\displaystyle{ X_t }[/math]所包含的信息量，这可以解释为时间[math]\displaystyle{ t }[/math][50][156]过滤背后的直觉是，随着时间的流逝，关于[math]\displaystyle{ t }[/math]的更多信息是已知的或可用的，这些信息可以在[math]\displaystyle{ \mathcal{F}t }[/math]中获得，使[math]\displaystyle{ \Omega }[/math]的分区越来越细。[157][158]


修正 Modification

随机过程的“修正”是另一个随机过程，它与原始随机过程密切相关。更确切地说，一个随机过程[math]\displaystyle{ X }[/math]，与另一个随机过程[math]\displaystyle{ Y }[/math] 具有相同的索引集[math]\displaystyle{ T }[/math]、集空间[math]\displaystyle{ S }[/math]和概率空间[math]\displaystyle{ （\Omega，{\cal F}，P） }[/math]具有相同的索引集[math]\displaystyle{ T }[/math]、集空间[math]\displaystyle{ S }[/math]和概率空间[math]\displaystyle{ (\Omega,{\cal F},P) }[/math]，被称为[math]\displaystyle{ Y }[/math]的修改，如果对所有[math]\displaystyle{ t\in T }[/math]有


[math]\displaystyle{ P(X_t=Y_t)=1 , }[/math]


持有。两个相互修正的随机过程具有相同的有限维法则[159]它们被称为“随机等价”或“等价物”[160]


除了修改，还使用了“版本”一词，[151][161][162][163]然而，当两个随机过程具有相同的有限维分布，但它们可能定义在不同的概率空间上，因此两个过程是相互修改的，在后一种意义上，它们也是彼此的版本，但不是相反。[164][143] .

如果一个连续时间的实值随机过程在其增量上满足一定的矩条件，则Kolmogorov连续性定理指出，该过程存在一个修正，其具有概率为1的连续样本路径，因此随机过程有一个连续的修改或版本[162][163][165]该定理也可以推广到随机域，因此索引集是[math]\displaystyle{ n }[/math]-维欧几里德空间[166]以及以度量空间为状态空间的随机过程。[167]


难以区分 Indistinguishable

两个随机过程[math]\displaystyle{ X }[/math]和[math]\displaystyle{ Y }[/math]定义在同一概率空间[math]\displaystyle{ （\Omega，\mathcal{F}，P） }[/math]上，具有相同的索引集[math]\displaystyle{ T }[/math]和集空间[math]\displaystyle{ S }[/math]上的两个随机过程如果

[math]\displaystyle{ P(X_t=Y_t \text{ for all } t\in T )=1 , }[/math]


成立，则称为“难以区分的”。[143][159]如果两个[math]\displaystyle{ X }[/math]和[math]\displaystyle{ Y }[/math]是相互修改的，几乎肯定是连续的，那么[math]\displaystyle{ X }[/math]和[math]\displaystyle{ Y }[/math]是无法区分的。[168]


可分性 Separability

“可分性”是随机过程的一种性质，它基于与概率测度有关的指标集。假设随机过程或具有不可数指标集的随机场的泛函可以形成随机变量。对于一个随机过程是可分离的，除了其他条件外，它的指标集必须是一个可分离空间，{efn |术语“可分离”在这里出现了两次，有两种不同的含义，第一种含义来自概率，第二种含义来自拓扑和分析。对于一个随机过程是可分的（概率意义上），它的指标集必须是一个可分空间（在拓扑或分析意义上），除了其他条件。[137]}}，这意味着索引集有一个稠密的可数子集。[151][169]


更精确地说，具有概率空间[math]\displaystyle{ (\Omega,{\cal F},P) }[/math]的实值连续时间随机过程[math]\displaystyle{ X }[/math]是可分离的，如果它的指数集[math]\displaystyle{ T }[/math]有一个稠密的可数子集[math]\displaystyle{ \Omega_0 \subset \Omega }[/math]，因此<[math]\displaystyle{ P(\Omega_0)=0 }[/math]，这样对于每个开集[math]\displaystyle{ G\subset T }[/math]和每个闭集[math]\displaystyle{ F\subset \textstyle R =(-\infty,\infty) }[/math]，[math]\displaystyle{ \{ X_t \in F \text{ for all } t \in G\cap U\} }[/math]和[math]\displaystyle{ \{ X_t \in F \text{ for all } t \in G\} }[/math]这两个事件最多在[math]\displaystyle{ \Omega_0 }[/math]的一个子集上不同。[170][171][172]


可分离性的定义（连续时间实值随机过程的可分性定义可以用其他方式表述。[173][174]）也可以为其他索引集和状态空间而声明，[175]例如在随机场的情况下，索引集和状态空间可以是[math]\displaystyle{ n }[/math]维欧几里德空间。[32][151]


随机过程可分性的概念是由Joseph Doob，[169]提出的。可分性的基本思想是使指标集的可数点集决定随机过程的性质，[173]因此离散时间随机过程总是可分离的。[176]Doob的一个定理，有时被称为Doob的可分性定理，表示任何实值连续时间随机过程都有一个可分离的修改。[169][171][177]对于具有索引集和状态空间而不是实数的更一般的随机过程，也存在该定理的版本。[137]


独立性 Independence

两个在相同的概率空间[math]\displaystyle{ (\Omega,\mathcal{F},P) }[/math]上定义，具有相同索引集[math]\displaystyle{ T }[/math]的随机过程[math]\displaystyle{ X }[/math]和[math]\displaystyle{ Y }[/math]被称为“相互独立”，如果对于所有[math]\displaystyle{ n \in \mathbb{N} }[/math]，以及每个特定的[math]\displaystyle{ t_1,\ldots,t_n \in T }[/math]，随机向量[math]\displaystyle{ \left( X(t_1),\ldots,X(t_n) \right) }[/math] 和[math]\displaystyle{ \left( Y(t_1),\ldots,Y(t_n) \right) }[/math]是独立的。[178]


不相关 Uncorrelatedness

两个随机过程[math]\displaystyle{ \left\{X_t\right\} }[/math]和[math]\displaystyle{ \left\{Y_t\right\} }[/math] 称为“不相关的”的，如果它们的互协方差[math]\displaystyle{ \operatorname{K}_{\mathbf{X}\mathbf{Y}}(t_1,t_2) = \operatorname{E} \left[ \left( X(t_1)- \mu_X(t_1) \right) \left( Y(t_2)- \mu_Y(t_2) \right) \right] }[/math]始终为零。[179]最后：

[math]\displaystyle{ \left\{X_t\right\},\left\{Y_t\right\} \text{ uncorrelated} \quad \iff \quad \operatorname{K}_{\mathbf{X}\mathbf{Y}}(t_1,t_2) = 0 \quad \forall t_1,t_2 }[/math].


独立意味着不相关 Independence implies uncorrelatedness

如果两个随机过程[math]\displaystyle{ X }[/math]和[math]\displaystyle{ Y }[/math]是独立的，那么它们也是不相关的


正交性 Orthogonality

如果两个随机过程[math]\displaystyle{ \left\{X_t\right\} }[/math]和[math]\displaystyle{ \left\{Y_t\right\} }[/math]的互相关[math]\displaystyle{ \operatorname{R}_{\mathbf{X}\mathbf{Y}}(t_1,t_2) = \operatorname{E}[X(t_1) \overline{Y(t_2)}] }[/math]一直为0，则称为“正交”，形式为[179]

[math]\displaystyle{ \left\{X_t\right\},\left\{Y_t\right\} \text{ orthogonal} \quad \iff \quad \operatorname{R}_{\mathbf{X}\mathbf{Y}}(t_1,t_2) = 0 \quad \forall t_1,t_2 }[/math].


斯科罗霍德空间 Skorokhod space

skorokod space也写为Skorohod space，是所有右连续左极限的函数的数学空间，定义在实数的某个区间上，例如[math]\displaystyle{ [0,1] }[/math]或[math]\displaystyle{ [0，\infty） }[/math]，取实数或度量空间上的值。[180][181][182]这些函数被称为cádLag或cadlag函数，这是基于法语表达式“continue a droite，limiteégauche”的首字母缩略词，因为这些函数是右连续的，具有左极限。[180][183]由Anatoliy Skorokod引入的Skorokod函数空间，[182]通常用字母[math]\displaystyle{ D }[/math]表示，[180][181][182][183]因此函数空间也被称为空间[math]\displaystyle{ D }[/math][180][184][185]此函数空间的表示法还可以包括定义所有cádlág函数的间隔，因此，例如，[math]\displaystyle{ D[0,1] }[/math]表示在单位间隔[math]\displaystyle{ [0，1] }[/math]。[183][185][186]


在随机过程理论中，由于通常假定连续时间随机过程的样本函数属于一个Skorokod空间，[182][184]因此经常使用Skorokod函数空间，对应于Wiener过程的样本函数。但是空间也有间断函数，这意味着随机过程的样本函数具有跳跃性，例如泊松过程（在实数上），同时也是这一领域的成员。[185][187]


规律性 Regularity

在随机过程的数学构造中，当讨论和假设随机过程的某些条件以解决可能的构造问题时，使用术语“正则性”。[188][189]例如，研究具有不可数索引集的随机过程，假设随机过程服从某种正则条件，例如样本函数是连续的。[190][191]


更多示例

马尔可夫过程与链

马尔可夫过程 Markov processes 是一种随机过程，传统上在离散或连续时间中，具有马尔可夫特性，即马尔可夫过程的下一个值取决于当前值，但它与随机过程的先前值条件无关。换句话说，给定进程的当前状态，进程在未来的行为与它过去的行为是随机独立的。[192][193]


布朗运动过程和泊松过程（一维）都是马尔可夫过程的例子[194]，整数上的随机游走和赌徒破产问题是离散时间中马尔可夫过程的例子。[195][196]


马尔可夫链是一种具有离散状态空间或离散索引集（通常表示时间）的马尔可夫过程，但马尔可夫链的精确定义各不相同[197]例如，通常将马尔可夫链定义为具有可数状态空间的离散或连续时间中的马尔可夫过程（因此不管时间的性质），[198][199][200][201]但通常将马尔可夫链定义为在可数状态空间或连续状态空间中具有离散时间（因此与状态空间无关），[197]有人认为，现在倾向于使用具有离散时间的马尔可夫链的第一个定义，尽管Joseph Doob和Kai Lai Chung等研究人员使用了第二个定义。[202]


马尔可夫过程是一类重要的随机过程，在许多领域有着广泛的应用。[41][203]例如，它们是一种称为Markov chain Monte Carlo的一般随机模拟方法的基础，该方法用于模拟具有特定概率分布的随机对象，并在贝叶斯统计中得到应用。[204][205]


马尔可夫特性的概念最初是针对连续和离散时间的随机过程，但它也适用于其它指标集，如[math]\displaystyle{ n }[/math]维欧氏空间，这导致随机变量的集合被称为马尔可夫随机场。[206][207][208]


鞅 Martingale

鞅 Martingale是一个离散时间或连续时间的随机过程，其性质是在给定过程的当前值和所有过去值的情况下，每个未来值的条件期望值等于当前值。在离散时间中，如果此属性适用于下一个值，则它适用于所有未来值。鞅的精确数学定义需要另外两个条件与过滤的数学概念相结合，这与随时间推移增加可用信息的直觉有关。鞅通常被定义为实值，[209][210][156] but they can also be complex-valued[211]或更一般的。[212]


对称随机游动和Wiener过程（具有零漂移）分别是离散时间和连续时间的鞅的例子。[209][210]对于一个独立且同分布随机变量的序列[math]\displaystyle{ X_1, X_2, X_3, \dots }[/math]且平均值为零，由连续部分和[math]\displaystyle{ X_1,X_1+ X_2, X_1+ X_2+X_3, \dots }[/math] 构成的随机过程是一个离散时间鞅[213]，离散时间鞅推广了独立随机变量的部分和的概念。[214]


通过应用适当的变换，也可以从随机过程中产生鞅'，这就是齐次泊松过程（在实数上）的情形，其结果是一个称为“补偿泊松过程”的鞅。[210]也可以从其他鞅中构建鞅。[213]例如，有基于鞅的鞅Wiener过程，形成连续时间鞅。[209][215]


数学上的鞅形式化了公平博弈的概念，[216]它们最初的开发目的是表明不可能赢得一场公平的比赛。[217]但现在它们被用于许多概率领域，这是研究它们的主要原因之一。[156][217][218]许多概率问题已经通过在问题中找到鞅并加以研究而得到解决。[219]在给定鞅矩的条件下，鞅会收敛，因此经常使用鞅得到收敛结果，这主要是由于鞅收敛定理s。[214][220][221]


鞅在统计学中有许多应用，但有人指出，它的使用和应用并不像它在统计学领域那样广泛，尤其是统计推断。[222]他们在排队论和棕榈微积分等概率论领域找到了应用[223]以及其他领域，如经济学。[224] and finance.[19]


莱维过程 Lévy process

莱维过程是随机过程的一种类型，可以看作是连续时间中随机游动的推广。[48][225]这些过程在金融、流体力学等领域有着广泛的应用。[226][227]这些过程和过程的独立性被称为平稳过程的主要特征。换句话说，一个随机过程[math]\displaystyle{ X }[/math]是一个Lévy过程，如果对非负数[math]\displaystyle{ n }[/math]，[math]\displaystyle{ 0\leq t_1\leq \dots \leq t_n }[/math]，当[math]\displaystyle{ n-1 }[/math]递增

[math]\displaystyle{ X_{t_2}-X_{t_1}, \dots , X_{t_{n-1}}-X_{t_n}, }[/math]


它们彼此独立，每个增量的分布只取决于时间的差异。[48]


随机 Random field

随机场是由一个[math]\displaystyle{ n }[/math]维欧几里德空间或流形索引的随机变量的集合。一般来说，随机场可以看作是随机过程的一个例子，其中，索引集不一定是实行的子集。[32]但是有一个约定，当索引具有两个或多个维度时，随机变量的索引集合称为随机字段。[5][30][228]如果随机过程的具体定义要求索引集是实数的子集，那么随机场可以看作是随机过程的一个推广。[229]


点过程 Point process

点过程是随机分布在某些数学空间（如实数、[math]\displaystyle{ n }[/math]维欧几里德空间或更抽象的空间）上的点的集合。有时“点过程”一词并不可取，因为历史上“过程”一词表示某个系统在时间上的演变，因此，点过程也被称为“随机点域”。[230]>点过程有不同的解释，这样一个随机计数度量或随机集。[231][232]一些作者将点过程和随机过程视为两个不同的对象，因此点过程是随机过程产生或与随机过程相关联的随机对象，[233][234]尽管已经注意到点过程和随机过程之间的区别并不清楚。[234]


另一些作者认为点过程是一个随机过程，其中过程由一组底层空间（在点过程的上下文中，“状态空间”一词可以指定义点过程的空间，如实数，[235][236]其中与随机过程术语中的指标集相对应的指标集。）其上定义它的地方，如实数或[math]\displaystyle{ n }[/math]-维的欧几里得空间。[237][238]其他随机过程，如更新和计数过程，在点过程理论中进行了研究一、基本理论与方法。[239][240]


历史 History

早期概率论 Early probability theory

概率论起源于机会博弈，它有着悠久的历史，有些游戏在几千年前就已经开始了，[241][242]但很少从概率的角度对其进行分析。[241][243]在概率论上有过书面通信时，1654年通常被认为是概率论的诞生，受[[点数问题|赌博问题].[241][244][245]但是早期有关于赌博游戏概率的数学研究，比如Gerolamo Cardano的“Liber de Ludo Aleae”，16世纪写于16世纪，死后于1663年发表。[241][246]


继Cardano之后，雅各布·伯努利 Jakob Bernoulli（也被称为James Bernoulli或Jacques Bernoulli[247]）写了魔术师Ars conjuctandi，在概率论史上被认为是重大事件。[241]伯努利的书出版于1713年，也是在他死后出版的，激发了许多数学家研究概率。[241][248][249] 但尽管一些著名的数学家对概率论做出了贡献，比如[[皮埃尔-西蒙-拉普拉斯]、亚伯拉罕-德-莫伊夫、卡尔-高斯、西蒙-泊阿松 Siméon Poisson和帕夫努蒂·切比雪夫 Pafnuty Chebyshev，[250][251]指导20世纪，大多数数学界人士（一个显著的例外是俄罗斯的圣彼得堡学派，在那里，以切比雪夫为首的数学家研究概率论[252]）才认为概率论是数学的一部分。[250][252][253][254]


统计力学 Statistical mechanics

在物理科学中，科学家们在19世纪发展了统计力学这门学科，在这个学科中，物理系统，例如装满气体的容器，可以从数学上看作或处理为许多运动粒子的集合。尽管有些科学家试图将随机性纳入统计物理学，比如鲁道夫·克劳修斯，大部分工作没有或几乎没有随机性。[255][256]


这种情况在1859年发生了变化，当时詹姆斯·克拉克·麦克斯韦 James Clerk Maxwell对该领域做出了重大贡献，更具体地说，是对气体动力学理论的贡献，通过介绍他的工作，他假设气体粒子以随机速度随机方向移动[257][258]气体动力学理论和统计物理在19世纪后半叶继续发展，主要由克劳修斯，路德维希·玻尔兹曼和约西亚·威拉德·吉布斯完成，这项工作后来对阿尔伯特·爱因斯坦关于布朗运动的数学模型产生了影响。[259]


测度论与概率论 Measure theory and probability theory

1900年在巴黎举行的国际数学家大会上，David Hilbert提出了一份数学问题的清单，其中他的第六个问题要求对涉及公理的物理和概率进行数学处理。[251]大约在20世纪初，数学家发展了测量理论，这是研究数学函数积分的数学分支，其中两位创始人是法国数学家Henri Lebesgue和Émile Borel。1925年，另一位法国数学家Paul Lévy出版了第一本使用测度论思想的概率论书籍。[251]


20世纪20年代，苏联的数学家们对概率论做出了重大贡献，比如Sergei Bernstein，Aleksandr Khinchin，（Khinchin这个名字也用英语写成（或音译成）Khintchine。[63]）和Andrei Kolmogorov。[254] Kolmogorov于1984年发表了基于测量理论的数学基础的首次尝试。概率论的概率论。[260]在20世纪30年代初，Khinchin和Kolmogorov在20世纪30年代初建立了概率研讨会，这些研讨会由研究者参加，如Eugene Slutsky等和Nikolai Smirnov，[261]还有Khinchin给出了第一个随机变量的数学定义，把随机过程作为以实数线索引的一组随机变量。[63][262]（Doob在引用Khinchin时，使用了“机会变量”这个词，它曾经是“随机变量”的替代词。[263]）


现代概率论的诞生 Birth of modern probability theory

1933年，Andrei Kolmogorov在德国出版了一本关于概率论基础的书，名为“概率计算的基本概念”，后来翻译成英文，1950年出版，作为概率论的基础。这本书的出版现在被广泛认为是现代概率论的诞生，当时概率论和随机过程理论成为数学的一部分。[251][254]


在Kolmogorov的书出版后，钦钦和科尔莫戈洛夫以及其他数学家如Joseph Doob、William Feller、Maurice Fréchet、Paul Lévy、Wolfgang Doeblin等对概率论和随机过程进行了进一步的基础性工作，和Harald Cramér。[251][254]


几十年后，Cramér把20世纪30年代称为“数学概率论的英雄时期”。[254]第二次世界大战极大地中断了概率论的发展，例如，Feller从瑞典迁移到美国。[264]


数学家Joseph Doob在随机过程理论方面做了早期的工作，做出了基本贡献，尤其是在鞅理论方面。[265][263]他的书《随机过程》被认为在概率论领域具有很高的影响力。[266]


二战后的随机过程 Stochastic processes after World War II

第二次世界大战后，概率论和随机过程的研究得到了数学家的更多关注，在概率论和数学的许多领域做出了重大贡献，并开创了新的领域统计学[254][267]从20世纪40年代开始，Kiyosi Itô发表了发展随机微积分领域的论文，它包括基于维纳或布朗运动过程的随机积分和随机微分方程。[268]


同样从20世纪40年代开始，随机过程（尤其是鞅）与势理论的数学领域之间建立了联系，Shizuo Kakutani的早期思想和Joseph Doob后来的工作。[267]在1950年代Gilbert Hunt完成了被认为是开创性的进一步工作，把马尔可夫过程和势理论联系起来，这对Lévy过程理论产生了重大影响，并使人们对用Itô开发的方法研究马尔可夫过程产生了更多的兴趣[23][269][270]


1953年，Joseph Doob出版了《随机过程 Stochastic processes》一书，这本书对随机过程理论产生了重大影响，并强调了测度理论在概率论中的重要性。[267]


[266]Doob还主要发展了鞅理论，后来Paul-André Meyer也作出了重大贡献。早期的研究是由Sergei Bernstein、Paul Lévy和Jean Ville进行的，后者采用了随机过程的鞅项。[271][272]鞅理论中的方法已成为解决各种概率问题的常用方法。研究马尔可夫过程的技术和理论发展到鞅上。相反地，从鞅理论中也建立了处理Markov过程的方法。[267]


概率的其他领域也被发展和用于研究随机过程，其中一个主要方法是大偏差理论。[267] 该理论在统计物理等领域有许多应用，其核心思想至少可以追溯到20世纪30年代。20世纪60年代和70年代后期，苏联的亚历山大·温策尔和美利坚合众国的Monroe D.Donsker和Srinivasa Varadhan完成了基础工作，[273]这将使瓦拉丹获得2007年阿贝尔奖。[274]上世纪90年代和2000年代的理论施拉姆–Loewner演化][275]和粗略路径[143]被引入和发展来研究概率论中的随机过程和其他数学对象，分别在2008年和2014年分别授予Wendelin Werner[276]和Martin Hairer菲尔兹奖。[277]


随机过程理论仍然是研究的焦点，每年都有关于随机过程的国际会议。[44][226]


参考文献

  1. ↑ 1.0 1.1 1.2 1.3 1.4 1.5 1.6 1.7 1.8 Joseph L. Doob (1990). Stochastic processes. Wiley. pp. 46, 47. https://books.google.com/books?id=7Bu8jgEACAAJ. 
  2. ↑ 2.0 2.1 2.2 2.3 L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. p. 1. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA1. 
  3. ↑ 3.0 3.1 3.2 J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 29. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  4. ↑ 4.0 4.1 4.2 4.3 4.4 Emanuel Parzen (2015). Stochastic Processes. Courier Dover Publications. pp. 7, 8. ISBN 978-0-486-79688-8. https://books.google.com/books?id=0mB2CQAAQBAJ. 
  5. ↑ 5.00 5.01 5.02 5.03 5.04 5.05 5.06 5.07 5.08 5.09 5.10 Iosif Ilyich Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. p. 1. ISBN 978-0-486-69387-3. https://books.google.com/books?id=q0lo91imeD0C. 
  6. ↑ Gagniuc, Paul A. (2017). Markov Chains: From Theory to Implementation and Experimentation. NJ: John Wiley & Sons. pp. 1–235. ISBN 978-1-119-38755-8. 
  7. ↑ Paul C. Bressloff (2014). Stochastic Processes in Cell Biology. Springer. ISBN 978-3-319-08488-6. https://books.google.com/books?id=SwZYBAAAQBAJ. 
  8. ↑ N.G. Van Kampen (2011). Stochastic Processes in Physics and Chemistry. Elsevier. ISBN 978-0-08-047536-3. https://books.google.com/books?id=N6II-6HlPxEC. 
  9. ↑ Russell Lande; Steinar Engen; Bernt-Erik Sæther (2003). Stochastic Population Dynamics in Ecology and Conservation. Oxford University Press. ISBN 978-0-19-852525-7. https://books.google.com/books?id=6KClauq8OekC. 
  10. ↑ Carlo Laing; Gabriel J Lord (2010). Stochastic Methods in Neuroscience. OUP Oxford. ISBN 978-0-19-923507-0. https://books.google.com/books?id=RaYSDAAAQBAJ. 
  11. ↑ Wolfgang Paul; Jörg Baschnagel (2013). Stochastic Processes: From Physics to Finance. Springer Science & Business Media. ISBN 978-3-319-00327-6. https://books.google.com/books?id=OWANAAAAQBAJ. 
  12. ↑ Edward R. Dougherty (1999). Random processes for image and signal processing. SPIE Optical Engineering Press. ISBN 978-0-8194-2513-3. https://books.google.com/books?id=ePxDAQAAIAAJ. 
  13. ↑ Dimitri P. Bertsekas (1996). Stochastic Optimal Control: The Discrete-Time Case. Athena Scientific]. ISBN 1-886529-03-5. http://www.athenasc.com/socbook.html. 
  14. ↑ Thomas M. Cover; Joy A. Thomas (2012). Elements of Information Theory. John Wiley & Sons. p. 71. ISBN 978-1-118-58577-1. https://books.google.com/books?id=VWq5GG6ycxMC=PT16. 
  15. ↑ Michael Baron (2015). Probability and Statistics for Computer Scientists, Second Edition. CRC Press. p. 131. ISBN 978-1-4987-6060-7. https://books.google.com/books?id=CwQZCwAAQBAJ. 
  16. ↑ Jonathan Katz; Yehuda Lindell (2007). Introduction to Modern Cryptography: Principles and Protocols. CRC Press. p. 26. ISBN 978-1-58488-586-3. https://archive.org/details/Introduction_to_Modern_Cryptography. 
  17. ↑ François Baccelli; Bartlomiej Blaszczyszyn (2009). Stochastic Geometry and Wireless Networks. Now Publishers Inc. ISBN 978-1-60198-264-3. https://books.google.com/books?id=H3ZkTN2pYS4C. 
  18. ↑ J. Michael Steele (2001). Stochastic Calculus and Financial Applications. Springer Science & Business Media. ISBN 978-0-387-95016-7. https://books.google.com/books?id=H06xzeRQgV4C. 
  19. ↑ 19.0 19.1 Marek Musiela; Marek Rutkowski (2006). Martingale Methods in Financial Modelling. Springer Science & Business Media. ISBN 978-3-540-26653-2. https://books.google.com/books?id=iojEts9YAxIC. 
  20. ↑ Steven E. Shreve (2004). Stochastic Calculus for Finance II: Continuous-Time Models. Springer Science & Business Media. ISBN 978-0-387-40101-0. https://books.google.com/books?id=O8kD1NwQBsQC. 
  21. ↑ Iosif Ilyich Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. ISBN 978-0-486-69387-3. https://books.google.com/books?id=yJyLzG7N7r8C. 
  22. ↑ Murray Rosenblatt (1962). Random Processes. Oxford University Press. https://archive.org/details/randomprocesses00rose_0. 
  23. ↑ 23.0 23.1 23.2 Jarrow, Robert; Protter, Philip (2004). "A short history of stochastic integration and mathematical finance: the early years, 1880–1970". A Festschrift for Herman Rubin. Institute of Mathematical Statistics Lecture Notes - Monograph Series. pp. 75–80. doi:10.1214/lnms/1196285381. ISBN 978-0-940600-61-4. ISSN 0749-2170. 
  24. ↑ 24.0 24.1 Stirzaker, David (2000). "Advice to Hedgehogs, or, Constants Can Vary". The Mathematical Gazette. 84 (500): 197–210. doi:10.2307/3621649. ISSN 0025-5572. JSTOR 3621649.
  25. ↑ Donald L. Snyder; Michael I. Miller (2012). Random Point Processes in Time and Space. Springer Science & Business Media. p. 32. ISBN 978-1-4612-3166-0. https://books.google.com/books?id=c_3UBwAAQBAJ. 
  26. ↑ Guttorp, Peter; Thorarinsdottir, Thordis L. (2012). "What Happened to Discrete Chaos, the Quenouille Process, and the Sharp Markov Property? Some History of Stochastic Point Processes". International Statistical Review. 80 (2): 253–268. doi:10.1111/j.1751-5823.2012.00181.x. ISSN 0306-7734.
  27. ↑ Gusak, Dmytro; Kukush, Alexander; Kulik, Alexey; Mishura, Yuliya; Pilipenko, Andrey (2010). Theory of Stochastic Processes: With Applications to Financial Mathematics and Risk Theory. Springer Science & Business Media. p. 21. ISBN 978-0-387-87862-1. https://books.google.com/books?id=8Nzn51YTbX4C. 
  28. ↑ Valeriy Skorokhod (2005). Basic Principles and Applications of Probability Theory. Springer Science & Business Media. p. 42. ISBN 978-3-540-26312-8. https://books.google.com/books?id=dQkYMjRK3fYC. 
  29. ↑ 29.0 29.1 29.2 29.3 29.4 29.5 Olav Kallenberg (2002). Foundations of Modern Probability. Springer Science & Business Media. pp. 24–25. ISBN 978-0-387-95313-7. https://books.google.com/books?id=L6fhXh13OyMC. 
  30. ↑ 30.00 30.01 30.02 30.03 30.04 30.05 30.06 30.07 30.08 30.09 30.10 30.11 30.12 30.13 30.14 30.15 John Lamperti (1977). Stochastic processes: a survey of the mathematical theory. Springer-Verlag. pp. 1–2. ISBN 978-3-540-90275-1. https://books.google.com/books?id=Pd4cvgAACAAJ. 
  31. ↑ 31.0 31.1 31.2 31.3 Loïc Chaumont; Marc Yor (2012). Exercises in Probability: A Guided Tour from Measure Theory to Random Processes, Via Conditioning. Cambridge University Press. p. 175. ISBN 978-1-107-60655-5. https://books.google.com/books?id=1dcqV9mtQloC&pg=PR4. 
  32. ↑ 32.0 32.1 32.2 32.3 32.4 32.5 Robert J. Adler; Jonathan E. Taylor (2009). Random Fields and Geometry. Springer Science & Business Media. pp. 7–8. ISBN 978-0-387-48116-6. https://books.google.com/books?id=R5BGvQ3ejloC. 
  33. ↑ Gregory F. Lawler; Vlada Limic (2010). Random Walk: A Modern Introduction. Cambridge University Press. ISBN 978-1-139-48876-1. https://books.google.com/books?id=UBQdwAZDeOEC. 
  34. ↑ David Williams (1991). Probability with Martingales. Cambridge University Press. ISBN 978-0-521-40605-5. https://books.google.com/books?id=e9saZ0YSi-AC. 
  35. ↑ L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA1. 
  36. ↑ David Applebaum (2004). Lévy Processes and Stochastic Calculus. Cambridge University Press. ISBN 978-0-521-83263-2. https://books.google.com/books?id=q7eDUjdJxIkC. 
  37. ↑ Mikhail Lifshits (2012). Lectures on Gaussian Processes. Springer Science & Business Media. ISBN 978-3-642-24939-6. https://books.google.com/books?id=03m2UxI-UYMC. 
  38. ↑ Robert J. Adler (2010). The Geometry of Random Fields. SIAM. ISBN 978-0-89871-693-1. https://books.google.com/books?id=ryejJmJAj28C&pg=PA1. 
  39. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  40. ↑ Bruce Hajek (2015). Random Processes for Engineers. Cambridge University Press. ISBN 978-1-316-24124-0. https://books.google.com/books?id=Owy0BgAAQBAJ. 
  41. ↑ 41.0 41.1 G. Latouche; V. Ramaswami (1999). Introduction to Matrix Analytic Methods in Stochastic Modeling. SIAM. ISBN 978-0-89871-425-8. https://books.google.com/books?id=Kan2ki8jqzgC. 
  42. ↑ D.J. Daley; David Vere-Jones (2007). An Introduction to the Theory of Point Processes: Volume II: General Theory and Structure. Springer Science & Business Media. ISBN 978-0-387-21337-8. https://books.google.com/books?id=nPENXKw5kwcC. 
  43. ↑ Applebaum, David (2004). "Lévy processes: From probability to finance and quantum groups". Notices of the AMS. 51 (11): 1336–1347.
  44. ↑ 44.0 44.1 Jochen Blath; Peter Imkeller; Sylvie Rœlly (2011). Surveys in Stochastic Processes. European Mathematical Society. ISBN 978-3-03719-072-2. https://books.google.com/books?id=CyK6KAjwdYkC. 
  45. ↑ Michel Talagrand (2014). Upper and Lower Bounds for Stochastic Processes: Modern Methods and Classical Problems. Springer Science & Business Media. pp. 4–. ISBN 978-3-642-54075-2. https://books.google.com/books?id=tfa5BAAAQBAJ&pg=PR4. 
  46. ↑ Paul C. Bressloff (2014). Stochastic Processes in Cell Biology. Springer. pp. vii–ix. ISBN 978-3-319-08488-6. https://books.google.com/books?id=SwZYBAAAQBAJ&pg=PA1. 
  47. ↑ 47.0 47.1 47.2 47.3 Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 27. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  48. ↑ 48.0 48.1 48.2 48.3 48.4 48.5 48.6 48.7 48.8 Applebaum, David (2004). "Lévy processes: From probability to finance and quantum groups". Notices of the AMS. 51 (11): 1337.
  49. ↑ 49.0 49.1 L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. pp. 121–124. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA1. 
  50. ↑ 50.0 50.1 50.2 50.3 50.4 Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. pp. 294, 295. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  51. ↑ 51.0 51.1 Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 26. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  52. ↑ Donald L. Snyder; Michael I. Miller (2012). Random Point Processes in Time and Space. Springer Science & Business Media. pp. 24, 25. ISBN 978-1-4612-3166-0. https://books.google.com/books?id=c_3UBwAAQBAJ. 
  53. ↑ 53.0 53.1 Patrick Billingsley (2008). Probability and Measure. Wiley India Pvt. Limited. p. 482. ISBN 978-81-265-1771-8. https://books.google.com/books?id=QyXqOXyxEeIC. 
  54. ↑ 54.0 54.1 Alexander A. Borovkov (2013). Probability Theory. Springer Science & Business Media. p. 527. ISBN 978-1-4471-5201-9. https://books.google.com/books?id=hRk_AAAAQBAJ. 
  55. ↑ 55.0 55.1 55.2 Pierre Brémaud (2014). Fourier Analysis and Stochastic Processes. Springer. p. 120. ISBN 978-3-319-09590-5. https://books.google.com/books?id=dP2JBAAAQBAJ&pg=PA1. 
  56. ↑ 56.0 56.1 Jeffrey S Rosenthal (2006). A First Look at Rigorous Probability Theory. World Scientific Publishing Co Inc. pp. 177–178. ISBN 978-981-310-165-4. https://books.google.com/books?id=am1IDQAAQBAJ. 
  57. ↑ Peter E. Kloeden; Eckhard Platen (2013). Numerical Solution of Stochastic Differential Equations. Springer Science & Business Media. p. 63. ISBN 978-3-662-12616-5. https://books.google.com/books?id=r9r6CAAAQBAJ=PA1. 
  58. ↑ Davar Khoshnevisan (2006). Multiparameter Processes: An Introduction to Random Fields. Springer Science & Business Media. pp. 153–155. ISBN 978-0-387-21631-7. https://books.google.com/books?id=XADpBwAAQBAJ. 
  59. ↑ Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. pp. 294, 295. ISBN 978-1-118-59320-2.
  60. ↑ 60.0 60.1 {Cite OED | random}
  61. ↑ O. B. Sheĭnin (2006). Theory of probability and statistics as exemplified in short dictums. NG Verlag. p. 5. ISBN 978-3-938417-40-9. https://books.google.com/books?id=XqMZAQAAIAAJ. 
  62. ↑ Oscar Sheynin; Heinrich Strecker (2011). Alexandr A. Chuprov: Life, Work, Correspondence. V&R unipress GmbH. p. 136. ISBN 978-3-89971-812-6. https://books.google.com/books?id=1EJZqFIGxBIC&pg=PA9. 
  63. ↑ 63.0 63.1 63.2 Doob, Joseph (1934). "Stochastic Processes and Statistics". Proceedings of the National Academy of Sciences of the United States of America. 20 (6): 376–379. Bibcode:1934PNAS...20..376D. doi:10.1073/pnas.20.6.376. PMC 1076423. PMID 16587907.
  64. ↑ Khintchine, A. (1934). "Korrelationstheorie der stationeren stochastischen Prozesse". Mathematische Annalen. 109 (1): 604–615. doi:10.1007/BF01449156. ISSN 0025-5831.
  65. ↑ Kolmogoroff, A. (1931). "Über die analytischen Methoden in der Wahrscheinlichkeitsrechnung". Mathematische Annalen. 104 (1): 1. doi:10.1007/BF01457949. ISSN 0025-5831.
  66. ↑ {Cite OED | random}
  67. ↑ Bert E. Fristedt; Lawrence F. Gray (2013). A Modern Approach to Probability Theory. Springer Science & Business Media. p. 580. ISBN 978-1-4899-2837-5. https://books.google.com/books?id=9xT3BwAAQBAJ&pg=PA716. 
  68. ↑ 68.0 68.1 68.2 68.3 L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. pp. 121, 122. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA1. 
  69. ↑ 69.0 69.1 69.2 Søren Asmussen (2003). Applied Probability and Queues. Springer Science & Business Media. p. 408. ISBN 978-0-387-00211-8. https://books.google.com/books?id=BeYaTxesKy0C. 
  70. ↑ 70.0 70.1 David Stirzaker (2005). Stochastic Processes and Models. Oxford University Press. p. 45. ISBN 978-0-19-856814-8. https://books.google.com/books?id=0avUelS7e7cC. 
  71. ↑ Murray Rosenblatt (1962). Random Processes. Oxford University Press. p. 91. https://archive.org/details/randomprocesses00rose_0. 
  72. ↑ John A. Gubner (2006). Probability and Random Processes for Electrical and Computer Engineers. Cambridge University Press. p. 383. ISBN 978-1-139-45717-0. https://books.google.com/books?id=pa20eZJe4LIC. 
  73. ↑ 73.0 73.1 Kiyosi Itō (2006). Essentials of Stochastic Processes. American Mathematical Soc.. p. 13. ISBN 978-0-8218-3898-3. https://books.google.com/books?id=pY5_DkvI-CcC&pg=PR4. 
  74. ↑ M. Loève (1978). Probability Theory II. Springer Science & Business Media. p. 163. ISBN 978-0-387-90262-3. https://books.google.com/books?id=1y229yBbULIC. 
  75. ↑ Pierre Brémaud (2014). Fourier Analysis and Stochastic Processes. Springer. p. 133. ISBN 978-3-319-09590-5. https://books.google.com/books?id=dP2JBAAAQBAJ&pg=PA1. 
  76. ↑ 76.0 76.1 p. 1
  77. ↑ Richard F. Bass (2011). Stochastic Processes. Cambridge University Press. p. 1. ISBN 978-1-139-50147-7. https://books.google.com/books?id=Ll0T7PIkcKMC. 
  78. ↑ 78.0 78.1 ,John Lamperti (1977). Stochastic processes: a survey of the mathematical theory. Springer-Verlag. p. 3. ISBN 978-3-540-90275-1. https://books.google.com/books?id=Pd4cvgAACAAJ. 
  79. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 55. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  80. ↑ 80.0 80.1 Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. p. 293. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  81. ↑ Florescu, Ionut (2014). Probability and Stochastic Processes. John Wiley & Sons. p. 301. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  82. ↑ Dimitri P. Bertsekas; John N. Tsitsiklis (2002). Introduction to Probability. Athena Scientific. p. 273. ISBN 978-1-886529-40-3. https://books.google.com/books?id=bcHaAAAAMAAJ. 
  83. ↑ Oliver C. Ibe (2013). Elements of Random Walk and Diffusion Processes. John Wiley & Sons. p. 11. ISBN 978-1-118-61793-9. https://books.google.com/books?id=DUqaAAAAQBAJ&pg=PT10. 
  84. ↑ Achim Klenke (2013). Probability Theory: A Comprehensive Course. Springer. pp. 347. ISBN 978-1-4471-5362-7. https://books.google.com/books?id=aqURswEACAAJ. 
  85. ↑ Gregory F. Lawler; Vlada Limic (2010). Random Walk: A Modern Introduction. Cambridge University Press. p. 1. ISBN 978-1-139-48876-1. https://books.google.com/books?id=UBQdwAZDeOEC. 
  86. ↑ Olav Kallenberg (2002). Foundations of Modern Probability. Springer Science & Business Media. p. 136. ISBN 978-0-387-95313-7. https://books.google.com/books?id=L6fhXh13OyMC. 
  87. ↑ Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. p. 383. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  88. ↑ Rick Durrett (2010). Probability: Theory and Examples. Cambridge University Press. p. 277. ISBN 978-1-139-49113-6. https://books.google.com/books?id=evbGTPhuvSoC. 
  89. ↑ {cite book | last1=Weiss | first1=George H.| title=Statistical Sciences | chapter=Random Walks | year=2006 | doi=10.1002/0471667196.ess2180.pub2 | page=1 | isbn=978-0471667193}}
  90. ↑ Aris Spanos (1999). Probability Theory and Statistical Inference: Econometric Modeling with Observational Data. Cambridge University Press. p. 454. ISBN 978-0-521-42408-0. https://books.google.com/books?id=G0_HxBubGAwC. 
  91. ↑ Weiss, George H. (2006). "Random Walks". Encyclopedia of Statistical Sciences. p. 1. doi:10.1002/0471667196.ess2180.pub2. ISBN 978-0471667193.
  92. ↑ 92.0 92.1 Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 81. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  93. ↑ Allan Gut (2012). Probability: A Graduate Course. Springer Science & Business Media. p. 88. ISBN 978-1-4614-4708-5. https://books.google.com/books?id=XDFA-n_M5hMC. 
  94. ↑ Geoffrey Grimmett; David Stirzaker (2001). Probability and Random Processes. OUP Oxford. p. 71. ISBN 978-0-19-857222-0. https://books.google.com/books?id=G3ig-0M4wSIC. 
  95. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 56. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  96. ↑ Brush, Stephen G. (1968). "A history of random processes". Archive for History of Exact Sciences. 5 (1): 1–2. doi:10.1007/BF00328110. ISSN 0003-9519.
  97. ↑ 97.0 97.1 Applebaum, David (2004). "Lévy processes: From probability to finance and quantum groups". Notices of the AMS. 51 (11): 1338.
  98. ↑ Iosif Ilyich Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. p. 21. ISBN 978-0-486-69387-3. https://books.google.com/books?id=yJyLzG7N7r8C&pg=PR2. 
  99. ↑ Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. p. 471. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  100. ↑ 100.0 100.1 Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. pp. 21, 22. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  101. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. VIII. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  102. ↑ Daniel Revuz; Marc Yor (2013). Continuous Martingales and Brownian Motion. Springer Science & Business Media. p. IX. ISBN 978-3-662-06400-9. https://books.google.com/books?id=OYbnCAAAQBAJ. 
  103. ↑ Jeffrey S Rosenthal (2006). A First Look at Rigorous Probability Theory. World Scientific Publishing Co Inc. p. 186. ISBN 978-981-310-165-4. https://books.google.com/books?id=am1IDQAAQBAJ. 
  104. ↑ Donald L. Snyder; Michael I. Miller (2012). Random Point Processes in Time and Space. Springer Science & Business Media. p. 33. ISBN 978-1-4612-3166-0. https://books.google.com/books?id=c_3UBwAAQBAJ. 
  105. ↑ J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 118. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  106. ↑ 106.0 106.1 Peter Mörters; Yuval Peres (2010). Brownian Motion. Cambridge University Press. pp. 1, 3. ISBN 978-1-139-48657-6. https://books.google.com/books?id=e-TbA-dSrzYC. 
  107. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. 78. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  108. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. 61. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  109. ↑ Steven E. Shreve (2004). Stochastic Calculus for Finance II: Continuous-Time Models. Springer Science & Business Media. p. 93. ISBN 978-0-387-40101-0. https://books.google.com/books?id=O8kD1NwQBsQC. 
  110. ↑ Olav Kallenberg (2002). Foundations of Modern Probability. Springer Science & Business Media. pp. 225, 260. ISBN 978-0-387-95313-7. https://books.google.com/books?id=L6fhXh13OyMC. 
  111. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. 70. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  112. ↑ Peter Mörters; Yuval Peres (2010). Brownian Motion. Cambridge University Press. p. 131. ISBN 978-1-139-48657-6. https://books.google.com/books?id=e-TbA-dSrzYC. 
  113. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  114. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  115. ↑ Applebaum, David (2004). "Lévy processes: From probability to finance and quantum groups". Notices of the AMS. 51 (11): 1341.
  116. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 340. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  117. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 124. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  118. ↑ Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. 47. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  119. ↑ Ubbo F. Wiersema (2008). Brownian Motion Calculus. John Wiley & Sons. p. 2. ISBN 978-0-470-02171-2. https://books.google.com/books?id=0h-n0WWuD9cC. 
  120. ↑ 120.0 120.1 Henk C. Tijms (2003). A First Course in Stochastic Models. Wiley. pp. 1, 2. ISBN 978-0-471-49881-0. https://books.google.com/books?id=eBeNngEACAAJ. 
  121. ↑ D.J. Daley; D. Vere-Jones (2006). An Introduction to the Theory of Point Processes: Volume I: Elementary Theory and Methods. Springer Science & Business Media. pp. 19–36. ISBN 978-0-387-21564-8. https://books.google.com/books?id=6Sv4BwAAQBAJ. 
  122. ↑ Henk C. Tijms (2003). A First Course in Stochastic Models. Wiley. pp. 1, 2. ISBN 978-0-471-49881-0.
  123. ↑ Mark A. Pinsky; Samuel Karlin (2011). An Introduction to Stochastic Modeling. Academic Press. p. 241. ISBN 978-0-12-381416-6. https://books.google.com/books?id=PqUmjp7k1kEC. 
  124. ↑ J. F. C. Kingman (1992). Poisson Processes. Clarendon Press. p. 38. ISBN 978-0-19-159124-2. https://books.google.com/books?id=VEiM-OtwDHkC. 
  125. ↑ D.J. Daley; D. Vere-Jones (2006). An Introduction to the Theory of Point Processes: Volume I: Elementary Theory and Methods. Springer Science & Business Media. p. 19. ISBN 978-0-387-21564-8. https://books.google.com/books?id=6Sv4BwAAQBAJ. 
  126. ↑ J. F. C. Kingman (1992). Poisson Processes. Clarendon Press. p. 22. ISBN 978-0-19-159124-2. https://books.google.com/books?id=VEiM-OtwDHkC. 
  127. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. pp. 118, 119. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  128. ↑ Leonard Kleinrock (1976). Queueing Systems: Theory. Wiley. p. 61. ISBN 978-0-471-49110-1. https://archive.org/details/queueingsystems00klei. 
  129. ↑ Murray Rosenblatt (1962). Random Processes. Oxford University Press. p. 94. https://archive.org/details/randomprocesses00rose_0. 
  130. ↑ 130.0 130.1 Martin Haenggi (2013). Stochastic Geometry for Wireless Networks. Cambridge University Press. pp. 10, 18. ISBN 978-1-107-01469-5. https://books.google.com/books?id=CLtDhblwWEgC. 
  131. ↑ 131.0 131.1 Sung Nok Chiu; Dietrich Stoyan; Wilfrid S. Kendall; Joseph Mecke (2013). Stochastic Geometry and Its Applications. John Wiley & Sons. pp. 41, 108. ISBN 978-1-118-65825-3. https://books.google.com/books?id=825NfM6Nc-EC. 
  132. ↑ J. F. C. Kingman (1992). Poisson Processes. Clarendon Press. p. 11. ISBN 978-0-19-159124-2. https://books.google.com/books?id=VEiM-OtwDHkC. 
  133. ↑ 133.0 133.1 Roy L. Streit (2010). Poisson Point Processes: Imaging, Tracking, and Sensing. Springer Science & Business Media. p. 1. ISBN 978-1-4419-6923-1. https://books.google.com/books?id=KAWmFYUJ5zsC&pg=PA11. 
  134. ↑ J. F. C. Kingman (1992). Poisson Processes. Clarendon Press. p. v. ISBN 978-0-19-159124-2. https://books.google.com/books?id=VEiM-OtwDHkC. 
  135. ↑ 135.0 135.1 Alexander A. Borovkov (2013). Probability Theory. Springer Science & Business Media. p. 528. ISBN 978-1-4471-5201-9. https://books.google.com/books?id=hRk_AAAAQBAJ&pg. 
  136. ↑ Georg Lindgren; Holger Rootzen; Maria Sandsten (2013). Stationary Stochastic Processes for Scientists and Engineers. CRC Press. pp. 11. ISBN 978-1-4665-8618-5. https://books.google.com/books?id=FYJFAQAAQBAJ&pg=PR1. 
  137. ↑ 137.0 137.1 137.2 Valeriy Skorokhod (2005). Basic Principles and Applications of Probability Theory. Springer Science & Business Media. pp. 93, 94. ISBN 978-3-540-26312-8. https://books.google.com/books?id=dQkYMjRK3fYC. 
  138. ↑ Donald L. Snyder; Michael I. Miller (2012). Random Point Processes in Time and Space. Springer Science & Business Media. p. 25. ISBN 978-1-4612-3166-0. https://books.google.com/books?id=c_3UBwAAQBAJ. 
  139. ↑ Valeriy Skorokhod (2005). Basic Principles and Applications of Probability Theory. Springer Science & Business Media. p. 104. ISBN 978-3-540-26312-8. https://books.google.com/books?id=dQkYMjRK3fYC. 
  140. ↑ Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. p. 296. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  141. ↑ Patrick Billingsley (2008). Probability and Measure. Wiley India Pvt. Limited. p. 493. ISBN 978-81-265-1771-8. https://books.google.com/books?id=QyXqOXyxEeIC. 
  142. ↑ Bernt Øksendal (2003). Stochastic Differential Equations: An Introduction with Applications. Springer Science & Business Media. p. 10. ISBN 978-3-540-04758-2. https://books.google.com/books?id=VgQDWyihxKYC. 
  143. ↑ 143.0 143.1 143.2 143.3 143.4 Peter K. Friz; Nicolas B. Victoir (2010). Multidimensional Stochastic Processes as Rough Paths: Theory and Applications. Cambridge University Press. p. 571. ISBN 978-1-139-48721-4. https://books.google.com/books?id=CVgwLatxfGsC. 
  144. ↑ Sidney I. Resnick (2013). Adventures in Stochastic Processes. Springer Science & Business Media. pp. 40–41. ISBN 978-1-4612-0387-2. https://books.google.com/books?id=VQrpBwAAQBAJ. 
  145. ↑ Ward Whitt (2006). Stochastic-Process Limits: An Introduction to Stochastic-Process Limits and Their Application to Queues. Springer Science & Business Media. p. 23. ISBN 978-0-387-21748-2. https://books.google.com/books?id=LkQOBwAAQBAJ&pg=PR5. 
  146. ↑ David Applebaum (2004). Lévy Processes and Stochastic Calculus. Cambridge University Press. p. 4. ISBN 978-0-521-83263-2. https://books.google.com/books?id=q7eDUjdJxIkC. 
  147. ↑ Daniel Revuz; Marc Yor (2013). Continuous Martingales and Brownian Motion. Springer Science & Business Media. p. 10. ISBN 978-3-662-06400-9. https://books.google.com/books?id=OYbnCAAAQBAJ. 
  148. ↑ L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. pp. 123. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA356. 
  149. ↑ 149.0 149.1 149.2 149.3 John Lamperti (1977). Stochastic processes: a survey of the mathematical theory. Springer-Verlag. pp. 6 and 7. ISBN 978-3-540-90275-1. https://books.google.com/books?id=Pd4cvgAACAAJ. 
  150. ↑ Iosif I. Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. p. 4. ISBN 978-0-486-69387-3. https://books.google.com/books?id=yJyLzG7N7r8C&pg=PR2. 
  151. ↑ 151.0 151.1 151.2 151.3 Robert J. Adler (2010). The Geometry of Random Fields. SIAM. pp. 14, 15. ISBN 978-0-89871-693-1. https://books.google.com/books?id=ryejJmJAj28C&pg=PA263. 
  152. ↑ Sung Nok Chiu; Dietrich Stoyan; Wilfrid S. Kendall; Joseph Mecke (2013). Stochastic Geometry and Its Applications. John Wiley & Sons. p. 112. ISBN 978-1-118-65825-3. https://books.google.com/books?id=825NfM6Nc-EC. 
  153. ↑ 153.0 153.1 Joseph L. Doob (1990). Stochastic processes. Wiley. pp. 94–96. https://books.google.com/books?id=NrsrAAAAYAAJ. 
  154. ↑ 154.0 154.1 Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. pp. 298, 299. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  155. ↑ Iosif Ilyich Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. p. 8. ISBN 978-0-486-69387-3. https://books.google.com/books?id=yJyLzG7N7r8C&pg=PR2. 
  156. ↑ 156.0 156.1 156.2 David Williams (1991). Probability with Martingales. Cambridge University Press. pp. 93, 94. ISBN 978-0-521-40605-5. https://books.google.com/books?id=e9saZ0YSi-AC. 
  157. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. pp. 22–23. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  158. ↑ Peter Mörters; Yuval Peres (2010). Brownian Motion. Cambridge University Press. p. 37. ISBN 978-1-139-48657-6. https://books.google.com/books?id=e-TbA-dSrzYC. 
  159. ↑ 159.0 159.1 L. C. G. Rogers; David Williams (2000). Diffusions, Markov Processes, and Martingales: Volume 1, Foundations. Cambridge University Press. p. 130. ISBN 978-1-107-71749-7. https://books.google.com/books?id=W0ydAgAAQBAJ&pg=PA356. 
  160. ↑ Alexander A. Borovkov (2013). Probability Theory. Springer Science & Business Media. p. 530. ISBN 978-1-4471-5201-9. https://books.google.com/books?id=hRk_AAAAQBAJ&pg. 
  161. ↑ Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 48. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  162. ↑ 162.0 162.1 Bernt Øksendal (2003). Stochastic Differential Equations: An Introduction with Applications. Springer Science & Business Media. p. 14. ISBN 978-3-540-04758-2. https://books.google.com/books?id=VgQDWyihxKYC. 
  163. ↑ 163.0 163.1 Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. pp. 472. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  164. ↑ Daniel Revuz; Marc Yor (2013). Continuous Martingales and Brownian Motion. Springer Science & Business Media. pp. 18–19. ISBN 978-3-662-06400-9. https://books.google.com/books?id=OYbnCAAAQBAJ. 
  165. ↑ David Applebaum (2004). Lévy Processes and Stochastic Calculus. Cambridge University Press. p. 20. ISBN 978-0-521-83263-2. https://books.google.com/books?id=q7eDUjdJxIkC. 
  166. ↑ Hiroshi Kunita (1997). Stochastic Flows and Stochastic Differential Equations. Cambridge University Press. p. 31. ISBN 978-0-521-59925-2. https://books.google.com/books?id=_S1RiCosqbMC. 
  167. ↑ Olav Kallenberg (2002). Foundations of Modern Probability. Springer Science & Business Media. p. 35. ISBN 978-0-387-95313-7. https://books.google.com/books?id=L6fhXh13OyMC. 
  168. ↑ Monique Jeanblanc; Marc Yor; Marc Chesney (2009). Mathematical Methods for Financial Markets. Springer Science & Business Media. p. 11. ISBN 978-1-85233-376-8. https://books.google.com/books?id=ZhbROxoQ-ZMC. 
  169. ↑ 169.0 169.1 169.2 Kiyosi Itō (2006). Essentials of Stochastic Processes. American Mathematical Soc.. pp. 32–33. ISBN 978-0-8218-3898-3. https://books.google.com/books?id=pY5_DkvI-CcC&pg=PR4. 
  170. ↑ Iosif Ilyich Gikhman; Anatoly Vladimirovich Skorokhod (1969). Introduction to the Theory of Random Processes. Courier Corporation. p. 150. ISBN 978-0-486-69387-3. https://books.google.com/books?id=yJyLzG7N7r8C&pg=PR2. 
  171. ↑ 171.0 171.1 Petar Todorovic (2012). An Introduction to Stochastic Processes and Their Applications. Springer Science & Business Media. pp. 19–20. ISBN 978-1-4613-9742-7. https://books.google.com/books?id=XpjqBwAAQBAJ&pg=PP5. 
  172. ↑ Ilya Molchanov (2005). Theory of Random Sets. Springer Science & Business Media. p. 340. ISBN 978-1-85233-892-3. https://books.google.com/books?id=kWEwk1UL42AC. 
  173. ↑ 173.0 173.1 Patrick Billingsley (2008). Probability and Measure. Wiley India Pvt. Limited. pp. 526–527. ISBN 978-81-265-1771-8. https://books.google.com/books?id=QyXqOXyxEeIC. 
  174. ↑ Alexander A. Borovkov (2013). Probability Theory. Springer Science & Business Media. p. 535. ISBN 978-1-4471-5201-9. https://books.google.com/books?id=hRk_AAAAQBAJ&pg. 
  175. ↑ Gusak, Dmytro; Kukush, Alexander; Kulik, Alexey; Mishura, Yuliya; Pilipenko, Andrey (2010). Theory of Stochastic Processes: With Applications to Financial Mathematics and Risk Theory. Springer Science & Business Media. p. 21. ISBN 978-0-387-87862-1.
  176. ↑ Joseph L. Doob (1990). Stochastic processes. Wiley. pp. 56. https://books.google.com/books?id=NrsrAAAAYAAJ. 
  177. ↑ Davar Khoshnevisan (2006). Multiparameter Processes: An Introduction to Random Fields. Springer Science & Business Media. p. 155. ISBN 978-0-387-21631-7. https://books.google.com/books?id=XADpBwAAQBAJ. 
  178. ↑ Lapidoth, Amos, A Foundation in Digital Communication, Cambridge University Press, 2009.
  179. ↑ 179.0 179.1 Kun Il Park, Fundamentals of Probability and Stochastic Processes with Applications to Communications, Springer, 2018, 978-3-319-68074-3
  180. ↑ 180.0 180.1 180.2 180.3 Ward Whitt (2006). Stochastic-Process Limits: An Introduction to Stochastic-Process Limits and Their Application to Queues. Springer Science & Business Media. pp. 78–79. ISBN 978-0-387-21748-2. https://books.google.com/books?id=LkQOBwAAQBAJ&pg=PR5. 
  181. ↑ 181.0 181.1 Gusak, Dmytro; Kukush, Alexander; Kulik, Alexey; Mishura, Yuliya; Pilipenko, Andrey (2010). Theory of Stochastic Processes: With Applications to Financial Mathematics and Risk Theory. Springer Science & Business Media. p. 21. ISBN 978-0-387-87862-1., p. 24
  182. ↑ 182.0 182.1 182.2 182.3 Vladimir I. Bogachev (2007). Measure Theory (Volume 2). Springer Science & Business Media. p. 53. ISBN 978-3-540-34514-5. https://books.google.com/books?id=CoSIe7h5mTsC. 
  183. ↑ 183.0 183.1 183.2 Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 4. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  184. ↑ 184.0 184.1 Søren Asmussen (2003). Applied Probability and Queues. Springer Science & Business Media. p. 420. ISBN 978-0-387-00211-8. https://books.google.com/books?id=BeYaTxesKy0C. 
  185. ↑ 185.0 185.1 185.2 Patrick Billingsley (2013). Convergence of Probability Measures. John Wiley & Sons. p. 121. ISBN 978-1-118-62596-5. https://books.google.com/books?id=6ItqtwaWZZQC. 
  186. ↑ Richard F. Bass (2011). Stochastic Processes. Cambridge University Press. p. 34. ISBN 978-1-139-50147-7. https://books.google.com/books?id=Ll0T7PIkcKMC. 
  187. ↑ Nicholas H. Bingham; Rüdiger Kiesel (2013). Risk-Neutral Valuation: Pricing and Hedging of Financial Derivatives. Springer Science & Business Media. p. 154. ISBN 978-1-4471-3856-3. https://books.google.com/books?id=AOIlBQAAQBAJ. 
  188. ↑ Alexander A. Borovkov (2013). Probability Theory. Springer Science & Business Media. p. 532. ISBN 978-1-4471-5201-9. https://books.google.com/books?id=hRk_AAAAQBAJ&pg. 
  189. ↑ Davar Khoshnevisan (2006). Multiparameter Processes: An Introduction to Random Fields. Springer Science & Business Media. pp. 148–165. ISBN 978-0-387-21631-7. https://books.google.com/books?id=XADpBwAAQBAJ. 
  190. ↑ Petar Todorovic (2012). An Introduction to Stochastic Processes and Their Applications. Springer Science & Business Media. p. 22. ISBN 978-1-4613-9742-7. https://books.google.com/books?id=XpjqBwAAQBAJ&pg=PP5. 
  191. ↑ Ward Whitt (2006). Stochastic-Process Limits: An Introduction to Stochastic-Process Limits and Their Application to Queues. Springer Science & Business Media. p. 79. ISBN 978-0-387-21748-2. https://books.google.com/books?id=LkQOBwAAQBAJ&pg=PR5. 
  192. ↑ Richard Serfozo (2009). Basics of Applied Stochastic Processes. Springer Science & Business Media. p. 2. ISBN 978-3-540-89332-5. https://books.google.com/books?id=JBBRiuxTN0QC. 
  193. ↑ Y.A. Rozanov (2012). Markov Random Fields. Springer Science & Business Media. p. 58. ISBN 978-1-4613-8190-7. https://books.google.com/books?id=wGUECAAAQBAJ. 
  194. ↑ Sheldon M. Ross (1996). Stochastic processes. Wiley. pp. 235, 358. ISBN 978-0-471-12062-9. https://books.google.com/books?id=ImUPAQAAMAAJ. 
  195. ↑ Ionut Florescu (2014). Probability and Stochastic Processes. John Wiley & Sons. pp. 373, 374. ISBN 978-1-118-59320-2. https://books.google.com/books?id=Z5xEBQAAQBAJ&pg=PR22. 
  196. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 49. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  197. ↑ 197.0 197.1 Søren Asmussen (2003). Applied Probability and Queues. Springer Science & Business Media. p. 7. ISBN 978-0-387-00211-8. https://books.google.com/books?id=BeYaTxesKy0C. 
  198. ↑ Emanuel Parzen (2015). Stochastic Processes. Courier Dover Publications. p. 188. ISBN 978-0-486-79688-8. https://books.google.com/books?id=0mB2CQAAQBAJ. 
  199. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. pp. 29, 30. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  200. ↑ John Lamperti (1977). Stochastic processes: a survey of the mathematical theory. Springer-Verlag. pp. 106–121. ISBN 978-3-540-90275-1. https://books.google.com/books?id=Pd4cvgAACAAJ. 
  201. ↑ Sheldon M. Ross (1996). Stochastic processes. Wiley. pp. 174, 231. ISBN 978-0-471-12062-9. https://books.google.com/books?id=ImUPAQAAMAAJ. 
  202. ↑ Sean Meyn; Richard L. Tweedie (2009). Markov Chains and Stochastic Stability. Cambridge University Press. p. 19. ISBN 978-0-521-73182-9. https://books.google.com/books?id=Md7RnYEPkJwC. 
  203. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 47. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  204. ↑ Reuven Y. Rubinstein; Dirk P. Kroese (2011). Simulation and the Monte Carlo Method. John Wiley & Sons. p. 225. ISBN 978-1-118-21052-9. https://books.google.com/books?id=yWcvT80gQK4C. 
  205. ↑ Dani Gamerman; Hedibert F. Lopes (2006). Markov Chain Monte Carlo: Stochastic Simulation for Bayesian Inference, Second Edition. CRC Press. ISBN 978-1-58488-587-0. https://books.google.com/books?id=yPvECi_L3bwC. 
  206. ↑ Y.A. Rozanov (2012). Markov Random Fields. Springer Science & Business Media. p. 61. ISBN 978-1-4613-8190-7. https://books.google.com/books?id=wGUECAAAQBAJ. 
  207. ↑ Donald L. Snyder; Michael I. Miller (2012). Random Point Processes in Time and Space. Springer Science & Business Media. p. 27. ISBN 978-1-4612-3166-0. https://books.google.com/books?id=c_3UBwAAQBAJ. 
  208. ↑ Pierre Bremaud (2013). Markov Chains: Gibbs Fields, Monte Carlo Simulation, and Queues. Springer Science & Business Media. p. 253. ISBN 978-1-4757-3124-8. https://books.google.com/books?id=jrPVBwAAQBAJ. 
  209. ↑ 209.0 209.1 209.2 Fima C. Klebaner (2005). Introduction to Stochastic Calculus with Applications. Imperial College Press. p. 65. ISBN 978-1-86094-555-7. https://books.google.com/books?id=JYzW0uqQxB0C. 
  210. ↑ 210.0 210.1 210.2 Ioannis Karatzas; Steven Shreve (1991). Brownian Motion and Stochastic Calculus. Springer. p. 11. ISBN 978-1-4612-0949-2. https://books.google.com/books?id=w0SgBQAAQBAJ&pg=PT5. 
  211. ↑ Joseph L. Doob (1990). Stochastic processes. Wiley. pp. 292, 293. https://books.google.com/books?id=NrsrAAAAYAAJ. 
  212. ↑ Gilles Pisier (2016). Martingales in Banach Spaces. Cambridge University Press. ISBN 978-1-316-67946-3. https://books.google.com/books?id=n3JNDAAAQBAJ&pg=PR4. 
  213. ↑ 213.0 213.1 J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. pp. 12, 13. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  214. ↑ 214.0 214.1 P. Hall; C. C. Heyde (2014). Martingale Limit Theory and Its Application. Elsevier Science. p. 2. ISBN 978-1-4832-6322-9. https://books.google.com/books?id=gqriBQAAQBAJ&pg=PR10. 
  215. ↑ J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 115. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  216. ↑ Sheldon M. Ross (1996). Stochastic processes. Wiley. p. 295. ISBN 978-0-471-12062-9. https://books.google.com/books?id=ImUPAQAAMAAJ. 
  217. ↑ 217.0 217.1 J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 11. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  218. ↑ Olav Kallenberg (2002). Foundations of Modern Probability. Springer Science & Business Media. pp. 96. ISBN 978-0-387-95313-7. https://books.google.com/books?id=L6fhXh13OyMC. 
  219. ↑ J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 371. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  220. ↑ J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 22. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  221. ↑ Geoffrey Grimmett; David Stirzaker (2001). Probability and Random Processes. OUP Oxford. p. 336. ISBN 978-0-19-857222-0. https://books.google.com/books?id=G3ig-0M4wSIC. 
  222. ↑ Glasserman, Paul; Kou, Steven (2006). "A Conversation with Chris Heyde". Statistical Science. 21 (2): 292, 293. arXiv:math/0609294. Bibcode:2006math......9294G. doi:10.1214/088342306000000088. ISSN 0883-4237.
  223. ↑ Francois Baccelli; Pierre Bremaud (2013). Elements of Queueing Theory: Palm Martingale Calculus and Stochastic Recurrences. Springer Science & Business Media. ISBN 978-3-662-11657-9. https://books.google.com/books?id=DH3pCAAAQBAJ&pg=PR2. 
  224. ↑ P. Hall; C. C. Heyde (2014). Martingale Limit Theory and Its Application. Elsevier Science. p. x. ISBN 978-1-4832-6322-9. https://books.google.com/books?id=gqriBQAAQBAJ&pg=PR10. 
  225. ↑ Jean Bertoin (1998). Lévy Processes. Cambridge University Press. p. viii. ISBN 978-0-521-64632-1. https://books.google.com/books?id=ftcsQgMp5cUC&pg=PR8. 
  226. ↑ 226.0 226.1 Applebaum, David (2004). "Lévy processes: From probability to finance and quantum groups". Notices of the AMS. 51 (11): 1336.
  227. ↑ David Applebaum (2004). Lévy Processes and Stochastic Calculus. Cambridge University Press. p. 69. ISBN 978-0-521-83263-2. https://books.google.com/books?id=q7eDUjdJxIkC. 
  228. ↑ Leonid Koralov; Yakov G. Sinai (2007). Theory of Probability and Random Processes. Springer Science & Business Media. p. 171. ISBN 978-3-540-68829-7. https://books.google.com/books?id=tlWOphOFRgwC. 
  229. ↑ David Applebaum (2004). Lévy Processes and Stochastic Calculus. Cambridge University Press. p. 19. ISBN 978-0-521-83263-2. https://books.google.com/books?id=q7eDUjdJxIkC. 
  230. ↑ Sung Nok Chiu; Dietrich Stoyan; Wilfrid S. Kendall; Joseph Mecke (2013). Stochastic Geometry and Its Applications. John Wiley & Sons. p. 109. ISBN 978-1-118-65825-3. https://books.google.com/books?id=825NfM6Nc-EC. 
  231. ↑ Sung Nok Chiu; Dietrich Stoyan; Wilfrid S. Kendall; Joseph Mecke (2013). Stochastic Geometry and Its Applications. John Wiley & Sons. p. 108. ISBN 978-1-118-65825-3. https://books.google.com/books?id=825NfM6Nc-EC. 
  232. ↑ Martin Haenggi (2013). Stochastic Geometry for Wireless Networks. Cambridge University Press. p. 10. ISBN 978-1-107-01469-5. https://books.google.com/books?id=CLtDhblwWEgC. 
  233. ↑ D.J. Daley; D. Vere-Jones (2006). An Introduction to the Theory of Point Processes: Volume I: Elementary Theory and Methods. Springer Science & Business Media. p. 194. ISBN 978-0-387-21564-8. https://books.google.com/books?id=6Sv4BwAAQBAJ. 
  234. ↑ 234.0 234.1 D.R. Cox; Valerie Isham (1980). Point Processes. CRC Press. p. 3. ISBN 978-0-412-21910-8. https://books.google.com/books?id=KWF2xY6s3PoC. 
  235. ↑ J. F. C. Kingman (1992). Poisson Processes. Clarendon Press. p. 8. ISBN 978-0-19-159124-2. https://books.google.com/books?id=VEiM-OtwDHkC. 
  236. ↑ Jesper Moller; Rasmus Plenge Waagepetersen (2003). Statistical Inference and Simulation for Spatial Point Processes. CRC Press. p. 7. ISBN 978-0-203-49693-0. https://books.google.com/books?id=dBNOHvElXZ4C. 
  237. ↑ Samuel Karlin; Howard E. Taylor (2012). A First Course in Stochastic Processes. Academic Press. p. 31. ISBN 978-0-08-057041-9. https://books.google.com/books?id=dSDxjX9nmmMC. 
  238. ↑ Volker Schmidt (2014). Stochastic Geometry, Spatial Statistics and Random Fields: Models and Algorithms. Springer. p. 99. ISBN 978-3-319-10064-7. https://books.google.com/books?id=brsUBQAAQBAJ&pg=PR5. 
  239. ↑ D.J. Daley; D. Vere-Jones (2006). An Introduction to the Theory of Point Processes: Volume I: Elementary Theory and Methods. Springer Science & Business Media. ISBN 978-0-387-21564-8. https://books.google.com/books?id=6Sv4BwAAQBAJ. 
  240. ↑ D.R. Cox; Valerie Isham (1980). Point Processes. CRC Press. ISBN 978-0-412-21910-8. https://books.google.com/books?id=KWF2xY6s3PoC. 
  241. ↑ 241.0 241.1 241.2 241.3 241.4 241.5 Gagniuc, Paul A. (2017). Markov Chains: From Theory to Implementation and Experimentation. US: John Wiley & Sons. pp. 1–2. ISBN 978-1-119-38755-8. 
  242. ↑ David, F. N. (1955). "Studies in the History of Probability and Statistics I. Dicing and Gaming (A Note on the History of Probability)". Biometrika. 42 (1/2): 1–15. doi:10.2307/2333419. ISSN 0006-3444. JSTOR 2333419.
  243. ↑ L. E. Maistrov (2014). Probability Theory: A Historical Sketch. Elsevier Science. p. 1. ISBN 978-1-4832-1863-2. https://books.google.com/books?id=2ZbiBQAAQBAJ&pg=PR9. 
  244. ↑ Seneta, E. (2006). "Probability, History of". Encyclopedia of Statistical Sciences. p. 1. doi:10.1002/0471667196.ess2065.pub2. ISBN 978-0471667193. 
  245. ↑ John Tabak (2014). Probability and Statistics: The Science of Uncertainty. Infobase Publishing. pp. 24–26. ISBN 978-0-8160-6873-9. https://books.google.com/books?id=h3WVqBPHboAC. 
  246. ↑ Bellhouse, David (2005). "Decoding Cardano's Liber de Ludo Aleae". Historia Mathematica. 32 (2): 180–202. doi:10.1016/j.hm.2004.04.001. ISSN 0315-0860.
  247. ↑ Anders Hald (2005). A History of Probability and Statistics and Their Applications before 1750. John Wiley & Sons. p. 221. ISBN 978-0-471-72517-6. https://books.google.com/books?id=pOQy6-qnVx8C. 
  248. ↑ L. E. Maistrov (2014). Probability Theory: A Historical Sketch. Elsevier Science. p. 56. ISBN 978-1-4832-1863-2. https://books.google.com/books?id=2ZbiBQAAQBAJ&pg=PR9. 
  249. ↑ John Tabak (2014). Probability and Statistics: The Science of Uncertainty. Infobase Publishing. p. 37. ISBN 978-0-8160-6873-9. https://books.google.com/books?id=h3WVqBPHboAC. 
  250. ↑ 250.0 250.1 Chung, Kai Lai (1998). "Probability and Doob". The American Mathematical Monthly. 105 (1): 28–35. doi:10.2307/2589523. ISSN 0002-9890. JSTOR 2589523.
  251. ↑ 251.0 251.1 251.2 251.3 251.4 Bingham, N. (2000). "Studies in the history of probability and statistics XLVI. Measure into probability: from Lebesgue to Kolmogorov". Biometrika. 87 (1): 145–156. doi:10.1093/biomet/87.1.145. ISSN 0006-3444.
  252. ↑ 252.0 252.1 Benzi, Margherita; Benzi, Michele; Seneta, Eugene (2007). "Francesco Paolo Cantelli. b. 20 December 1875 d. 21 July 1966". International Statistical Review. 75 (2): 128. doi:10.1111/j.1751-5823.2007.00009.x. ISSN 0306-7734.
  253. ↑ Doob, Joseph L. (1996). "The Development of Rigor in Mathematical Probability (1900-1950)". The American Mathematical Monthly. 103 (7): 586–595. doi:10.2307/2974673. ISSN 0002-9890. JSTOR 2974673.
  254. ↑ 254.0 254.1 254.2 254.3 254.4 254.5 Cramer, Harald (1976). "Half a Century with Probability Theory: Some Personal Recollections". The Annals of Probability. 4 (4): 509–546. doi:10.1214/aop/1176996025. ISSN 0091-1798.
  255. ↑ Truesdell, C. (1975). "Early kinetic theories of gases". Archive for History of Exact Sciences. 15 (1): 22–23. doi:10.1007/BF00327232. ISSN 0003-9519.
  256. ↑ Brush, Stephen G. (1967). "Foundations of statistical mechanics 1845?1915". Archive for History of Exact Sciences. 4 (3): 150–151. doi:10.1007/BF00412958. ISSN 0003-9519.
  257. ↑ Truesdell, C. (1975). "Early kinetic theories of gases". Archive for History of Exact Sciences. 15 (1): 31–32. doi:10.1007/BF00327232. ISSN 0003-9519.
  258. ↑ Brush, S.G. (1958). "The development of the kinetic theory of gases IV. Maxwell". Annals of Science. 14 (4): 243–255. doi:10.1080/00033795800200147. ISSN 0003-3790.
  259. ↑ Brush, Stephen G. (1968). "A history of random processes". Archive for History of Exact Sciences. 5 (1): 15–16. doi:10.1007/BF00328110. ISSN 0003-9519.
  260. ↑ Kendall, D. G.; Batchelor, G. K.; Bingham, N. H.; Hayman, W. K.; Hyland, J. M. E.; Lorentz, G. G.; Moffatt, H. K.; Parry, W.; Razborov, A. A.; Robinson, C. A.; Whittle, P. (1990). "Andrei Nikolaevich Kolmogorov (1903–1987)". Bulletin of the London Mathematical Society. 22 (1): 33. doi:10.1112/blms/22.1.31. ISSN 0024-6093.
  261. ↑ Vere-Jones, David (2006). "Khinchin, Aleksandr Yakovlevich". Encyclopedia of Statistical Sciences. p. 1. doi:10.1002/0471667196.ess6027.pub2. ISBN 978-0471667193. 
  262. ↑ Vere-Jones, David (2006). "Khinchin, Aleksandr Yakovlevich". Encyclopedia of Statistical Sciences. p. 4. doi:10.1002/0471667196.ess6027.pub2. ISBN 978-0471667193. 
  263. ↑ 263.0 263.1 Snell, J. Laurie (2005). "Obituary: Joseph Leonard Doob". Journal of Applied Probability. 42 (1): 251. doi:10.1239/jap/1110381384. ISSN 0021-9002.
  264. ↑ Lindvall, Torgny (1991). "W. Doeblin, 1915-1940". The Annals of Probability. 19 (3): 929–934. doi:10.1214/aop/1176990329. ISSN 0091-1798.
  265. ↑ Getoor, Ronald (2009). "J. L. Doob: Foundations of stochastic processes and probabilistic potential theory". The Annals of Probability. 37 (5): 1655. arXiv:0909.4213. Bibcode:2009arXiv0909.4213G. doi:10.1214/09-AOP465. ISSN 0091-1798. S2CID 17288507.
  266. ↑ 266.0 266.1 Bingham, N. H. (2005). "Doob: a half-century on". Journal of Applied Probability. 42 (1): 257–266. doi:10.1239/jap/1110381385. ISSN 0021-9002.
  267. ↑ 267.0 267.1 267.2 267.3 267.4 Meyer, Paul-André (2009). "Stochastic Processes from 1950 to the Present". Electronic Journal for History of Probability and Statistics. 5 (1): 1–42.
  268. ↑ "Kiyosi Itô receives Kyoto Prize". Notices of the AMS. 45 (8): 981–982. 1998.
  269. ↑ Jean Bertoin (1998). Lévy Processes. Cambridge University Press. p. viii and ix. ISBN 978-0-521-64632-1. https://books.google.com/books?id=ftcsQgMp5cUC&pg=PR8. 
  270. ↑ J. Michael Steele (2012). Stochastic Calculus and Financial Applications. Springer Science & Business Media. p. 176. ISBN 978-1-4684-9305-4. https://books.google.com/books?id=fsgkBAAAQBAJ&pg=PR4. 
  271. ↑ P. Hall; C. C. Heyde (2014). Martingale Limit Theory and Its Application. Elsevier Science. pp. 1, 2. ISBN 978-1-4832-6322-9. https://books.google.com/books?id=gqriBQAAQBAJ&pg=PR10. 
  272. ↑ Dynkin, E. B. (1989). "Kolmogorov and the Theory of Markov Processes". The Annals of Probability. 17 (3): 822–832. doi:10.1214/aop/1176991248. ISSN 0091-1798.
  273. ↑ Ellis, Richard S. (1995). "An overview of the theory of large deviations and applications to statistical mechanics". Scandinavian Actuarial Journal. 1995 (1): 98. doi:10.1080/03461238.1995.10413952. ISSN 0346-1238.
  274. ↑ Raussen, Martin; Skau, Christian (2008). "Interview with Srinivasa Varadhan". Notices of the AMS. 55 (2): 238–246.
  275. ↑ Malte Henkel; Dragi Karevski (2012). Conformal Invariance: an Introduction to Loops, Interfaces and Stochastic Loewner Evolution. Springer Science & Business Media. p. 113. ISBN 978-3-642-27933-1. https://books.google.com/books?id=fnCQWd0GEZ8C&pg=PA113. 
  276. ↑ "2006 Fields Medals Awarded". Notices of the AMS. 53 (9): 1041–1044. 2015.
  277. ↑ Quastel, Jeremy (2015). "The Work of the 2014 Fields Medalists". Notices of the AMS. 62 (11): 1341–1344.


编辑推荐

集智课程

熵率（Entropy-Rate）

在本课程中，程帆老师主要介绍了随机过程，熵率，热力学第二定律以及马氏链的函数相关知识。


集智文章


本中文词条水流心不竞翻译，薄荷编辑，如有问题，欢迎在讨论页面留言。


本词条内容源自wikipedia及公开资料，遵守 CC3.0协议。

取自“https://wiki.swarma.org/index.php?title=随机过程&oldid=28498”