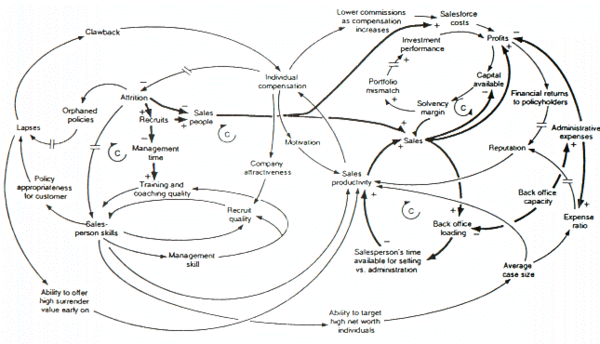
因果回路图

此词条暂由彩云小译翻译，未经人工整理和审校，带来阅读不便，请见谅。

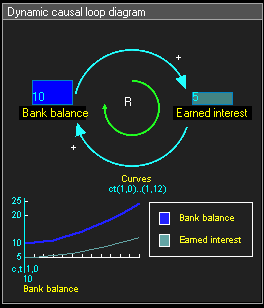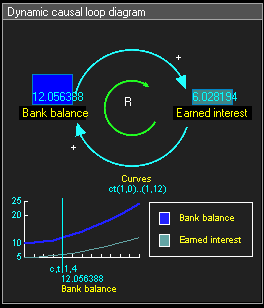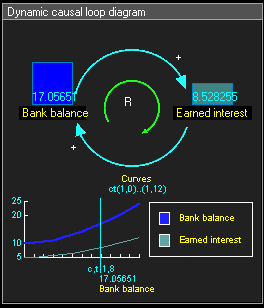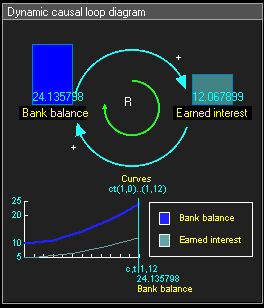
Example of positive reinforcing loop: Bank balance and Earned interest

Example of positive reinforcing loop: Bank balance and Earned interest

A causal loop diagram (CLD) is a causal diagram that aids in visualizing how different variables in a system are interrelated. The diagram consists of a set of nodes and edges. Nodes represent the variables and edges are the links that represent a connection or a relation between the two variables. A link marked positive indicates a positive relation and a link marked negative indicates a negative relation. A positive causal link means the two nodes change in the same direction, i.e. if the node in which the link starts decreases, the other node also decreases. Similarly, if the node in which the link starts increases, the other node increases as well. A negative causal link means the two nodes change in opposite directions, i.e. if the node in which the link starts increases, the other node decreases and vice versa.

A causal loop diagram (CLD) is a causal diagram that aids in visualizing how different variables in a system are interrelated. The diagram consists of a set of nodes and edges. Nodes represent the variables and edges are the links that represent a connection or a relation between the two variables. A link marked positive indicates a positive relation and a link marked negative indicates a negative relation. A positive causal link means the two nodes change in the same direction, i.e. if the node in which the link starts decreases, the other node also decreases. Similarly, if the node in which the link starts increases, the other node increases as well. A negative causal link means the two nodes change in opposite directions, i.e. if the node in which the link starts increases, the other node decreases and vice versa.

环路图图是一种因果关系图，有助于可视化地显示一个系统中不同的变量是如何相互关联的。该图由一组节点和边组成。节点表示变量，边是表示两个变量之间的联系或关系的链接。标记为肯定的链接表示肯定关系，标记为否定的链接表示否定关系。一个积极的因果关系意味着两个节点朝着同一个方向改变，即。如果链接开始的节点减少，另一个节点也减少。类似地，如果链接开始的节点增加，其他节点也会增加。负的因果关系是指两个节点朝相反的方向变化，即。如果链接开始的节点增加，另一个节点减少，反之亦然。Br /

Closed cycles in the diagram are very important features of the CLDs. A closed cycle is either defined as a reinforcing or balancing feedback loop. A reinforcing loop is a cycle in which the effect of a variation in any variable propagates through the loop and returns to the variable reinforcing the initial deviation i.e. if a variable increases in a reinforcing loop the effect through the cycle will return an increase to the same variable and vice versa. A balancing loop is the cycle in which the effect of a variation in any variable propagates through the loop and returns to the variable a deviation opposite to the initial one i.e. if a variable increases in a balancing loop the effect through the cycle will return a decrease to the same variable and vice versa.

Closed cycles in the diagram are very important features of the CLDs. A closed cycle is either defined as a reinforcing or balancing feedback loop. A reinforcing loop is a cycle in which the effect of a variation in any variable propagates through the loop and returns to the variable reinforcing the initial deviation i.e. if a variable increases in a reinforcing loop the effect through the cycle will return an increase to the same variable and vice versa. A balancing loop is the cycle in which the effect of a variation in any variable propagates through the loop and returns to the variable a deviation opposite to the initial one i.e. if a variable increases in a balancing loop the effect through the cycle will return a decrease to the same variable and vice versa.

图中的闭合循环是 cld 的重要特征。一个封闭的循环要么被定义为一个强化的或平衡的反馈回路。强化循环是一个循环，在这个循环中，任何变量的变化影响通过循环传播，并返回到强化初始偏差的变量。如果一个变量在一个强化循环中增加，循环中的效应将返回到相同变量的增加，反之亦然。平衡回路是一个循环，在这个循环中，任何变量的变化影响通过回路传播，并返回给变量一个与初始变量相反的偏差。如果一个变量在平衡循环中增加，那么循环中的效应将会使同一个变量减少，反之亦然。

If a variable varies in a reinforcing loop the effect of the change reinforces the initial variation. The effect of the variation will then create another reinforcing effect. Without breaking the loop the system will be caught in a vicious cycle of circular chain reactions. For this reason, closed loops are critical features in the CLDs.

如果一个变量在一个强化循环中发生变化，这种变化的影响加强了初始变化。这种变化的影响会产生另一种强化效应。如果不打破循环，系统将陷入循环链反应的恶性循环。因此，闭环是 cld 中的关键特性。

Example of positive reinforcing loop:

正向强化循环的例子:

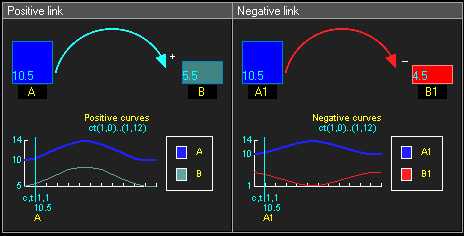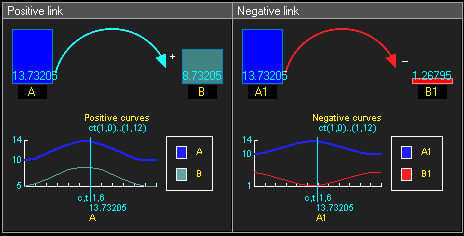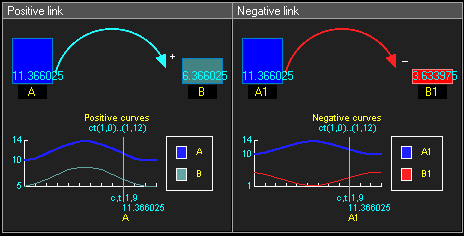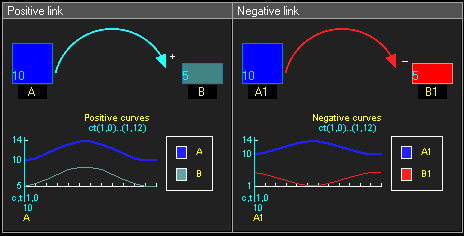
The amount of the Bank Balance will affect the amount of the Earned Interest, as represented by the top blue arrow, pointing from Bank Balance to Earned Interest.

Since an increase in Bank balance results in an increase in Earned Interest, this link is positive, which is denoted with a "+".

The Earned interest gets added to the Bank balance, also a positive link, represented by the bottom blue arrow.

The causal effect between these nodes forms a positive reinforcing loop, represented by the green arrow, which is denoted with an "R".^[1]

</ref>

/ 参考

History

模板:Main article

The use of nodes and arrows to construct directed graph models of cause and effect dates back to the invention of path analysis by Sewall Wright in 1918, long before System Dynamics. Due to the limitations of genetic data, however, these early causal graphs contained no loops — they were directed acyclic graphs. The first formal use of Causal Loop Diagrams was explained by Dr. Dennis Meadows at a conference for educators (Systems Thinking & Dynamic Modeling Conference for K-12 Education in New Hampshire sponsored by Creative Learning Exchange ^[2]).

The use of nodes and arrows to construct directed graph models of cause and effect dates back to the invention of path analysis by Sewall Wright in 1918, long before System Dynamics. Due to the limitations of genetic data, however, these early causal graphs contained no loops — they were directed acyclic graphs. The first formal use of Causal Loop Diagrams was explained by Dr. Dennis Meadows at a conference for educators (Systems Thinking & Dynamic Modeling Conference for K-12 Education in New Hampshire sponsored by Creative Learning Exchange ).

使用节点和箭头来构建因果关系的有向图模型可以追溯到1918年 Sewall Wright 发明的路径分析，远在系统动力学之前。然而，由于遗传数据的局限性，这些早期因果图不包含环路ーー它们是有向无环图。丹尼斯 · 梅多斯博士在新罕布什尔州的一次教育工作者会议(由创造性学习交流组织主办的 K-12教育系统思考与动态建模会议)上解释了因果循环图的第一次正式使用。

Meadows explained that when he and others were working on the World3 model (circa 1970–72), they realized they would not be able to use the computer output to explain how the feedback loops worked in their model when presenting their results to others. They decided to show feedback loops (without the stocks, flows and every variable), using arrows connecting the names of major model components in the feedback loops. This may have been the first formal use of Causal Loop Diagrams.^[3]

Meadows explained that when he and others were working on the World3 model (circa 1970–72), they realized they would not be able to use the computer output to explain how the feedback loops worked in their model when presenting their results to others. They decided to show feedback loops (without the stocks, flows and every variable), using arrows connecting the names of major model components in the feedback loops. This may have been the first formal use of Causal Loop Diagrams.

Meadows 解释说，当他和其他人在研究 World3模型(大约在1970-72年)时，他们意识到他们不能用计算机输出来解释他们的模型中的反馈回路在向其他人展示结果时是如何工作的。他们决定显示反馈回路(没有存量、流量和每个变量) ，使用箭头连接反馈回路中主要模型组件的名称。这可能是因果循环图的第一次正式使用。

Positive and negative causal links

Positive causal link means that the two nodes change in the same direction, i.e. if the node in which the link starts decreases, the other node also decreases. Similarly, if the node in which the link starts increases, the other node increases.

Negative causal link means that the two nodes change in opposite directions, i.e. if the node in which the link starts increases, then the other node decreases, and vice versa.

Example

Dynamic causal loop diagram: positive and negative links

frame

框架

Reinforcing and balancing loops

To determine if a causal loop is reinforcing or balancing, one can start with an assumption, e.g. "Node 1 increases" and follow the loop around. The loop is:

为了确定一个因果循环是在加强还是在平衡，我们可以从一个假设开始，例如:。“节点1增加”并跟随循环。回路是:

reinforcing if, after going around the loop, one ends up with the same result as the initial assumption.

balancing if the result contradicts the initial assumption.

Or to put it in other words:

或者换句话说:

reinforcing loops have an even number of negative links (zero also is even, see example below)

balancing loops have an odd number of negative links.

Identifying reinforcing and balancing loops is an important step for identifying Reference Behaviour Patterns, i.e. possible dynamic behaviours of the system.

Identifying reinforcing and balancing loops is an important step for identifying Reference Behaviour Patterns, i.e. possible dynamic behaviours of the system.

识别加强和平衡循环是识别参照行为模式的一个重要步骤，即。系统可能的动态行为。

Reinforcing loops are associated with exponential increases/decreases.

Balancing loops are associated with reaching a plateau.

If the system has delays (often denoted by drawing a short line across the causal link), the system might fluctuate.

如果系统有延迟(通常表示在因果关系之间画一条短线) ，系统可能会发生波动。

Example

文件:Adoption CLD.svg

Causal loop diagram of Adoption model, used to demonstrate systems dynamics

frame

框架

Causal loop diagram of a model examining the growth or decline of a life insurance company

frame

框架

References

↑ John D.Sterman, Business Dynamics: Systems Thinking and Modeling for a Complex World. McGraw Hill/Irwin, 2000.

John D.Sterman, Business Dynamics: Systems Thinking and Modeling for a Complex World. McGraw Hill/Irwin, 2000.

约翰 · d · 斯特曼，《商业动力学: 复杂世界的系统思考与建模》。麦格劳 · 希尔 / 欧文，2000年。

↑ http://www.clexchange.org/
↑ Anecdote by Richard Turnock attending informal discussion where Dennis Meadows explained origin of CLD

External links

模板:Commons category

WikiSD the System Dynamics Society Wiki

Learn to Read Causal Loop Diagrams via SystemsAndUs

Category:Causal diagrams

类别: 因果图表

Category:Diagrams

类别: 图表

Category:Causality

分类: 因果关系

This page was moved from wikipedia:en:Causal loop diagram. Its edit history can be viewed at 环路图/edithistory

[sterman-1] John D.Sterman, Business Dynamics: Systems Thinking and Modeling for a Complex World. McGraw Hill/Irwin, 2000.
v
t
e
Inline tags
Attribution

{{Attribution needed}}

{{By whom}}

{{Weasel-inline}}

{{Which?}}

{{Who}}

Citation

{{Better source}}

{{Citation needed}}
{{Citation needed span}}

{{Reference necessary}}

{{Citation needed (lead)}}

{{Failed verification}}

{{Full}}

{{Primary source-inline}}

{{Request quotation}}

{{Retracted}}

{{Third-party-inline}}

Incomplete

{{Author missing}}

{{Author incomplete}}

{{Date missing}}

{{ISBN missing}}

{{Page needed}}

{{Publisher missing}}

{{Title incomplete}}

{{Year missing}}

{{Dead link}}

Content

{{Contradict-inline}}

{{Contradiction-inline}}

{{Dubious|talk page section name}}

{{Examples}}

{{Inconsistent}}

{{List fact}}

{{Lopsided}}

{{POV-statement}}

Date and place

{{Clarify timeframe}}

{{Quantify}}

{{Update after}}

{{Update-small}}

{{When}}

{{Where}}

{{Year needed}}

Wikification

{{Disambiguation needed}}

{{Pronunciation needed}}

Wording

{{Ambiguous}}

{{Awkward}}

{{Buzz}}

{{Clarify}}

{{Clarify span}}

{{Definition}}

{{Elucidate}}

{{Expand acronym}}

{{Technical-statement}}

{{Vague|optional message to be displayed on mouseover}}

{{Why?}}

Category

John D.Sterman, Business Dynamics: Systems Thinking and Modeling for a Complex World. McGraw Hill/Irwin, 2000.
约翰 · d · 斯特曼，《商业动力学: 复杂世界的系统思考与建模》。麦格劳 · 希尔 / 欧文，2000年。

[2] v

[3] t

[4] e

[5] {{Attribution needed}}

[6] {{By whom}}

[7] {{Weasel-inline}}

[8] {{Which?}}

[9] {{Who}}

[10] {{Better source}}

[11] {{Citation needed}}
{{Citation needed span}}

{{Reference necessary}}

[12] {{Citation needed span}}

[13] {{Reference necessary}}

[14] {{Citation needed (lead)}}

[15] {{Failed verification}}

[16] {{Full}}

[17] {{Primary source-inline}}

[18] {{Request quotation}}

[19] {{Retracted}}

[20] {{Third-party-inline}}

[21] {{Author missing}}

[22] {{Author incomplete}}

[23] {{Date missing}}

[24] {{ISBN missing}}

[25] {{Page needed}}

[26] {{Publisher missing}}

[27] {{Title incomplete}}

[28] {{Year missing}}

[29] {{Dead link}}

[30] {{Contradict-inline}}

[31] {{Contradiction-inline}}

[32] {{Dubious|talk page section name}}

[33] {{Examples}}

[34] {{Inconsistent}}

[35] {{List fact}}

[36] {{Lopsided}}

[37] {{POV-statement}}

[38] {{Clarify timeframe}}

[39] {{Quantify}}

[40] {{Update after}}

[41] {{Update-small}}

[42] {{When}}

[43] {{Where}}

[44] {{Year needed}}

[45] {{Disambiguation needed}}

[46] {{Pronunciation needed}}

[47] {{Ambiguous}}

[48] {{Awkward}}

[49] {{Buzz}}

[50] {{Clarify}}

[51] {{Clarify span}}

[52] {{Definition}}

[53] {{Elucidate}}

[54] {{Expand acronym}}

[55] {{Technical-statement}}

[56] {{Vague|optional message to be displayed on mouseover}}

[57] {{Why?}}

[2] ttp://www.clexchange.org/

[3] Anecdote by Richard Turnock attending informal discussion where Dennis Meadows explained origin of CLD

[1]

[2]

[3]

因果回路图

目录

History

Positive and negative causal links

Example

Reinforcing and balancing loops

Example

See also

References

External links

导航菜单

搜索