规模法则

Introduction 引言

规模（scale）是除去时间、空间之外另一个重要的维度。规模缩放（Scaling）的过程中隐藏着世界非线性本质奥秘背后的共性——规模法则。

生命或许是宇宙中最复杂、最多样化的现象，它展现出了大大小小、纷繁异常的组织、功能和行为。据估计，地球上有超过800万个不同的生物物种。［1］它们体形不一，最小的细菌质量不足1皮克，而最大的动物——蓝鲸则重100多吨。前往巴西的热带雨林，你可以在一块足球场面积大小的区域内找到100多种树木和分属数千个物种的数百万只昆虫。每个物种的孕育、出生、繁殖和死亡有太多令人惊异的不同。许多细菌仅能存活1小时，只需十万亿分之一瓦特的代谢率便能存活；而鲸类可以存活100年之久，其代谢率达到数百瓦特。［2］我们人类为这个星球所带来的社会生活的复杂性和多样性则在这幅绚丽多彩的生物生命画卷上增添了浓墨重彩的一笔，尤其是那些潜藏在城市外表下的商业、建筑及每位城市居民所表现出来的多样文化和他们背后隐藏的喜怒哀乐，以及所有这些非同寻常的现象。

当我们将以上任何一种复杂的现象与非常简单的行星围绕太阳公转的规律或手表和苹果手机的计时规律相比的时候，自然会思考：在所有这些复杂性和多样性的背后，有没有可能也存在一种类似的潜在规律呢？是否存在一些令人信服的简单法则，确实是从植物、动物等生物体到城市、公司等所有复杂系统都会遵循的？全球各地的森林、草原和城市中正在上演的一幕幕景象是否都是随机的、变化无常的，是一个又一个的偶然事件吗？鉴于产生多样化结果进化过程的随机性，与直觉不同的是，任何规律或系统性行为的出现似乎都不太可能。毕竟，组成生物圈的每个生物体、每个子系统、每个器官、每个细胞、每个基因都是在独特的历史轨迹上，在与众不同的生态环境中，通过自然选择过程进化而来的。现在，让我们来看看图1~图4。每幅图都呈现一个已知变量与其规模大小的关系，这些变量都在人们的生活中扮演着重要的角色。图1是动物代谢率与其体重的关系图。图2是不同动物一生中的心跳次数与其体重的关系图。图3是一座城市所产生的专利数量与该城市人口的关系图。图4是上市公司的净收入和总资产与其雇员人数的关系图。

无须成为一名科学家或以上任何一个领域的专家，你马上就可以发现，尽管它们代表了我们在生命中遇到过的最复杂、最多样化的过程，但每幅图都揭示了一些简单、系统性、规律性的东西。在每一幅图中，所有的数据都奇迹般地差不多排列成一条直线，并没有出现任意分布的现象。而我们此前曾预测，由于每一种动物、每一座城市、每一家公司的历史和所处地理环境不同，可能会出现任意分布的状况。或许最令人吃惊的是图1–2，所有哺乳动物一生中的平均心跳次数大致相当，尽管体形较小的老鼠只能存活几年时间，而大型动物鲸则可以存活100年之久。图1~图4中的例子只是为数众多的缩放关系中的一小部分，动物、植物、生态系统、城市和公司中几乎任何可量化的特点都与规模存在可量化的缩放关系，这些显著规律的存在表明，在所有这些迥异的高度复杂现象中，都存在着共同的概念框架——动物、植物、人类社会行为、城市与公司的活力、增长和组织事实上都遵循类似的一般规律。这种宏观性的框架可以帮助我们解决一系列问题：

• 为何我们最多只能活到120岁，而不是1 000岁或100万岁？为何我们会死亡？是什么限制了人类的寿命？人们能否通过组成自身肌体的细胞和复杂分子计算出自己的寿命？它们能否被改变？寿命是否可以延长？

• 为何身体成分与我们几乎相同的老鼠只能存活两三年时间，而大象却能活到75岁？尽管存在这样的差异，但是为何包括大象、老鼠在内的所有哺乳动物一生中的心跳次数几乎相同，都达到了大约15亿次？

• 从细胞、鲸类到森林，为何生物体和生态系统都以一种普遍、系统性和可预测的方式与规模大小存在比例关系？看上去能够控制它们从生到死的大部分心理和生理历史的神奇数字“4”源自哪里？

• 为何我们会停止生长？为何我们每天必须睡8个小时？为何我们长肿瘤比老鼠少得多，而鲸类几乎不长肿瘤？

• 为何几乎所有公司都只能生存数年时间，而城市却能不断增长，且能够避开即便是最强大、看上去最完美的公司也无法逃避的命运？我们能否预测各家公司的大致生存周期？

• 我们能否发展出一门城市和公司科学，通过一种可量化、可预测的概念性框架了解它们的活力、增长和进化？

• 城市规模大小有限制吗？是否存在最优规模？动物和植物的生长规模有限制吗？是否会出现巨型昆虫或巨型城市？

• 为何生活节奏持续加速？为何创新速度必须持续加速才能维持社会经济生活？

• 我们如何确保人类设计的仅有1万年进化历史的系统能够继续与已经进化了数十亿年的自然生物世界共存？我们能否维持一个受思想和财富创造所驱动、充满生机活力、不断创新的社会？地球是否注定会变成一个充斥着贫民窟、冲突和破坏的星球？

生命的简单性、一致性与复杂性

从最小的细菌到最大的城市和生态系统，生命系统是典型的复杂适应系统，运行在范围广阔的多个空间、时间、能量和质量的尺度上。仅在质量规模上，生命便跨越了30个数量级以上，从为新陈代谢和遗传密码提供能量的分子到生态系统和城市。这一范围的广度大大超过了地球的质量与整个银河系的质量之间的比例，后者仅跨越了18个数量级，相当于一个电子的质量与一只老鼠的质量之间的比例。

在这一庞大的范围内，生命事实上利用相同的基本构成要素和建造过程创造了令人惊叹的各种各样的形式、功能和动力学行为。所有生命的运行都是通过把物理或化学来源的能量转化为有机分子，这些有机分子通过新陈代谢过程构建、维持和繁殖复杂的、高度组织化的系统。这又是通过两个截然不同而又密切相互作用的系统运行实现的：遗传密码系统（储存及处理构建和维持生物体运作的信息与“指令”）和新陈代谢系统（获取、转化、分配能量和物质，用于维持、增长和繁殖）。人们在从分子到生物体的各个层级阐释这两个系统方面已经取得了很大的进展。然而，想要了解信息处理（基因组学）如何与能量和资源处理（新陈代谢）相互融合以维持生命，却依然是一个巨大的挑战。寻找作为这些系统结构、动力和结合的普遍基础原则是理解生命的根本所在，也是在医学、农业、环境学等不同背景中管理生物和社会经济系统的基础。

潜藏在复杂性下的简单性：网络原理与克莱伯定律、自相似性和异速生长律

规模法则在生物学中的机理源头根植于多重网络的通用数学、动力学和组织特性，这些网络将能量、物质和信息分配至细胞、线粒体等渗透进生物体内的细微点。由于生物网络的结构如此多样，并与规模法则的同一性形成鲜明对比，它们的一般属性必须独立于它们各自的进化设计之外。

空间填充

空间填充背后的理念很简单，也很直观。粗略地说，它意味着网络的触角必须延伸至它所服务的整个系统的各个角落，正如图3–7所示。更加具体地说，无论网络的几何学和拓扑结构如何，它都必须服务生物体的所有生物子单元或子系统。我们可以用一个更加熟悉的例子来理解：人体循环系统是一个经典的分级网络，心脏会向始于主动脉的多层次网络输送血液，经过规模不断缩小的血管到达最小的毛细血管，然后再通过网络系统返回至心脏。空间填充就是指毛细血管作为终端单元或网络的末支，必须服务于人体内的每一个细胞，高效地为细胞供给足够的血液和氧气。事实上，这一切只需要毛细血管距离细胞足够近，以使得足够的氧气能够高效地穿透毛细血管壁，并通过细胞的外膜。

终端单元的恒定性

这意味着一个给定网络的终端单元，如我们刚刚讨论过的循环系统中的毛细血管，都有近似相同的尺寸和特点，无论生物体的体形多大。终端单元是网络的重要组成部分，因为它们是能量和资源交换的传输点与分配点。例如植物的叶柄、体内的细胞、细胞内的线粒体。当个体从新生儿成长为成年人时，或者当不同体形大小的新物种进化时，终端单元不会重新改造，也不会重组或重新调节。

终端单元的恒定性可以放在自然选择的节约天性的背景下来了解。毛细血管、线粒体、细胞等是新物种的相应网络的“现成”基石，会相应地进行调节。终端单元的恒定性构成了分类的特性。例如，所有的哺乳动物都有相同的毛细血管。这一类别中的不同物种，如大象、人和老鼠之间的区别就在于网络布局的大小。从这个角度而言，分类之间的差别，即哺乳动物、植物和鱼等之间的差别，是由它们自身不同网络的终端单元的不同特性决定的。尽管所有的哺乳动物都有相似的毛细血管和线粒体，鱼类也同样如此，但哺乳动物的毛细血管和线粒体与鱼的毛细血管和线粒体存在大小及整体特点的不同。

优化

最后一个假设认为，在自然选择过程中隐含的连续的多重反馈和调整机制在过去长期发挥作用，使得网络性能得到了“优化”。举例来说，包括我们人类在内，任何哺乳动物的心脏用来通过循环系统输送血液的平均能量值都最小化，即它是在既定的设计和不同的网络限制条件下能够得到的最小可能。换句话说，在循环系统的架构和动力学的无限种可能中，那些能够进化并最终为所有哺乳动物所共有的充满恒定终端单元的空间是能够将心脏输出最小化的。网络不断进化的结果是维持个体生命、完成生命日常生活任务的能量被最小化，以使得留给性生活、繁殖、抚育后代的能量最大化。这被称作达尔文适应度 Darwinian Fitness，是普通个体为下一代基因库所做的基因贡献。

优化原则位于自然界所有基本法则的核心，无论是牛顿定律、麦克斯韦的电磁学理论、量子力学、爱因斯坦的相对论，还是基本粒子的大一统理论。它们的现代构成都是一个数学框架，其中一个被称作“作用量”的数值被最小化，这个数值与能量存在松散关系。所有的物理学定律都源自“最小作用量原理”，该原理认为，在一个系统能够拥有或遵循的所有可能配置中，最终得以实现的是作用量最小的那个配置。因此，宇宙自大爆炸以来的动力学、架构和时间演化，来自黑洞及传输手机信息所用的卫星和信息本身，所有的电子、光子、希格斯粒子，以及物理学中的一切，都是由这个优化原则决定的。

城市和公司是大型的生物体吗？

类似于生物网络的是，城市中的许多基础设施网络也是空间填充的，例如，天然气、水和电等公用事业网络的终端单元或终点都必须为构成城市的所有不同建筑物提供供给。连接你的房屋与城市水路和电路的管道就像毛细血管，可以把你的房屋想象成细胞。与此相似的是，公司的所有雇员都可以被看作终端单元，他们必须通过连接首席执行官与管理层的多重网络获得资源（如工资）和信息的供给。

地球科学中的规模法则

数学

Scaling laws 物理学中的标度律

Scaling laws are the expression of physical principles in the mathematical language of homogeneous functions.

标度律是物理原理在齐次函数数学语言中的表达。

A function [math]\displaystyle{ f (x, y, z,\ldots) }[/math] is said to be homogeneous of degree [math]\displaystyle{ n }[/math] in the variables [math]\displaystyle{ x,y,z,\ldots }[/math] if, identically for all [math]\displaystyle{ \lambda\ , }[/math]

[math]\displaystyle{ \label{eq:1} f(\lambda x, \lambda y, \lambda z, \ldots) \equiv \lambda^{n}f (x, y, z, \ldots). }[/math]

如果对所有 [math]\displaystyle{ \lambda\ }[/math] 都满足关系

[math]\displaystyle{ f(\lambda x, \lambda y, \lambda z, \ldots) \equiv \lambda^{n}f (x, y, z, \ldots). }[/math]

(1)

则称函数 [math]\displaystyle{ f (x, y, z,\ldots) }[/math] 是变量 [math]\displaystyle{ x,y,z,\ldots }[/math] 的 [math]\displaystyle{ n }[/math] 次齐次函数。

For example, [math]\displaystyle{ ax^2 + bxy + cy^2 }[/math] is homogeneous of degree 2 in [math]\displaystyle{ x }[/math] and [math]\displaystyle{ y }[/math] and of the first degree in [math]\displaystyle{ a, b, }[/math] and [math]\displaystyle{ c\ . }[/math]

例如，[math]\displaystyle{ ax^2 + bxy + cy^2 }[/math] 是 [math]\displaystyle{ x }[/math] 和 [math]\displaystyle{ y }[/math] 二次齐次函数，而对 [math]\displaystyle{ a, b, }[/math] [math]\displaystyle{ c\ }[/math]则是一次齐次的。

By setting [math]\displaystyle{ \lambda = 1/x }[/math] in (1) we have as an alternative expression of homogeneity: [math]\displaystyle{ f (x, y, z, \ldots) }[/math] is homogeneous of degree [math]\displaystyle{ n }[/math] in [math]\displaystyle{ x, y, z, \ldots }[/math] if

[math]\displaystyle{ \label{eq:2} f(x, y, z, \ldots) = x^nf(1, y/x, z/x, \ldots) \equiv x^n\phi(y/x, z/x, \ldots); }[/math]

将 [math]\displaystyle{ \lambda = 1/x }[/math] 带入(1)，则有齐次性的另一种表达式：如果[math]\displaystyle{ f (x, y, z, \ldots) }[/math]满足关系：

[math]\displaystyle{ f(x, y, z, \ldots) = x^nf(1, y/x, z/x, \ldots) \equiv x^n\phi(y/x, z/x, \ldots); }[/math]

(2)

则它是 [math]\displaystyle{ x, y, z, \ldots }[/math] 的 [math]\displaystyle{ n }[/math] 次齐次函数。

i.e., the [math]\displaystyle{ n^{th} }[/math] power of [math]\displaystyle{ x }[/math] times some function [math]\displaystyle{ \phi }[/math] of the ratios [math]\displaystyle{ y/x, z/x, \ldots }[/math] alone.

即等于 [math]\displaystyle{ x }[/math] 的 [math]\displaystyle{ n }[/math] 次方乘以某个以比值 [math]\displaystyle{ y/x, z/x, \ldots }[/math] 为变量的函数 [math]\displaystyle{ \phi }[/math]。

If [math]\displaystyle{ f (x, y, z, \ldots) }[/math] is homogeneous of degree [math]\displaystyle{ n }[/math] in [math]\displaystyle{ x, y, z, \ldots }[/math] it satisfies Euler's theorem :

[math]\displaystyle{ \label{eq:3} x\frac{\partial f}{\partial x}+y\frac{\partial f}{\partial y}+z\frac{\partial f}{\partial z}+\cdots \equiv nf. }[/math]

如果[math]\displaystyle{ f (x, y, z, \ldots) }[/math] 对 [math]\displaystyle{ x, y, z, \ldots }[/math] 是 [math]\displaystyle{ n }[/math] 次齐次的，则它满足欧拉定理：

[math]\displaystyle{ x\frac{\partial f}{\partial x}+y\frac{\partial f}{\partial y}+z\frac{\partial f}{\partial z}+\cdots \equiv nf. }[/math]

(3)

In thermodynamics, if the scale of a system is merely increased by a factor [math]\displaystyle{ \lambda }[/math] with no change in its intensive properties, then all its extensive properties including its entropy [math]\displaystyle{ S\ , }[/math] energy [math]\displaystyle{ E\ , }[/math] volume [math]\displaystyle{ V\ , }[/math] and the masses [math]\displaystyle{ m_1, m_2, \ldots }[/math] of each of its chemical constituents are increased by that factor, so the extensive function [math]\displaystyle{ S(E, V, m_1, m_2, \ldots) }[/math] is homogeneous of degree 1 in its extensive arguments:

[math]\displaystyle{ \label{eq:4} S(\lambda E, \lambda V, \lambda {m_1}, \lambda {m_2}, \ldots ) = \lambda S(E, V, {m_1}, {m_2}, \ldots). }[/math]

在热力学 Thermodynamics中，如果一个系统的标度增加 [math]\displaystyle{ \lambda }[/math] 倍而其强度量不发生变化，仅是该系统所有化学组分的广度量（如熵 [math]\displaystyle{ S\ }[/math]，能量 [math]\displaystyle{ E\ }[/math]，体积 [math]\displaystyle{ V\ }[/math]和质量 [math]\displaystyle{ m_1, m_2, \ldots }[/math] 等）也增加相同倍数。则有广度函数 [math]\displaystyle{ S(E, V, m_1, m_2, \ldots) }[/math] 在广义论证中满足齐次关系：

[math]\displaystyle{ S(\lambda E, \lambda V, \lambda {m_1}, \lambda {m_2}, \ldots ) = \lambda S(E, V, {m_1}, {m_2}, \ldots). }[/math]

(4)

With [math]\displaystyle{ T }[/math] the temperature, [math]\displaystyle{ p }[/math] the pressure, and [math]\displaystyle{ \mu_i }[/math] the chemical potential of the species [math]\displaystyle{ i\ , }[/math] we have the thermodynamic relations [math]\displaystyle{ \partial S/\partial E = 1/T\ , }[/math] [math]\displaystyle{ \partial S/\partial V = p/T\ , }[/math] and [math]\displaystyle{ \partial S/\partial m_i = - \mu_i/T\ ; }[/math] so from Euler's theorem,

[math]\displaystyle{ \label{eq:5} \frac{1}{T} (E + pV - \mu_1m_1 - \mu_2m_2 - \cdots) =S, }[/math]

以 [math]\displaystyle{ T }[/math]，[math]\displaystyle{ p }[/math]，[math]\displaystyle{ \mu_i }[/math] 分别表示温度，压力和不同组分 [math]\displaystyle{ i\ }[/math]的化学势，根据热力学关系 [math]\displaystyle{ \partial S/\partial E = 1/T\ }[/math]， [math]\displaystyle{ \partial S/\partial V = p/T\ }[/math]，和 [math]\displaystyle{ \partial S/\partial m_i = - \mu_i/T\ }[/math]：再由欧拉定理可得：

[math]\displaystyle{ \frac{1}{T} (E + pV - \mu_1m_1 - \mu_2m_2 - \cdots) =S, }[/math]

(5)

an important identity. Any extensive function [math]\displaystyle{ X(T, p, m_1, m_2, \ldots)\ , }[/math] such as the volume V or the Gibbs free energy [math]\displaystyle{ E+pV-TS\ , }[/math] is homogeneous of the first degree in the [math]\displaystyle{ m_i }[/math] at fixed [math]\displaystyle{ p }[/math] and [math]\displaystyle{ T\ , }[/math] so

[math]\displaystyle{ \label{eq:6} X = m_1 \frac{\partial X}{\partial m_1} + m_2 \frac{\partial X}{\partial m_2} + \cdots , }[/math]

任何广度函数 [math]\displaystyle{ X(T, p, m_1, m_2, \ldots)\ }[/math]（如体积 [math]\displaystyle{ V\ }[/math]或者吉布斯自由能[math]\displaystyle{ E+pV-TS\ }[/math]）在等温等压状态下，对 [math]\displaystyle{ m_i }[/math] 都是一次齐次的，因此：

[math]\displaystyle{ X = m_1 \frac{\partial X}{\partial m_1} + m_2 \frac{\partial X}{\partial m_2} + \cdots , }[/math]

(6)

an important class of relations.

是重要的一类关系。

The foregoing are scaling relations in classical thermodynamics. In more recent times, in statistical mechanics, the expression "scaling laws" has been taken to refer to the homogeneity of form of the thermodynamic and correlation functions near critical points, and to the resulting relations among the exponents that occur in those functions. There are many excellent references for critical phenomena and the associated scaling laws, among them the superb book by Domb [1] and the historic early review by Fisher [2].

引言交代了经典热力学语境下的标度关系。在其后发展出的统计力学中，“标度律”一词指代热力学函数和相关函数在临界点附近的齐次形式，以及这些函数中指数之间的关系。

Near the Curie point (critical point) of a ferromagnet, which occurs at [math]\displaystyle{ T = T_c\ ， }[/math] the magnetic field [math]\displaystyle{ H\ , }[/math] magnetization [math]\displaystyle{ M\ , }[/math] and [math]\displaystyle{ t = T/T_c-1\ , }[/math] are related by

[math]\displaystyle{ \label{eq:7} H = M\mid M\mid ^{\delta-1} j(t/\mid M\mid ^{1/\beta}) }[/math]

当 [math]\displaystyle{ t = T/T_c-1\ }[/math]时，铁磁物质临近居里点（临界点），磁场强度 [math]\displaystyle{ H\ }[/math]，磁化强度 [math]\displaystyle{ M\ }[/math]以及 [math]\displaystyle{ t = T/T_c-1\ }[/math]满足

[math]\displaystyle{ H = M\mid M\mid ^{\delta-1} j(t/\mid M\mid ^{1/\beta}) }[/math]

(7)

where [math]\displaystyle{ j(x) }[/math] is the "scaling" function and [math]\displaystyle{ \beta }[/math] and [math]\displaystyle{ \delta }[/math] are two critical-point exponents [3-7]. Thus, from (2) and (7), as the critical point is approached [math]\displaystyle{ (H\rightarrow 0 }[/math] and [math]\displaystyle{ t\rightarrow 0)\ , }[/math] [math]\displaystyle{ \mid H\mid }[/math] becomes a homogeneous function of [math]\displaystyle{ t }[/math] and [math]\displaystyle{ \mid M\mid ^{1/\beta} }[/math] of degree [math]\displaystyle{ \beta \delta\ . }[/math] The scaling function [math]\displaystyle{ j(x) }[/math] vanishes proportionally to [math]\displaystyle{ x+b }[/math] as [math]\displaystyle{ x }[/math] approaches [math]\displaystyle{ -b\ , }[/math] with [math]\displaystyle{ b }[/math] a positive constant; it diverges proportionally to [math]\displaystyle{ x^{\beta(\delta-1)} }[/math] as [math]\displaystyle{ x\rightarrow \infty\ ; }[/math] and [math]\displaystyle{ j(0) = c\ , }[/math] another positive constant (Fig. 1). Although (7) is confined to the immediate neighborhood of the critical point [math]\displaystyle{ (t, M, H }[/math] all near 0), the scaling variable [math]\displaystyle{ x = t/\mid M\mid ^{1/\beta} }[/math] nevertheless traverses the infinite range [math]\displaystyle{ -b \lt x \lt \infty\ . }[/math]

其中 [math]\displaystyle{ j(x) }[/math] 是“标度”函数，[math]\displaystyle{ \beta }[/math] 和[math]\displaystyle{ \delta }[/math] 是临界点指数。因此由(2)和(7)，当铁磁物质趋近于临界点时[math]\displaystyle{ (H\rightarrow 0 }[/math] 且 [math]\displaystyle{ t\rightarrow 0)\ }[/math]，[math]\displaystyle{ \mid H\mid }[/math] 是 [math]\displaystyle{ t }[/math] 和 [math]\displaystyle{ \mid M\mid ^{1/\beta} }[/math] 的 [math]\displaystyle{ \beta \delta\ }[/math] 次齐次函数。当 [math]\displaystyle{ x }[/math] 趋近于[math]\displaystyle{ -b\ }[/math]（正常数）时，标度函数 [math]\displaystyle{ j(x) }[/math] 趋近于零；当 [math]\displaystyle{ x\rightarrow \infty\ }[/math]时，它依 [math]\displaystyle{ x^{\beta(\delta-1)} }[/math] 成比例发散（如图一），且 [math]\displaystyle{ j(0) = c\ }[/math]（正常数）。尽管(7)局限在临界点[math]\displaystyle{ (t, M, H }[/math] 都接近零）附近的极小范围内，但标度变量 [math]\displaystyle{ x = t/\mid M\mid ^{1/\beta} }[/math]却遍历[math]\displaystyle{ -b \lt x \lt \infty\ }[/math]的无穷范围。

Scaling function [math]\displaystyle{ j(x) }[/math]

When [math]\displaystyle{ \mid H\mid = 0+ }[/math] and [math]\displaystyle{ t\lt 0\ , }[/math] so that [math]\displaystyle{ M }[/math] is then the spontaneous magnetization, we have from (7), [math]\displaystyle{ \mid M\mid = (-\frac{t}{b})^\beta\ , }[/math] where [math]\displaystyle{ \beta }[/math] is the conventional symbol for this critical-point exponent. When [math]\displaystyle{ M\rightarrow 0 }[/math] on the critical isotherm [math]\displaystyle{ (t=0)\ , }[/math] we have [math]\displaystyle{ H \sim cM\mid M\mid ^{\delta-1}\ , }[/math] where [math]\displaystyle{ \delta }[/math] is the conventional symbol for this exponent. From the first of the two properties of [math]\displaystyle{ j(x) }[/math] noted above, and Eq.(7), one may calculate the magnetic susceptibility [math]\displaystyle{ (\partial M/\partial H)_T\ , }[/math] which is then seen to diverge proportionally to [math]\displaystyle{ \mid t\mid ^{-\beta(\delta-1)}\ , }[/math] both at [math]\displaystyle{ \mid H\mid = 0+ }[/math] with [math]\displaystyle{ t\lt 0 }[/math] and at [math]\displaystyle{ H=0 }[/math] with [math]\displaystyle{ t\gt 0 }[/math] (although with different coefficients). The conventional symbol for the susceptibility exponent is [math]\displaystyle{ \gamma\ , }[/math] so we have [8]

[math]\displaystyle{ \label{eq:8} \gamma = \beta(\delta-1). }[/math]

Equations (7) and (8) are examples of scaling laws, Eq.(7) being a statement of homogeneity and the exponent relation (8) a consequence of that homogeneity.

当[math]\displaystyle{ \mid H\mid = 0+ }[/math] 且 [math]\displaystyle{ t\lt 0\ }[/math]，[math]\displaystyle{ M }[/math] 是自发磁化率，由(7)可得[math]\displaystyle{ \mid M\mid = (-\frac{t}{b})^\beta\ }[/math]，其中 [math]\displaystyle{ \beta }[/math] 为临界指数。在临界等温线[math]\displaystyle{ (t=0)\ }[/math]，当 [math]\displaystyle{ M\rightarrow 0 }[/math] 时，我们有[math]\displaystyle{ H \sim cM\mid M\mid ^{\delta-1}\ }[/math]，其中 [math]\displaystyle{ \delta }[/math] 为此时的临界指数。由前文中 [math]\displaystyle{ j(x) }[/math] 的第一个性质和(7)式，我们可以计算磁化率 [math]\displaystyle{ (\partial M/\partial H)_T\ }[/math]，它在[math]\displaystyle{ \mid H\mid = 0+ }[/math] 且 [math]\displaystyle{ t\lt 0 }[/math]，以及在 [math]\displaystyle{ H=0 }[/math]且[math]\displaystyle{ t\gt 0 }[/math] 时依 [math]\displaystyle{ \mid t\mid ^{-\beta(\delta-1)}\ }[/math]成比例发散（尽管系数不同）。磁化率指数的常用符号是 [math]\displaystyle{ \gamma\ }[/math]，因此有

[math]\displaystyle{ \gamma = \beta(\delta-1). }[/math]

(8)

方程(7)和(8)都是标度律的范例，(7)是齐次性的表述，(8)作为指数关系式则是这种齐次性的结果。

A free energy [math]\displaystyle{ F }[/math] may be obtained from (7) by integrating at fixed temperature, since [math]\displaystyle{ M = -(\partial F/\partial H)_T\ , }[/math] and the corresponding heat capacity [math]\displaystyle{ C_H }[/math] then follows from [math]\displaystyle{ C_H = -(\partial ^2 F/\partial T^2)_H\ . }[/math] One then finds from (7) that [math]\displaystyle{ C_H }[/math] at [math]\displaystyle{ H=0 }[/math] diverges at the critical point proportionally to [math]\displaystyle{ \mid t\mid ^{-\alpha} }[/math] (with different coefficients for [math]\displaystyle{ t\rightarrow 0- }[/math] and [math]\displaystyle{ t\rightarrow 0+)\ , }[/math] with the critical-point exponent [math]\displaystyle{ \alpha }[/math] related to [math]\displaystyle{ \beta }[/math] and [math]\displaystyle{ \gamma }[/math] by the scaling law [9]

[math]\displaystyle{ \label{eq:9} \alpha +2\beta +\gamma=2. }[/math]

由于[math]\displaystyle{ M = -(\partial F/\partial H)_T\ }[/math]，等温条件下自由能 [math]\displaystyle{ F }[/math] 可以通过积分由(7)式得出，且相应的热容 [math]\displaystyle{ C_H = -(\partial ^2 F/\partial T^2)_H\ }[/math]。由(7)式可知，在[math]\displaystyle{ H=0 }[/math] 时 [math]\displaystyle{ C_H }[/math] 在临界点处依 [math]\displaystyle{ \mid t\mid ^{-\alpha} }[/math] 比例发散（其中 [math]\displaystyle{ t\rightarrow 0- }[/math] 和 [math]\displaystyle{ t\rightarrow 0+ }[/math] 各有不同的系数），临界点指数 [math]\displaystyle{ \alpha }[/math] 与[math]\displaystyle{ \beta }[/math] 和 [math]\displaystyle{ \gamma }[/math] 满足以下标度律：

[math]\displaystyle{ \alpha +2\beta +\gamma=2. }[/math]

(9)

When [math]\displaystyle{ 2\beta+\gamma=2 }[/math] the resulting [math]\displaystyle{ \alpha =0 }[/math] means, generally, a logarithmic rather than power-law divergence together with a superimposed finite discontinuity occurring between [math]\displaystyle{ t=0+ }[/math] and [math]\displaystyle{ t=0- }[/math] [4]. In the 2-dimensional Ising model the discontinuity is absent and only the logarithm remains, while in mean-field (van der Waals, Curie-Weiss, Bragg-Williams) approximation the logarithm is absent but the discontinuity is still present.

当 [math]\displaystyle{ 2\beta+\gamma=2 }[/math]，则有 [math]\displaystyle{ \alpha =0 }[/math]，这通常意味着对数发散而不是幂律发散，并且在 [math]\displaystyle{ t=0+ }[/math] 和 [math]\displaystyle{ t=0- }[/math] 之间存在叠加有限不连续。在二维伊辛模型中，仅有对数关系而这种不连续是不存在的；而在平均场近似中情形相反。

Critical exponents 临界指数

What were probably the historically earliest versions of critical-point exponent relations like (8) and (9) are due to Rice [10] and to Scott [11]. It was before Domb and Sykes [12] and Fisher [13] had noted that the exponent [math]\displaystyle{ \gamma }[/math] was in reality greater than its mean-field value [math]\displaystyle{ \gamma =1 }[/math] but when it was already clear from Guggenheim's corresponding-states analysis [14] that [math]\displaystyle{ \beta }[/math] had a value much closer to 1/3 than to its mean-field value of 1/2. Then, under the assumption [math]\displaystyle{ \gamma =1 }[/math] and [math]\displaystyle{ \beta \simeq 1/3\ , }[/math] Rice had concluded from the equivalent of (8) that [math]\displaystyle{ \delta = 1+1/\beta \simeq 4 }[/math] (the correct value is now known to be closer to 5) and Scott had concluded from the equivalent of (9) that [math]\displaystyle{ \alpha =1-2\beta \simeq 1/3 }[/math] (the correct value is now known to be closer to 1/10). The mean-field values are [math]\displaystyle{ \delta =3 }[/math] and (as noted above) [math]\displaystyle{ \alpha =0\ . }[/math]

(8)和(9)分别来自里斯和斯考特的贡献。它们大概是历史上最早版本的临界指数关系。在此之后，Domb和Sykes以及Fisher注意到指数 [math]\displaystyle{ \gamma }[/math] 实际上比平均场值[math]\displaystyle{ \gamma =1 }[/math] 大。而在更早之前，Guggenheim的对应状态分析就清楚地表明 [math]\displaystyle{ \beta }[/math]值更靠近1/3而非平均场值的1/2。之后在 [math]\displaystyle{ \gamma =1 }[/math] 和 [math]\displaystyle{ \beta \simeq 1/3\ }[/math]的假设下，里斯由(8)式总结出 [math]\displaystyle{ \delta = 1+1/\beta \simeq 4 }[/math]（如今已知正确值接近5）。同时斯考特由(9)式得出 [math]\displaystyle{ \alpha =1-2\beta \simeq 1/3 }[/math]（正确值接近1/10）。另外平均场值 [math]\displaystyle{ \delta =3 }[/math]，[math]\displaystyle{ \alpha =0\ }[/math]。

The long-range spatial correlation functions in ferromagnets and fluids also exhibit a homogeneity of form near the critical point. At magnetic field [math]\displaystyle{ H=0 }[/math] (assumed for simplicity) the correlation function [math]\displaystyle{ h(r,t) }[/math] as a function of the spatial separation [math]\displaystyle{ r }[/math] (assumed very large) and temperature near the critical point (t assumed very small), is of the form [5,15]

[math]\displaystyle{ \label{eq:10} h(r,t)=r^{-(d-2+\eta)}G(r/\xi). }[/math]

铁磁体和流体中的长程空间相关函数在临界点附近也表现出齐次性。简单起见，考虑磁场强度 [math]\displaystyle{ H=0 }[/math] 且温度接近临界点的情况，关联函数 [math]\displaystyle{ h(r,t) }[/math] 作为空间分离 [math]\displaystyle{ r }[/math]（假设很大）的函数，如下所示：

[math]\displaystyle{ h(r,t)=r^{-(d-2+\eta)}G(r/\xi). }[/math]

(10)

Here [math]\displaystyle{ d }[/math] is the dimensionality of space, [math]\displaystyle{ \eta }[/math] is another critical-point exponent, and [math]\displaystyle{ \xi }[/math] is the correlation length (exponential decay length of the correlations), which diverges as

[math]\displaystyle{ \label{eq:11} \xi\sim \mid t\mid ^{-\nu} }[/math]

其中 [math]\displaystyle{ d }[/math] 是空间维度，[math]\displaystyle{ \eta }[/math] 是另一临界点指数，[math]\displaystyle{ \xi }[/math] 是关联长度（相关关系的指数衰减长度），当趋近于临界点时，其发散过程满足：

[math]\displaystyle{ \xi\sim \mid t\mid ^{-\nu} }[/math]

(11)

as the critical point is approached, with [math]\displaystyle{ \nu }[/math] still another critical-point exponent. Thus, [math]\displaystyle{ h(r,t) }[/math] (with [math]\displaystyle{ H=0) }[/math] is a homogeneous function of [math]\displaystyle{ r }[/math] and [math]\displaystyle{ \mid t\mid ^{-\nu} }[/math] of degree [math]\displaystyle{ -(d-2+\eta)\ . }[/math] The scaling function [math]\displaystyle{ G(x) }[/math] has the properties (to within constant factors of proportionality),

[math]\displaystyle{ \label{eq:12} G(x) \sim \left\{ \begin{array} {lc }x^{\frac{1}{2}(d-3)+\eta} e^{-x}, & x\rightarrow \infty \\ 1, & x\rightarrow 0 . \end{array} \right. }[/math]

其中 [math]\displaystyle{ \nu }[/math] 是另外的临界指数。因此 [math]\displaystyle{ h(r,t) }[/math]（[math]\displaystyle{ H=0 }[/math]）是 [math]\displaystyle{ r }[/math] 和 [math]\displaystyle{ \mid t\mid ^{-\nu} }[/math] 的[math]\displaystyle{ -(d-2+\eta)\ }[/math]次齐次方程。标度函数 [math]\displaystyle{ G(x) }[/math] 具有以下性质(在常数比例因子范围内)：

[math]\displaystyle{ G(x) \sim \left\{ \begin{array} {lc }x^{\frac{1}{2}(d-3)+\eta} e^{-x}, & x\rightarrow \infty \\ 1, & x\rightarrow 0 . \end{array} \right. }[/math]

(12)

Thus, as [math]\displaystyle{ r\rightarrow \infty }[/math] in any fixed thermodynamic state (fixed t) near the critical point, [math]\displaystyle{ h }[/math] decays with increasing [math]\displaystyle{ r }[/math] proportionally to [math]\displaystyle{ r^{-\frac{1}{2}(d-1)}e^{-r/\xi}\ , }[/math] as in the Ornstein-Zernike theory. If, instead, the critical point is approached [math]\displaystyle{ (\xi \rightarrow \infty) }[/math] with a fixed, large [math]\displaystyle{ r\ , }[/math] we have [math]\displaystyle{ h(r) }[/math] decaying with [math]\displaystyle{ r }[/math] only as an inverse power, [math]\displaystyle{ r^{-(d-2+\eta)}\ , }[/math] which corrects the [math]\displaystyle{ r^{-(d-2)} }[/math] that appears in the Ornstein-Zernike theory in that limit. The scaling law(10) with scaling function [math]\displaystyle{ G(x) }[/math] interpolates between these extremes.

因此，在任何靠近临界点的恒温热力学状态下，当 [math]\displaystyle{ r\rightarrow \infty }[/math] 时，[math]\displaystyle{ h }[/math] 随[math]\displaystyle{ r }[/math] 的增加依 [math]\displaystyle{ r^{-\frac{1}{2}(d-1)}e^{-r/\xi}\ }[/math] 成比例衰减（参见奥恩斯泰因-泽尔尼克理论 Ornstein-Zernike theory）。如果相反，在固定的大 [math]\displaystyle{ r\ }[/math] 条件下，迫近临界点（[math]\displaystyle{ \xi \rightarrow \infty }[/math]），会有 [math]\displaystyle{ h(r) }[/math] 作为逆幂 [math]\displaystyle{ r^{-(d-2+\eta)}\ }[/math] 随 [math]\displaystyle{ r }[/math] 衰减，这也修正了在此极限条件下奥恩斯泰因-泽尔尼克理论中出现的 [math]\displaystyle{ r^{-(d-2)} }[/math]。标度律(10)及标度函数 [math]\displaystyle{ G(x) }[/math] 内插于这些极限之间。

In the language of fluids, with [math]\displaystyle{ \rho }[/math] the number density and [math]\displaystyle{ \chi }[/math] the isothermal compressibility, we have as an exact relation in the Ornstein-Zernike theory

[math]\displaystyle{ \label{eq:13} \rho kT \chi =1+\rho \int h(r) \rm{d}\tau }[/math]

在流体研究中，由数密度 [math]\displaystyle{ \rho }[/math] 和等温压缩率 [math]\displaystyle{ \chi }[/math]，我们可以得到一个奥恩斯泰因-泽尔尼克理论的精确表达式：

[math]\displaystyle{ \rho kT \chi =1+\rho \int h(r) \rm{d}\tau }[/math]

(13)

with [math]\displaystyle{ k }[/math] Boltzmann's constant and where the integral is over all space with [math]\displaystyle{ \rm{d} \tau }[/math] the element of volume. The same relation holds in the ferromagnets with [math]\displaystyle{ \chi }[/math] then the magnetic susceptibility and with the deviation of [math]\displaystyle{ \rho }[/math] from the critical density [math]\displaystyle{ \rho_c }[/math] then the magnetization [math]\displaystyle{ M\ . }[/math] At the critical point [math]\displaystyle{ \chi }[/math] is infinite and correspondingly the integral diverges because the decay length [math]\displaystyle{ \xi }[/math] is then also infinite. The density [math]\displaystyle{ \rho }[/math] is there just the finite positive constant [math]\displaystyle{ \rho_c }[/math] and [math]\displaystyle{ T }[/math] the finite [math]\displaystyle{ T_c\ . }[/math] Then from the scaling law (10), because of the homogeneity of [math]\displaystyle{ h(r,t) }[/math] and because the main contribution to the diverging integral comes from large [math]\displaystyle{ r\ , }[/math] where (10) holds, it follows that [math]\displaystyle{ \chi }[/math] diverges proportionally to [math]\displaystyle{ \xi^{2-\eta} \int G(x)x^{d-1}\rm{d} }[/math][math]\displaystyle{ x\ . }[/math] But the integral is now finite because, by (12), [math]\displaystyle{ G(x) }[/math] vanishes exponentially rapidly as [math]\displaystyle{ x\rightarrow \infty\ . }[/math] Thus, from (11) and from the earlier [math]\displaystyle{ \chi \sim \mid t\mid^{-\gamma} }[/math] we have the scaling law [15]

[math]\displaystyle{ \label{eq:14} (2-\eta)\nu = \gamma . }[/math]

其中 [math]\displaystyle{ k }[/math] 是玻尔兹曼常数，[math]\displaystyle{ \rm{d} \tau }[/math] 是体积元，积分区域是整个空间。对铁磁体也有相同的关系成立，包含磁化率 [math]\displaystyle{ \chi }[/math]，[math]\displaystyle{ \rho }[/math] 与临界密度 [math]\displaystyle{ \rho_c }[/math] 的差值，以及磁化强度 [math]\displaystyle{ M\ }[/math]。在临界点处，[math]\displaystyle{ \chi }[/math] 无穷大，且对应积分式也发散，因为衰减长度 [math]\displaystyle{ \xi }[/math] 也是无穷大的。而密度 [math]\displaystyle{ \rho }[/math]为有限正常数 [math]\displaystyle{ \rho_c }[/math]，[math]\displaystyle{ T }[/math] 为 [math]\displaystyle{ T_c\ }[/math]。

[math]\displaystyle{ (2-\eta)\nu = \gamma . }[/math]

(14)

The surface tension [math]\displaystyle{ \sigma }[/math] in liquid-vapor equilibrium, or the analogous excess free energy per unit area of the interface between coexisting, oppositely magnetized domains, vanishes at the critical point (Curie point) proportionally to [math]\displaystyle{ (-t)^\mu }[/math] with [math]\displaystyle{ \mu }[/math] another critical-point exponent. The interfacial region has a thickness of the order of the correlation length [math]\displaystyle{ \xi }[/math] so [math]\displaystyle{ \sigma/\xi }[/math] is the free energy per unit volume associated with the interfacial region. That is in its magnitude and in its singular critical-point behavior the same free energy per unit volume as in the bulk phases, from which the heat capacity follows by two differentiations with respect to the temperature. Thus, [math]\displaystyle{ \sigma/\xi }[/math] vanishes proportionally to [math]\displaystyle{ (-t)^{2-\alpha}\ ; }[/math] so, together with (9),

[math]\displaystyle{ \label{eq:15} \mu + \nu = 2-\alpha= \gamma +2\beta, }[/math]

液-气平衡时的表面张力 [math]\displaystyle{ \sigma }[/math]，或共存的、相反磁化畴之间的界面单位面积上的类似过剩自由能，在临界点(居里点)与 [math]\displaystyle{ (-t)^\mu }[/math]（[math]\displaystyle{ \mu }[/math]对应此处临界点指数）成比例消失。界面区域的厚度与关联长度 [math]\displaystyle{ \xi }[/math] 的数量级相当，因此 [math]\displaystyle{ \sigma/\xi }[/math] 是与界面区域相关的单位体积自由能。在它的大小和它的奇异临界点行为中，每单位体积的自由能和在体相中是一样的，从体相中，依据关于温度的两个微分可以得出热容。因此，[math]\displaystyle{ \sigma/\xi }[/math] 依 [math]\displaystyle{ (-t)^{2-\alpha}\ }[/math] 成比例消失；再联系(9)式可以得到另一个标度关系：

[math]\displaystyle{ \mu + \nu = 2-\alpha= \gamma +2\beta, }[/math]

(15)

another scaling relation [16,17].

Exponents and space dimension 临界点指数与空间维度

The critical-point exponents depend on the dimensionality [math]\displaystyle{ d\ . }[/math] The theory was found to be illuminated by treating [math]\displaystyle{ d }[/math] as continuously variable and of any magnitude. There is a class of critical-point exponent relations, often referred to as hyperscaling, in which [math]\displaystyle{ d }[/math] appears explicitly. The correlation length [math]\displaystyle{ \xi }[/math] is the coherence length of density or magnetization fluctuations. What determines its magnitude is that the excess free energy associated with the spontaneous fluctuations in the volume [math]\displaystyle{ \xi ^d }[/math] must be of order [math]\displaystyle{ kT\ , }[/math] which has the finite value [math]\displaystyle{ kT_c }[/math] at the critical point. But the typical fluctuations that occur in such an elemental volume are just such as to produce the conjugate phase. The free energy [math]\displaystyle{ kT }[/math] is then that for creating an interface of area [math]\displaystyle{ \xi^{d-1}\ , }[/math] which is [math]\displaystyle{ \sigma \xi^{d-1}\ . }[/math] Thus, as the critical point is approached [math]\displaystyle{ \sigma \xi^{d-1} }[/math] has a finite limit of order [math]\displaystyle{ kT_c\ . }[/math] Then from the definitions of the exponents [math]\displaystyle{ \mu }[/math] and [math]\displaystyle{ \nu\ , }[/math]

[math]\displaystyle{ \label{eq:16} \mu = (d-1)\nu, }[/math]

临界点指数取决于维数 [math]\displaystyle{ d\ }[/math]。人们发现，将 [math]\displaystyle{ d }[/math] 视为具有任意大小的连续变量可以解释说明这一观点。在一类被称为超标度的临界点指数关系中，可以清楚地看到 [math]\displaystyle{ d }[/math]。关联长度 [math]\displaystyle{ \xi }[/math] 为密度或磁化波动的相干长度。决定其大小的是体积 [math]\displaystyle{ \xi ^d }[/math] 中与自发波动有关的过剩自由能，且一定是 [math]\displaystyle{ kT\ }[/math] 阶的，在临界点处具有有限值 [math]\displaystyle{ kT_c }[/math] 。但在这样的微元体中，典型的波动只会产生共轭相。则自由能 [math]\displaystyle{ kT }[/math] 为创建区域 [math]\displaystyle{ \xi^{d-1}\ }[/math]的界面 [math]\displaystyle{ \sigma \xi^{d-1}\ }[/math]的自由能。因此，当接近临界点时，[math]\displaystyle{ \sigma \xi^{d-1} }[/math] 具有 [math]\displaystyle{ kT_c\ }[/math] 阶的有限极限。再由指数 [math]\displaystyle{ \mu }[/math] 和 [math]\displaystyle{ \nu\ }[/math]的定义可得超标度关系：

[math]\displaystyle{ \mu = (d-1)\nu, }[/math]

(16)

a hyperscaling relation [16]. With (15) we then have also [16]

[math]\displaystyle{ \label{eq:17} d\nu = 2-\alpha = \gamma+2\beta, }[/math]

再由(15)有：

[math]\displaystyle{ d\nu = 2-\alpha = \gamma+2\beta, }[/math]

(17)

which, with (8) and (14), yields also [18]

[math]\displaystyle{ \label{eq:18} 2-\eta = \frac{\delta -1}{\delta +1} d. }[/math]

结合(8)和(14)得到：

[math]\displaystyle{ 2-\eta = \frac{\delta -1}{\delta +1} d. }[/math]

(18)

Unlike the scaling laws (8), (9), (14), and (15), which make no explicit reference to the dimensionality, the [math]\displaystyle{ d }[/math]-dependent exponent relations (16)-(18) hold only for [math]\displaystyle{ d\lt 4\ . }[/math] At [math]\displaystyle{ d=4 }[/math] the exponents assume the values they have in the mean-field theories but logarithmic factors are then appended to the simple power laws. Then for [math]\displaystyle{ d\gt 4\ , }[/math] the terms in the thermodynamic functions and correlation-function parameters that have as their exponents those given by the mean-field theories are the leading terms. The terms with the original [math]\displaystyle{ d }[/math]-dependent exponents, which for [math]\displaystyle{ d\lt 4 }[/math] were the leading terms, have been overtaken, and, while still present, are now sub-dominant.

标度律(8)，(9)，(14)和(15)没有明显的和空间维数相联系，而(16)-(18)则是依赖于 [math]\displaystyle{ d }[/math] 的指数关系式，且仅对 [math]\displaystyle{ d\lt 4\ }[/math]成立。对于 [math]\displaystyle{ d=4 }[/math]，热力学函数中依据平均场理论给出的以相关函数参数为指数的项是主导项。而本身在 [math]\displaystyle{ d\lt 4 }[/math] 时，包含依赖于 [math]\displaystyle{ d }[/math] 的指数的主导项，虽然依然存在，但是已经被取代而变成次要项。

This progression in critical-point properties from [math]\displaystyle{ d\lt 4 }[/math] to [math]\displaystyle{ d=4 }[/math] to [math]\displaystyle{ d\gt 4 }[/math] is seen clearly in the phase transition that occurs in the analytically soluble model of the ideal Bose gas. There is no phase transition or critical point in it for [math]\displaystyle{ d \le 2\ . }[/math] When [math]\displaystyle{ d\gt 2 }[/math] the chemical potential [math]\displaystyle{ \mu }[/math] (not to be confused with the surface-tension exponent [math]\displaystyle{ \mu }[/math]) vanishes identically for all [math]\displaystyle{ \rho \Lambda ^d \ge \zeta (d/2)\ , }[/math] where [math]\displaystyle{ \rho }[/math] is the density, [math]\displaystyle{ \Lambda }[/math] is the thermal de Broglie wavelength [math]\displaystyle{ h/\sqrt {2\pi mkT} }[/math] with [math]\displaystyle{ h }[/math] Planck's constant and [math]\displaystyle{ m }[/math] the mass of the atom, and [math]\displaystyle{ \zeta (s) }[/math] is the Riemann zeta function. As [math]\displaystyle{ \rho \Lambda^d \rightarrow \zeta(d/2) }[/math] from below, [math]\displaystyle{ \mu }[/math] vanishes through a range of negative values. As [math]\displaystyle{ \mu \rightarrow 0-\ , }[/math] the difference [math]\displaystyle{ \zeta(d/2)-\rho \Lambda^d }[/math] vanishes (to within positive proportionality factors) as

[math]\displaystyle{ \label{eq:19} \zeta(d/2)-\rho \Lambda^d \sim \left\{ \begin{array} {lc }(-\mu)^{d/2-1}, & 2\lt d\lt 4 \\ \\ \mu \ln(-\mu/kT), & d=4 \\ \\ -\mu , & d\gt 4 . \end{array}\right. }[/math]

在理想玻色气体的解析溶解模型中，可以清楚地看到临界点性质从 [math]\displaystyle{ d\lt 4 }[/math] 到 [math]\displaystyle{ d=4 }[/math] 再到 [math]\displaystyle{ d\gt 4 }[/math] 的变化过程。在 [math]\displaystyle{ d \le 2\ }[/math] 的情形下，不存在相变或者临界点。当 [math]\displaystyle{ d\gt 2 }[/math] 时，对于所有 [math]\displaystyle{ \rho \Lambda ^d \ge \zeta (d/2)\ }[/math]，化学势 [math]\displaystyle{ \mu }[/math]（此处不要与表面张力指数 [math]\displaystyle{ \mu }[/math] 混淆）都会变为零。其中 [math]\displaystyle{ \rho }[/math] 是密度，[math]\displaystyle{ \Lambda }[/math] 是热德布罗意波长，即 [math]\displaystyle{ h/\sqrt {2\pi mkT} }[/math]（其中 [math]\displaystyle{ h }[/math] 是普朗克常数，[math]\displaystyle{ m }[/math] 是原子质量），[math]\displaystyle{ \zeta (s) }[/math] 是黎曼 [math]\displaystyle{ \zeta }[/math] 函数。当由下 [math]\displaystyle{ \rho \Lambda^d \rightarrow \zeta(d/2) }[/math] 时，[math]\displaystyle{ \mu }[/math] 从负值范围变为零。当 [math]\displaystyle{ \mu \rightarrow 0- }[/math] 时，[math]\displaystyle{ \zeta(d/2)-\rho \Lambda^d }[/math] 之差（在正比例因子内）变为零，且满足以下关系：

[math]\displaystyle{ \zeta(d/2)-\rho \Lambda^d \sim \left\{ \begin{array} {lc }(-\mu)^{d/2-1}, & 2\lt d\lt 4 \\ \\ \mu \ln(-\mu/kT), & d=4 \\ \\ -\mu , & d\gt 4 . \end{array}\right. }[/math]

(19)

When [math]\displaystyle{ 2\lt d\lt 4 }[/math] the mean-field [math]\displaystyle{ -\mu }[/math] is still present but is dominated by [math]\displaystyle{ (-\mu)^{d/2-1}\ ; }[/math] when [math]\displaystyle{ d\gt 4 }[/math] the singular [math]\displaystyle{ (-\mu)^{d/2-1} }[/math] is still present but is dominated by the mean-field [math]\displaystyle{ -\mu\ . }[/math]

当 [math]\displaystyle{ 2\lt d\lt 4 }[/math] 时，平均场指数 [math]\displaystyle{ -\mu }[/math] 依然存在，但是主导指数则是[math]\displaystyle{ (-\mu)^{d/2-1}\ }[/math]；当 [math]\displaystyle{ d\gt 4 }[/math] 时，奇异指数 [math]\displaystyle{ (-\mu)^{d/2-1} }[/math] 依然存在，但是主导指数为 [math]\displaystyle{ -\mu\ }[/math]。

This behavior is reflected again in the Renormalization-group theory [19-21]. In the simplest cases there are two competing fixed points for the renormalization-group flows, one of them associated with [math]\displaystyle{ d }[/math]-dependent exponents that satisfy both the [math]\displaystyle{ d }[/math]-independent scaling relations and the hyperscaling relations, the other with the [math]\displaystyle{ d }[/math]-independent exponents of the mean-field theories [21]. The first determines the leading critical-point behavior when [math]\displaystyle{ d\lt 4\ . }[/math] At [math]\displaystyle{ d=4 }[/math] the two fixed points coincide and the exponents are now those of the mean-field theories but with logarithmic factors appended to the mean-field power laws. For [math]\displaystyle{ d\gt 4 }[/math] the two fixed points separate again and the leading critical-point behavior now comes from the one whose exponents are those of the mean-field theories. The effects of both fixed points are present at all [math]\displaystyle{ d\ , }[/math] but the dominant critical-point behavior comes from only the one or the other, depending on [math]\displaystyle{ d\ . }[/math]

这一行为也反映在重整化群理论中。最简单的情形是，重整化群流中有两个相互竞争的不动点，一点与依赖 [math]\displaystyle{ d }[/math] 的指数相关，同时满足与 [math]\displaystyle{ d }[/math] 无关的标度关系和超标度关系，另一点则与平均场理论的 [math]\displaystyle{ d }[/math] 无关指数相关。前者决定了当 [math]\displaystyle{ d\lt 4\ }[/math] 时的主导临界点行为。[math]\displaystyle{ d=4 }[/math] 时，这两个不动点重合，指数现在是平均场理论的指数，但在平均场幂律中增加了对数因子。对于 [math]\displaystyle{ d\gt 4 }[/math]，两固定点再次分开，此时主导临界点行为源自平均场理论的指数。综上所述，两固定点产生的影响覆盖所有 [math]\displaystyle{ d\ }[/math]的取值范围，但是随着 [math]\displaystyle{ d\ }[/math]取值的变化，主导临界点行为会在二者之间切换。

Origin of homogeneity; block spins 齐次性的成因与块自旋

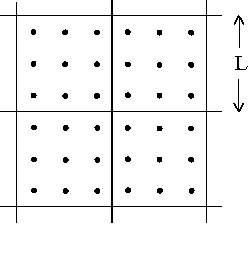
A physical explanation for the homogeneity in (7) and (10) and for the exponent relations that are consequences of them is provided by the Kadanoff block-spin picture [5], which was itself one of the inspirations for the renormalization-group theory [19,20].

来自于重整化群理论的卡丹诺夫块自旋（图2）为(7)和(10)中的齐次性以及由它们推导出的指数关系提供了物理解释。

In a lattice spin model (Ising model), one considers blocks of spins, each of linear size [math]\displaystyle{ L\ , }[/math] thus containing [math]\displaystyle{ L^d }[/math] spins, with [math]\displaystyle{ L }[/math] much less than the diverging correlation length [math]\displaystyle{ \xi }[/math] (Fig. 2).

在格子自旋模型（伊辛模型）中，假设有许多自旋块，每一个的线性尺寸为 [math]\displaystyle{ L\ }[/math]，因此包含 [math]\displaystyle{ L^d }[/math]，而 [math]\displaystyle{ L }[/math] 远小于发散关联长度 [math]\displaystyle{ \xi }[/math]（图2）。

Block spins

Each block interacts with its neighbors through their common boundary as though it were a single spin in a re-scaled model. Each block is of finite size so the spins in its interior contribute only analytic terms to the free energy of the system. The part of the free-energy density (free energy per spin) that carries the critical-point singularities and their exponents comes from the interactions between blocks. Let this free-energy density be [math]\displaystyle{ f(t,H)\ , }[/math] a function of temperature through [math]\displaystyle{ t=T/T_c-1 }[/math] and of the magnetic field [math]\displaystyle{ H\ . }[/math] The correlation length is the same in the re-scaled picture as in the original, but measured as a number of lattice spacings it is smaller in the former by the factor [math]\displaystyle{ L\ . }[/math] Thus, the re-scaled model is effectively further from its critical point than the original was from its; so with [math]\displaystyle{ H }[/math] and [math]\displaystyle{ t }[/math] both going to 0 as the critical point is approached, the effective [math]\displaystyle{ H }[/math] and [math]\displaystyle{ t }[/math] in the re-scaled model are [math]\displaystyle{ L^xH }[/math] and [math]\displaystyle{ L^yt }[/math] with positive exponents [math]\displaystyle{ x }[/math] and [math]\displaystyle{ y\ , }[/math] so increasing with [math]\displaystyle{ L\ . }[/math] From the point of view of the original model the contribution to the singular part of the free energy made by the spins in each block is [math]\displaystyle{ L^df(t,H)\ , }[/math] while that same quantity, from the point of the view of the re-scaled model, is [math]\displaystyle{ f(L^yt, L^xH)\ . }[/math] Thus,

[math]\displaystyle{ \label{eq:20} f(L^yt, L^xH) \equiv L^df(t,H); }[/math]

每一个自旋块通过与相邻块的共同边界相互作用。在对应的重标度模型中可以将它们视作单独的自旋。每个块的大小都是有限的，因此其内部的自旋只对系统的自由能提供解析项。自由能密度（单位自旋自由能）中包含临界点奇点及其指数的部分源自于自旋块间的相互作用。设自由能密度为[math]\displaystyle{ f(t,H)\ }[/math]，它是温度（由 [math]\displaystyle{ t=T/T_c-1 }[/math]）和磁场强度 [math]\displaystyle{ H\ }[/math]的函数。在重标度后的图像中，相关长度与原始图像中相同，但以格子间距的数量来度量，前者比后者小 [math]\displaystyle{ L\ }[/math]倍。因此，重标度模型实际上比原始模型离临界点更远。当逼近临界点时，[math]\displaystyle{ H }[/math] 和 [math]\displaystyle{ t }[/math]趋近于0，重标度模型中的有效 [math]\displaystyle{ H }[/math] 和[math]\displaystyle{ t }[/math] 为 [math]\displaystyle{ L^xH }[/math] 和 [math]\displaystyle{ L^yt }[/math]，其中 [math]\displaystyle{ x }[/math] 和 [math]\displaystyle{ y\ , }[/math] 是正指数。从原始模型的角度来看，每个块的自旋对自由能奇异部分的贡献是 [math]\displaystyle{ L^df(t,H)\ }[/math]，而对重标度模型来说，则是 [math]\displaystyle{ f(L^yt, L^xH)\ }[/math]。因此有：

[math]\displaystyle{ f(L^yt, L^xH) \equiv L^df(t,H); }[/math]

(20)

i.e., by (1), [math]\displaystyle{ f(t,H) }[/math] is a homogeneous function of [math]\displaystyle{ t }[/math] and [math]\displaystyle{ H^{y/x} }[/math] of degree [math]\displaystyle{ d/y\ . }[/math]

由(1)可得，[math]\displaystyle{ f(t,H) }[/math] 是 [math]\displaystyle{ t }[/math] 和 [math]\displaystyle{ H^{y/x} }[/math] 的 [math]\displaystyle{ d/y\ }[/math]次齐次函数。

Therefore, by (2), [math]\displaystyle{ f(t,H)=t^{d/y} \phi(H^{y/x}/t)=H^{d/x}\psi(t/H^{y/x}) }[/math] where [math]\displaystyle{ \phi }[/math] and [math]\displaystyle{ \psi }[/math] are functions only of the ratio [math]\displaystyle{ H^{y/x}/t\ . }[/math] At [math]\displaystyle{ H=0 }[/math] the first of these gives [math]\displaystyle{ f(t,0)=\phi(0)t^{d/y}\ . }[/math] But two temperature derivatives of [math]\displaystyle{ f(t,0) }[/math] gives the contribution to the heat capacity per spin, diverging as [math]\displaystyle{ t^{-\alpha}\ ; }[/math] so [math]\displaystyle{ d/y=2-\alpha\ . }[/math] Also, on the critical isotherm [math]\displaystyle{ (t=0)\ , }[/math] the second relation above gives [math]\displaystyle{ f(0,H)=\psi(0)H^{d/x}\ . }[/math] But the magnetization per spin is [math]\displaystyle{ -(\partial f/\partial H)_T\ , }[/math] vanishing as [math]\displaystyle{ H^{d/x-1}\ , }[/math] so [math]\displaystyle{ d/x-1=1/\delta\ . }[/math] The exponents [math]\displaystyle{ d/x }[/math] and [math]\displaystyle{ d/y }[/math] have thus been identified in terms of the thermodynamic exponents: the heat-capacity exponent [math]\displaystyle{ \alpha }[/math] and the critical-isotherm exponent [math]\displaystyle{ \delta\ . }[/math] In the meantime, again with [math]\displaystyle{ -(\partial f/\partial H)_T }[/math] the magnetization per spin, the homogeneity of form of [math]\displaystyle{ f(t,H) }[/math] in (20) is equivalent to that of [math]\displaystyle{ H(t,M) }[/math] in (7), from which the scaling laws [math]\displaystyle{ \gamma=\beta(\delta-1) }[/math] and [math]\displaystyle{ \alpha + 2\beta + \gamma =2 }[/math] are known to follow.

因此，由(2)得

[math]\displaystyle{ f(t,H)=t^{d/y} \phi(H^{y/x}/t)=H^{d/x}\psi(t/H^{y/x}) }[/math]，

其中 [math]\displaystyle{ \phi }[/math] 和 [math]\displaystyle{ \psi }[/math] 仅仅是 [math]\displaystyle{ H^{y/x}/t\ }[/math] 的函数。当 [math]\displaystyle{ H=0 }[/math]，由第一个关系式可得 [math]\displaystyle{ f(t,0)=\phi(0)t^{d/y}\ }[/math]。但是 [math]\displaystyle{ f(t,0) }[/math] 的两个温度导数对单位自旋热容有贡献，且以 [math]\displaystyle{ t^{-\alpha}\ }[/math]发散，所以有[math]\displaystyle{ d/y=2-\alpha\ }[/math]。另外，在临界等温线[math]\displaystyle{ (t=0)\ }[/math]上，由第二个关系式可得[math]\displaystyle{ f(0,H)=\psi(0)H^{d/x}\ }[/math]。但单位自旋磁化强度[math]\displaystyle{ -(\partial f/\partial H)_T\ }[/math]随 [math]\displaystyle{ H^{d/x-1}\ }[/math]衰减，因此 [math]\displaystyle{ d/x-1=1/\delta\ }[/math]。指数 [math]\displaystyle{ d/x }[/math] 与 [math]\displaystyle{ d/y }[/math] 可以由热容指数 [math]\displaystyle{ \alpha }[/math] 和临界等温线指数 [math]\displaystyle{ \delta\ }[/math]定义。同时再有单位自旋磁化强度[math]\displaystyle{ -(\partial f/\partial H)_T }[/math]，(20)中[math]\displaystyle{ f(t,H) }[/math] 的齐次形式与(7)式 [math]\displaystyle{ H(t,M) }[/math] 的齐次形式等价，由此得到标度律 [math]\displaystyle{ \gamma=\beta(\delta-1) }[/math] 和[math]\displaystyle{ \alpha + 2\beta + \gamma =2 }[/math]。

A related argument yields the scaling law (10) for the correlation function [math]\displaystyle{ h(r,t)\ , }[/math] with [math]\displaystyle{ H=0 }[/math] again for simplicity. In the re-scaled model, [math]\displaystyle{ t }[/math] becomes [math]\displaystyle{ L^yt\ , }[/math] as before, while [math]\displaystyle{ r }[/math] becomes [math]\displaystyle{ r/L\ . }[/math] There may also be a factor, say [math]\displaystyle{ L^p }[/math] with some exponent [math]\displaystyle{ p\ , }[/math] relating the magnitudes of the original and rescaled functions; thus,

[math]\displaystyle{ \label{eq:21} h(r,t) \equiv L^{p}h(r/L,L^yt); }[/math]

在重标度模型中，[math]\displaystyle{ t }[/math] 变为[math]\displaystyle{ L^yt\ }[/math]，[math]\displaystyle{ r }[/math] 则为 [math]\displaystyle{ r/L\ }[/math]。对于关联函数 [math]\displaystyle{ h(r,t)\ }[/math]标度律(10)，也存在某一指数 [math]\displaystyle{ p\ }[/math]使 [math]\displaystyle{ L^p }[/math] 成为联系原始模型和重标度模型的因子；所以有：

[math]\displaystyle{ h(r,t) \equiv L^{p}h(r/L,L^yt); }[/math]

(21)

i.e., [math]\displaystyle{ h(r,t) }[/math] is homogeneous of degree [math]\displaystyle{ p }[/math] in [math]\displaystyle{ r }[/math] and [math]\displaystyle{ t^{-1/y}\ . }[/math] Then from the alternative form (2) of the property of homogeneity,

[math]\displaystyle{ \label{eq:22} h(r,t)\equiv r^p G(r/t^{-1/y}) }[/math]

即 [math]\displaystyle{ h(r,t) }[/math] 是 [math]\displaystyle{ r }[/math]和 [math]\displaystyle{ t^{-1/y}\ }[/math] 的 [math]\displaystyle{ p }[/math] 次齐次函数。再由齐次性表达式(2)有：

[math]\displaystyle{ h(r,t)h(r,t)\equiv r^p G(r/t^{-1/y}) }[/math]

(22)

with a scaling function [math]\displaystyle{ G\ . }[/math] Comparing this with (10), and recalling that the correlation length [math]\displaystyle{ \xi }[/math] diverges at the critical point as [math]\displaystyle{ t^{-\nu} }[/math] with exponent [math]\displaystyle{ \nu\ , }[/math] we identify [math]\displaystyle{ p=-(d-2+\eta) }[/math] and [math]\displaystyle{ 1/y=\nu\ . }[/math] The scaling law [math]\displaystyle{ (2-\eta)\nu=\gamma\ , }[/math] which was a consequence of the homogeneity of form of [math]\displaystyle{ h(r,t)\ , }[/math] again holds, while from [math]\displaystyle{ 1/y=\nu }[/math] and the earlier [math]\displaystyle{ d/y=2-\alpha }[/math] we now have the hyperscaling law (17), [math]\displaystyle{ d\nu=2-\alpha\ . }[/math]

其中 [math]\displaystyle{ G\ }[/math]是标度函数。与(10)对比，由临界点处的关联长度服从(11)，我们可得[math]\displaystyle{ p=-(d-2+\eta) }[/math] 以及 [math]\displaystyle{ 1/y=\nu\ }[/math]。由此齐次性表达式 [math]\displaystyle{ h(r,t)\ }[/math]得出的标度律[math]\displaystyle{ (2-\eta)\nu=\gamma\ }[/math]依然成立，且再由[math]\displaystyle{ 1/y=\nu }[/math] 和 [math]\displaystyle{ d/y=2-\alpha }[/math]，得到超标度律(17) — [math]\displaystyle{ d\nu=2-\alpha\ }[/math]。

The block-spin picture thus yields the critical-point scaling of the thermodynamic and correlation functions, and both the [math]\displaystyle{ d }[/math]-independent and [math]\displaystyle{ d }[/math]-dependent relations among the scaling exponents. The essence of this picture is confirmed in the renormalization-group theory [19,20].

因此，块自旋图产生了热力学函数和相关函数的临界点标度关系，以及标度指数之间的 [math]\displaystyle{ d }[/math] 无关和 [math]\displaystyle{ d }[/math] 依赖关系。重正化群理论证实了块自旋图的本质。

References 参考文献

[1] C. Domb, The Critical Point (Taylor & Francis, 1996).

[2] M.E. Fisher, Repts. Prog. Phys. 30, part 2 (1967) 615.

[3] C. Domb and D.L. Hunter, Proc. Phys. Soc. 86 (1965) 1147.

[4] B. Widom, J. Chem. Phys. 43 (1965) 3898.

[5] L.P. Kadanoff, Physics 2 (1966) 263.

[6] A.Z. Patashinskii and V.L. Pokrovskii, Soviet Physics JETP 23 (1966) 292.

[7] R.B. Griffiths, Phys. Rev. 158 (1967) 176.

[8] B. Widom, J. Chem. Phys. 41 (1964) 1633.

[9] J.W. Essam and M.E. Fisher, J. Chem. Phys. 38 (1963) 802.

[10] O.K. Rice, J. Chem. Phys. 23 (1955) 169.

[11] R.L. Scott, J. Chem. Phys. 21 (1953) 209.

[12] C. Domb and M.F. Sykes, Proc. Roy. Soc. A 240 (1957) 214.

[13] M.E. Fisher, Physica 25 (1959) 521.

[14] E.A. Guggenheim, J. Chem. Phys. 13 (1945) 253.

[15] M.E. Fisher, J. Math. Phys. 5 (1964) 944.

[16] B. Widom, J. Chem. Phys. 43 (1965) 3892.

[17] P.G. Watson, J. Phys. C1 (1968) 268.

[18] G. Stell, Phys. Rev. Lett. 20 (1968) 533.

[19] K.G. Wilson, Phys. Rev. B 4 (1971) 3174.

[20] K.G. Wilson, Phys. Rev. B 4 (1971) 3184.

[21] K.G. Wilson and M.E. Fisher, Phys. Rev. Lett. 28 (1972) 240.